Page 1 :
\, , Second Quarter Module 8, Week 8, , 0
Page 2 :
Introductory Message, For the facilitator:, This module was collaboratively designed, developed and evaluated by the Development and, Quality Assurance Teams of SDO TAPAT to assist you in helping the learners meet the, standards set by the K to 12 Curriculum while overcoming their personal, social, and economic, constraints in schooling., As a facilitator, you are expected to orient the learners on how to use this module. You also, need to keep track of the learners' progress while allowing them to manage their own learning., Furthermore, you are expected to encourage and assist the learners as they do the tasks, included in the module., For the learner:, This module was designed to provide you with fun and meaningful opportunities for guided, and independent learning at your own pace and time. You will be helped to process the, contents of the learning resource while being an active learner., The following are some reminders in using this module:, 1. Use the module with care. Do not put unnecessary mark/s on any part of the module., Use a separate sheet of paper in answering the exercises., 2. Don’t forget to answer Let’s Try before moving on to the other activities included in the, module., 3. Read the instruction carefully before doing each task., 4. Observe honesty and integrity in doing the tasks and in checking your answers., 5. Finish the task at hand before proceeding to the next., 6. Return this module to your teacher/facilitator once you are through with it., If you encounter any difficulty in answering the tasks in this module, do not hesitate to, consult your teacher or facilitator. Always bear in mind that you are not alone., We hope that through this material, you will experience meaningful learning and gain deep, understanding of the relevant competencies. You can do it!, , 1
Page 3 :
Let’s Learn, This module was designed and written with you in mind. It is here to help you master Radical, Equations. The scope of this module permits it to be used in many different learning situations., The language used recognizes the diverse vocabulary level of students. The lessons are, arranged to follow the standard sequence of the course. But the order in which you read them, can be changed to correspond with the textbook you are now using., After going through this module, you are expected to solve equations involving radical, expressions., , Let’s Try, Directions: Read each question carefully and solve if necessary. Choose the letter of the, correct answer and write it before the number., 1. If 2√3, , = √𝑏, then b is equal to, , A. 36, 2. If √𝑥, , B.12, , C. 9, , D.4, , B. 9, , C. 27, , D. 81, , − 2 = √12, then 𝑥 is equal to, B. 10, , C. 14, , D. 144, , C. 16, , D. 64, , C. 3, , D. 4, , − 6 = 3, then (𝑥 − 6)2 equals, , A. 3, 3. If √𝑥, A.8, , 4. Solve:√𝑥, A. 4, , =8, , 5. Solve: √2𝑥, A. 1, , B. 8, , − 1 − √𝑥 + 2 = 0, B. 2, , 6. Which one of the following is a root of equation √3𝑥, A. 𝑥 = 6, B. 𝑥 = 15, C. 𝑥 = 32, 7. Solve: 2√𝑥, A. 1, , = 12?, D. 𝑥, , = 48, , −3=4, B. 3, , C. 5, , D. 7, , 2
Page 4 :
8. In what power should √2𝑥, A. 4, B. 3, , + 5 be raised to remove the radical sign?, C. 2, D. 1, , 9. Which equation has an extraneous root?, A. √2𝑥, , = −8, , B. √𝑥, , +1=4, , C. √2𝑥, , 10. Which of the following is the extraneous root of 2, A. 0, B. 1, C. 2, , −1=5, , D. √4𝑥, , = 3√ 𝑥 − 7, , = 𝑥 + √𝑥, D. 4, , https://forms.gle/SChFD4bavUV3L5V5Ac5hh9, , 3
Page 5 :
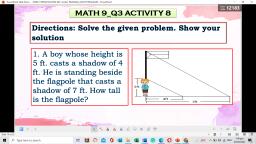
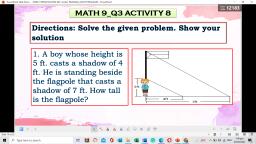
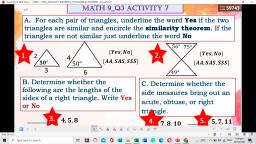
Lesson, , Equation Involving Radical, Expressions, , 8, , Let’s Recall, , 1. Evaluate:, , 2. Solve:, , a. (√25), b. (2√7), 3, , c. (√8), 3, , 2, , a. 4𝑥 − 3 = 2𝑥 + 5, , 2, , b. 2𝑥 = 7𝑥 + 5, , 3, , c. 2(𝑥 − 3) = 7(𝑥 − 1), 3, , d. 𝑥 2 − 4𝑥 − 5 = 0, , 3, , e. 0 = 3𝑥 2 + 8𝑥 + 5, , d. (√12), 3, , e. (3√2), , Let’s Explore, One theory about the construction of the ancient pyramids involves the use of special, right triangle. The Egyptians may have used wooden right triangles during the construction, process to help determine the slope. (https://mathstat.slu.edu)., , During Pythagoras’ trip to Egypt, he noticed that Egyptians has a very interesting, strategy to improve the stability of the pyramid walls. They used rope, with 12 knots tied, evenly spaced, which resulted in the famous 3-4-5 triangle forming a 90°angle. Pythagoras, became famous for the Pythagorean theorem which had been used by the ancient Egyptians, in building their famous pyramid almost 2000 years ago. (https://pythagoreantheorempyramids.tumblr.com)., , 4
Page 7 :
Checking:, if 𝑥, , Checking:, , 1, , if 𝑥 = 9, , =4, , 3 + 5√𝑥, 3 + 5√9, 3 + 5(3), 3 + 15, 18, , = 2𝑥, , 3 + 5√𝑥, , 1, 1, = 2( ), 3 + 5√, 4, 4, 1, 1, 3 + 5( ) =, 2, 2, 1, 5, =, 3+, 2, 2, 1, 11, =, , 2, 2, , = 2𝑥, = 2(9), = 18, = 18, = 18 ✓, , In solving 3 + 5√𝑥 = 2𝑥, we arrive with two solutions but upon checking only 𝑥 = 9 satisfies, 1, the radical equation. 𝑥 = 4 is not the solution and considered as extraneous root (an, extraneous root is a solution of an equation derived from original equation, however, it is not, a solution of the original equation). Thus 𝑥 = 9 is the only real solution of 3 + 5√𝑥 = 2𝑥., , Example 2: Solve and check, 3, , a. √2𝑥 + 3 = −5, , b. √2𝑥 − 1 − 3 = 0, , c. √3𝑥 + 3 − √5𝑥 − 1 = 0, , Solutions:, a., √2𝑥 + 3 = −5, 2, 2, (√2𝑥 + 3) = (−5), 2𝑥 + 3 = 25, 2𝑥 = 25 − 3, 2𝑥 = 22, 2𝑥, 22, =, 2, 2, 𝑥 = 11, , Checking:, √2𝑥 + 3 = −5, √2(11) + 3 = −5, , Square both sides, Subtract 3 to both sides, Divide both sides by 2, , √22 + 3 = −5, √25 = −5, 5 = −5 , , Upon checking, 𝑥 = 11 is an extraneous root since it does not satisfy the radical equation., Thus, √2𝑥 + 3 = −5 has no solution., b., 3, √2𝑥 − 1 − 3 = 0, 3, √2𝑥 − 1 = 3, 3, , 3, , (√2𝑥 − 1), 2𝑥 − 1, 2𝑥, 2𝑥, 𝑥, , = 33, = 27, = 27 + 1, = 28, = 14, , Checking:, 3, √2𝑥 − 1 − 3 = 0, 3, √2(14) − 1 − 3 = 0, 3, √28 − 1 − 3 = 0, 3, √27 − 3 = 0, 3−3 =0, 0 =0, , The solution is 14., 6
Page 8 :
c., √3𝑥 + 3 − √5𝑥 − 1 = 0, √3𝑥 + 3 = √5𝑥 − 1, 2, , (√3𝑥 + 3), 3𝑥 + 3, 3𝑥 − 5𝑥, −2𝑥, 𝑥, , Checking:, √3𝑥 + 3 − √5𝑥 − 1 = 0, √3(2) + 3 − √5(2) − 1 = 0, 2, , √6 + 3 − √10 − 1 = 0, √9 − √9 = 0, 3−3 = 0, 0 =0, , = (√5𝑥 − 1), = 5𝑥 − 1, = −1 − 3, = −4, =2, , The solution is 2., , Example 3: Let’s solve the problems posted in Let’s Explore., To find the height of the pyramid the formula 𝑐 2 = 𝑎2 + 𝑏 2 , where 𝑎 is the height, 𝑏 is the, length of base from the center and 𝑐 is the slanting side of the pyramid. Can you tell me what, the height of the structure is if 𝑐 = 12𝑚 and 𝑏 = 4𝑚 ?, , 𝑐2, (12)2, 144, 144 − 16, 128, √128, 8√2, , = 𝑎2 + 𝑏 2, = 𝑎2 + (4)2, = 𝑎2 + 16, = 𝑎2, = 𝑎2, = √𝑎 2, =𝑎, , Pythagorean Formula, Substitute 𝑐 = 12 and 𝑏 = 4, APE, Subtract, Get the square root of both sides, Simplify, , The height of the pyramid is 8√2 m or approximately 11.31 m., To watch a video tutorial on Solving Radical Equations by Khan Academy (2011), visit this, link https://youtu.be/pFFoAGIEyJc, , To watch a video tutorial on How to Solve Radical Equations that have Two Radicals by, PreMath (2018), visit this link https://youtu.be/8mAeeUgPMxA, , To watch a video tutorial on Solving Radical Equations by EnginerdMath (2019), visit this, link https://youtu.be/fMX2mkOyhFg, , 7
Page 9 :
Let’s Dig In, Solve each carefully. Check for a possible presence of extraneous root/s., 1., , √𝑥 = 2, √𝑥 − 7 = 0, , 2., 3., , √3𝑥 + 7 = 7, , 4., , 3 − √𝑥 − 4 = 0, , 5., , 3, , √𝑥 + 1 − 2 = 0, , Let’s Remember, In solving radical equation involving one variable, the following procedures are suggested:, •, •, •, •, •, , Isolate the radical in one side of the equation by applying properties of equality., Combine similar terms whenever possible., Get the nth root of both sides to eliminate radical signs., Solve the resulting equation., Check the solution for possible presence of extraneous root., , Let’s Apply, Solve each carefully. Check for a possible presence of extraneous root/s., 1., , √𝑥 + 5 = √2𝑥 − 3, , 2., , √𝑥 − √𝑥 − 5 = −1, , 3., , √5𝑥 2 + 7𝑥 − 2 = 𝑥√5, , 4., , √𝑥 + 5 − √𝑥 = 1, , 5., , 3, , √3𝑥 − 1 − 2 = 0, , 8
Page 10 :
Let’s Evaluate, Directions: Read each question carefully and solve if necessary. Choose the letter of the, correct answer and write it before the number., 1. Which one of the following is a root of equation √3𝑥, A. 𝑥 = 1, B. 𝑥 = 5, C. 𝑥 = 3, 2. Solve:1 + √𝑥, A. 31, , + 1 – √𝑥 + 8 = 1?, D. 𝑥 = 8, , −8=6, B. 33, , 3. In what power should, A. 4, , C. 35, , D. 37, , 3, , √2𝑥 + 5 be raised to remove the radical sign?, , B. 3, , C. 2, , D. 1, , 4. Which equation has an extraneous root?, A. √3𝑥 + 1 = 5, 2, , 5. If (3√3), , B. √𝑥 − 1 = 7, , C. √4𝑥 + 1 = 5√𝑥 − 2, , = √𝑘, then k is equal to, , A. √27, , B. 27, , 6. If √𝑥, , + 1 = 4, then (𝑥 + 1)2 equals, , A. 8, , B. 16, , 7. Solve: √3𝑥, A. 1, , C. √729, , D. 729, , C. 64, , D. 256, , C. 3, , D. 4, , − 1 − √𝑥 + 5 = 0, B. 2, , 8. Which of the following is the extraneous root of √5𝑥, A. 0, 9. If 2√𝑥, A.7, , D. √2𝑥 + 1 = −5, , B., , 1, 2, , C., , 3, 2, , + 1 = 1 + 3 √𝑥, D., , 9, 4, , − 1 = √32, then 𝑥 is equal to, , 10. Solve:√2𝑥, A. 18, , B. 9, , C. 16, , D. 32, , B. 36, , C. 72, , D. 144, , = 12, , 9