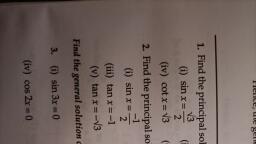Question 1 :
Solve $\cos { \left[ \tan ^{ -1 }{ \left[ \sin { \left( \cot ^{ -1 }{ x }  \right)  }  \right]  }  \right]  } $
Question 2 :
$\sin ^ { - 1 } 5 + \cos ^ { - 1 } 5 = \ldots \ldots$
Question 3 :
$\quad \sin ^{ -1 }{ x } +\sin ^{ -1 }{ \cfrac { 1 }{ x } } +\cos ^{ -1 }{ x } +\cos ^{ -1 }{ \cfrac { 1 }{ x } = } $
Question 4 :
The number of real values of x satisfying the equation $\tan^{-1}\left(\dfrac{x}{1-x^2}\right)+\tan^{-1}\left(\dfrac{1}{x^3}\right)=\dfrac{3\pi}{4}$, is?
Question 5 :
Simplify ${\cot ^{ - 1}}\dfrac{1}{{\sqrt {{x^2} - 1} }}$ for $x <  - 1$
Question 6 :
The value of $\sin^{-1} \left( \dfrac{2 \sqrt 2}{3} \right ) + \sin^{-1} \left( \dfrac{1}{3}\right )$ is equal to
Question 8 :
Consider the following statements:<br/>1. $\tan^{-1} 1+ \tan^{-1} (0.5) = \dfrac {\pi}2$<br/>2. $\sin^{-1}{\cfrac{1}{3} }+ \cos^{-1}{\cfrac{1}{3}} =\cfrac{\pi}{2}$<br/>Which of the above statements is/are correct ? 
Question 9 :
${ tan }^{ -1 }x+{ tan }^{ -1 }y={ tan }^{ -1 }\dfrac { x+y }{ 1-xy } $,      $xy<1$<br/>                                    $=\pi +{ tan }^{ -1 }\dfrac { x+y }{ 1-xy } $,      $xy>1$.<br/> Evaluate:  ${ tan }^{ -1 }\dfrac { 3sin2\alpha  }{ 5+3cos2\alpha  } +{ tan }^{ -1 }\left( \dfrac { tan\alpha  }{ 4 }  \right) $<br/>                                  where $-\dfrac { \pi  }{ 2 } <\alpha <\dfrac { \pi  }{ 2 } $
Question 10 :
Calculate the value of $\displaystyle \sin^{-1} \cos \left ( \sin^{-1} x\right ) + \cos^{-1} \sin \left ( \cos^{-1} x \right ) $. where $\displaystyle\left | x \right | \leq 1$
Question 13 :
Consider $x = 4\tan^{-1}\left (\dfrac {1}{5}\right ), y = \tan^{-1} \left (\dfrac {1}{70}\right )$ and $z = \tan^{-1}\left (\dfrac {1}{99}\right )$.What is $x$ equal to?
Question 14 :
Solve ${\cos ^{ - 1}}\left( {\frac{4}{5}} \right) + {\cos ^{ - 1}}\left( {\frac{{63}}{{65}}} \right) = $
Question 18 :
The value of $\cos^{-1} (\cos 12) - \sin^{-1} (\sin 12)$ is 
Question 19 :
Solve:$\displaystyle \sin { \left( { \tan }^{ -1 }x \right) } ,\left| x \right| <1$ is equal to
Question 23 :
${\text{If}}\;{\tan ^{ - 1}}\left( {\dfrac{a}{x}} \right) + {\tan ^{ - 1}}\left( {\dfrac{b}{x}} \right) = \dfrac{\pi }{2},{\text{then}}\;x\;{\text{is}}\;{\text{equal}}\;{\text{to}}:$
Question 24 :
The value of $ Sin^{-1}\left( cot \left( Sin^{-1}\sqrt{\frac{2-\sqrt3}{4}}+ Cos^{-1}\frac{\sqrt{12}}{4}+ Sec^{-1}\sqrt2\right)\right)$ is
Question 27 :
If $\sin ^{ -1 }{ x } +\sin ^{ -1 }{ y } +\sin ^{ -1 }{ z } =\cfrac { 3\pi }{ 2 } $, the value of$\quad { x }^{ 100 }+{ y }^{ 100 }+{ z }^{ 100 }-\cfrac { 9 }{ { x }^{ 101 }+{ y }^{ 101 }+{ z }^{ 101 } } $ is-
Question 29 :
The sum of the solution of the equation $\displaystyle 2\:sin^{-1}\sqrt{x^{2}+x+1}+cos^{-1}\sqrt{x^{2}+x}=\frac{3\pi}{2}$ is
Question 30 :
A tower stands at the top of a hill whose height is three times the height of the tower. The tower is found to subtend an angle of\$\tan ^{ -1 }{ \left( { 1 }/{ 7 } \right)  } $ at a point $2km$ away on the horizontal throught the foot of the hill. Then the height of the tower is<br/>
Question 31 :
A vertical pole more than $100ft$ high consists of two portions, the lower being $ 1/3$ of the whole. lf the upper portion subtends an angle $\tan^{-1}(1/2)$ at a point distant 40 ft. from the foot of the pole, the height of the pole is<br/>
Question 32 :
If $x_{ 1 }=2\: tan^{ -1 }\left( \frac { 1+x }{ 1-x }  \right) ,x_{ 2 }=sin^{ -1 }\left( \frac { 1-x^{ 2 } }{ 1+x^{ 2 } }  \right) $, where $x_1,x_2\:\epsilon\:(0,1)$, then $2\left( x_{ 1 }+x_{ 2 } \right) $ is equal  to
Question 33 :
$\sin ^{ -1 }{ \left( \cos { \left( \sin ^{ -1 }{ x }  \right)  }  \right)  } +\cos ^{ -1 }{ \left( \sin { \left( \cos ^{ -1 }{ x }  \right)  }  \right)  } $ is equal to
Question 34 :
The value of $ \lim_{\left | x \right |\rightarrow \infty} $ $ \cos \left ( \tan^{-1}\left ( \sin \left ( \tan^{-1} x \right ) \right ) \right ) $ is equal to :<br>
Question 35 :
The number of real solution of the equation $\displaystyle \sqrt{1+cos2x}=\sqrt{2}sin^{-1}(sin\:x),-\pi< x\leq \pi$, is
Question 36 :
There exists a positive real number $x$ satisfying $\displaystyle \cos(\tan^{-1}x)=x$. Then the value of $\displaystyle \cos^{-1}\left(\frac{x^{2}}{2}\right)$ is<br>
Question 38 :
If $\displaystyle \tan^{-1} \frac{1}{\sqrt{x^{a} - b}} = \frac{\pi}{c} - \sec^{-1} x$,$\displaystyle \left | x \right | > 1$.<br>Find the value of a,b and c.<br>
Question 39 :
If $\tan^{-1}\left ( \dfrac{x}{\sqrt{a^p-x^q}} \right )= \sin^{-1}\left ( \dfrac{x}{a} \right ),a> 0$. Find the value of p and q.<br/><br/>
Question 42 :
If$\displaystyle \tan \left ( \cos^{-1} x \right ) = \sin \left ( \cot^{-1} \frac{1}{2} \right )$, then find the value of$\displaystyle x$
Question 43 :
The value of $x$ for which $\sin { \left( \cot ^{ -1 }{ \left( 1+x \right)  }  \right)  } =\cos { \left( \tan ^{ -1 }{ x }  \right)  } $ is
Question 44 :
Assertion: If $ \displaystyle x<0, \tan^{-1}x+\tan^{-1} \frac{1}{x}=\frac{\pi}{2}$
Reason: $ \displaystyle \tan^{-1}x +\cot^{-1}x=\frac{\pi}{2} \forall x \in R$.
Question 45 :
Evaluate the following:<br>i. $\displaystyle \sin \left ( \cos^{-1}\frac{3}{5} \right )$<br>ii. $\displaystyle \cos \left ( \tan^{-1}\frac{3}{4} \right )$<br>iii. $\displaystyle \sin \left ( \frac{\pi }{2}-\sin^{-1}\left ( \frac{1}{2} \right ) \right )$<br><br>
Question 47 :
Assertion (A) : The maximum value of $f(x)=\sin^{-1}x+\cos^{-1}x+\tan^{-1}x$ is $\displaystyle \frac{3\pi}{4}$<br>Reason (R) : $\sin ^{-1} x>\cos^{-1}x$ for all $x$ in $R$<br>
Question 48 :
If $y = sec^{-1} \displaystyle \left [ \frac{x + 1}{x -1} \right ] + sin^{-1} \left [ \frac{x - 1}{x + 1} \right ]$ $z = cosec^{-1} \displaystyle \left [ \frac{2x + 3}{3x + 2} \right ] + cos^{-1} \left [ \frac{3x + 2}{2x + 3} \right ] $ then<br>
Question 49 :
If $\displaystyle { \sin }^{ -1 }\frac { x }{ 5 } +{ cosec }^{ -1 }\frac { 5 }{ 4 } =\frac { \pi  }{ 2 } $, then $x$ is equal to:
Question 50 :
If $\sin ^{ -1 }{ x } +\cot ^{ -1 }{ \cfrac { 1 }{ 2 } } =\cfrac { \pi }{ 2 } $, then $x$ is equal to-
Question 51 :
If $\cos ^{ -1 }{ \left( \cfrac { 1 }{ x } \right) } =\theta $ then $\tan { \theta } =$
Question 52 :
$\tan ^{ -1 }{ x } +2\cot ^{ -1 }{ x } =\cfrac { 2\pi }{ 3 } $, then the value of $x$ is-
Question 53 :
The value of $\sin ^{ -1 }{ \left[ \cos { \left\{ \cos ^{ -1 }{ \left( \cos { x } \right) } +\sin ^{ -1 }{ \left( \sin { x } \right) } \right\} } \right] } $, where $x\in \left( \cfrac { \pi }{ 2 } ,\pi \right) $ is equal to
Question 54 :
If $x=\sin ^{ -1 }{ K } $, $y=\cos ^{ -1 }{ K } $, $-1\le K\le 1$, then the correct relationship is-
Question 55 :
If $\displaystyle \cos \left ( 2\sin^{-1}x \right )=\frac{1}{9}$,  the value of x which satify equation is $\pm \dfrac{a}{b}$. Find the value of $a+b$<br/>
Question 56 :
Find the value of $x$ if $\sin (arc \sin x) = \dfrac {\sqrt {2}}{4}$
Question 57 :
The value of $ \tan^{-1}\left (\displaystyle\frac{1}{2}\tan 2A \right )+\tan^{-1}\left ( \cot A\right )+\tan^{-1}\left ( \cot ^{3}A\right ) $, for $ 0< A< \pi /4 $, is :<br>
Question 58 :
Assertion: If $\alpha, \beta$ are the roots of the equation $18(tan^{-1} x)^2 - 9\pi tan^{-1} x + \pi^2 = 0$ then $\alpha + \beta = \dfrac {4}{\sqrt{3}}$.
Reason: $sec^2 \left(cos^{-1} \left(\dfrac 14\right)\right) + cosec^2 \left(sin^{-1} \left(\dfrac 15\right)\right) = 41$
Question 59 :
The value of $\sin ^{ -1 }{ \left( \cos { \cfrac { 53\pi }{ 5 } } \right) } $ is
Question 60 :
If $\dfrac {(x + 1)^{2}}{x^{3} + x} = \dfrac {A}{x} + \dfrac {Bx + C}{x^{2} + 1}$, then $\csc^{-1}\left (\dfrac {1}{A}\right ) + \cot^{-1}\left (\dfrac {1}{B}\right ) + \sec^{-1}C =$ ____
Question 62 :
What is $\tan ^{ -1 }{ \left( \dfrac { 1 }{ 2 } \right) } +\tan ^{ -1 }{ \left( \dfrac { 1 }{ 3 } \right) } $ equal to?
Question 63 :
If $\sin ^{ -1 }{ \left( \cfrac { x }{ 13 } \right) } +co\sec ^{ -1 }{ \left( \cfrac { 13 }{ 12 } \right) } =\cfrac { \pi }{ 2 } $, then the value if $x$ is
Question 64 :
What is $\sin { \left[ \sin ^{ -1 }{ \left( \cfrac { 3 }{ 5 } \right) } +\sin ^{ -1 }{ \left( \cfrac { 4 }{ 5 } \right) } \right] } $ equal to?
Question 65 :
$ \sin \left( 2 \sin^{-1} \sqrt{\dfrac{63}{65}} \right) $<br/>is equal to :
Question 66 :
If two angles of a triangle are $\tan ^{ -1 }{ (2) } $ and $\tan ^{ -1 }{ (3) } $, then the third angle is
Question 67 :
The number of solutions for the equation $2\sin ^{ -1 }{ \sqrt { { x }^{ 2 }-x+1 }  } +\cos ^{ -1 }{ \sqrt { { x }^{ 2 }-x }  } =\dfrac { 3\pi }{ 2 } $ is
Question 68 :
Consider the following :<br>1. ${\sin}^{-1}\dfrac{4}{5}+{\sin}^{-1}\dfrac{3}{5}=\dfrac{\pi}{2}$<br>2. ${\tan}^{-1}\sqrt{3}+{\tan}^{-1}1=-{\tan}^{-1}(2+\sqrt{3})$<br>Which of the above is/are correct?
Question 69 :
The value of $\tan { \left[ \dfrac { 1 }{ 2 } \cos ^{ -1 }{ \left( \dfrac { 2 }{ 3 } \right) } \right] } $ is
Question 70 :
The value of $ \cos \left( \sin^{-1} \left( \dfrac {2}{3} \right) \right) $ is equal to :
Question 71 :
If $\alpha$ and $\beta$ are two real values of x which satisfy the equation $sin^{-1} x + sin^{-1} (1 - x) = cos^{-1} x$, then
Question 72 :
The number of solution of the equation $ 1+x^{2}+2x\:\sin \left ( \cos^{-1}y \right )= 0 $ is :
Question 74 :
Domain of $f(x)=\cot ^{ -1 }{ x } +\cos ^{ -1 }{ x } +co\sec ^{ -1 }{ x } $ is
Question 75 :
The value of $\displaystyle \:\sin ^{-1}\left ( \cot \left ( \sin ^{-1}\sqrt{\frac{2-\sqrt{3}}{4}}+\cos ^{-1} \frac{\sqrt{12}}{4}+\sec ^{-1}\sqrt{2}\right ) \right ) $ is
Question 76 :
Match the entries of Column - I and Column - II.<br><table class="wysiwyg-table"><tbody><tr><td></td><td>Column - I</td><td></td><td>Column - II</td></tr><tr><td>a</td><td>x = $cosec^{2}$ $(cot^{-1} 3)$ - $sec^{2}$ $(tan^{-1} 2)$</td><td>p</td><td>x = 2</td></tr><tr><td>b</td><td>$tan^{-1}$ x + $tan^{-1}$ $\dfrac{1}{y}$ = $(tan^{-1} 3)$ and $y^{2}$ + y - 56 = 0</td><td>q</td><td>x = 5</td></tr><tr><td>c</td><td>$cos^{-1}$ x = $tan^{-1}$ y and $y^{2}$ = 3</td><td>r</td><td>$x = \dfrac{1}{2}$</td></tr><tr><td>d</td><td>$sin^{-1}$ $\left (tan \dfrac{\pi}{4} \right )$ - $sin^{-1}$ $\sqrt{\dfrac{3}{y}}$ = $\dfrac{\pi}{6}$ and $x^{2}$ = y<br></td><td>s</td><td>x = - $\dfrac {1}{2}$</td></tr></tbody></table>
Question 77 :
Let $a, b, c$ be a positive real numbers $\theta = \tan^{-1} \sqrt{\dfrac{a(a + b +c)}{bc}} + \tan^{-1} \sqrt{\dfrac{b(a + b+ c)}{ca}} + \tan^{-1} \sqrt{\dfrac{c(a + b + c)}{ab}}$, then $\tan \theta$<br>
Question 78 :
$\tan { ^{ -1 }\left( 3/5 \right) } +\tan { ^{ -1 }\left( 1/4 \right) } =$
Question 79 :
The value of<b></b>$\left( {{{\tan }^{ - 1}}\pi + {{\tan }^{ - 1}}\left( {\frac{1}{\pi }} \right)} \right) + {\tan ^{ - 1}}\sqrt 3 - {\sec ^{ - 1}}( - 2)$ is equal to
Question 80 :
If $a<\cfrac { 1 }{ 32 } $, then the number of solutions of ${ \left( \sin ^{ -1 }{ x } \right) }^{ 3 }+{ \left( \cos ^{ -1 }{ x } \right) }^{ 3 }=a{ \pi }^{ 3 }$, is<br>
Question 81 :
Let $\displaystyle f:A\rightarrow B$ be a function defined by $\displaystyle y=f(x)$ where f is a bijective function, means f is injective (one-one) as well as surjective (onto), then there exist a unique mapping $\displaystyle g:B\rightarrow A$ such that $\displaystyle f(x)=y$ if and only if $\displaystyle g(y)=x\forall x \epsilon A,y \epsilon B $ Then function g is said to be inverse of f and vice versa so we write $\displaystyle g=f^{-1}:B\rightarrow A[\left \{ f(x),x \right \}:\left \{ x,f(x) \right \}\epsilon f^{-1}] $when branch of an inverse function is not given (define) then we consider its principal value branch.<br/><br/>If $\displaystyle -1<x<0$,then $\displaystyle \tan^{-1}x $ equals?<br/>
Question 82 :
The value of $a$ for which $\displaystyle ax^{2}+sin^{-1}(x^{2}-2x+2)+cos^{-1}(x^{2}-2x+2)=0$ has areal solution is
Question 85 :
$\displaystyle \sum _{ k=1 }^{ k=n }{ \tan ^{ -1 }{ \frac { 2k }{ 2+{ k }^{ 2 }+{ k }^{ 4 } }  }  } =\tan ^{ -1 }{ \left( \dfrac { 6 }{ 7 }  \right)  } $, then the value of '$n$' is equal to
Question 86 :
If $sin^{-1} \left( tan \dfrac {17\pi} {{4}}  \right )- sin^{-1} \left ( \sqrt{ \dfrac {3}{x}} \right ) - \left (\dfrac {\pi}{6} \right ) = 0$, then x is a root of the equation
Question 87 :
If $3\cos ^{ -1 }{ x } +\sin ^{ -1 }{ x } =\pi $, then $x=.....$
Question 88 :
The value of $sin^{-1} x + cos^{-1} x (|x| \geq 1)$ is
Question 89 :
<b>Statement I :</b>  The equation $(sin^{-1}x)^3+(cos^{-1}x)^3-a\pi^3=0$ has a solution for all $a\geqslant \dfrac {1}{32}.$<br/><b>Statement II :</b> For any $x\epsilon R, sin^{-1}x+cos^{-1}x=\dfrac {\pi}{2}$ and $0\leq (sin^{-1}x-\dfrac {\pi}{4})^2\leq \dfrac {9\pi^2}{16}$.<br/>