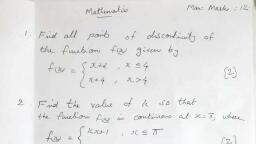Question 1 :
If $f(x)=\displaystyle \frac{\sin x}{x},x\neq 0$ is to be continuous at ${x}=0$ then $\mathrm{f}({0})=$<br/>
Question 2 :
If $ f\left( x \right) =\begin{cases} 1 & x<0 \\ { x }^{ 2 } & x\ge 0 \end{cases}$ then at $x=0$
Question 3 :
The function $f\left( x \right)=\left[ x \right] ,$  at ${ x }=5$ is:<br/>
Question 5 :
Function $f(x) = \left\{\begin{matrix}(\log_{2}2x)^{\log_{x} 8};& x\neq 1\\(k - 1)^{3};& x = 1\end{matrix}\right.$ is continuous at $x = 1$, then $k =$ _______.<br>
Question 6 :
The integer $'n'$ for which $\mathop {\lim }\limits_{x \to 0} \dfrac{{\cos 2x - 1}}{{{x^n}}}$ is a finite non-zero number is
Question 7 :
$f(x)=\left\{\begin{matrix} 2x-1& if &x>2 \\ k & if &x=2 \\  x^{2}-1& if & x<2\end{matrix}\right.$is continuous at $x= 2$ then $k =$
Question 8 :
If $f(x) = \begin{cases}\dfrac{x^2-(a+2)x+a}{x-2} & x\ne 2\\ 2 & x = 2 \end{cases}$ is continuous at $x = 2$, then the value of $a$ is
Question 9 :
Following function is continous at the point $x=2$ <br/>          $f\left( x \right) = \left\{ \begin{array}{l}1 + x,\,\,\,\,when\,\,x < 2\\5 - x,\,\,\,\,when\,\,x \ge 2\end{array} \right.$
Question 10 :
If $f(x) = (1 + 2x)^{\frac{1}{x}}$, for $x \neq 0$ is continuous at $x = 0$, then $f(0) = $_______.
Question 11 :
The function $f(x)=\dfrac{1-\sin x+\cos x}{1+\sin x+\cos x}$ is not defined at $x=\pi$. The value of $f(\pi)$ so that $f(x)$ is continuous at $x=\pi$ is
Question 12 :
If as continuous function 'f' satisfies the realation <br/>$\int_{0}^{t}(f(x) \, - \, \sqrt{f^1(x)})dx \, = \, 0 \, and \, f(0) \, = \, {-1}$<br/>the f(x) is equal to
Question 13 :
If $f\left( x \right) =\dfrac { 3sinx-sin\left( 3x \right) }{ { 2x }^{ 3 } } ,x\neq 0,f\left( 0 \right) =2,$ at $x=0,f$ is
Question 14 :
<br/>The function $f(x)=\begin{cases}(1+x)^{\frac{5}{x}}& for \ {x}\neq 0,\\ e^{5} & for \  {x}=0\end{cases}$ is ........ at ${x}=0$<br/>
Question 15 :
If $f(x)=\displaystyle\frac{\log(1+ax)-log(1-bx)}{x}$ for $x\neq 0$ and $f(0)=k$ and $f(x)$ is continuous at $x=0$, then $k$ is equal to:<br>
Question 16 :
If the function $\displaystyle f(x)=\begin{cases} 2x^{2}-3 & \text{ if } 0<x\leq 1 \\ x^{2}+bx-1 & \text{ if } 1<x<2 \end{cases}$ is continuous at every point of its domain then the value of $b$ is 
Question 17 :
Evaluate : $\lim _ { x \rightarrow \infty } \left( 1 + \dfrac { \lambda } { x } + \dfrac { \mu } { x ^ { 2 } } \right) ^ { 2 x } = e ^ { 4 } \text { then } \lambda = ( \mu \in \mathbf { R } )$
Question 18 :
Consider the piecewise defined function$\begin{cases} \sqrt{-x} , & \mbox{if } x< 0 \\ 0, & \mbox{if } 0\leq x\leq 4 \\ x-4, & \mbox{if } x> 4\end{cases}$.Choose the answer which best describes the continuity of this function-<br>
Question 19 :
$f$ is a continuous function in $[a,b]$and $g$ is a continuous function $h(x)$ is defined as<br>$h(x)=\begin{cases} f(x),\quad x\in [a,b) \\ g(x),\quad x\in (b,c] \end{cases}$<br>Now, if $f(b)=g(b)$, then
Question 20 :
<br>lf $f(x)=<br>\left\{\begin{matrix}(1+|\sin x|)^{\displaystyle \frac{a}{|\sin x|}}&-\displaystyle \frac{\pi}{6}<x<0\\b&x=0 \\e^{\displaystyle \frac{\tan 2x}{\tan 3x}} &0<x<\displaystyle \frac{\pi}{6}\end{matrix}\right.$ is<br><br>continuous at $\mathrm{x}=0$ then<br>
Question 21 :
Let $f: R \rightarrow R$ be defined by $f(x) = \left\{\begin{matrix}\alpha + \dfrac {\sin [x]}{x}&if\ x > 0\\ 2& if\ x = 0\\ \beta + \left [\dfrac {\sin x - x}{x^{3}}\right ]& if\ x < 0\end{matrix}\right.$<br>where $[x]$ denotes the integral part of y. If f is continuous at $x = 0$, then $\beta - \alpha =$
Question 22 :
If $\displaystyle f\left( x \right)=\frac { 1 }{ 2 } x-1$, then on the interval $[0,\pi]$
Question 23 :
The value of $f\left ( 0 \right )$ so that the function$\displaystyle f\left ( x \right )=\frac{1-\cos \left ( 1-\cos x \right )}{x^{4}}$is continuous everywhere is
Question 24 :
At which point the function $f\left( x \right) =\dfrac { { x }^{ 2 } }{ \left[ x \right]  }$, where $ \left[ \cdot  \right] $ is greatest integer function, is discontinuous?
Question 25 :
If $f(x) = \left\{\begin{matrix} x + 1&, x \leq 1\\ 3 - ax^{2} &, x > 1\end{matrix}\right.$ is continuous at $x = 1$, then the value of $a$ is.<br/>
Question 26 :
If $f(x)=\cfrac { { e }^{ { x }^{ 2 } }-\cos { x }  }{ { x }^{ 2 } } $, for $x\ne 0$ is continuous at $x=0$, then value of $f(0)$ is
Question 27 :
Let $f(x) =\dfrac{\sqrt{sgn(\alpha x^2+\alpha x+1)}}{\cot^{-1}(x^2-\alpha)}$. If $f(x)$ is continuous for all $x\in R$, then number of integer in the range of $\alpha$, is<br>[Note : sgn k denotes signum function of k.]
Question 28 :
If $f(x)=\left\{\begin{matrix} \displaystyle\frac{1-\cos 4x}{x^2}, & when x < 0\\ a, & when x=0 \\ \displaystyle\frac{\sqrt{x}}{\sqrt{(16+\sqrt{x})}-4}, & when x> 0\end{matrix}\right.$ is continuous at $x=0$, then the value of a will be.<br>
Question 29 :
If $f(x) = \begin{cases}\dfrac{(1-\sin^3x)}{3\cos^2x},&x<\dfrac{\pi}{2}\\\quad a, & x = \dfrac{\pi}{2} \\\dfrac{b(1-\sin x)}{(\pi-2x)^2},& x > \dfrac{\pi}{2} \end{cases}$ is continuous at $x=\dfrac{\pi}{2}$, then the value of $\left(\dfrac{b}{a}\right)^{5/3}$ is
Question 30 :
If $f(x)=\begin{cases} \sin { x } \quad if\quad x\le 0 \\ { x }^{ 2 }+{ a }^{ 2 }\quad if\quad 0<x<1 \\ bx+2\quad if\quad 1\le x\le 2 \\ 0\quad if\quad x>2 \end{cases}$ is continuous on $R$, then $a+b+ab=$
Question 31 :
lf the function $\mathrm{f}({x})=\begin{cases}\dfrac{\sin 3x}{x} &(x\neq 0) \\ \dfrac{k}{2}&(x=0) \end{cases}$ is continuous at ${x}=0$, then ${k}$ is:<br/>
Question 32 :
If the function $f(x)=\begin{cases} { \left( \cos { x } \right) }^{ 1/x } \\ k,\quad \quad x=0 \end{cases},\quad x\neq 0$ is continuous at $x=0$ then the value of $k$ is-
Question 33 :
Select and write the most appropriate answer from the given alternatives for question :<br/>If $f(x)=1-x$, for $0 < x \le 1=k$ for $x=0$ is continuous at $x=0$, then $k$ =_____.
Question 34 :
The function $f(x)=\cfrac { \tan { \left\{ \pi \left[ x-\cfrac { \pi }{ 2 } \right] \right\} } }{ 2+{ \left[ x \right] }^{ 2 } } $, where $[x]$ denotes the greatest integer $\le x$, is
Question 35 :
If $f(x) = \begin{cases} \cfrac{\sin{x}}{x} & x \le 0 \\ {k -1} & x > 0 \end{cases}$ is continuous at $x = 0 $ then the value of $k$ is-<br/>
Question 36 :
Let $f\left( x \right)$ be a continuous function whose range is $\left[ 2,6,5 \right]$. If $h\left( x \right) =\left[ \dfrac { \cos { x } +f\left( x \right) }{ \lambda } \right]$, $\lambda \in N$ be continuous, where $\left[ . \right]$ denotes the greatest integer function, then the least value of $\lambda $ is
Question 37 :
Let $f\left( x \right) =\begin{cases} { \left( x-1 \right) }^{ \dfrac { 1 }{ 2-x } },\quad x>1,\quad x\neq 2 \\ k\quad \quad \quad \quad \quad ,x=2 \end{cases}$<br>The value of $k$ for which $f$ is continuous at $x = 2$ is<br>
Question 38 :
Let $f\left( x \right)  = \dfrac { 1-\tan { x }  }{ 4x-\pi  } , x \neq  \pi /4and x \in \left[ 0, \pi /2 \right]  = \lambda , x= \pi /4$ if $f\left( x \right)$ is continuous in $\left( 0, \pi /2 \right) , then\lambda $ then
Question 39 :
If $f(x)=\begin{cases} x+2,\quad \quad when\quad x<1 \\ 4x-1,\quad when\quad 1\le x\le 3 \\ { x }^{ 2 }+5,\quad when\quad x>3 \end{cases}$, then correct statement is-
Question 40 :
The number of continuous functions $f : [0, 1] \rightarrow\mathbb{R}$ that satisfy $\int_{0}^{1} xf(x) dx = \dfrac {1}{3} + \dfrac {1}{4} \int_{0}^{1} (f(x))^{2} dx$ is
Question 41 :
The function $y = f(x)$ is defined by $x = 2t - |t|, y =t^2+t |t|, t\in R$ in the interval $x\in [-1,1]$ then<br>
Question 42 :
If $\displaystyle f\left( x \right)=\begin{cases} \begin{matrix} \dfrac { x\log { \cos { x }  }  }{ \log { \left( 1+{ x }^{ 2 } \right)  }  } , & x\neq 0 \end{matrix} \\ \begin{matrix} 0, & x=0 \end{matrix} \end{cases}$ then 
Question 43 :
The function of $f(x)=\left[ x \right] $ where $\left[ x \right] $ the greatest integer function is continuous at
Question 44 :
If $f(x)$ is continuous in $[0, 1]$ and $\displaystyle f\left ( \dfrac{1}{3} \right )=1$ then $\displaystyle \lim_{n\to \infty }f\left ( \frac{n}{\sqrt{9n^{2}+1}} \right )$ is
Question 45 :
The function $f(x)=\begin{cases} 0,&  \text{x  is irrational }\\  1,& \text{x is rational }\end{cases}$ is
Question 46 :
If $f(x)=\begin{cases} mx-1,\quad x\le 5 \\ 3x-5,\quad x>5 \end{cases} $ is continuous then value of m is:
Question 47 :
The value of $f$ at $x = 0$ so that function $f(x) = \dfrac {2^{x} - 2^{-x}}{x}, x \neq 0$, is continuous at $x = 0$, is
Question 48 :
If f is defined by $f(x) = \left\{\begin{matrix} x, for \ 0 \le x < 1  \\ 2 - x, for \ x \ge 1\end{matrix}\right.$ , then at $X = 1$, is Discuss the nature of the function
Question 49 :
If $f(x)=\begin{cases} \cfrac { x(1+a\cos { x } )-b\sin { x } }{ { x }^{ 3 } } ,\quad x\neq 0 \\ 1,\quad \quad \quad \quad x=0 \end{cases}$ then $f$ is continuous for values of $a$ and $b$ given by-
Question 50 :
Let $[x]$ denote the integral part of $\displaystyle x\in R,\> g(x)=x-[x]$. Let $f(x)$ be any continuous function with $f(0)=f(1)$, then the function $h(x)=f(g(x))$:
Question 51 :
Consider the function<br>$f(x)=\begin{cases}-2\sin x & if & x\le -\dfrac{\pi}{2} \\ A\sin x+B & if & -\dfrac{\pi}{2} < x < \dfrac{\pi}{2} \\ \cos x & if & x \ge \dfrac{\pi}{2}\end{cases}$<br>which is continuous everywhere.<br>The value of A is
Question 52 :
If $f(x)=\dfrac{1}{1-x},$ the the point of discontinuity of the function $f[f\{f(x)\}]$ is /are
Question 53 :
Find $m$ and $n$ it few$ = \left\{ \begin{array}{l}{x^2} + mx + n\,\,\,0 \le x \subset 2\\4x - 1\,\,\,\,\,\,\,\,\,\,\,\,\,\,2 \le x \subset 4\\4{x^2} + 17n\,\,\,\,\,\,4 \le x \subset 6\end{array} \right.$ is continous function
Question 54 :
If $f(x) = \left\{\begin{matrix}\dfrac {A + 3\cos x}{x^{2}},& if\ x < 0\\ B\tan \left (\dfrac {\pi}{[x + 3]}\right ),& if\ x \geq 0\end{matrix}\right.$ Where $[.]$ represents the greatest integer function, is continuous at $x = 0$ Then.<br>
Question 55 :
If $f(x)=\left\{\begin{matrix}mx +1, &x \leq \frac{\pi}{2} \\  sin \, x+n, & x >\frac{\pi}{2}\end{matrix}\right.$ is continuous at $x=\dfrac{\pi}{2}$, then<br/>
Question 56 :
If $f(x) = \left\{\begin{matrix} \dfrac {e^{3x} - 1}{4x}& for & x\neq 0\\ \dfrac {k + x}{4} & for &x = 0 \end{matrix}\right.$ is continuous at $x = 0$, then $k =$<br/>
Question 57 :
If $f(x) = \begin{cases}bx^{2}-a;\>x < -1\\ ax^{2}-bx-2;\>x\geq -1 \end{cases}$<br/>If $f$ and $f '$ are continuous everywhere, then the equation whose roots are $a$ and $b$ is:
Question 58 :
Given, $f(x)=\begin{cases} \cfrac { \tan { 4x } \times \cos { 3x }  }{ x }& x\neq 0 \\\quad\quad \quad k& x=0 \end{cases}$. If $f$ is continuous at $x=0$, then $k=$ ____
Question 59 :
Let $f\left ( x \right )=x-1$ and $g\left ( x \right )=\dfrac{1}{2}$. Then the set of points where $g(f(g(x)))$ is continuous is
Question 60 :
If $f(x) = \dfrac {x}{1 + x} + \dfrac {x}{(1 + x)(1 + 2x)} + \dfrac {x}{(1 + 2x)(1 + 3x)} + ....\infty$, then
Question 61 :
If the function $\displaystyle f\left( x \right)=\begin{cases} \begin{matrix} 1+\sin { \dfrac { \pi }{ 2 } x } , for & -\infty <x\le 1 \end{matrix} \\ \begin{matrix} ax+b, for & 1<x<3 \end{matrix} \\ \begin{matrix} 6\tan { \dfrac { \pi x }{ 12 } } ,for & 3\le x<6 \end{matrix} \end{cases}$ is continous in the interval $(-\infty,6)$ then the value of $a$ and $b$ are?
Question 62 :
If $ f\left ( x \right )=\dfrac{\left ( a+x \right )^{2}\sin \left ( a+x \right )-a^{2}\sin a}{x},x\neq 0$, the value of $f(0)$ so that f is continuous at $x = 0$ is<br>
Question 63 :
Assertion: If $f\left( x \right)=sgn\left( x \right) $ and $g\left( x \right)=x\left( 1-{ x }^{ 2 } \right) ,$ then $fog\left( x \right) $ and $gof\left( x \right) $ are continuous everywhere 
Reason: $fog\left( x \right) =\begin{cases} \begin{matrix} -1, & x\in \left( -1,0 \right) \cup \left( 1,\infty  \right)  \end{matrix} \\ \begin{matrix} 0, &  \end{matrix}x\in \left\{ -1,0,1 \right\}  \\ \begin{matrix} 1, & x\in \left( -\infty ,-1 \right) \cup \left( 0,1 \right)  \end{matrix} \end{cases}$ and $gof\left( x \right) =0,\quad \forall x\in R$
Question 64 :
Let $f\left( x \right) =\begin{cases} { x }^{ 3 }-{ x }^{ 2 }+10x-5\quad ,x\le 1 \\ -2x+\log _{ 2 }{ \left( { b }^{ 2 }-2 \right) ,x>1 }  \end{cases}$ the set of values of $b$ for which $f\left( x \right) $ has greatest <br/>value at $x=1$ is given by:
Question 65 :
The value of $k$ for which the function$\displaystyle f\left ( x \right )=\frac{\left ( e^{x} -1\right )^{4}}{\sin \left ( \frac{x^{2}}{k^{2}} \right )\log \left \{ 1+\left ( \frac{x^{2}}{2} \right ) \right \}},x\neq 0;f\left ( 0 \right )=8$may be continuous function is<br>
Question 66 :
Let $f(x)=\cfrac { x(1+a\cos { x } )-b\sin { x } }{ { x }^{ 3 } } , x\ne 0$ and $f(0)=1$. The value of $a$ and $b$ so that $f$ is a continuous function are-
Question 67 :
Let $f(x)$ be a continuous function on $[0,4]$ satisfying $f(x)f(4-x) = 1$ <br>The value of the definite integral $\int_{0}^{4} \frac{1}{1+f(x)} \;dx $ equals
Question 68 :
If $f(x) = \left\{\begin{matrix}\dfrac {\sin 5x}{x^{2} + 2x},&x\neq 0\\ k + \dfrac {1}{2},& x = 0\end{matrix}\right.$ is continuous at $x = 0$, then the value of k is<br>
Question 69 :
Let $f(x)$ be a continuous function which satisfies$\displaystyle f(x^{2}+1)=\frac{2}{f(2^{x})-1}$ &$\displaystyle f(x)> 0 \forall x\varepsilon R$ Then$\displaystyle \lim_{x\rightarrow 1}f(x)$ is-
Question 70 :
The value of $k$ for which the function $f(x)=\left\{\begin{matrix} \left(\displaystyle\dfrac{4}{5}\right)^{\dfrac{\tan 4x}{\tan 5x}}, & 0<x<\displaystyle\dfrac{\pi}{2}\\ \displaystyle k+\dfrac{2}{5}, & \displaystyle x=\dfrac{\pi}{2}\end{matrix}\right.$ is continuous at $x=\displaystyle\dfrac{\pi}{2}$, is
Question 71 :
The function $f$ : $R/\{0\}\rightarrow R$ given by $f(x)=\displaystyle \frac{1}{x}-\frac{2}{e^{2x}-1}$ can be made continuous at $x=0$ by defining $f(0)$ as -<br>
Question 72 :
If $f(x)=\dfrac{\sin 3x+A\sin 2x+B\sin x}{x^5},x\neq 0$ then the value of $\left ( f(0)+A+B \right )$ equal to $(Considering\:  f(x)\:  to\:  be\:  continuous\:  at \: x = 0 )$<br/>
Question 73 :
If it is possible to make f(x) continuous at x = 2 then f(2) is equal to
Question 75 :
If $\displaystyle f\left( x \right)=\left[ \tan { x }  \right] +\sqrt { \tan { x } -\left[ \tan { x }  \right]  } ,0\le x<\frac { \pi  }{ 2 } $, where $[.]$ denotes thegreatest integer function, then
Question 76 :
$f(x)=\left\{\begin{array}{ll}\dfrac{(x+bx^{2})^{1/_{2}}-x^{1/2}}{bx^{3/2}} & x>0\\c & x=0\\\dfrac{\sin(a+1)x+\sin x}{x} & x<0\end{array}\right.$ is continuous at ${x}=0$, then<br/>
Question 77 :
The function $f(x) = x - |x - x^2|, -1 \leq x \leq 1$ is continuous on the interval<br>
Question 78 :
If$\displaystyle \lim_{x\rightarrow 1}f(x)$ exists finitely then$\displaystyle \lim_{x\rightarrow \infty }\left (f(x) \right )^{x}$ is equal to
Question 79 :
$\displaystyle\lim _{ x\rightarrow { 0 }^{ + } }{ \left( \left( { x }^{ { x }^{ x } } \right) -{ x }^{ x } \right) } $ is
Question 80 :
The values of $p$ and $q$ so that the function $  f(x)=      \begin{cases} { \left| 1+\sin { x }  \right|  }^{ \tfrac { p }{ \sin { x }  }  }&\cfrac { -\pi  }{ 6 } <x<0 \\ q                    &x=0 \\ { e }^{ \tfrac { \sin { 2x }  }{ \sin { 3x }  }  } &0<x<\cfrac { \pi  }{ 6 }  \end{cases}$ is continuous at $x=0$ is
Question 81 :
The function defined by<br>$f(x) = \left \{\begin{matrix} x.\sin \dfrac{1}{x} & for \,\,x \neq 0 \\ 0 & for \,\,x = 0\end{matrix}\right.$ at $x = 0$ is
Question 82 :
At $x = \dfrac {3}{2}$, the function $f(x) = \dfrac {|2x - 3|}{2x - 3}$ is
Question 83 :
Let $f: \mathbb{R}\rightarrow (0, 1)$ be a continuous function. Then, which of the following function(s) has (have) the value zero at some point in the interval $(0, 1)$?
Question 84 :
If $f(x) = \dfrac {x}{2} - 1$, then on the interval $[0, \pi]$ which one of the following is correct?
Question 85 :
Assertion: Statement -1: If $ \displaystyle f(x)=\left\{ \begin{matrix} x\cos x.\sin\left( \dfrac { 1 }{ x\cos x } \right) , & \text{whenever defined} \\ 0 & \text{otherwise} \end{matrix} \right. $, then $f(x)$ is continuous
Reason: Statement -2 : $ \displaystyle \lim_{x\rightarrow \infty }\displaystyle\dfrac{\sin x}{x}=0$
Question 86 :
If $f(x)=\displaystyle \lim_{p\to\infty }\dfrac{x^{p}g(x)+h(x)+7}{7x^{p}+3x+1};x\neq 1$ and $f(1)=7, f(x),g(x)$ and $h(x)$ are all continuous functions at $x=1$ . Then which of the following statement(s) is/are correct
Question 87 :
If $f(x)=\left\{\begin{matrix}<br/>[tan(\dfrac{\pi}{4}+x)]^{1/x} &  x\neq 0\\ <br/>k & x=0<br/>\end{matrix}\right.$ ,then for what value of $k$, $f(x)$ is continuous at $x = 0$?<br/>
Question 88 :
The function$f(x)=\cfrac { \log { \left( 1+ax \right) } -\log { \left( 1-bx \right) } }{ x } $ is not defined at $x=0$. The value of which should be assigned to $f$ at $x=0$, is
Question 89 :
Let $\displaystyle f(x)=\left \{ \frac{\log(1+x)^{1+x}}{x^{2}}-\frac{1}{x} \right \}$. Then the value of $f(0)$ so that the function $f$ is continuous is
Question 90 :
Suppose that $\displaystyle f(\dfrac{1}{2})=1$ and f is continuous on $[0, 1]$ assuming only rational value in the entire interval. The number of such function is :<br/>
Question 91 :
$\displaystyle f\left( x \right)=\begin{cases} \begin{matrix} \frac { \cos ^{ 2 }{ x } -\sin ^{ 2 }{ x } -1 }{ \sqrt { { x }^{ 2 }+4 } -2} , & x\neq 0 \end{matrix} \\ \begin{matrix} a, & x=0 \end{matrix} \end{cases}$ then the value of $a$ in order that $f(x)$ may be continuous at $x=0$ is
Question 92 :
Let $f$ be a continuous function on R such that $\displaystyle f\left ( \frac{1}{4n} \right )=(\sin \:e^{n})e^{-n^{2}}+\frac{n^{2}}{n^{2}+1}$. Then the value of $f(0)$ is
Question 93 :
Let $f(x)=(1+\sin x)^{\csc x}$, the value of $f(0)$ so that $f$ is a continuous function is
Question 94 :
Assertion(A):$f(x)=\left\{\begin{array}{ll}x^{2}\sin(\frac{1}{x}) , & x\neq 0\\0, & x=0\end{array}\right.$ is continuous at ${x}=0$<br>Reason(R): Both $h(x)=x^{2},g(x)=<br>\left\{\begin{array}{ll}\sin(\frac{1}{x}) , & x\neq 0\\0, & x=0\end{array}\right.$are continuous at $x = 0$
Question 95 :
If $\displaystyle f(x)=\frac{\sin 3x+A\sin 2x+B\sin x}{x^{5}}$ for $x\neq 0$ , is continuous at $x=0$, then
Question 96 :
If $f\left( x \right) = \left\{ {\begin{array}{*{20}{c}}{{x^\alpha }\cos \left( {\dfrac{1}{x}} \right),\,\,\,if}&{x \ne 0}\\{0\,\,\,\,\,\,\,\,\,\,\,\,,\,\,\,\,\,\,\,if}&{x = 0}\end{array}} \right.\,\,is$ continuous at $x = 0$ then 
Question 97 :
Consider $f(x) = x^2 + ax +3$ and $g(x) = x + b $ and $F(x) = \displaystyle \lim_{n \rightarrow \infty} \frac{f(x) + x^{2n} g(x)}{1 + x^{2n}}$<br/><br/>If F(x) is continuous at $x = -1$, then
Question 98 :
Which of the following is(can be)continuous at each point of its domain-
Question 99 :
<br/>Let $f(x)=\displaystyle \frac{1-\tan x}{4x-\pi},x\neq\frac{\pi}{4},x\in[0,\frac{\pi}{4}]$. lf $f(x)$ is continuous in $\left[0,\displaystyle \frac{\pi}{2}\right]$ then $f\left(\displaystyle \frac{\pi}{4}\right)$ is:<br/>