Test Details

Polynomials 2

Dec 15, 9:00 PM

120 minutes

Dec 16, 9:00 PM

100.0 marks

MCQ

50 questions

Kanu Da

Abhijit Mondal, BSC In Chemistry Honors From Ramakrishna Mission Residential College, Narendrapur. BE In Mechanical Engineering From Indian Institute Of Engineering Science And Technology, Shibpur. Expert in Mathematics, Mechanical Engineering and SCIENCE

Class Details

MATHEMATICS

Science

Basic Engineering

RRB JE, ALP, Technician

MATHEMATICS

Science

Basic Engineering

Eligible criteria for ALP and Technician - 12th pass with ITI, or Diploma /Btech in Mechanical or in Electrical. For ALP should be physically fit and eye 6/6. RRB JE - Diploma or Btech in Mechanical Engineering.

More from Kanu Da

(20)

Lecture

BEP and INVENTORY

b-sc

Mechanicalengineering

0 Likes

14 Views

Kanu Da

May 15, 2023

Lecture

Numerical TURBINE

b-sc

Mechanicalengineering

0 Likes

6 Views

Kanu Da

May 13, 2023

Lecture

Viscosity Surface Tension

b-sc

Mechanicalengineering

0 Likes

9 Views

Kanu Da

May 08, 2023

Lecture

AJM USM EDM

b-sc

Mechanicalengineering

0 Likes

8 Views

Kanu Da

May 01, 2023

Lecture

ECM

b-sc

Mechanicalengineering

0 Likes

8 Views

Kanu Da

Apr 26, 2023

Lecture

PSC JE PAPER 1

b-sc

Mechanicalengineering

0 Likes

5 Views

Kanu Da

Apr 03, 2023

Lecture

Fluid Conceptual Class

b-sc

Mechanicalengineering

0 Likes

4 Views

Kanu Da

Mar 31, 2023

Lecture

Machine Tool Last

b-sc

Mechanicalengineering

0 Likes

10 Views

Kanu Da

Mar 29, 2023

Lecture

Machine Tool _SPCT 2

b-sc

Mechanicalengineering

0 Likes

9 Views

Kanu Da

Mar 24, 2023

Lecture

Mechine Tool/SPCT 1

b-sc

Mechanicalengineering

0 Likes

10 Views

Kanu Da

Mar 20, 2023

Lecture

Heat Transfer _Radiation

b-sc

Mechanicalengineering

0 Likes

8 Views

Kanu Da

Mar 17, 2023

Lecture

Heat Transfer

b-sc

Mechanicalengineering

0 Likes

8 Views

Kanu Da

Mar 15, 2023

Study Material

Welding.pdf

b-sc

Mechanicalengineering

0 Likes

111 Views

Kanu Da

Mar 09, 2023

Lecture

AC/Refrigerator

b-sc

Mechanicalengineering

0 Likes

8 Views

Kanu Da

Mar 06, 2023

Lecture

Vapour Power Cycle

b-sc

Mechanicalengineering

0 Likes

11 Views

Kanu Da

Mar 01, 2023

Lecture

Thermodynamics /cycle 2

b-sc

Mechanicalengineering

0 Likes

10 Views

Kanu Da

Feb 27, 2023

Lecture

Thermodynamics 4/cycle 1

b-sc

Mechanicalengineering

0 Likes

12 Views

Kanu Da

Feb 22, 2023

Lecture

Thermodynamics 4

b-sc

Mechanicalengineering

0 Likes

10 Views

Kanu Da

Feb 21, 2023

Lecture

Thermodynamics/SFEE

b-sc

Mechanicalengineering

0 Likes

10 Views

Kanu Da

Feb 15, 2023

Lecture

Thermodynamics/1st Law

b-sc

Mechanicalengineering

0 Likes

9 Views

Kanu Da

Feb 13, 2023

Recommended Content

(20)

Test

Polynomials

b-tech

Pcmb

0 Likes

1 Views

Rohit PUROHIT

Apr 28, 2022

Homework

Polynomials

b-tech

Mathematics

0 Likes

5 Views

S.kumar sir

Jun 06, 2022

Test

Polynomials

b-tech

Mathematics

0 Likes

16 Views

Kanu Da

Dec 14, 2021

Test

Polynomials

b-tech

Mathematics

0 Likes

12 Views

DEEPAK KUMAR TIWARI

Mar 13, 2022

Homework

Polynomials,Polynomials - NCERT

b-tech

Mathematics

0 Likes

2 Views

DEEPAK KUMAR TIWARI

Feb 19, 2022

Homework

Polynomials

b-tech

Mathematics

0 Likes

3 Views

Sunil Vaghela

Jan 09, 2022

Test

Polynomials

b-tech

Mathematics

0 Likes

2 Views

Rakesh

Jan 30, 2022

Test

Polynomials

b-tech

Mathematics

0 Likes

1 Views

Raman Kumar ray

May 31, 2022

Homework

Polynomials

b-tech

Maths

1 Likes

10 Views

Tejesh Dixit

May 13, 2022

Test

Polynomials 3

b-tech

Mathematics

0 Likes

14 Views

Kanu Da

Dec 20, 2021

Test

Polynomials,Arithmetic Progressions

b-tech

Mathematics

0 Likes

9 Views

TIMS EDUCATION HUB

Apr 29, 2022

Test

Polynomials

b-tech

Pcmb

0 Likes

6 Views

Rohit PUROHIT

Apr 22, 2022

Test

Motion 2

b-tech

Mathematics

0 Likes

9 Views

Kanu Da

Dec 15, 2021

Test

Chapter 2

b-tech

Mathematics

0 Likes

11 Views

Pawan Sir

Nov 02, 2021

Test

Chapter 2

b-tech

Mathematics

0 Likes

19 Views

Pawan Sir

Nov 01, 2021

Test

Polynomials

b-tech

Maths

0 Likes

5 Views

Shweta

Dec 01, 2021

Test

Polynomials

b-tech

Maths

0 Likes

2 Views

Usama Shaikh

Jan 08, 2022

Test

Polynomials

b-tech

Maths

0 Likes

2 Views

Krishan Kumar

Jan 12, 2022

Test

Polynomials

b-tech

Maths9Girls

0 Likes

3 Views

Ajay Kumar

Jan 11, 2022

Test

Polynomials

b-tech

Maths

0 Likes

6 Views

Nandan sir

Feb 04, 2022

Question Text

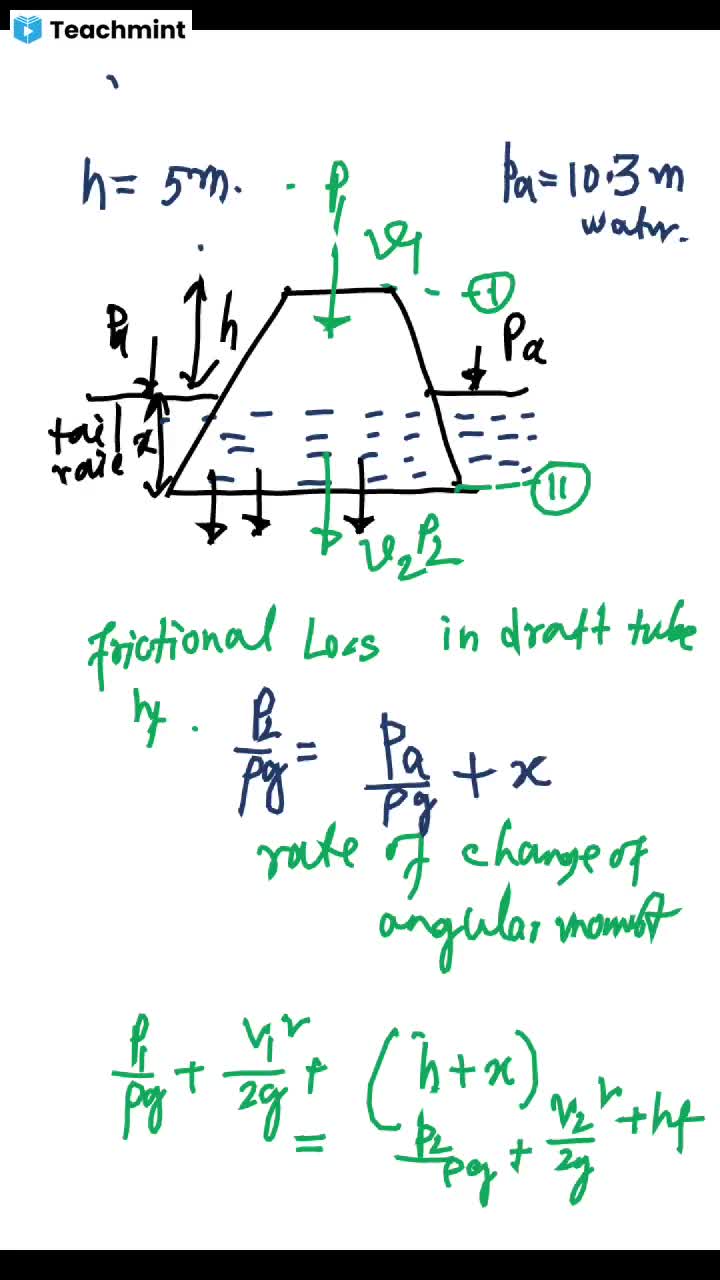
Question 1 :
When ${ x }^{ 2 }-2x+k$ divided the polynomial ${ x }^{ 2 }-{ 6x }^{ 3 }+16{ x }^{ 2 }-25x+10$ the reminder is (x+a), the value of is

Question 2 :
Calculate $\left( 6{ p }^{ 2 }+p-12 \right) \div \left( 2p+3 \right) $.

Question 4 :
What is the remainder when $(x^4+1) $ is divided by $(x-2)?$

Question 6 :
One of the factors of $(-25x^2 -1) + (1 + 5x)^2$ is

Question 8 :
If $ax^2+2a^2x+b^3$ is divisible by $x+a$, then what is the relation between $'a'$ and $'b'$ is possible?

Question 9 :
Let $r(x)$ be the remainder when the polynomial $x^{135} + x^{125} - x^{115} + x^{5} + 1$ is divided by $x^{3} - x$. Then

Question 11 :
Evaluate :$\displaystyle \frac { 50xyz\left( x+y \right) \left( y+z \right) \left( z+x \right) }{ 100xy\left( x+y \right) \left( y+z \right) }$

Question 12 :
When $p (x)$ is divided by $ax - b$ then the remainder is :

Question 13 :
$\displaystyle \left ( 1-a \right )\left ( 1+a+a^{2} \right )+\left ( 1+a \right )\left ( 1-a+a^{2} \right )$ is equal to

Question 15 :
If $p(x)$ and $g(x)$ are any two polynomials with $g(x)\neq 0$, then we can find polynomial $q(x)$ and $r(x)$ such that $p(x) = q(x) g(x) + r(x)$ where

Question 17 :
What must be added to $f(x)=4x^4+2x^3+2x^2+x-1$ so that the resulting polynomial is divisible by $g(x)=x^2+2x-3$

Question 18 :
Determine all the zeros of $x^3+5x^2-2x-10$ if two of its zeros are $\sqrt 2$ and $-\sqrt 2$

Question 20 :
The simplified value of the experession$\displaystyle \left ( a+b-c \right )^{2}+2\left ( a+b-c \right )\left ( a-b+c \right )+\left ( a-b+c \right )^{2}$ is

Question 21 :
The value of $k$ for which $x+k$ is a factor of $x^3 + kx^2 - 2x = k = 4$ is

Question 22 :
In the real number system, the equation $\sqrt { x+3-4\sqrt { x-1 } } +\sqrt { x+8-6\sqrt { x-1 } } =1$

Question 23 :
When N is divided by 4, the remainder is 3. What is the remainder when 2N is divided by 4?

Question 24 :
Factorise : ${ (ax+by) }^{ 2 }+{ (2bx-2ay) }^{ 2 }-6abxy$

Question 25 :
If $\displaystyle 2 \left ( x^{2} + 1 \right ) = 5x$, find $\displaystyle x - \dfrac{1}{x}$

Question 26 :
State whether the following statement is true or not:If $\dfrac { a }{ b } +\dfrac { b }{ a } =-1$, then ${ a }^{ 3 }-{ b }^{ 3 }=0$.

Question 29 :
Check whether the first polynomial is a factor of the second polynomial by dividing the second polynomial by the first polynomial.$t^2-3, 2t^4+3t^3-2t^2-9t-12$

Question 30 :
The value of $m$ for which $x^3 - 2mx^2 + 16$ is divisible by $(x + 2)$, is

Question 31 :
The value of $\displaystyle \left ( 5x-3y \right )^{2}-\left ( 5x+3y \right )^{2}$, when $\displaystyle x=-1$ and $y=\sqrt{\cfrac{1}{25}}$ is

Question 34 :
If $a = \dfrac{1}{3 - 2\sqrt{2}}, b = \dfrac{1}{3 + 2\sqrt{2}}$, then the value of $a^{3} + b^{3}$ is

Question 35 :
If $(x+1)$ and $(x-2)$ are the factors of the expression $(2x^3-px^2+x+q)$, then the values of $p$ and $q$ are given by:

Question 36 :
When $3x^{3} + 2x^{2} + 2x + k$ is divided by $x + 2$, the remainder is $4$. Calculate the value of $k$.

Question 38 :
If $x^4 \, + \, 2x^3 \, - \, 3x^2 \, + \, x \, - \, 1$ is divided by $x - 2$. then the remainder is

Question 39 :
Find the number of zeros in the end in product $5 ^ { 6 } \cdot 6 ^ { 7 } .7 ^ { 8 } \cdot 8 ^ { 9 } \dots 30 ^ { 31 }.$

Question 40 :
If $\displaystyle \left ( x+2 \right )$ is a factor of the polynomial $\displaystyle f\left ( x \right )= x^{2}+ax+2b$ and $\displaystyle a+b=4$, then the value of $a$ and $b$ are-

Question 41 :
(64x$^3$ + y$^3$) $\div$ (16x$^2$ - 4xy + y$^2$) is equal to

Question 42 :
Divide $\displaystyle 8\left( 3x+4 \right) \left( 8x+9 \right) $ by $\displaystyle \left( 3x+4 \right) $

Question 43 :
If ${ x }^{ 3 }-{ ax }^{ 2 }+bx-6\quad is\quad exactly\quad divisible\quad by\quad { x }^{ 2 }-5x+6.\quad then\quad \frac { a }{ b } \quad is$

Question 44 :
Workout the following divisions $a(a + 1) (a + 2) (a + 3) \div a(a + 3)$

Question 49 :
If $2x+ky+z$ is a factor of $\displaystyle 9y^{2}-z^{2}-2xz+6xy $, then the value of k is equal to

Question 50 :
The value of $k$ for which $(x - 1)$ is a factor of $x^{3} - kx^{2} + 11x - 6$ is

More from Kanu Da

Recommended Content

Learn from Anywhere on Any Device