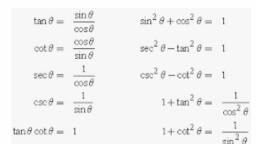Test Details

Trigonometry

Dec 04, 9:00 PM

30 minutes

Dec 04, 9:30 PM

45.0 marks

MCQ

25 questions

Ankush Kowale

skills in communication, listening, collaboration, adaptability, empathy and patience.

Class Details

Maths

Mathematics

Std 10th GURUKUL

Maths

Mathematics

In this class room we will cover entire syllabus of mathematics[Algebra+Geometry] prescribed by state board for STD 10th . There would be weekly doubt clearing session along with biweekly assignment, periodic test and many more.......

More from Ankush Kowale

(20)

Test

Algebraic Expressions and Identities

class-8th

Maths

0 Likes

28 Views

Ankush Kowale

Dec 13, 2021

Test

Differentiation

class-12th

Entrance

0 Likes

32 Views

Ankush Kowale

Dec 13, 2021

Test

Cubes and Cube Roots

class-8th

Maths

0 Likes

26 Views

Ankush Kowale

Dec 13, 2021

Lecture

Lecture 2021-12-13

class-11th

Entrance

0 Likes

28 Views

Ankush Kowale

Dec 13, 2021

Test

Differential Equations

class-12th

Entrance

0 Likes

27 Views

Ankush Kowale

Dec 12, 2021

Lecture

Lecture 2021-12-10

class-11th

Entrance

0 Likes

24 Views

Ankush Kowale

Dec 10, 2021

Test

Alcohols, Phenols and Ethers

class-12th

Entrance

0 Likes

17 Views

Ankush Kowale

Dec 10, 2021

Test

Plant Growth and Mineral Nutrition

class-12th

Entrance

0 Likes

88 Views

Ankush Kowale

Dec 09, 2021

Test

Rotational Dynamics

class-12th

Entrance

0 Likes

12 Views

Ankush Kowale

Dec 09, 2021

Test

Molecular Basis of Inheritance

class-12th

Entrance

0 Likes

19 Views

Ankush Kowale

Dec 08, 2021

Test

Halogen Derivatives

class-12th

Entrance

0 Likes

348 Views

Ankush Kowale

Dec 08, 2021

Test

Mechanical Properties of Fluids

class-12th

Entrance

0 Likes

6 Views

Ankush Kowale

Dec 08, 2021

Test

Mathematical Logic

class-11th

Entrance

0 Likes

24 Views

Ankush Kowale

Dec 07, 2021

Test

Blood Relation,Mathematical Operations

class-8th

Maths

0 Likes

20 Views

Ankush Kowale

Dec 07, 2021

Test

Chemical Thermodynamics

class-12th

Entrance

0 Likes

37 Views

Ankush Kowale

Dec 07, 2021

Test

Respiration and Circulation

class-12th

Entrance

1 Likes

991 Views

Ankush Kowale

Dec 07, 2021

Test

Applications of Derivatives

class-12th

Entrance

0 Likes

22 Views

Ankush Kowale

Dec 07, 2021

Test

Solid State

class-12th

Entrance

0 Likes

16 Views

Ankush Kowale

Dec 07, 2021

Test

Reproduction in Lower and Higher Plants

class-12th

Entrance

0 Likes

870 Views

Ankush Kowale

Dec 06, 2021

Test

Rotational Dynamics

class-12th

Entrance

0 Likes

25 Views

Ankush Kowale

Dec 06, 2021

Recommended Content

(20)

Homework

Trigonometry

class-10th

Maths

0 Likes

6 Views

Thakursachin Chauhan

Jan 21, 2022

Homework

Trigonometry

class-10th

Maths

0 Likes

0 Views

p p

Jan 28, 2022

Test

Trigonometry

class-10th

Maths

0 Likes

0 Views

ashi s

Jan 31, 2022

Test

Trigonometry

class-10th

Maths

0 Likes

2 Views

Mamta Gupta

Jan 31, 2022

Test

Trigonometry

class-10th

Maths

0 Likes

1 Views

Puja Bag

Jan 31, 2022

Homework

Trigonometry

class-10th

Maths

0 Likes

16 Views

Mastan shareef

Nov 13, 2021

Test

Trigonometry

class-10th

Maths

0 Likes

4 Views

Raja Babu

Dec 11, 2021

Test

Trigonometry

class-10th

Maths

0 Likes

8 Views

Satyajeet Pandey

Dec 20, 2021

Test

Trigonometry

class-10th

Maths

0 Likes

12 Views

shewaj khan

Dec 05, 2021

Test

Trigonometry

class-10th

Maths

0 Likes

14 Views

Unique Family

Dec 22, 2021

Test

Trigonometry

class-10th

Maths

0 Likes

2 Views

Avneesh pandey

Jan 10, 2022

Test

Trigonometry

class-10th

Maths

0 Likes

1 Views

Avneesh pandey

Jan 10, 2022

Test

Trigonometry

class-10th

Maths

0 Likes

2 Views

vicky singh

Jan 21, 2022

Test

Trigonometry

class-10th

Maths

0 Likes

0 Views

Shubham

Jan 13, 2022

Test

Trigonometry

class-10th

Maths

0 Likes

1 Views

vicky singh

Jan 21, 2022

Homework

Trigonometry

class-10th

Maths

0 Likes

8 Views

Rishabh Singh

Oct 22, 2021

Test

Trigonometry

class-10th

Maths

0 Likes

8 Views

Raj kumar

Nov 22, 2021

Test

Trigonometry

class-10th

Maths

0 Likes

1 Views

Suraj kumar

Jan 09, 2022

Test

Trigonometry

class-10th

Maths

0 Likes

9 Views

ARAFATH ET - AR-

Nov 06, 2021

Homework

Trigonometry

class-10th

Maths

0 Likes

21 Views

Mrs.Priya

Nov 13, 2021

Question Text

Question 3 :
If $\displaystyle \cos \theta =\frac{1}{2}$ then the value of $\displaystyle \frac{2\sec \theta }{1+\tan ^{2}\theta }$ is

Question 4 :
$\displaystyle \sin { \theta } +\cos { \theta } =1$ where $\displaystyle \theta= $

Question 5 :
If $cosec\,\theta=\dfrac{29}{21}$ where $0 < \theta < 90^0$, then what is the value of $4\sec\theta+4\tan\theta$ ?

Question 7 :
If $\sin\alpha + \sin\beta = a$ and $\cos \alpha - \cos \beta =b, $ then $\tan \left( \frac{\alpha -\beta}{2} \right) $ is equal to-

Question 8 :
The value of $\cos ^{ 2 }{ 73 }^{o} +\cos ^{ 2 }{ 47 }^{o} -\sin ^{ 2 }{ 43 }^{o} +\sin ^{ 2 }{ 107 }^{o}$ is equal to :

Question 9 :
If $\displaystyle \sin \theta +\sin ^{2}\theta =1$ then the value of $\displaystyle \left ( \cos ^{2}\theta +\cos ^{4}\theta \right )$ is

Question 10 :
If $\displaystyle \theta =45$ then $\displaystyle \frac { 2\tan { \theta } }{ 1+{ \tan }^{ 2 }\theta } $ is :

Question 11 :
A ladder 20 m long is placed against a vertical wall of height 10 m, determine the distance between foot of the ladder and the wall and also the inclination of the ladder with the horizontal.

Question 12 :
If $ \alpha \epsilon \left[ \frac { \pi }{ 2 } ,\pi \right] $ then the value of $\sqrt { 1+sin\alpha } -\sqrt { 1-sin\alpha } $ is equal to

Question 16 :
Choose the correct answer from the alternatives given : If $\frac{tan \theta \, + \, cot \theta}{tan \theta \, - \, cot \theta} \, = \, 2, \, (0 \leq \theta \leq 90^\circ)$ then the value of $sin \theta$ is

Question 17 :
If $\theta$ is an acute angle such that $\tan^{2}{\theta}=\dfrac{8}{7}$,then the value of $\displaystyle\frac{(1+\sin{\theta})(1-\sin{\theta})}{(1+\cos{\theta})(1-\cos{\theta})}$ is

Question 18 :
If $\displaystyle \sin { \theta } =\frac { 8 }{ 17 } $ and $\displaystyle { 90 }^{ o }<\theta <{ 180 }^{ o }$, then the value of the expression $\displaystyle \frac { 2\sin { \theta } +\cos { \theta } }{ 3\cos { \theta } +5\sin { \theta } } $ is :

Question 19 :
If $(\sec{A}-\tan{A})(\sec{B}-\tan{B})(\sec{C}-\tan{C})=(\sec{A}+\tan{A})(\sec{B}+\tan{B})(\sec{C}+\tan{C})$ represents each side of a equilateral triangle, then each side is equal to -

Question 20 :
If $A=\begin{bmatrix} \cos\theta & \sin\theta\\ -\sin\theta & \cos\theta\end{bmatrix}$ then $\displaystyle\underset{n\rightarrow \infty}{Lt}\dfrac{1}{n}|A^n|=?$

Question 21 :
${\cos ^2}{48^ \circ } - {\sin ^2}{12^ \circ }$ is equal to -

Question 22 :
If $\displaystyle \frac{\sin x}{a}= \frac{\cos x}{b}= \frac{\tan x}{c}= k,$ then $\displaystyle bc+\frac{1}{ck}+\frac{ak}{1+bk} $ is equal to

Question 23 :
If $ABCD$ is a cyclic quadrilateral such that $12$ $\tan A-5=0$ and 5 $\cos B+3=0$, then $\cos C\tan D=$

Question 24 :
If $\displaystyle \sin^4\theta+\frac {1}{\sin^4\theta}=194$, then the value of $(2 \text{cosec}\theta-\cot\theta \cos\theta)$ can be

Question 25 :
If $2 \sec 2\alpha = \tan\beta + \cot \beta$, then one of the value of $\alpha+\beta$ is-

More from Ankush Kowale

Recommended Content

Learn from Anywhere on Any Device