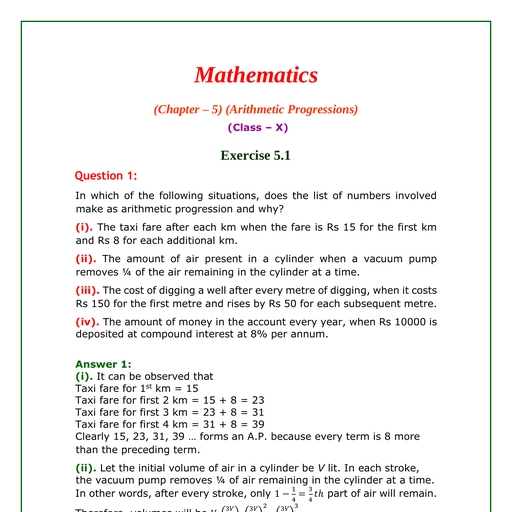
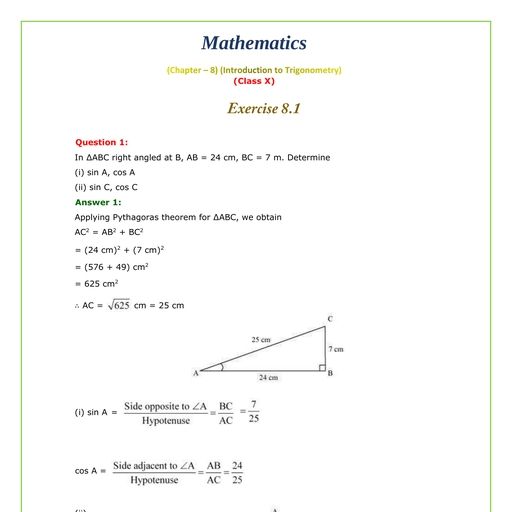
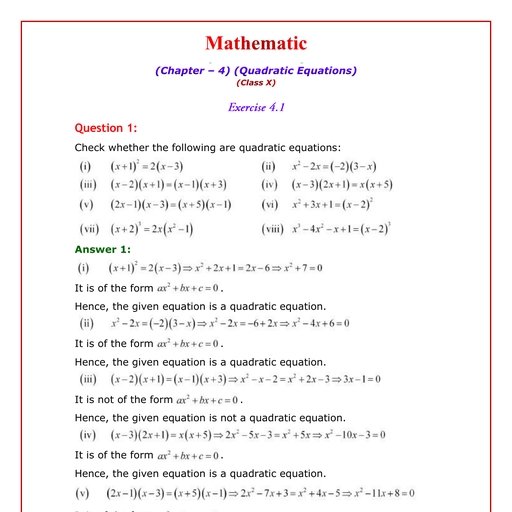
Question 1 :
The distance between the points (0, 5) and (5, 0) is<br><br>
Question 2 :
The area of triangle whose vertices are $A (-3, -1), B(5, 3)$ and $C(2, -8)$ is ____ $\text{ sq. units}$.
Question 3 :
The distance formula between two points $A(x_1, y_1)$ and $B(x_2,\,y_2)$ is given by
Question 4 :
The distance between the points $A(0, 6)$ and $B (0, 2)$ is<br/>
Question 5 :
Find the distance between the point (4, -2) and (-5, 1).
Question 6 :
Find the positive value of x if the distance between the points (x, -1) and (3, 2) is 5.
Question 7 :
If $y$ is a positive integer such that the distance between the points $(-6, -1)$ and $( -6, y )$ is $12$ units, then $y$ is equal to <br/>
Question 8 :
If the distance between the points $(8, 7)$ and $(3, y)$ is 13 what is the value of y?
Question 10 :
Area of a triangle formed by the points A(5, 2), B(4, 7) and C(7, -4) is _____.
Question 12 :
If the points (1,1) (2,3) and (5,-1) form a right-angled triangle, then the hypotenuse is of length
Question 13 :
The distance between the points $(a , b)$ and $(-1, -b)$ is 
Question 14 :
<div>Find the distance between the following pair of points.<br/></div>$(7, 8)$ and $(-2, -3)$
Question 15 :
On an xy-graph, what is the length of a line segment drawn from $(3, 7)$ to $(6, 5)$?
Question 16 :
Which one among the following points is not $10$ units away from the origin?
Question 18 :
The distance between the points $(0, 0)$ and $ (x_1, y_1) $ is _____ units.
Question 19 :
Find the distance between P and Q if P is a point on the x axis with abscissa 12 and Q is (8, 3)
Question 21 :
The distance of the point $(x_1, y_1)$ from the origin ........
Question 22 :
If the area of the triangle formed by $ (-2,5), (x,-3) $ and $(3,2)$ is $14 $ square units, then $x=$ ____.
Question 25 :
If the area of the triangle formed by the points $(-2,3), (4,-5)$ and $(-3,y)$ is 10 square units then $y =$
Question 27 :
The point on the X-axis which is equidistant from the points A(-2, 3) and B(5, 4) is
Question 29 :
Find $a$ if the distance between $(a , 2)$ and $(3 , 4)$  is $8 $.
Question 31 :
Find the distance between the points (3, -2) and (6, 4)
Question 34 :
Find the distance between the following pair of points.<br>$(2, 3)$ and $(4, 1)$.<br>
Question 35 :
The coordinates of a point on ____ are of the form $(0, y).$
Question 36 :
The area of a triangle whose vertices are $(a, c+a), (a, c) $ and $(-a, c-a) $ are 
Question 37 :
If the distance between the points $(4,y)$ and $(1,0)$ is $5$, then $y$ equals.
Question 38 :
Find the distance between the points $P(3, 2)$ and $Q(-2, -1).$
Question 39 :
<div><span>Based on this information answer the question.</span><br/><span>Given the points $A(-1,3)$ and $B(4,9)$</span><br/></div>What is the length of the line segment $AB$?
Question 40 :
<span>The distance between the given points </span><span>$K (0, -5)$ and $L (-5, 0)$ is </span>
Question 41 :
The distance between the points $A(-6, 7)$ and $B(-1, - 5)$ is.<br/>
Question 42 :
Find the area of triangle having vertices $A (6, 10), B (4, 5)$ and $C (3, 8)$ .<br/>
Question 43 :
If the point $(0, 2)$ is equidistant from the points $(3, k)$ and $(k, 5)$ then the value of $k$ is
Question 44 :
$A$ is the point on the y-axis whose ordinate is $5$ and $B$ is the point $(-3, 1)$. Calculate the length of $AB$.
Question 45 :
If the coordinates of two points A and B are $(3, 4)$ and $(5, -2)$, respectively, then the coordinates of any point P if $PA = PB$ and area of $\displaystyle \Delta PAB=10$ is
Question 46 :
Find the value of a, if distance between $A(-9,\,8)$ and $B(-5,\,a)$ is $5$ units.
Question 47 :
If the points $A(-2,3)$, $B(1,2)$ and $C(k,0)$ are collinear, then $k=$?
Question 48 :
If D (3, -1), E (2, 6) and F (5, 7) are the vettices of the sides of $\Delta DEF$, the area of triangle DEF is sq. units. 
Question 49 :
Area of triangle whose vertices are $(0, 0), (2, 3), (5, 8)$ is ____ square units.
Question 50 :
If distance between the points $(k,2)$ and (3,4) is $8$ then $k$ =
Question 52 :
If the vertices of a triangle are $(1,2),(4,-6)$ and $(3,5)$, then its area is
Question 53 :
Area of the triangle formed by the points $(0,0),(2,0)$ and $(0,2)$ is
Question 54 :
Find the area (in square units) of the triangle whose vertices are $(a, b+c), (a, b-c) $ and $(-a, c). $
Question 55 :
Find the distance between the points (2, 4) and (1, 2) on the x-y graph
Question 56 :
Find the $x$ so that the distance between the points $(-2,-3)$ and $(-3,x)$ is equal to $5$.
Question 57 :
If $A (y, 2),$ $B(1, y)$ and $AB = 5$, then the possible values of $y$ are<br/>
Question 59 :
Find the area of the triangle formed by the mid points of sides of the triangle whose vertices are $(2, 1)$, $(-2, 3)$, $(4, -3)$.
Question 61 :
The area of a triangle with vertices $(a, b + c), (b, c + a)$ and $(c, a + b)$ is<br/>
Question 62 :
Find the area of the triangle formed by joining the mid points of the sides of the triangle whose vertices are $(0.-1), (2, 1) and (0, 3)$
Question 63 :
Find the value of $a$ if area of the triangle is $17$ square units whose vertices are<span> $(0,0), (4,a), (6,4)$.                         </span>
Question 64 :
If the distance between the points $P(-3, 5)$ and $Q(-x, -2)$ is $\sqrt {58}$, then find the value(s) of $x.$
Question 65 :
Find the area of $\Delta ABC$, in which $A = (2, 1), B = (3, 4)$ and $C = (-3, -2).$
Question 66 :
The area inside the parabola $5x^2 - y = 0 $ but outside the parabola $2x^2 - y + 9 = 0$ is
Question 68 :
If ${ p }_{ 1 },{ p }_{ 2 },{ p }_{ 3 }$ are the altitudes of a triangle from its vertices $A,B,C$ and $\triangle $, the area of the triangle $ABC$, then $\frac { 1 }{ { p }_{ 1 } } +\frac { 1 }{ { p }_{ 2 } } +\frac { 1 }{ { p }_{ 3 } } $ is equal to-
Question 69 :
If the area of the triangle formed by the line -y = 0, x + y = 0 and x - c = 0 is 16 units, c is
Question 70 :
If the distance of given point $\left( \alpha ,\beta \right) $ from each of two straight lines through the origin is d, then the value of $\left( \alpha y-\beta x \right) ^{ 2 }$ is equal to
Question 71 :
If the point P (x, y) is equidistant from the points $A ( a + b , b - a )$ and $B ( a - b , a + b )$ then
Question 72 :
Area of the triangle formed by ${ (x }_{ 1 },{ y }_{ 1 })$,${ (x }_{ 2 },{ y }_{ 2 })$,<br>${ (3x }_{ 2 }-{ 2x }_{ 1 },{ 3y }_{ 2 }-{ 2y }_{ 1 })$ is
Question 73 :
Let P and Q be points $(4, 4)$ and $(9, 6)$ of parabola ${y^2} = 4a\left( {x - b} \right)$ If R be a point on the arc of the parabola between P and Q, such that the area of $\Delta PRQ$ is largest, then R is
Question 74 :
A line L passes through the points $ (1,1)  $and $ (2,0)  $ and another line $  L^{\prime}  $ passes through $ \left(\frac{1}{2}, 0\right)  $ and perpendicular to L.Then the area of the triangle formed by <span>the lines $  L, L^{\prime}  $ and $  y- $ axis, is</span>
Question 75 :
If four points are $A(6,3),B(-3,5),C(4,-2)$ and $P(x,y),$ then the ratio of the areas of $\triangle PBC$ and $\triangle ABC$ is:
Question 76 :
Length of chords of points (4,4) with respect to the circle ${ x }^{ 2 }+{ y }^{ 2 }-2x-2y-7=0$
Question 77 :
One vertex of an equilateral triangle is (2, 3) and the equation of on side is x-y+5=0. Then the equation to other sides are
Question 78 :
The area of the triangle inscribed in the parabola $y^2$ = $4x$ , the ordinates of whose vertices are 1 , 2 and 4 is :
Question 79 :
$\left( \dfrac { 1 }{ 2 } ,2 \right) $ is one extremity of a focal chord of the parabola ${ y }^{ 2 }=8x$. The co-ordinotes of the other extremity is
Question 80 :
The distance between the focii of the ellipse<br>${ \left( 3x-9 \right) }^{ 2 }+{ 9y }^{ 2 }={ \left( \sqrt { 2x } +y+1 \right) }^{ 2 }\quad is\quad -$