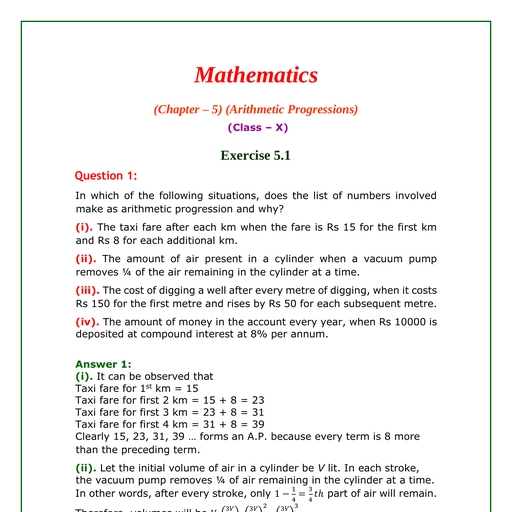
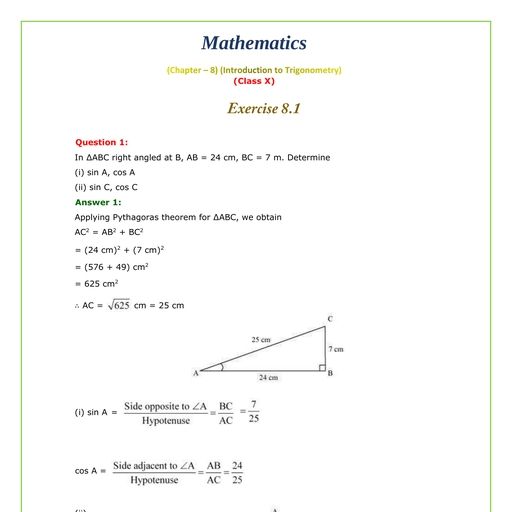
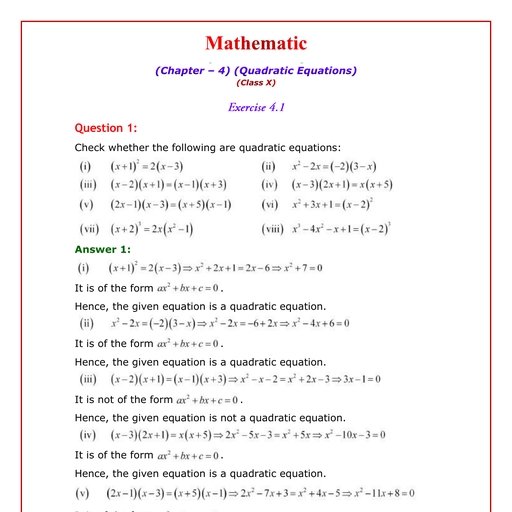
Question 1 :
A boat goes 30 km upstream and 44 km downstream in 10 hours. In 13 hours, it can go 40 km upstream and 55 km down-stream. Determine the speed of the stream and that of the boat in still water.
Question 2 :
Solve the following pair of equations by reducing them to a pair of linear equations : $6x + 3y = 6xy ; 2x + 4y = 5xy$.
Question 3 :
The cost of 2 pencils and 3 erasers is Rs. 9 and the cost of 4 pencils and 6 erasers is Rs. 18. Find the cost of each pencil and each eraser.
Question 4 :
From a bus stand in Bangalore , if we buy 2 tickets to Malleswaram and 3 tickets to Yeshwanthpur, the total cost is Rs. 46; but if we buy 3 tickets to Malleswaram and 5 tickets to Yeshwanthpur the total cost is Rs. 74. Find the fares from the bus stand to Malleswaram, and to Yeshwanthpur.
Question 5 :
Five years ago, Nuri was thrice as old as Sonu. Ten years later, Nuri will be twice as old as Sonu. How old are Nuri and Sonu?
Question 6 :
Solve the following pair of equations by reducing them to a pair of linear equations : $\frac{1}{2x} + \frac{1}{3y} = 2 ; \frac{1}{3x} + \frac{1}{2y} = \frac{13}{6}$.
Question 7 :
Places A and B are 100 km apart on a highway. One car starts from A and another from B at the same time. If the cars travel in the same direction at different speeds, they meet in 5 hours. If they travel towards each other, they meet in 1 hour. What are the speeds of the two cars?
Question 8 :
What is/ are the algebraic method/ methods that can solve a pair of linear equations?
Question 10 :
Solve the following pair of linear equations by the elimination method and the substitution method : $3x + 4y = 10 ~and ~2x – 2y = 2$
Question 11 :
If the lines are represented by the equation $a_1x + b_1y + c_1 =0$ and $a_2x + b_2y + c_2 =0$, then the lines are parallel when _____________.
Question 12 :
A pair of linear equations in two variables, which has a solution, is called a _________________ pair of linear equations.
Question 13 :
A lending library has a fixed charge for the first three days and an additional charge for each day thereafter. Saritha paid Rs. 27 for a book kept for seven days, while Susy paid Rs. 21 for the book she kept for five days. Find the fixed charge and the charge for each extra day.
Question 14 :
Find out whether the lines representing a pair of linear equations are consistent or inconsistent: $x – y = 8 , 3x – 3y = 16$
Question 15 :
For which values of p does the pair of equations given below has unique solution?$4x + py + 8 =0 ; 2x + 2y + 2 =0$
Question 16 :
One says, “Give me a hundred, friend! I shall then become twice as rich as you”. The other replies, “If you give me ten, I shall be six times as rich as you”. Tell me what is the amount of their (respective) capital?
Question 17 :
The pair of equations 5x – 15y = 8 and $3x-9y=\frac{24}{5}$ has __________.
Question 18 :
10 students of Class X took part in a Mathematics quiz. If the number of girls is 4 more than the number of boys, find the number of boys and girls who took part in the quiz. Find the solution graphically.
Question 19 :
Solve the following pair of linear equations by the elimination method and the substitution method : $x + y = 5 ~and ~2x – 3y = 4$
Question 20 :
Solve, by substitution : $x + 2y – 4 =0 , 2x + 4y – 12 =0$
Question 21 :
In the following pair of equations: 2x + y = 6 and 2x – y + 2 = 1, find the ratio of the areas of the two triangles formed by the lines representing these equations with the x-axis and the lines with the y-axis.
Question 22 :
Aftab tells his daughter, “Seven years ago, I was seven times as old as you were then. Also, three years from now, I shall be three times as old as you will be.” Which of these represent this situation algebraically?
Question 23 :
Find out whether the lines representing a pair of linear equations are consistent or inconsistent: $x + y = 5 , 2x + 2y = 10$
Question 24 :
Solve the following pair of linear equations: $152x – 378y = – 74 ; –378x + 152y = – 604$
Question 25 :
Solve the following pair of linear equations by the elimination method and the substitution method : $\frac{x}{2}+\frac{2y}{3}=-1 ~and~ x-\frac{y}{3}=3$
Question 26 :
If the lines are represented by the equation $a_1x + b_1y + c_1 =0$ and $a_2x + b_2y + c_2 =0$, then the lines are coinciding when _____________.
Question 27 :
Half the perimeter of a rectangular garden, whose length is 4 m more than its width, is 36 m. Find the dimensions of the garden.
Question 28 :
Solve the following pair of linear equations by the substitution method : $\sqrt{2}x + \sqrt{3}y =0 ; \sqrt{3}x - \sqrt{8}y =0$
Question 29 :
On comparing the ratios $\frac{a_1}{a_2]$, $\frac{b_1}{b_2}$ and $\frac{c_1}{c_2}$, find out whether the lines representing a pair of linear equations intersect at a point, are parallel or coincident: $9x + 3y + 12 = 0 ; 18x + 6y + 24 = 0$
Question 30 :
In case of infinitely many solutions, the pair of linear equations is said to be __________.
Question 31 :
The sum of the digits of a two-digit number is 9. If 27 is added to it, the digits of the number get reversed. The number is ___________.
Question 32 :
Draw the graphs of the equations x – y + 1 = 0 and 3x + 2y – 12 = 0. Determine the coordinates of the vertices of the triangle formed by these lines and the x-axis.
Question 33 :
Romila went to a stationery shop and purchased 2 pencils and 3 erasers for Rs. 9. Her friend Sonali saw the new variety of pencils and erasers with Romila, and she also bought 4 pencils and 6 erasers of the same kind for Rs. 18. Which of these represent this situation algebraically ?
Question 34 :
Is it true to say that the pair of equations – x + 2y + 2 = 0 and $\frac{1}{2}x-\frac{1}{4}y-1=0$ has a unique solution?
Question 35 :
State whether the following pair of linear equations has unique solution, no solution, or infinitely many solutions : $3x – 5y = 20 ; 6x – 10y = 40$
Question 37 :
Solve the following pair of linear equations: $px + qy = p – q ; qx – py = p + q$
Question 38 :
Solve the following pair of equations by reducing them to a pair of linear equations : $\frac{10}{x+y} + \frac{2}{x-y} = 4 ; \frac{15}{x+y} - \frac{5}{x-y} = -2$.
Question 39 :
For what values of k will the following pair of linear equations have infinitely many solutions? $kx + 3y – (k – 3) =0 ; 12x + ky – k =0$
Question 40 :
Find out whether the lines representing a pair of linear equations are consistent or inconsistent: $2x + y – 6 = 0 , 4x – 2y – 4 = 0$
Question 42 :
Solve the following pair of linear equations by the substitution method : $x + y = 14 ; x - y = 4$
Question 43 :
Do the equations 4x + 3y – 1 = 5 and 12x + 9y = 15 represent a pair of coincident lines?
Question 44 :
The area of a rectangle gets reduced by 9 square units, if its length is reduced by 5 units and breadth is increased by 3 units. If we increase the length by 3 units and the breadth by 2 units, the area increases by 67 square units. Find the dimensions of the rectangle.
Question 45 :
A pair of linear equations which has no solution, is called an __________________ pair of linear equations.
Question 46 :
The coach of a cricket team buys 3 bats and 6 balls for Rs. 3900. Later, she buys another bat and 3 more balls of the same kind for Rs. 1300. Which of these represent this situation algebraically?
Question 47 :
A train covered a certain distance at a uniform speed. If the train would have been10 km/h faster, it would have taken 2 hours less than the scheduled time. And, if the train were slower by 10 km/h; it would have taken 3 hours more than the scheduled time. Find the distance covered by the train.
Question 49 :
A part of monthly hostel charges is fixed and the remaining depends on the number of days one has taken food in the mess. When a student A takes food for 20 days she has to pay Rs. 1000 as hostel charges whereas a student B, who takes food for 26 days, pays Rs. 1180 as hostel charges. Find the fixed charges and the cost of food per day.
Question 50 :
The taxi charges in a city consist of a fixed charge together with the charge for the distance covered. For a distance of 10 km, the charge paid is Rs. 105 and for a journey of 15 km, the charge paid is Rs. 155. How much does a person have to pay for travelling a distance of 25 km?