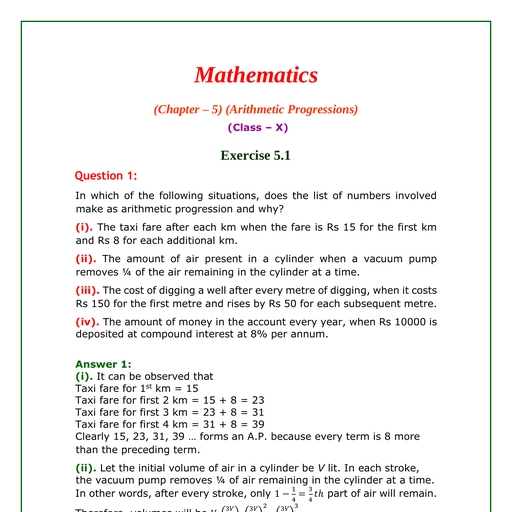
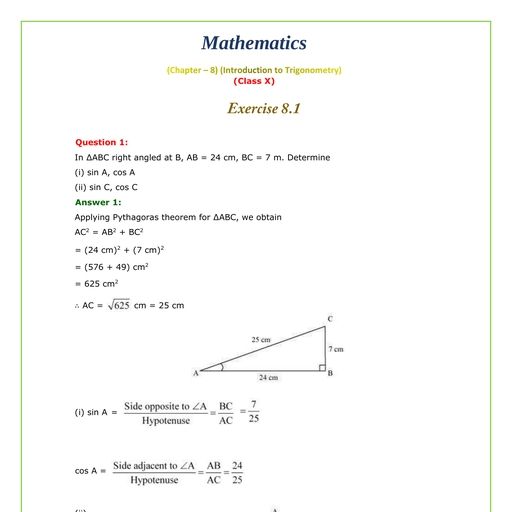
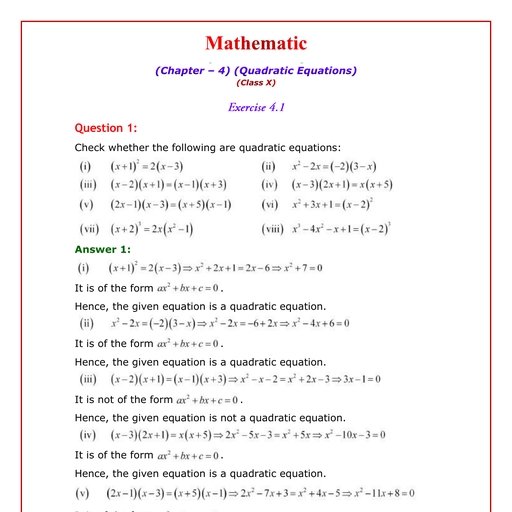
Question 1 :
Find the zeroes of the quadratic polynomial $3x^{2} + 5x - 2$.
Question 2 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b19a54273b230584979923.png' />
In the image above, the graph of $y=p\left(x\right)$, where $p\left(x\right)$ is a polynomial. Here, find the number of zeroes of $p\left(x\right)$
Question 3 :
Find the zeroes of the quadratic polynomial using the given sum and product respectively of the zeroes: $-2\sqrt{3}$, -9
Question 6 :
The number of polynomials having zeroes as -2 and 5 is:
Question 8 :
The quadratic polynomial whose sum and product of zeros being $\sqrt{2}$ and $-\frac{3}{2}$ respectively, is:
Question 9 :
State true or false: If two of the zeroes of a cubic polynomial are zero, then it does not have linear and constant terms.
Question 10 :
The zeroes of the polynomial $x^4-6x^3-26x^2-138x-35$ are $2\pm \sqrt {3}$, 7, -5.
Question 11 :
Find a quadratic polynomial, the sum and product of whose zeroes are 1 and 1, respectively.
Question 12 :
If the zeroes of the quadratic polynomial $x^2+\left(a+1\right)x+b$ are 2 and -3, then:
Question 13 :
Find all the zeros of $2x^4-3x^3-3x^2+6x-2$, if you know that two of its zeroes are $\sqrt{2}$ and $-\sqrt{2}$ .
Question 14 :
If the zeroes of $q\left(x\right)=x^3+2x^2+a$ are also the zeroes of the polynomial $p\left(x\right)=x^5-x^4-4x^3+3x^2+3x+b$ Which zeroes of $p\left(x\right)$ are not the zeroes of $q\left(x\right)$?
Question 16 :
State true or false: If the graph of a polynomial intersects the X-axis at exactly two points, it need not be a quadratic polynomial.
Question 17 :
If $x^2+2x+k$ is a factor of $2x^4+x^3-14x^2+5x+6$. Find all the zeroes of the two polynomials
Question 18 :
Find a quadratic polynomial, the sum and product of whose zeroes are 4 and 1, respectively.
Question 19 :
Find a quadratic polynomial, the sum and product of whose zeroes are $\sqrt{2}$ and $\frac{1}{3}$, respectively.
Question 20 :
Given that the $\sqrt{2}$ is a zero of the cubic polynomial $6x^3+\sqrt{2}x^2-10x-4\sqrt{2}$, find its other two zeroes
Question 21 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b19be7273b230584979a3d.png' />
Look at the graph given above. The graph of $y=p\left(x\right)$, where $p\left(x\right)$ is a polynomial. Here, find the number of zeroes of $p\left(x\right)$.
Question 22 :
State true or false: The zeroes of a polynomial p(x) are precisely the x-coordinates of the points where the graph of y = p(x) intersects the x-axis.
Question 23 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b19be6273b230584979a3c.png' />
Look at the graph given above. The graph of $y=p\left(x\right)$, where $p\left(x\right)$ is a polynomial. Here, find the number of zeroes of $p\left(x\right)$.
Question 24 :
Can the quadratic polynomial $x^2+kx+k$ have equal zeroes for some odd integer k>1?
Question 25 :
What will the quotient be on division of $ax^2+bx+c$ by $px^3+qx^2+rx+s$, $p\ne0$ ?
Question 26 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b19be3273b230584979a38.png' />
Look at the graph given above. The graph of $y=p\left(x\right)$, where $p\left(x\right)$ is a polynomial. Here, find the number of zeroes of $p\left(x\right)$.
Question 27 :
Divide the polynomial $p\left(x\right)$ by the polynomial $g\left(x\right)$ and find the quotient and remainder in the following : $p\left(x\right)$ = $x^4–5x+6$, $g\left(x\right)$ = $2-x^2$
Question 28 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b19bdf273b230584979a33.png' />
Look at the graph given above. The graph of $y=p\left(x\right)$, where $p\left(x\right)$ is a polynomial. Here, find the number of zeroes of $p\left(x\right)$.
Question 29 :
If the remainder on division of $x^3+2x^2+kx+3$ by $x-3$ is 21, find the quotient.
Question 31 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b19be3273b230584979a39.png' />
Look at the graph given above. The graph of $y=p\left(x\right)$, where $p\left(x\right)$ is a polynomial. Here, find the number of zeroes of $p\left(x\right)$.
Question 32 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b19a59273b230584979927.png' />
In the image above, the graph of $y=p\left(x\right)$, where $p\left(x\right)$ is a polynomial. Here, find the number of zeroes of $p\left(x\right)$
Question 33 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b19a50273b23058497991d.png' />
In the image above, the graph of $y=p\left(x\right)$, where $p\left(x\right)$ is a polynomial. Here, find the number of zeroes of $p\left(x\right)$
Question 34 :
Find the zeroes of the quadratic polynomial $2x^{2} - 8x + 6$.
Question 35 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b19a54273b230584979922.png' />
In the image above, the graph of $y=p\left(x\right)$, where $p\left(x\right)$ is a polynomial. Here, find the number of zeroes of $p\left(x\right)$
Question 37 :
Check whether the first polynomial is a factor of the second polynomial by dividing the second polynomial by the first polynomial: $x^2+3x+1$, $3x^4+5x^3–7x^2+2x+2$
Question 39 :
State true or false: If all three zeroes of a cubic polynomial $x^3+ax^2-bx+c$ are positive, then at least one of a, b and c is non-negative.
Question 40 :
Divide $3x^2 – x^3 – 3x + 5$ by $x – 1 – x^2$ and find the remainder and the quotient?
Question 41 :
State true or false: The only value of $k$ for which the quadratic polynomial $kx^2+x+k$ has equal zeroes is $\frac{1}{2}$.
Question 42 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b19a58273b230584979926.png' />
In the image above, the graph of $y=p\left(x\right)$, where $p\left(x\right)$ is a polynomial. Here, find the number of zeroes of $p\left(x\right)$
Question 43 :
The zeroes of $3x^4+6x^3–2x^2–10x–5$ are $\sqrt{\frac{5}{3}}$, $-\sqrt{\frac{5}{3}}$, -1 and -1. Is it true or false?
Question 44 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b19bdf273b230584979a34.png' />
Look at the graph given above. The graph of $y=p\left(x\right)$, where $p\left(x\right)$ is a polynomial. Here, find the number of zeroes of $p\left(x\right)$.
Question 45 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b19a51273b23058497991e.png' />
In the image above, the graph of $y=p\left(x\right)$, where $p\left(x\right)$ is a polynomial. Here, find the number of zeroes of $p\left(x\right)$
Question 46 :
If the zeroes of the polynomial $x^3-3x^2+x+1$ are a – b, a, a + b, then a and b are 1 and $\pm \sqrt{2}$.
Question 47 :
If on division of a non-zero polynomial $p\left(x\right)$ by a $g\left(x\right)$, the remainder is zero, what is the relation between the degrees of $p\left(x\right)$ and $g\left(x\right)$ ?
Question 48 :
If one of the zeroes of the quadratic polynomial $\left(k-1\right)x^2+kx+1$ is -3, then the value of k is:
Question 49 :
Find a quadratic polynomial, the sum and product of whose zeroes are 0 and $\sqrt {5}$, respectively.
Question 50 :
If one zero of the quadratic polynomial $x^2+3x+k$ is 2, then the value of $k$ is: