Question 2 :
The ratio of lateral strain to the linear strain within elastic limit is known as:
Question 4 :
The property of metals which allows them to be drawn readily into thin wires is:
Question 7 :
The limit upto which the stress is directly proportional to strain is called<br>
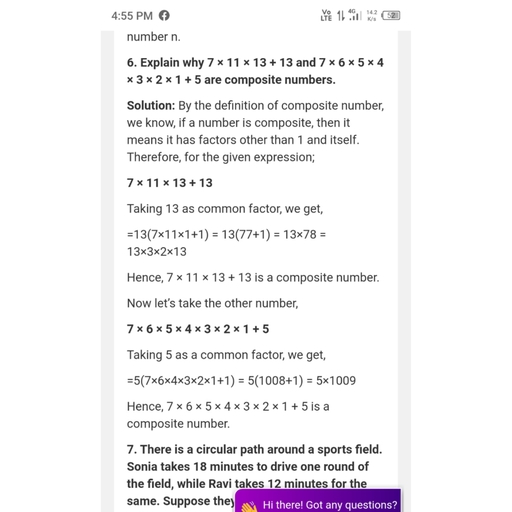
Question 8 :
The elastic energy stored per unit volume in a stretched wire is
Question 10 :
A wire whose cross-sectional area is $4\ mm^{2}$ is stretched by $0.1\ mm$ by a certain load. If a similar wire of double the area of cross-section is under the same load, then the elongation would be
Question 11 :
A wire of initial length $L$ and radius $r$ is stretched by a length $l$. Another wire of same material but with initial length $2L$ and radius $2r$ is stretched by a length $2l$. The ratio of stored elastic energy per unit volume in the first and second wire is:
Question 12 :
An elongation of $0.1$% in a wire of cross-section $10^{-6}m^{2}$ causes a tension of $100N$. $Y$ for the wire is
Question 13 :
When load is applied to a wire, the extension is $3mm.$ The extension in the wire of same material and length but half the radius extended by the same load is :
Question 14 :
Two wires of the same material have masses in the ratio 3:4. The ratio of their extensions under the same load if their lengths are in the ratio 9:10 is
Question 15 :
The Young's modulus of the material of a wire is $6\times 10^{12}$ <br> $N/m^{2}$ and there is no transverse in it, then its modulus of rigidity will be 
Question 16 :
Two wires of the same radius and material and having length in the ratio $8.9:7.6$ are stretched by the same force. The strains produced in the two cases will be in the ratio:
Question 17 :
Two wires of different material and radius have their length in ratio of $1:2.$ if these were stretched by the same force$,$ the strain produced will be in the ratio$.$  
Question 18 :
Two wires of the same material (young's modules Y) and same length L but radii $R$ and $2R$ respectively are joined end to end and a weight $W$ is suspended from the combination as shown in the figure. the elastic potential energy in the system in equilibrium is <br>
Question 19 :
The increase in energy of a metal bar of length 'L' and cross-sectional area 'A' when compressed with a load 'M' along its length is<br>(Y = Young's modulus of the material of metal bar)<br>
Question 20 :
A uniformly tapering conical wire is made from a material of Young's modulus $Y$ and has a normal, unextended length $L$. The radii, at the upper and lower ends of this conical wire, have values $R$ and $3R$, respectively. The upper end of the wire is fixed to a rigid support and a mass $M$ is suspended from its lower end. The equilibrium extended length, of this wire, would equal to:<br/>
Question 21 :
The electric current is produced by the stored water in dams,which possess:<br/>
Question 23 :
Imagine x-z plane to be fixed. A particle moving along the line 4x + 3y - 12 = 0, with a speed of 10 m/s, from the positive y-axis, approaches towards the plane and collides. If the coefficient of restitution be $e = 0.75,$ then the speed of the particle after collision will be:
Question 26 :
A tennis ball is thrown from the height h above the ground. If the ball strikes to the ground with elastic collision,what height will height will the ball achieve after the first collision?
Question 27 :
A massive ball moving with speed v collides head-on with a tiny ball at rest having a very small mass as compared to the first ball. If the collision is elastic, then immediately after the impact, the second ball will move with a speed approximately equal to 
Question 29 :
Name the type of energy (kinetic energy $K$ or potential energy $U$) possessed in the following case.The bob of a simple pendulum at its extreme position.
Question 31 :
A block having mass m collides with an another stationary block having mass $2$ m. The lighter block comes to rest after collision. If the initial velocity of first block is v, then the value of coefficient of restitution will must be?
Question 32 :
Consider the following statements<br/>A) Linear momentum of a system of particles is zero<br/>B) Kinetic energy of a system of particles is zero<br/>Then
Question 33 :
A ball P moving with a speed of $v \ ms^{-1}$ collides directly with another identical ball Q moving with a speed $10\ ms^{-1}$ in the opposite direction. P comes to rest after the collision. If the coefficient of restitution is 0.6, the value of $v$ is:
Question 34 :
A smooth sphere $A$ of mass $0.1kg$ is moving with speed $5m/s$ when it collides head on with another smooth stationary sphere of same radius. If $A$ is brought to rest by the impact and $e=\cfrac { 1 }{ 2 } $, then,<br>
Question 35 :
A bullet is fired from a riffle. If the riffle recoils freely, then the kinetic energy of the rifle will be
Question 36 :
Let $\displaystyle \vec{a},\vec{b},\vec{c}$ be vectors of length $3, 4, 5$ respectively. Let $\displaystyle \vec{a}$ be perpendicular to $\displaystyle \vec{b}+\vec{c},  \vec{b}$  to  $\displaystyle \vec{c}+\vec{a}$  & $\displaystyle \vec{c}$ to $\displaystyle \vec{a}+\vec{b}.$ Then $\displaystyle \left | \vec{a}+\vec{b}+\vec{c} \right |$ is:
Question 37 :
A bullet of mass $5g$ travels with a speed of $500ms^{-1}.$ if it penetrates a fixed target which offers a constant resistive force of 1000 N to the motion of the bullet, find :<br><br>(a) the initial kinetic energy of the bullet,<br><br>(b) the distance through which the bullet has penetrated.
Question 38 :
A particle moves in $xy\ plane.$ The position vector at any time $t$ is $\vec { r } =\left\{ \left( 2t \right) \hat { i } +\left( 2{ t }^{ 2 } \right) \hat { j }  \right\} m.$ The rate of change of $\theta$ at time $t=2\ second$ (where $\theta$ is an angle which its velocity vector makes with positive $x-axis$) is:
Question 39 :
A ray of light travelling in the direction $\dfrac{1}{2}(\hat{i}+\sqrt{3}\hat{j})$ is incident on a plane mirror. After reflection, it travels along the direction $\dfrac{1}{2}(\hat{i}-\sqrt{3}\hat{j})$. The angle of incidence is:-<br>
Question 40 :
A block of mass $m$ is suddenly released from the top of a spring of stiffness constant $k$. Given acceleration due to gravity is $=g$, then<br/>(i) the maximum compression in the spring and<br/>(ii) the compression in the spring at equilibrium respectively are <br/>