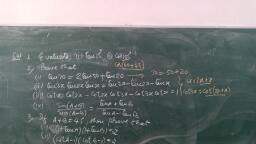Question 2 :
Change the following radian measures to degree measure:<br/>$-\cfrac { 2\pi &#160;}{ 3 } $

Question 4 :
If tan A = 4 /3, tanB = 1/ 7,then A - B =

Question 7 :
Find the value of $\dfrac{sin (-660^o) tan (1050^o) sec (-420^o)}{cos (225^o ) cosec (315^o) cos(510^o)}$

Question 10 :
Evaluate $8 \sqrt{3} \, \text{cosec}^2 30^o \, \sin \, 60^o \, \cos \, 60^o \, \cos^2 45^o \, \sin \, 45^o \, \tan \, 30^o \, \text{cosec}^3 45^o$

Question 12 :
$A+B=\cfrac { \pi }{ 3 } ;\cos { A } +\cos { B } =1$, value of $\left| \cos { A } -\cos { B } \right| $ is <br><br>

Question 13 :
&#160;If $ p=\tan 1^{0}, q=\tan 1(in\ radians) $, then which of the following is true?<br/>

Question 16 :
$ ABC $ is a triangle in which $ AB = AC = 4 $&#160;cm&#160;and $ \angle&#160;A = 90&#160;^{\circ}&#160;$. Calculate the length of perpendicular from $ A $&#160;to $ BC $.

Question 19 :
The value of&#160;$\displaystyle { \sin }^{ 2 }{ 20 }^{ o }+{ \sin }^{ 2 }{ 70 }^{ o }-{ \tan }^{ 2 }{ 45 }^{ o }$ is :

Question 20 :
Express the following angle in terms of first-quadrant reference angle:<br/>$\tan { { 336 }^{ o } } \quad $

Question 21 :
The measure of an angle in degrees, grades and radians be D, G and C respectively, then relation between them $\displaystyle \frac{D}{90}=\frac{G}{100}=\frac{2C}{\pi }$ but $\displaystyle 1^{\circ}=\left ( \frac{180}{\pi } \right )^{\circ}\:\simeq 57^{\circ},17',44.{8}''$ and sum of interior angles of a $n$-sided regular polygon is $\displaystyle \left ( 2n-4 \right )\dfrac {\pi }2$. On the basis of above information, answer the following questions :Which of the following are correct<br/>

Question 22 :
The appropriate value is $\cos { { 61 }^{ o } }$ is

Question 23 :
If A lies in the second quadrant and $3 \tan A+4=0$, the value of $2 \cot A-5 \cos A+\sin A$ is equal to

Question 24 :
If ${ x }_{ 1 }=3sin\omega t$ and ${ x }_{ 2 }=4cos\omega t$ then

Question 26 :
If $\displaystyle&#160;\frac { 3\pi &#160;}{ 4 } &lt;\alpha &lt;\pi $, then $\displaystyle&#160;\sqrt { 2\cot { \alpha &#160;} +\frac { 1 }{ \sin ^{ 2 }{ \alpha &#160;} &#160;} &#160;} $ is equal to

Question 29 :
If a is any real number, the number of roots of $\cot x - \tan x = a$ in the first quardrant is<br/><br/>

Question 30 :
If the angles of a triangle are in arithmetic progression such that $\sin (2A + B) =\dfrac 12$, then