Question 1 :
An experiment is performed to obtain the value of acceleration due to gravity g by using a simple pendulum of length L. In this experiment time for 100 oscillations is measured by using a watch of 1 second least count and the value is 90.0 seconds. The length L is measured by using a meter scale of least count 1 mm and the value is 20.0 cm The error in the determination of g would be:

Question 2 :
In the equation {tex} \mathrm { P } = \frac { \mathrm { RT } } { \mathrm { V } - \mathrm { b } } \mathrm { e } ^ { \frac { \mathrm { aV } } { \mathrm { RT } } } {/tex} {tex} \mathrm { V } = {/tex} volume, {tex} \mathrm { P } = {/tex} pressure, {tex} \mathrm { R } = {/tex} universal gas constant, and {tex} \mathrm { T } = {/tex} temperature. The dimensional formula of 'a' is same as that of

Question 4 :
It is found that {tex} | \mathrm { A } + \mathrm { B } | = | \mathrm { A } | . {/tex} This necessarily implies,

Question 5 :
The minimum number of non-zero non-collinear vectors required to produce a zero vector is<br>

Question 6 :
If {tex} \vec { a } , \vec { b } {/tex} and {tex} \vec { c } {/tex} are three unit vectors such that {tex} \vec { a } + \vec { b } + \vec { c } = 0 {/tex} then the value of {tex} \vec { a } \cdot \vec { b } + \vec { b } \cdot \vec { c } + \vec { c } . \vec { a } {/tex} is

Question 8 :
If the resultant of the vectors {tex} 3 \hat { \mathrm { i } } + 4 \hat { \mathrm { j } } + 5 \hat { \mathrm { k } } {/tex} and {tex} 5 \hat { \mathrm { i } } + 3 \hat { \mathrm { j } } + 4 \hat { \mathrm { k } } {/tex} makes an angle {tex} \theta {/tex} with {tex} x {/tex} -axis, then {tex} \cos \theta {/tex} is

Question 9 :
The sum of the magnitudes of two forces acting at a point is {tex} 18 \mathrm { N } {/tex} and the magnitude of their resultant is {tex} 12 \mathrm { N } {/tex}. If the resultant is at {tex} 90 ^ { \circ } {/tex} with the smaller force, then their magnitudes are (in newtons)

Question 10 :
How many minimum number of vectors in different planes can be added to give zero resultant?

Question 11 :
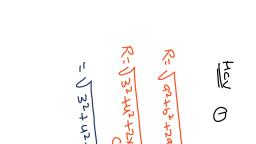
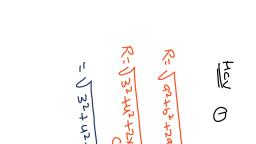
The resultant of two vectors {tex} \overrightarrow { \mathrm { A } } {/tex} and {tex} \overrightarrow { \mathrm { B } } {/tex} is perpendicular to the vector {tex} \overrightarrow { \mathrm { A } } {/tex} and its magnitude is equal to half the magnitude of vector {tex} \overrightarrow { \mathrm { B } } {/tex}. The angle between {tex} \overrightarrow { \mathrm { A } } {/tex} and {tex} \overrightarrow { \mathrm { B } } {/tex} is

Question 12 :
If {tex} \overrightarrow { \mathbf { A } } = \overrightarrow { \mathbf { B } } - \overrightarrow { \mathbf { C } } , {/tex} then, the angle between {tex} \overrightarrow { \mathbf { A } } {/tex} and {tex} \overrightarrow { \mathbf { B } } {/tex} is

Question 13 :
A particle moving with velocity {tex} \vec { v } = k ( y \hat { i } + x \hat { j } ) , {/tex} where {tex} k {/tex} {tex} = {/tex} constant. The general equation for its path is {tex} [ \mathrm { C } = \text { constant } ] {/tex}