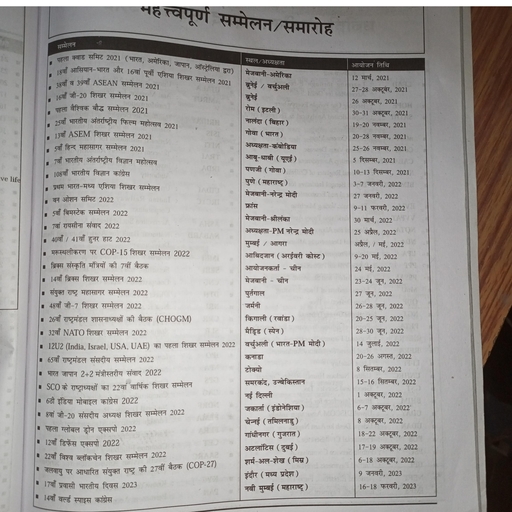
Question 2 :
<p><span class="wysiwyg-font-size-small"><span class="wysiwyg-font-size-small">A body has a charge of <font size="3">$9.6 \times 10^{-20}$</font><span class="wysiwyg-font-size-small"><span class="wysiwyg-font-size-small">coulomb. It is :</p>

Question 3 :
A charge &#34;Q&#34; and &#34;2Q&#34; are 0.05 meters apart and isolated. The ratio of the electrostatic force on the charge Q to the force on charge 2Q is:

Question 4 :
Two coils 'P' and 'Q' are separated by some distance. When a current of $3$ A flows through coil 'P', a magnetic flux of $10^{-3}$ Wb passes through 'Q'. No current is passed through 'Q'. When no current passes through 'P' and a current of $2$A passes through 'Q', the flux through 'P' is?

Question 6 :
Two positive point charges $q_1$ and $q_2$ are kept at the distance d. If the distance between them is triple. Calculate by which factor new electrostatic force is decreased.<br/>

Question 8 :
If electrical force between two charges is $200 N$ and we increase $10$% charge on one of the charges and decrease $10$% charge on the other, then electrical force between them for the same distance becomes :<br>

Question 10 :
The process of charging a conductor from a chargedbody without touching them together is calledinduction

Question 11 :
Three identical charges, each having a value $1.0 \times 10 ^ { - 3 } \mathrm { C }$are placed at the corners of an equilateral triangle of side 20 cm Find the potential at the center of the triangle

Question 13 :
<span class="wysiwyg-font-size-small"><span class="wysiwyg-font-size-small">Another unit for the quantity having the unit $\dfrac{C^2 }{Nm^2}$ is:

Question 15 :
Eight dipoles of charges of magnitude $e$ are placed inside a cube. The total electric flux coming out of the cube will be

Question 16 :
An uncharged insulator can be charged by ______ with another suitable body.<br/><br/>

Question 17 :
Two metallic spheres, one hollow and the other solid, have same diameter. The hollow sphere will hold charge

Question 19 :
An electron (of charge $-e$) revolves around a long wire with uniform charge density $\lambda$ in a circular path of radius $r$. Its kinetic energy is given by:

Question 20 :
The sum total of the number of electrons in a system may change. The statement is :<br/>

Question 21 :
State whether true or false :Electric flux through a closed surface is equal to total flux due to all the charges enclosed within that surface only.

Question 22 :
Negative electric flux indicates that electric lines of force are directed

Question 23 :
A small sphere of mass $m$ and electric charge $q$, is suspended by a light thread. A second sphere carrying a charge $q_2$ is placed directly below the first sphere at a distance $'d' $ away. Then :

Question 24 :
the number of electric field lines emerged out of 1 C charge is

Question 25 :
A plane area of $100\ {cm}^{2}$ is placed in uniform electric field of $100\ N/C$ such that the angle between area vector and electric field is $60^o$. The electric flux over the surface is

Question 27 :
Two electric dipoles of dipole moment $2 \space cm$ and $4 \space cm$ respectively are kept inside a cube of side $'a' \space m$. Total electric flux linked with the cube is (in SI units)

Question 28 :
<span class="wysiwyg-font-size-small"><span class="wysiwyg-font-size-small"><p class="wysiwyg-text-align-left">Two unlike charges attract each other with a force of 10N. If the distance between them is doubled, the force between them is :</p>

Question 29 :
Two point charges $+3\mu C$ and $+8\mu C$ repel each other with a force of $40 N$. If a charge of $-5\mu C$ is added to each of them, the force between them will become:

Question 30 :
Find out on which of following factors does strength of the force between charged particles depend.<br/>

Question 31 :
The force between two point charges placed in a material medium of dielectric constant $\varepsilon_r$ is $F$. If the material is removed, then the force between them becomes:

Question 32 :
A hollow conducting sphere of charge does not have electric field at

Question 33 :
In a region of space, the electric field is given by $\vec {E} = 8\hat {j} + 4\hat {j} + 3\hat {k}$. The electric flux through a surface of area of $100$ units in $x - y$ plane is?

Question 34 :
Deuteron and $\alpha$-particle are put $1{A}^{o}$ apart in air. Magnitude of intensity of electric field due to deuteron of $\alpha$-particle is $(N/C)$<b></b>

Question 35 :
What would happen to the electrostatic force between a pair of charged particles if both charges were tripled and the distance between them were also tripled?<br/>

Question 37 :
A charged particle is accelerated by a potential of 200V. If its velocity is $8.4\times 10^8m/s$, then value of e/m for that particle in $C/kg$ &#160;is:

Question 38 :
<span class="wysiwyg-font-size-small"><span class="wysiwyg-font-size-small"><p class="wysiwyg-text-align-left">The electric field due to a charge at a distance of $3m$ from it is $500 N/C$. The magnitude of the charge is$\left [ \dfrac{1}{4\pi \varepsilon _{0}}=9\times 10^{9}Nm^{2}/C^{2} \right ]$ :</p>

Question 39 :
Which of the following statements are true:A: Charge cannot exist without mass but mass can exist without charge<br/>B: Charge is invariant but mass varies with velocity<br/>C: Charge is conserved but mass alone may not be conserved

Question 41 :
What happens when a charged balloon is placed near another balloon of the same charge?

Question 42 :
The ratio of electric force between two electrons to two protons separated by the same distance in air is

Question 43 :
Two charges are placed at certain distance apart. A metallic sheet is placed between them. What will happen to the force between the charges?

Question 44 :
A neutral water molecules$\displaystyle \left( { H }_{ 2 }O \right)$ in its vapour state has an electric dipole moment of$\displaystyle 6\times { 10 }^{ -30 }Cm$. If the molecule is placed in an electric field of$\displaystyle 1.5\times { 10 }^{ 4 }{ NC }^{ -1 }$, the maximum torque that the field can exert on it is nearly:

Question 45 :
What is the angle between the electric dipole moment and the electric field due to it on the axial line?

Question 46 :
A charge is kept at the centre of a shell. Shell&#160;has charge Q uniformally distibuted over its surface and radius R. The force on the central charge due to the shell is :

Question 48 :
If $\oint _{ s }^{&#160; }{ \overrightarrow { E } .\overrightarrow { d } s } =0$ over surface, then

Question 49 :
A charge $q_1$ exerts a force of $45N$ on a charge of $q_{2}=10^{-5} C$ located at a point $0.2m$ from $q_{1}$. The magnitude of $q_{1}$ is

Question 50 :
The electric field intensity at a point near and outside the surface of a charged conductor of any shape is '${ E }_{ 1 }$'. The electric field intensity due to uniformly charged infinite thin plane sheet is '${ E }_{ 2 }$'. The relation between '${ E }_{ 1 }$' and '${ E }_{ 2 }$' is :

Question 51 :
The sum of all electromagnetic forces between different particles of a system of charged particles is zero:<br/>

Question 52 :
The smallest electricforce between two changes placed at a distance of 1 m apart is (approximately)

Question 53 :
A cube of side $10$ cm enclose a charge of $0.1\mu C$ at its center. Calculate the number of lines of force through each face of the cube.

Question 54 :
A flat, square surface with sides of length L is described by the equations. $x = L, 0 \leq y \leq L, 0 \leq z \leq L$<br/>The electric flux through the square due to a positive point charge q located at the origin($x = 0, y = 0, z=0 $) is<br/><br/>

Question 55 :
Two lithium nuclei in lithium vapour at room temperature do not combine to form a carbon nucleus because

Question 56 :
Two charges 'Q' and 'Q' are kept at a distance 'd'. The charge 'q' is kept at the midpoint of line joining the charges. The magnitude of 'q' to keep the system in equilibrium is:

Question 57 :
A dipole is placed in a uniform electric field with its axis parallel to the field. It experiences :

Question 58 :
Two charges are placed a certain distance apart in air. If a brass plate is introduced between them, the force between them will

Question 59 :
Three charges, each of value $Q$, are placed at the vertices of an equilateral triangle. A fourth $q$ is placed at the centre of the triangle. if the charges remains stationary, then $q$ is

Question 60 :
A point charge $Q_{1}$ exerts some force on a second point charge $Q_{2}$. If a 3rd point charge $Q_{3}$ is<br/>brought near, the force of $Q_{1}$ exerted on $Q_{2}$(Without changing their respective positions):<br/>

Question 61 :
An electric charge is held at rest in a region of space filled with non-uniform magnetic field. As soon as it is left free.

Question 62 :
The total number of lines of force passing through a surface is called

Question 64 :
Two charged particles having charge $2\times 10^{-8}C$ each are joined by an insulating string of length $100\ cm$. The system is kept on a horizontal table. Find the tension in the string.

Question 65 :
Two point charges $+q$ and $-q$ are placed at a distance x apart. A third charge is placed in such a way so that all the three&#160;charges are in equilibrium. Then :

Question 66 :
Two charges $9e$ and $3e$ are placed at a separation $r$. The distance of the point where the electric field intensity will be zero, is-

Question 67 :
<img style='object-fit:contain' src="data:image/png;base64,iVBORw0KGgoAAAANSUhEUgAAANsAAADWCAYAAABYHfeeAAAABHNCSVQICAgIfAhkiAAAIABJREFUeF7tnQncfdXUx0/oNWbMFOofUaYM0agkFM3mKc2RyNBIKiRJqRSlSFLKkKGBRBo1Z4o0kT9lLBlCZvdd3/32O+++++5z75nvfZ5nr8/nfO49++xh7bX32sPaa6+1xMAgS5AokCjQOQXu0nkJqYBEgUQBR4HEbKkjJAr0RIHEbD0ROhWTKJCYLfWBRIGeKJCYrSdCFxWDfCrJqIqoM7/CE7NNuT3POeec7LrrrpsyFqn4PiiwRBL990HmVEaiQJJGpj6QKNAbBdIysjdSp4IWOgUSsy30HpDq3xsFErP1RupU0EKnQGK2hd4DUv17o0Bitt5InQpa6BRIzLbQe0Cqf28USMzWG6lTQQudAonZFnoPSPXvjQKJ2XojdSpooVMgMdtC7wGp/r1RIDFbb6ROBS10CiRmW+g9INW/NwokZuuN1KmghU6BxGwLvQek+vdGgcRsvZE6FbTQKZCYbaH3gFT/3iiQmK03UmfZf//737H2RpZaaqlsiSWWaPX50Ic+5MoM7ZzEwnokxYIsKplF6LHZV1999ezyyy/vscT/KwoGDpntj3/8Y3bf+97XMXaCfiiQZrYW6bzhhhuOnZXKMNq1116b/ec//8ne/e53u5nwC1/4gsNw2WWXdQyz1157VWaQkNHI7/73v392l7vcxeWlX/6vscYaI4zZIokWdlYY/ElQTAHr+APr9AP98p/n3//+9+CnP/0pfhJqP1tssUVxwS1+Ad8TTjihFp5f+cpXXH19EA0IE11aRHfeZpWWkRPGWmt5F4NZZu+9984OPPDAoRSxJRoR/HBmDmPOoXTkq/3ZBBQaf1YdVKaf4frrr59985vfdLMbdSwDN9xwQ7bCCivkUZmJ73a3u5VJuqDjJGa7s/npiHQ2Oh3gd0z+P+Yxj8l+9rOfjXSWkNnUaV/4whdmZ5555kj8WQygfkcddVT2pje9qRA9v55hnW2GzxYtWuRopvr3NZAUIjyDHxKz3dkoMNovfvGL7NJLL83YN/3zn/90s5hmBaLBcI985CPzPRPfHvKQh2RPeMITXC68b7bZZtlTnvKUnGnpdLMO4O0PLrfffnt22GGH5Wjz7cYbb8x++ctfurC//e1vQ4Ie6rjNNttkj3rUo9z3Bz/4wdlaa62VPfWpT531qveLnxFyQYL2Xey9XvSiFw2sw7g9jX757z+bb7750L4Novl7l/lMxLCe7NP03P3ud3c0K6Lbhz/8YUeaMI/5TK+iui2Ymc0IMDR6M6Rpych/LY38vQthf/rTn7L73Oc+lSWA/Q6Z0y9tnXXWyb71rW/ldBQ9hdnVV1+drwD0jd+FBAtC9M+SUAx15ZVXOia7613vOsRAMCPAcpKlIb88idHKscMFF1zgBrODDjrIJRA9lfpJT3qSo/unPvWpnLblcp5HsYqmvPkSruXLKaecUij6tk7gvr397W8fEXPPFzr0WQ9oft555xUuLddcc838+ETLUdLMd5j3y8gNNtggO/vss/MlZDjiIhBZbbXV8uGT7/7ych6Nq71VBRqGy0hmtmuuuWYIB1YXD33oQ7Obb765t2OQ3ogQKWjeLSNpaB4kYjDNN77xjXxJo07AMlHxnvnMZzqy0Dl45iujqb7HHHPMyBIv0i8aBWkvJpryy57tec973tDSnfO5X/3qV9mSSy7plvW33Xab055h+T4fYV4wmzrSP/7xj+yWW25xIuff/e53Q52KBkVFiY38b37zm7wtw73bXGhkOqPqrN9JeGumMenhpKidfKd8Vhgw03Oe8xy3FxaoPptuumn25z//OfvXv/7lGK5s3TpBuItMrUJzHr773e8O7EB2sPTSS4/syxDZv/Wtbx0sXrzYqVhpDzeXK00dtttuu8Hyyy8/MK3+UlVRvaddf5VvTOXU3exMc/DmN795wL6Zx5hy8KAHPWjw8pe/3NXtkksuKVW/uRBpzurYMPJx8HrxxRe75cdPfvITN5v5cOihh2bPetazsnvf+97uMJpZbD4AswSzsw0g2R/+8IdSVdLSrlTkDiMJD2Y22oVZbMcdd3SzHXu6d77zne64BY+sP/jBD5wWzllnneUwmpU61CXPnGW2q666Ktttt92G6v0///M/GUtJwEa6ujRJ6XqiAG3E8n6llVbKVlxxRad9c8UVV2SnnXaaG0B5cIFsK5aRgbQnFFstZs7t2WggHtb3IbzqVa/KgxgFwyeMn97bpYAta7Pdd9+9dKYIo9RG+v+lL30p22mnnYYEVb///e/d+9///vd8L1e6kBmKOCeYDeZCmkXDQHQe9BiBgw8+ON9IH3/88TNE2maoaFCJ5RLO2orL0loQxonlM4thtO2RRx7pBCnUYd99983RvOc97+m2AhdeeKELm2t1nBPMxr7kyU9+8sia/aSTTsp22WWXWewzreBkeoUjszMDzve///2cFnQ4EzBkLKslWSUO+565DtSNS7ThXnzdddd1+z0UpucUWIVmDnytAv6/4x3vyKWMSKwY1Ey8Py8ki0XER2p3xBFHjGhhqP76hRbGXCPKwEgrkfSRT1sQSjSVN7+006JFiwa2j86LI8yPUxcX9Yd73eteru2pL7/bb7/9iHJ4W3XtIp+ZFJAwMltls/PPPz/74Ac/OHQvjKXSG9/4xuy9732vG9SIR/z5BtSL+2Vrr712puUxSywOhjmoR4jA/bp3vetdTpeTC50+Ld7//vdn97jHPVonC2XYre8MGyYAOHJx1NThRmYaZp5PfvKTrn3sVnpmIv1a+FAmdf/LX/6S7b///hkH81z3OfbYYzM79sn22GOP7GUve9ns94MuOLhJnox+RsiBqVENTDqVj2ImtRo8+tGPHtgSYnDHHXc0KaKTtODNOd6tt946+Pa3vz0w0Xyrs0qI9EYbbeRGd2O28FNn79TRND4GD3/4w/N2McYfnHvuuYMnPvGJDp9XvvKV7p3Hlvn5iuTrX/96a+3GudzjH//4gTG5y3/llVce2D5u8POf/9y1wawCo+FMAA3J84hHPCJvIC2PHvCAB8wEjuOQEP7bbrutO5yFGbqEaTDbpPqEy8hJ8Zt8h6lsZhtZZtvZXKeDXBOcZ0pA8p73vMfpygmsYplp4mc2W9RafvSZCFyBj3/8427JlKBbCrCstIEtQ0gmYLlqs5yTXM8iTJXZ6KDswXh+/etfZ/vtt18uzoWYH/nIR9wafS5ofoAvja3fLhsbegGUp/1tl+WVyZu9ZcxGS5m0deKo7rZszbhL5wMMh9aJ37/qlNF2mqkz2xlnnOGYCXUqzQ5UksNN1Hjmo/CjjUZEQMJZ1D777NNGdnM6D1TyoAUDtgCDSwx8hx9++Mycx02V2T72sY9lL37xix1RfEbjjhkGT2dVIx9c0cnU6AqeP/zhD10ddthhByet47/qxExEnUyptpVbytBLtOljJi3Diaqr345l0jWNo9UEdEAq65/HQiM7Nsre8pa3DLVH0zJrpzfi9A4SJpj5tFwYYhVw/2+66abe8alToDHbCO7gb40fDVf9+C2rqV8HryZpaBeB2qhKfpyHciY2LQBns2wWpb/ZxpwqbtAEjp8KUHkbjRxh1EFNW2DqBClDDBrVjNuMNOrXvvY1d9BKfVQ3n8kIM3PiZYqYShwdQovRfOabhBBxST9N0btwsNltZNCzu3RTl1L2ymzqpCb0GNiU7zorv9xdsoPKqRNjUoca910d9bOf/ezAbn8Pjj766DlXH9snuzbBPJ3teVrDX7TpYtaLDQyEURcGPrP16epkTkScjRkGRPDoApdx/YNvvTIbFTSDpvlsZgIQ16AakSYhO8vfVQfUpGjcNjtrX/UWs5mwKleDalo2dOGcFJpwAN42nHzyyQMTkAwxj9+n7KbA4KMf/ejIKoQD8L6hNwGJVczZBZHRF9MEcSavJWRgg9sngA9iY+5P8d8aKBdo1MXDF1Z0KbjA7AP52+zZGOdYXSV0aEMS7OeBXwEJj2Ll1g276KKLssc+9rF5cr9Pccfx9a9/fbbeeusNZY+KGe3O0xd01sNVCX7/+te/Ov057iXNCtAg3BLGshbHDG0wBwxrs7erYhsdtYhW5A1d6+Dst4vyD8OoRwh+3cJvZd+Fd5e0KcLFL5P/pqnklCX6xKUzZlOl0QjhCnzYgL4t+SICdR2uw/I2CK48UI7Fh9rpp5/e2ajZBF+N5ve73/2Gji64piNAyZcy/Icb1Vx3qTsTkJftl7Itt9yydh51+wM4o9mj+qgOTehYB5dOmY1KbbLJJiN4mVJq9H7aSMSOA9BUbxOYaXje97735Q3bZv5t5EUHo12478YAqBmLw18t5XGQoW8bb7yxqwvv+l4HD8rUTNxnJ6csyl1kXna4cOwPFtis5KJq3QGkKh06vWLz+c9/Pvve976X40TFueSIwc6+KlhEEJZ7L33pS51nz2njUoRjl+GxDg9NNPrrO5oqYlD/t0vcusgb3O22glvma79GOVwRYvXFANk1dDKz0Xn1UAE1IDYd7SqGe28ySlYhihjJx0lhPg6M3D7OYR2qlNlW3BBnvYsRwD8WR3WJ4TGO9n5+SqvZyP+N5UvYZz7zmSFmVVnctOc/AhL1hfBXbRLLm5va/qzop5XSNz7iwjx5D91+EQZ9QvVAyh2HQwyvqmGtMpsaHk19jIFigIepGsEIQhIuD1LZvsHuv7llk/9w+VHLSPyNoYdJg6pRTVztDMxMC7ASFuKsd3kxhaaEIWkT7vxi6HQaYOelGbSGnnp4xzgufcNcBg998+ON6xf0G+rqx+e/ifRztTUsdIXfeQ8tsEEXcMH04Qc+8AFHpgMOOMBZ+WLL0yXDtbqMhGBo7jPCqUPQ+BB7WgBOdp3ePT6wnEBTHTdHGJKhUV796lfnURCecNN5XCfosk4MVkXWiyXYAW8EHdjH9G2OTMvzDniBUwiiIWL4OrfHSR/mC1PYnT63BOTWPp6KJuXttyW09ePTX9FfRZ7ADEy/bRtaZTamZ7x24ihB8NWvftWNFtPqtEUEo2PIShO4Pe5xj3NPH4BirNkXcffeTjzxxEb0AfenPe1praBtTiE7HdlbQfLOTOhryyyzTO7zrU7eO++8szMnwXkvfZTVzm9/+9vO+mqr7Pu2t70tM3WlzpCtQ9BZTQOTcPERS8CzNhDNKs18vJh58PXm2wqtgzcWzCQcgeHYTnS1lGyV2TjLAIQsRlgYdVNnGu0G0IiZzfyYjX4sCCEN5rl9CW9B1E6DWXKBC7MLF0YXmxl0PZwx0t7+IxPp4wQkYRq9M4Arbx1HqHIsA9FKYovAIXVVUL80vUknW6BO7NNZ9WBcCGiV8SyzxmBEcHnYujrXQTMi9a7sKTzKVghaGsGdJn7biqnkBz7Sgue/8MNgDWW/9rWvHdGSJ844XPiOGT/Sc0WpTxD+tvQa0TUEny4fY4Ch/I3RBsbEuW5t1bb36Qa9P/GJT4zgf/3117dKXji3MVBRKi9im5XinAiNM6+QgTr3uM6q7NT5iSvGaNJgIZp+/jCc8ubX9gqOVjazDdGJb2Vw8fMOy2373cfJbMTk3maKGIvBK/bN7II41MiP60lF8WJpx4W94hWvcDRrcrWHtDxYAPPLYlBpE2ozm9/g/GdWEwFhtj5BDGM2Ct31ELMY3Gfx0bKgyXe+8x1HE0bl1VdffbDGGmu4dz00rO3Z8vTUAxNtNHoZCNugbofTYOAzlso3Kd/AROJ5J1Qbx5jF9k9l0K4Ux6SsQzTzy/XpyFUagV8PDV6TCiUNdPfzh9liNJmUV9H32m5+LUMnbsaGCPpuAFfSMdKDSN2QdmF9gBEkQ1uFzTIi3csvvzyzG7t9FF1YBqpB0AaDqj5AN0wocAiLWhSeT61DDMWBdkgpfYeBsYLIC4tk1F2+EOrQnXw4d/rRj37kPMeg18kTAp5mOM9C5M4+h7Je8pKX5GeT5APUwSEsS+/Kk/cvfvGLOa3YN0JjNIDMTudQcuyPcCQCbY0Js+WWW845yBwHtAFCF3R2d9111zwqBqfo3xyCN65XERdOCofjWRr4I4Hp1+XLpUnp2/yumc2kUzMzs42rn79nGxevzDdutzMb0h5VQTOjMZprR+tsI0tAwk1ly+XvL4erltVVfNWBXxPbD60ajGNcfVhZYBlAs9Q4XDAArHT6/fSnPz12Hz0uP/9bZWmkJXZcb0ukER1Huxmbjwh9/pHWB6NdCMKXXz1hnLbemWHbLkN56tfHVWVpxFVdwzhF76TbYIMNshVWWCGXLiouNKVMRnwpk3dx0NsW7ckHySQ42wXloWypA2bbOZebVAfShzMYUmMOu5tCLWYDeZAKEQfJENGmCIbpKRdtApWlX3DBslXIXCx5iMMxBGm7APLliEO2Ctssw2ckf8BQPf3vYkg/XlhnfSPcZkTn51qg9mTJxF0/4oZ07rp9q9IuxI/0LNM5ZgD8Poq3VuIzKMcGJuJzjED/xktO62CFVgJrJCcmtbX70BISn9ZcQed7l0D+8mZixBia8o2Q0WUE4XaT19k5YcnZNtiImuNhZ40Ts2cZCU6I/icB9a0rvVt22WWd3wEts8kL/+Mh3TBbYFot7jFHJp3QaFI9u/hOvTH1gE91aKF6s6xEsmr6loXF7rnnngNTM8zTYDnMBqDC+GU+VFbXQgeNzT2KoVZAzvw4JUT3rQ9AABNTEmbkQv3mGc94xogu5Lrrrjs08/WBZ6wMaMYV/nXWWccJG8oAwohVV13VCX6qAIfJi+3A+elPf7pLhjoSghcfGOnJG/1KzVqzNntVqbPiQmfqsfnmm7sHxW4UlwFmLrz/vOAFL3BClFh98QLEDX556zn11FObX8Mpw5F+HLM6OzQywv2zsnE+7rjjpiIg0cxmS5aBGZ7tZHZnlA5XDbybRWTnQSb2XWHE8z3KWH/LVwASHIR5V+0Xsx6f+uEdiRUF9RcNTHo+Qle/Ljaw5/Ht/mOjalbaszFa8ACMBnpQb4mNDi5iTyC8uiqO/K3BCtf6lIu4PCYk8ulWF79wf6x8dBEyRn/CwJlVx2te85qhosEJ40tcz4mlrYvnLKfj8ij7Nb++XB5FxQyIta/i8ks/B2r3tSqsyuhggoac0/GXhoueaQN42bUeN2p1dajNLGFLLacqhdVdfvXY8jkfKe3sbGDLtqHvxG+63o/RmKMOcMA8YGxmMkFBbp+TPgJ9bDnt9m3+QXYs7/kYBo0020ObrbbaKp/p2LdiEg8/b8QBiM9jV6/y9l1rrbWitC5DL7i0NITMZuYNahdcutASEUUU249El1MlspgYxV+SqTz9yuCsXSfKG4hvdGpTcO2MRmaizXUCmA3m8TsJvss4fxOT2cw2hNvECi+ACNDLvNjmkwe0QhMqXJKjGcM3HpitLlRiNtto5oWifhQiVReJpul8RiAvOl7bAPMU7U3tJrGjC8wmUNwuaYQqFVI11IzE+CqfvTQzGQ9+rvVdDNk2feZifmobu2LjDvRFL/6jJiYgHkZ3mzJb5T2bFeiAtS/7CH/9q299/woP4aK1dZt4aM0eq29sP6V9bB0aGWMM7R+s0aNVYb+GyygObAFwIy4qa0jR+M9DuJ4YrtHMF0Cg2gZPN6ZX6/Zk0Av6o+6FCpsxmuvnagO8F9n2qRZ1ajGbGg6kEoxSoCldoO/SSy/tGhkLYDEG90sVA6HXSIfheIY0hJvHHGdro4hhR7FfeCHQCv1HbKT4AM1EW59+tWlpCScC0yjOIgwR92y99dYDk2R1theZiNCMRQgPtdtYxso+vin6lqotNx1oGw5srfMM8O1tisUjy8tSmS3QSPRzrj2pn/NrXk3ddgk9YGM8923RokW1KFRqZgtHVispKiYdGhZafME6Fzhg8aopgDtPEfANTXZZhCqKF4aD35lnnulE/10sY8Py9A6+iPB1cM2yBzBNEKcjCF5h+xXltdDDmcVw0AloRnv2s5/tDFgBrFigZd3+X4rZ0C6QY3l0xvAWitKnEJorjQSR0DI55JBDoijzHccPaAtUAYzE0MnRSJgGoJPpL10xnTDJ0tQ08JwLZaIFBS19y2YoIqN4z5KePmIecBx9xw3a0bpagolgh4H51Ioou2+wvYgrHwlblyCJnQ0mg6WWWqqXZfI555wztGyhnv6jpYvCtEy0/YUjhakd5WdFahuWPQmaUwB9WtGdpTm01zvnuVVh7MxmmTkG9Zche+yxhwvrCihT5aqM8D0sm5FoUpwwDe9acsXKCZdeyr+p8CPEY1J+4XfhrDoLL8zwocHP+1xbcYQ0mZV3TK9reQ5d/f6CEArtk0r9ziIXAiO9Vdw9GmHxrNkFaFbBEd9ll102dABr5qfzmU3xYr9V8VLd9ItmN6YVGMXwVMkM4Zez++67Ow1ywtqcPcK6kDcCEm4HhN9UNr941gR38EVLJUG7FBDtpUES9peqDi/Hav37o7sV3OmAY2Rylm256m4H5tGyODsqGrW5r4Z5gLLAKKXNrtLILjzht99++5ADBj9fXCp97nOfK1vUxHjhLKp3aCII4xDux6M+tFERfSYikSKMUCDWDn4kvkPzsgKxscw2UnrHAQ972MOitkPoSNjY4BAXxxwxMHFsLLgwDAJhlyIcRAjnChH28rGp4nd4MrM7aNkuu+xSmG9bH2hIDIjC2NjeCGHTTTfNsDYNc7GZl+QxjJfem1MAY7pcV0IQ5QM2eLjqVfai6cwwG50Gd6w8ISD6x7kCBnDsPCn8XPvddBeH0orxcP/LNXi+x2aU2gVWSCgmLyqf73rYW0iLpEIRKWoFCtA/GYj9fRvaJDfeeGO28sorl8qpUECihiyVyzyJRMc24y7Ou01RJ59GVcPZdZVVVnGzGuDjOUs4T4NOXZbJNSWf0eqUVchsZObbp+Cd8yfcAoVLrzoFV0nDCNIHzEpnhb4ckOMW1/eOSrgdFbgzT+3NuPGNubZZwb2Pduq7DGiL+pwZhG1UdCGzUYAaMPzf9yZ8zTXXdJUMR/hGNY8kpjNrIFFZXZcZQcMxEkIiDslD2p9//vnO0wp48g0Nh6r71ViZKWw8BewalVPmiEHpVaBFLAQzMJqLlq+88spOLkAWFu598EXwZeLXjWMdOBfr858Ln/zOCqBzaY2dP2ZTY2auOc0KjbrEwyTQQ/SnLWxgHKBgUKafFM5sPgdbBdysgjSwa2DEVnkqi5HeH+G7wkFlqDzqq9l9XJnCl98uIcwfPIVrl+XOct6iPd50aCs8/IR0agt//NdddNFFQ9lphVGmjFLMViajtuJAMLOJnyFhg2hNN6Vt4RXLRw3NEoNOzz6rDygzAPSBxyyUQRvAAMsvv7xDB4kh9Gmb4civaLIpu62aOWYz+37ZFVdc4dbHRx555Mwf0mLuDAfrQJ9MwPEEUsm2O9UsMFAVHLjxcPzxx7skdHr6DMrmbUPRyuqqq65yVqXLwMwxm5CmE5U9mS9T0S7idDGC+vVnicLDOSOSYKx3aRTltjBnj5pdu6jfXMgT68dmwtChCq24InPrrbf2NvAhHUZgVQZmktnoQCwjOeBeyCM3GiQsXbjOxB07HtHjgAMOcDb68WKzkGlUppN3GQdtolA5oqi8qTCbLwTRyKxfjdzaeGr20HelLarQuHDlgSqWOqnCxqXjm2aZEF/s5UtdJ/zGO+nqANaP5TtAZZOPjy/72f3222/EwUmd8vpMc9NNNzn3XrSthFD8Z7Y2rzzu7tikduE7bqWxh4nrLTOdngvRUMNj/0yctgE/CJQlYBm54YYblptJDaFCkOifNrZ9VGG8Oh8m3eOizKLH/B3XKdKJZ7F9r3xNcdnlQ/gkwBVwET7jwmXKrkwZPg5mO6R0eXVdRk2qcxffoYMJNMbWTVaKx9EMO/1mlMflYwf77gjE9mpD+WIhu20AJ9OdzcsxZitdxFRmNo0K/i8OyBnB+ZWggWWUmRnLo2HxiDh4GqkDoQDDqOSyCcNjeSOwoezwwRmf8sBgDN/NRovLE882aHtUlaiSRxmcYnjOehg0xyl9Uf0It6tFzhZ/LA7pebg9jSIwgqLzzjvP7WWRCk/bCeY4+nd/cBYpHWKh+UAnFEHDjo+mOx2ccEyNCWINECmiVJCuuVPGJPFtUbnCm1/FMS8/GQ/vhBeJjIuQBBflWxRnrocX1Y9wHgaccaD2CtvOz9eMHjlJsd1DHJdVo29qYzIp6iMqYCozmwhShBzhVSrRiFoliFQ1/6KOVDWf+Rifdp1kHwVvM9xtjNGR9AzUunbEvUL1FxjQzOE7sqlvIdGO5dMWbdH4R4e1DEyF2SCKDh99QolAWkbpm4in72UqRh5avmm09IkODn55sThlygnjkCd1U/6TZswwvd7Bp2x9dUTSZacqwrNqOHVCkjoO1BZhHMJpV9VzHH0Uhz4waZYMy6ny7vejiekMqSiYNGxos9mmgGScriNmEQzpAebNZTs/iuCEQBwNYtqAvIoebGHKPqPi4BjjkksumZD7/382rfw8f9/8+KQMrAPkgpm//e1vA0y7b7bZZgO7qe6S8h1f1zjzMyOrI3WwjubCbM/iBAN+fpPKnvZ3zFx8+ctfdo4XVQ/qgusr3DpRF9HAx5Vwu+Hg6o1Nfru5H60KPiiMCVw8DPO84Q1viMarEwgOvoAExzLCd1J+jBJRgLn8Ttoms4GcCQ8GO+2000jZMBsNYMuERkq2MLRN8SOdtIjx/HDKLgtiNoykUmZZIC5eaIrw2XnnnYdsoJj5tDwuDjug4Vz3RKMBwv/VQFxER5iN/sEgVERv8jOtjpxexLfrYqWZoqhswkNmqyKNLBSQWL7WD/5v7av/LqABkA/PySefnJlTxeha+uabb25Qwv8nZXrnDCQEznDwTAoY4Uov1cJ89C4hS9F3P5y6cz/t2muvdfp85ojQCU84vOe+lA+YDEcai/Kr9icsm8gDvHm0RB23nCqD17TixPD2w6grHkOxDUN9WX6yLCQOUmAzuONMQigN/idX0FPIAAAcjUlEQVRYUrPnw04/9CEd+SApfu5znztEtzr1Jq8Y3qXyssRR4DzKDmvz0aGNmc0fwZjZ2pzeo5WIBPrnbODTJ5gdi8Emm2ziaMpou+SSS7r3EMDLdPxy+lsHcssnWRkjva519F2HENeu3/Emg71QztQe+MAHul/eMfmOuyf1KfBgpsfHmuL66Vh2YgeyaDYsWw+uXdnNgpwvqsxshctICtehNutfDLWa1KUsTjkRtCzQr4izkJiNTmAzqts/wCg8O+6448B06grpCb0OO+ywoT2N0vJ7xhlntLIsKkRgnnxQfzN9yVboFR7IV2G2UtJIpmKWfUzpZUFTLQ7CdQhNmLWhm/65dxQDlgnEocz5AixnoJ1PP5zScwhbBKRBLaiKeb6ivBZyuPohStu1l38tEbAUs6ksmKAsENcMujolTcx9SRzOL5arqPjRRx/tfv2HPYzdCi9bTKV4MDAWbvnlqVKfSgUFkWONHAsLyygTJ0yT3kcpoP41+qXfkEIBSVM06Mxs+tddd908K3Vuc2XkTvZRzbJ19FBRxMFkXRcdTQIFFdhFGU3pltLPLgXCwdn2kKVtRlKrzpiNGQxbhs9//vNHmG399dfPzj333MzOljJTOh2hblipkQgpIFFgChTgTiHXnATcMzSF6NKYFC4j6fCrrrrqUEYs/7AWXJYZwplD0zmMqKVciKnyLltGmD58Jx8u+IE7/5vuBZVH30tRv17QEWVdxN/g0xatQtql92EKmCRyiNbmzD43x1CGVoXMRmK07H1gj2Wi8zL5RuOoY4xjKO4msdzjVnJbgDM77j4B4VKyThl0chPb1/atTJllmIQ4sQGLcHyEYcOT+1+J2eq0YvU04UCNkIstT1koZDYa2W9EzUplOsmkwsMO5Mcf921SvrHvfn5t5C06QHh1+Fi5fpgaqUr50Pnwww/PcBiidAiPcHTIu59nlXwn4Zq+xymAnMG82Qx9hO6VBjqLPBHadoZoHWXoMNJHQLqR6M61Bdttt92grG/qMmVy6dSo7h5jhjJJXByzGpanU/r11lsvmp7Lo9aYefzlllsuj8cZpdLziypSgu4owJmnGcwdonnrzhDjPN5OKCOzkaedzKaUizFApXMwLjmy9NPsCNqobaG+5QN7A8wdiD6rrbZahl3EBNOhgNqr6RakcBnZZbXoRCCuaXgS03HQDRTFI3zSo/Q6NA/jV6mv8ECPk2VeWcBfM2ePeKwU4MHSdzQPTYiHpIv/XKC99NJLh257+3sH6IiwhHqFe4qyeE2Kp7xFs0nx59t37JxwZ60pfUuJ/rnajyYIwhGM5aBIjFKn2deoRVdpRxxxxBEuPX7Z5LQAh4cAN52xaw+geYFwBqVTU3PKDjroINchBXRKNC0mOT5AChoyLGm4CY4vNn/ksmsvQzfE88Lsz49//GP3CvHRhDFvpP7njI0zBoXAKwbUm/3Ydddd56SkISgdV/y5KAnozhr/cZtlOpVOuRYciN901A1x8N8pG4kuZuPwj+cf54xLN1++he24yHwBLl682PWl8NvYOluCUsDdL8vIPVtvvfXAlj6l0hVFCvcdynvcrzHYYKutthqYRspItqEddrPe5PC0g3O392HPwzuKv7yrHLOM5IwAaR+pjLkrNQ6Xcd/Y45JfV8Ae4tBDDx2YH21XF8qzM6BOy+yqLrOcL23IHUXuVvrtbcxWC+1SM5sVNASMplZadc72cglt7WmExiIyrn5NQOIOvcsCeyGeEE488UQ3i7C/Mn/Z4efCd449qCPAVRdmcUkGmUUxNEMcZlz2U8yEjHKkkTiY/5VGvkJshj8wi2E0B9NvxnTZhRde6K7pMOPuuuuunZRZErV5EY12oz8eddRR2SGHHOKkzgKZzatV0bIsqpnNGtqNpry3OXqT17777puPIG1JI5m5jDCVpIY+TTTj6ZdvmkWZLf3vfAvfy9K3TjzKsuVqPlObsu3IDF0n34WeBrragBld2dhBdu1+X0pAYsTPLrvsMneR0RBpPKvFRgVmgC72HeBL3nVnGKX18xCeytP/5seP1TMWBn3rAHXbZ599Mjs+cMkRqoAblyj9dqqT90JMQzuoLeq2yTi6lWI2dSBJBf0M20LKz6cJc4SVxTKu7dVcB/SJGcYr+04nFgO3UXdua1fRQvDxRFgEc+HMnl/hg/Bohx12yG644Ybag0xZesyHeD6DmUzA0TKm88g2haOaugN3KWaDoBQgN7+8Y14ACV5bQIVtGeluApjBnWzjjTduJWvyPfbYY50eIdAGg/gzW9P86jYcdVFacGAA8IH9qRk9aqW+rTTEjGYi2vGLxBWf6l1BJQGJ3zHUyZp0Fr9S6sAIR9qEcGnaFF/yw+5I2LnbxLlqXojjEThxV09A+6iN+G1a76o4zZX40IWjDTO+5FZAXULpma1LJFLezSmAMwlcF/lMxepDZ5lNZ+DmGKYcKjMbYnSARr366qszLtD5o2hXJFUZ/OLYffvtt++qqGi+OD2kzoh+sUNfZWaTsCKa8Z2BdZjBTwNumFlgb4oyAJ51+M6B+yI7hMVngvCogvs4nOfyN/Un00HNzJiSm9WgIdaauWuJypx/sRlvRWj/NAIrtBJYQw3M0+NgpZVWykWjhuQAryJdAuWatC0v00byLosbyRtbkkbo/BgBfMoCcW35GRUlF4WrrHG/0D323SSUQ8aFFGf55Zdf0IaCaAcUAvjlQTldtKEdoA+WswCzmzNEWzvXbGyZq/LMBvcbLkMbb96RqHGwy/+uwEyTOR1AM0vWVRGl8/WXa2USSb+QWcV/UNfCIJL/XaJ8M9Tq4l588cVu1GVv4cez2wyuaK02+M+eEjv5GGjCxxvphOtiUzFC1xIg34UGPi3opygDAISj/4hSALb7oY3fvieddFJm1psbk6uSgITSQJKlI94wr7/++hwBwmNHA40xvDMDVd4nWFt5Kx8Rmb0PRor8gQPXsQJ0Nem4fjqkqL7BUB83H/cQZ33zBTlIxXxYc801o8yhtCgyxwY5jNFy/w0/02az0mXJ8oiycECx4oorukuwpO2SrmGd+3z36cISG+VxPLaqzvTlPffcM9tjjz1cmJk/d1sFAXRpjT6WUSVgGuYxC1gjSxiWknzrGpjiu1hGgjvLCzu8H6mbEd+FGfGj39CvrFN3O2dzS5Y6YGdpDpei+2zUhQdfASHevNuFSPcdu5Z1cK+Dc99pRAN+8SMQ0sFU8Vzd1fY2w420r81stbVG/PpWXkYyKvKsssoqTgvcB0wPWObRUbbKaMYMg7aKRlv/l7JZrqL5Hn5nc9ukfPID9OvjHAvzv3MroqzroCq0iMWljtDInw1j8UQf/JNZZ3JpmOUIJw+ODMjDFJrzMMLnA6gfsArBqT31xEWvABqwgjGryrlCAGlCmnIHkRvak9q/FM18zqvyn5EC8IUWVuDA7H20MgqMm10op+jhBq02uVXqo7jUKzbKS0Bi0r1o/USPqmVqZquSnrgbbbRRTgMsV48DP2/MmlvHGaEfjjyq4DCuvGl9A3/VQf9teTi0IqHu+hbW1/bPQ3QxDZxWq1J5ZvNHhhg3G3atzGw4k1Be/DIyo/sn4FzJiJXttttueRhqSnfccUcjV6+MYOHo5teTMmN1bDLy1Ulr5sedpn8ZIH/w5jH/Co6W3LkD+MbDzQa5MqZ+cxnAn+Mh2pG7jwBhdhXJ1V115tdvS78dzG+7E/23SgvLrBFoFrAl3NAIsssuu7Q+Us6K6N905Fqv26RGgM52q9vRmP1yOCpPSh9+J732KXYZdGCSznzGs06aj/DyKaD45MP/puWH+FR9Fw7Cg7qYBHZoZjLmcfWiPiZ0GinCzwP3XcQjDc/tt98+Er9pQGVpZDjiMXowWiCmNkcQ7lBVI4kh185aNyx0iu84peeWdN+ANNQuMbpiNSL7I3FVfGgbtQ+GcnGzhOgbxW3rhHl2KDSjnsbBL+GsHtjDmBPJqkW2Gp8VDHqMuAVjrwwt1PdUEEaCaS87E86w4wL+/qqF+qMAgKyBX945VkJZA6WA1qEpt5KeUYUHtzyGYD5Cclu6rVGQ/HG5RP48XUgjx9HCHwXbqtO48mLfNLPhDLEu4PJIbYQ0Um2HRFL1uummm0b2dYz2oj03xGP0IKwLAEeA/Pm/zjrrjOAn3PjFswzxiE99qVsMiIPbKb9uKGx0VQ+4uTGI8Gyy/UrzX14ymxbiNy6icjsvaZplpfRqAOFRKXFLkcUoTZlNSyV+EdAAYf3oiJiG8JeXYduG77i/9fOqU20xiZ/W90sXlum/my2bkXoU4UB9TRlgqL+aXZ3G+BeVR3jjZaRVNl8qsqHEfIAUYpnaOTxFmNEUtGQynDM2r32Dytdv3+W3WR40ZDnFobeWg7H6IUTBzAKaKALz3z2kzKBw0mOmAeA/1698zZay+GPh+YILLhiJTp7gLQB/DvsxXGs+tJ3CNd+NiZymzbh2Uj42g+f4YnRK/XRc2hHEqgSM48S636yB8hGD0WaWD01tLe9w5XBzVgDDreBU9cEb56QlkGZH8r7mmmtqVdlc6OZLL+vslfGsWi/Ft33axPqVrZCMASvvLbfcMmpIqmx+ZeLVFv2XZWhGwqWWWqps9BRvDlCAGQxhhHUwZ2YQta+ugL5DWTwoLXQBmDK0vdqQucAuymllGRkihtLmMsss45YgABofTO233Xab06tMMJ4CoW4ksbHwtMiuygBcFEXKBtDhzd+58zjEfy2BULKVtI7rIQLiACzD6GQod4eAl06sShUBZagc8sH+pQ+UwVYCBWr6ga9DW5Qn4SzlZJOS/kI+dmCfl9VkecfyEpuneHPloihAfigg4waK/yxJu4Tauath/Qb2EaUzbLHFFhn3wBDTAph9Q5SMk0SgCfH8sibhorjEU1zK1jvf+R/LU9/awnWokAovKDnDUDBWCIi1faBjmeP3zM6KHFOFCuPUhTiY4IvdoBjnfjgsO/ZO/jCOfJmZj4NYtKEwn/5qG1vmZsccc0wG86MeWAfU3jAae0gxGnlhBPd1r3vd0A33OmWUTmPIVAKkRRwAcp/NNqaFa2jtHczMwYiY1s5FCtNVQubOyJJgjduvmOBmYHZN3IGwNaY7wORw2gjlHhxZkJ7Hlr45zhxuSvRcB7e20ixevDjHe5I0UmL8mGRv0p6N+s9CfcHDbMcMzDZn3i51aKn6+PtgSVhvueWWVvvhJPwq79l0KGgZu5mpaMT3w4nrAw7sUSRmdG0DwOmUU06ZuKbHXDcKw+BD2RhbJS0PN5r1n1FZOLPMkrnxIlyJq6coTtNwcBPe/IYHuH7+ksaRpqh9ivAhvtq4KE5f4eCiPlK1HqKVDRwjNCCMqzZcE6uab5O6V2Y2Ks/yEM8rMEzISCEyrNnZswGqGL/oNPZVUXAs6kDUZxzT43sArfhxQON1DViKxgkH3m0QUcNQCcZTgP5F27B9wdSB+hvtjZ5k31B5z+Z32jLMQhykSFSavRqXGOn8rMdPOOEEZ26NOGXyqksc8sY5SAiYl8aeCIIbzml87zJh3Ng7jYazEZxfcNnU7MJnZoE5FrWVMC56YpS1DYAme+21l7NNwuXTrkAzftFgx3dwwQZICHbE4Oi6zTbbuMHSHxRJs/baa7tvgN9/NAFgwhBVNF0Fw1y8BD9F+IQ4tPpuiPUGRqyBbUrdHsmI434322wzVz7fmgAmwXG8URYoD0eGwqXuORuOEVUXnDAIyF9PWZz6iBfu2ZrSfRzOtvx29EWBPAbsDWOqYaSZ9EBzLs/6NPZpvsEGGwzJChaZM4wu6xqrXxhWeRnZlNNxsbT++uvny8/TTjstV7DtYzkm/GMjYZ26kY8RNZrUGrfwWzRBD4EsP8GLBwXdLkF0KaIPtONCJ+0unPTLnpSZCS2W8BvvpEF5uggok0fifPKaNvTKbBCX56yzzhoSt6IOhA0Pna0UEaWo0Qj3mSdMT8Pw+On5T6P5UJS/4sS+K4zy/fy4b4Y6VN3lSqyssF513tUG/m+dfNpIE8NFYRLu+PQN40sQ5MfBUC1pOZ8DiMMdPvwJjusjbdRnUh6V92yTMiz7/eCDD3YOA2XhCGtQbFqZ+ZAGhgBBWb/bPbnwk3s3JVjHUBA2BggVuGxJPiI6h+/o1XHewvp/HNx4443OPLXPBPwHX4D/WMrCYIyA8tiXmirQuKxHvmE1C2EI6Z/1rGeNfG8jAHyR4HIeh3SYwW7anbFuvcAbfVkcaOLfQMBemn3pzDhvNKJPBViv20wwwDWUjUT5Gt0IN7CZLl+LE0fA/6LHDGg6W4mx7ypL509+hYkfCw+JglkEcLOGHDk3LAojHEeFVUFnQqbtUDVp6fjU2zRLXF0wq+DTuXQmFnEc7TArAA1MABXNkjJpmxjwzZhnYOpaY3Ejnikuu3r47cNZLnmzR61btxheTcJ6XUZqxDGE8+WVCUicIw3/GxoRLAWQFhLXB0ax8OE7YcQNv/FOXiqT9xD0PQwveicvtCKYSZkdAMI4x2NW8suwhi7KZmJ4WPeJCWpEoIwm5UA7dCVjdJfOJBogse9oxJSBGI7CGSvFzGB+HFYbmEUAulbBKoO/4kxlGanOyC+dEeZCl47lHIrLAhyzowd4wAEHuKs7MUYhblG4T4iivZOPyzjCYe2LTbvyAe8wT/Li1q+sOZFf3Y7MMQlLuz4AHMvQsAgXluKI1UNgKYzeJFa8YueCvnnvMC04IdaXaD/8Dv3333//jCs5Ao5GEPNzLkp6tU+TuoXlNnmfCrP5CIsgdC72KFjz1WzA73HHHee0yjGiOU2gwfxGCxlNuIXxihqauvnf/P/MmCgO+MaM/LorbVHefdIJHBAGxQDNGzo+99OqKqAX0VflwMi4OPaBu3kyyxfDZ9phU1lGFlWaA2aIGC4vDjzwwKIkczocrXw6FY+YlF86Jw4fWAL54fqP5s5CBWYsM4vgTLajXCzARgqrororiT7oOTPMpg7H76WXXjrkvB6VKToaI5e/NheBCMO5PNLKuQLjZiUxVdO6QBcc3YfMTP4s61ji8R+tl5CpSYNeKEvnqh24yT51XJ3Jl6WnvH+q3QmXl9VJM+K4/Lv+NjPM5leUhsduJOpT/Fdjw3R0Eq7siOn0ra0OWoXglF23ccGXpaJfD/3nTIj/1D/2netLpJ8EpA3PF5XGzzdc0hJH32PfJpULblirmqRTOikfHw/w4SIpRzDCDbdhcuNUhh5lyus0jiE+c2ANnFtHQrSM7ULa33+4MmP2TQpFx31USnhSFv/BtQ2Q6N8ERI2yAyc9YUaEm06koyk2FXn3QfUpSh/mF6YlHWpadpY6sDOwcdGj30hvzhwHdk7mcMT8gg20Try/7bbbDszc3NAxTzSTGQucuoAkNpL4sxQzGepdKDBzALvoztvKdhcpW2uttdxMh712BCtAnyOcjyf/YxK3WP2sD/SC6yRaCA8824Rxq9QnrKPyQkKJkCd2QTVMo3fhhA1S9vAsY8nPmM89eFNlxtSKIsS7KN9ZCJ/JZWRIGES66Mgtt9xyjuA0Ho3CBpmlJUcDED+mOR7mNe138OZqPvhW1SyZNu5Vy4cR1FZl026++eaONjApWiG+6y7zkuTMQWBAtWq+ZcvvMt6cYDYazR/J2LOhwKpRTaMh63f+671LwtXNG0EOdvXHAfjLwvS4eE2+IfzgTmKXQPv4bTeprPPMY8zpp5/uoqkN2aex72SGQwKpPKvkO6ncvr7PCWaLEQP7GdxNwrGdgOUQ5rGxNXHqqae6ZeesMR43xWVfMVYvwrjoSB3qCl+K8vXDWRHIEBAmvPsGDYpcLKateDDrTp1hJJaKaBehIMD7rLVjLXpZJeYsaFNvh5sjAhRrILeZNtv1M1c/BB/WWAO7ODs13KAdupHgsbz5ku4bfB/l4EBb8cuDW6s6gpm+61C1PEaMOQ9IAVFEVmOFjUdDYsRHSrMop3YNlCUlWw0KklaiYGxm5EYkgMJJ8bvE0Wc2u/LUZVEubzEPv3bTY0j53GazvO1sGVlIly6RVNvQZjxtSZZ9nOcFs6khbTM9MsOJAWE4HAgSV0zQZeNRhml6DMwsnCvPrsq4X/OBlo/ids421An9Dtk1w/XNbKK5CbEKb02YQnFv7RO2vQZHVhv0GVxWtw3zgtliRDFdS0c0f3mipSVndBrBSOuPZm118tjZoM/4/iwc/sfZRRt4UK+QgfXON52zMbOJBrH4MfoWhfl4+3mpLLVH+NtGfYtwKhNO+b7nUZN0l0lWKc68ZTao4HcsuwoztHSJLTnDpV2TDhBLS5gZLs0HAWa2GGgWiH2rE2YXdUdmfDzUaM+mQShkejtucQ4Yx4HqKVrbhdyRssJBxgzN5m2jdhpXxjS+xdqvKR7zmtlEHHUE9gNhw4cdDAcLiq/fpkT204vZKLcvAUlRx8HQLngws4VxytKAeDAkA4RJWQsZjXLOPvvssZdN26TzLOY1Z0X/1nilwUZuJz5GtGyN4B7rHE6rPhSvY8aAMDkDIa51qFwhV+lLFz4DEal7CNTDDw/jiGb6JT1pAOghOvBf99UwTREC6TEVSHw8gaJlE5YVppmv7wuC2YoaD8cPKDzLfa4fD20FmE7qYhxGYwlMHa4oz/kczgCFHRYzE+dow4N2T2gxmisw+HjAJgvMWPUu23yl4UzqRvZBbEZX7s3RGdC75La4+QFw13tChqKDEZ+4HKbrPypkphSbv/uzQKwO5Mt1FjTX6aTofM4aaMbSjM8As3jxYocm37CEZgKcHG3CiAttuOSLOQKcYHAgHa4aZq2uveNjxFrQwJ5DwH89pn0ytP8wRhrZj4RhZoNk7H5PZflSwi6Ir3L8uoXl8E0CEn/PRri5pxqpK7xW9OAcUSDhTttCnhD/ufi+BEj3zuEzXiAkwd1SzHeZj7r2HsTX6E4YJvnMe89ILfnmk1vpRyI2DDCGcTmovEnlMHNx01kQ4hmiw3cu8pqv63z/y0ydYDwFErONp0/+lQ6MuQIeGAvFWHQvsQDGf/Z27GkA/7+fPb7pZMQH61xd2jPEdAJ7UoQTMeCmBCYoAH/Q0LsGBeqCnzX0KKEBuqfY5sRiVoJqFEjMVpJe/ozEf3+20EygONjCwOuMmK9kEVOJBu5YqIbx/XqFDCjk/FnPp8FUkJ9jhSZma7nB/CUczMbMErPw3HKxpbPjuAM/2D7TaBmcmKc0GWtFTMxWi2zjE8FwLDU1003qxFwiNW8u4zOt+BWpJ8cVptE/ktKfwfQ/nK1HEqWAxhRIzNaYhCmDRIFyFFjQh9rlSJRiJQq0Q4HEbO3QMeWSKDCRAonZJpIoRUgUaIcCidnaoWPKJVFgIgUSs00kUYqQKNAOBRKztUPHlEuiwEQKJGabSKIUIVGgHQokZmuHjimXRIGJFEjMNpFEKUKiQDsUSMzWDh1TLokCEymQmG0iiVKERIF2KJCYrR06plwSBSZSIDHbRBKlCIkC7VDgLlyt0FWQdrJMuSQKLFwKjOOnNLMt3H6Rat4yBfbee29nEmObbbaJ5ryE3SQeYLxl0gXHaOoUmCiQKJBTACO1XAI2XxK57zufPEvgrQOXqYnZUq9JFGhGAd3Q12+YW7qpHVIkvScKdESBtGfriLAp20SBkAKJ2UKKpPdEgY4okJitI8KmbBMFQgokZgspkt4TBTqiQGK2jgibsk0UCCmQmC2kSHpPFOiIAonZOiJsyjZRIKRAYraQIuk9UaAjCiRm64iwKdtEgZACidlCiqT3RIGOKJCYrSPCpmwTBUIK/C88OVBmHq0KMQAAAABJRU5ErkJggg==" alt=""/>A solid sphere having uniform charge density $\rho$ and radius R is shown in figure. A spherical cavity of radius $\dfrac{R}{2}$ is hollowed out. What is potential of O? (Assuming potential at infinity to be zero).

Question 68 :
As per Gauss law<br>$\int E.dS \ =\large{ \frac{q_{in}}{\epsilon_0}}$<br>Which of the following is true about this

Question 69 :
Two point charges having equal charges separatedby $1 m$ distance experience a force of $8 N$. What willbe the force experienced by them, if they are held inwater, at the same distance?<br>(Given, $K_{water} = 80$ )

Question 70 :
A spherical volume has a uniformly distributed charge density $2\times 10^{-4} Cm^{-3}$. The electric field at a point inside the volume at a distance 4.0 cm from the centre is :

Question 71 :
The total probability of finding a particles in space under normalized condition according to quantum mechanics is

Question 72 :
Two charges 'Q' and 'Q' are kept at a distance 'd'. The charge 'q' is kept at the midpoint of line joining the charges. The magnitude of 'q' to keep the system in equilibrium is:

Question 73 :
A hemisphere of radius R is placed in a uniform electric field E parallel to the axis of the hemisphere. If the lines of E enter through the hollow part (flat base) of the hemisphere, then the flux $\psi_E$ is

Question 75 :
A square current carrying loop is suspended in a uniform magnetic field acting in the plane of the loop. If the force on one arm of the loop is<b>F</b>, the net force on the remaining three arms of the loop is:

Question 76 :
The electric field strength in a region is given as $\bar { E } =\quad \cfrac { x\bar { i } +y\bar { j } }{ { x }^{ 2 }+{ y }^{ 2 } } $. Find the net charge inside a sphere of radius $a$ with its centre at origin.

Question 77 :
A small plastic ball of mass m covered with a thin zinc coating, is suspended by means of an insulating thread from a fixed point. There exists an electric field of magnitude E directed along horizontal. Now ultraviolet light is made to incident on the ball due to which it acquires a positive charge. Acceleration due to gravity is g.<br/>(a) What is the origin of positive charge acquired by the ball ?<br/>

Question 79 :
A uniform electric field $E=2\times { 10 }^{ 3 }N\quad { C }^{ -1 }$ is acting along the positive x-axis. The flux of this field through a square of $10cm$ on a side whose plane is parallel to the yz plane is:

Question 80 :
There is a solid sphere of radius $R$ having uniformly distributed charge throughout it. What is the relation between electric field $E$ and distance $r$ from the centre $(r&lt; R)$?

Question 81 :
A charge of magnitude $Q$ is placed at the origin. A second charge of magnitude $2Q$ is placed at the position $x=d$ along the x-axis.<br>Other than infinitely far away, at what position on the x-axis will a positive test charge experience a zero net force?

Question 82 :
Charge $Q$, is divided into two parts which are then kept some distance apart. The force between them will be maximum if the two parts are having the charge.

Question 83 :
Let $\displaystyle { E }_{ a }$&#160;be the electric field due to a dipole in its&#160;axial plane distant&#160;$\displaystyle \ell&#160;$&#160;and&#160;$\displaystyle { E }_{ q }$&#160;be the field in the equatorial plane distant&#160;$\displaystyle { l }^{ 1 }$, then the relation&#160;between&#160;$\displaystyle { E }_{ a }$ and&#160;$\displaystyle { E }_{ q }$ will be :

Question 84 :
A body has a charge of one coulomb. The number of excess (or lesser) electrons on it from its normal state will be :

Question 85 :
The numbers $2, 8, 20, 28, 50, 82$ and $126$ representing the number of protons in a nucleus are called.

Question 86 :
<span class="wysiwyg-font-size-small"><span class="wysiwyg-font-size-small"><p class="wysiwyg-text-align-left">The vertices of an equilateral triangle lie on the&#160;circumference of a circle of radius $6 cm$. Charges&#160;each of $3C$ are placed at the vertices. If a charge&#160;of $1C$ is placed at the center of the circle, the&#160;force acting on it is:</p>

Question 87 :
<p>A region contains both uniform electric field $\vec E$ and uniform magnetic field $\vec B$ . A charge particle</p>

Question 89 :
If the force between two charged objects is to be left unchanged, even though the charge on one of the objects is halved, keeping the other the same, the original distance of separation 'd' should be changed to:

Question 90 :
What will be the magnitude of torque on an electric dipole having dipole moment of $4\times { 10 }^{ -9 }cm$ placed in a uniform electric field of intensity of $5\times { 10 }^{ 4 \,\,}N { C }^{ -1 }$ making an angle ${180}^{o}$ with the field.

Question 91 :
When a $10\mu$C charge is enclosed by a closed surface, the flux passing through the surface is $\phi$. Now another $10\mu$C charge is placed inside the closed surface, then the flux passing through the surface is _________.

Question 92 :
Each of the two point charges are doubled and their distance is halved. Force of interaction becomes P times, where P is

Question 93 :
The force of interaction between any two point charges is proportional to :<br/>

Question 94 :
If potential (in volts) in a region is expressed as V(x , y , z) = 6xy - y+ 2yz, the electric field (in N/C) at point (1 , 1 , 0) is

Question 95 :
An electric dipole is placed at an angle of ${30}^{o}$ with an electric field of intensity $2\times {10}^{5}N{C}^{-1}$. It experiences a torque of $4Nm$. Calculate the charge on the dipole if the dipole length is $2cm$ :

Question 96 :
A particle of mass $m$ and carrying charge $-{q}_{1}$ is moving around a charge $+{q}_{2}$ along a circular path of radius $r$. Find period of revolution of the charge $-{q}_{1}$

Question 97 :
Two identical spheres having charges $8 \mu C$and $-4\mu C$ are kept at a certain distance apart. Now they are brought into contact, after that again they are kept at the same distance. Compare the forces in the two cases

Question 98 :
If $10^{10}$ electrons are acquired by a body every second, the time required for the body to get a total charge of $1\&#160; C$ will be :

Question 99 :
When a glass rod is rubbed with silk, it is said to be positively charged. Which of the following statement is true?

Question 100 :
Eight charges, $1\mu C, -7 \mu C, -4 \mu C,&#160;10\mu C,&#160;2\mu C, -5\mu C,&#160;-3\mu C, and \ 6\mu C$ are situated at the eight corners of a cube of side $20$ cm. A spherical surface of radius $80$ cm encloses this cube. The center of the sphere coincides with the center of the cube. Then, the total outgoing flux from the spherical surface (in units of Vm) is :

Question 101 :
The ratio of the energy required to set up in cube of side 10 cm uniform magnetic field of 4${ Wb/m }^{ 2 }$ and a uniform electric field of $10^{ 6 }V/m$ is:

Question 102 :
Assertion: STATEMENT-1 : In a region where uniform electric field exists, the net charge within volume of any size is zero. Reason: STATEMENT-2 : The electric flux within any closed surface in a region of uniform electric field is zero.

Question 104 :
The total electric flux through a closed surface is equal to

Question 105 :
Assertion: Gauss law holds good only to those closed surfaces &#160;which is enclosing non-zero electric charge. Reason: The electric flux through a closed surface is unaltered due to the presence of outside charge.

Question 106 :
The flux of $\overrightarrow{g}$ through this surface is given by :

Question 107 :
Using general logic for electric field, the flux of&#160;$\overrightarrow{g}$ through any closed surface is given by :

Question 108 :
A solid sphere of radius $R$ has a charge $Q$ distributed in its volume with a charge density $\rho =\kappa { r }^{ a }$, where $\kappa$ and $a$ are constants and $r$ is the distance from its centre.<br>If the electric field at $r=\cfrac{R}{8}$ is $\cfrac{1}{8}$ times that at $r=R$, find the value of $a$ .

Question 109 :
A particle of mass m and charge -q moves diametrically through a uniformly charged sphere of radius R with total charge Q. The angular frequency of the particle's simple harmonic motion, if its amplitude &lt; R, is given by :

Question 110 :
A charge Q is distributed uniformly in a sphere (solid). Then the electric field at any point r where r &lt; R (R is radius of the sphere) varies as

Question 111 :
Charges Q, 2Q and 4Q are uniformly distributed in three dielectric solid spheres 1,2 and 3 of radii R/2,R and 2R respectively. If magnitudes of the electric fields at point P at a distance R from the centre of sphere 1, 2 and 3 are $E_1$, $E_2$ and $E_3$ respectively, then :<br/>

Question 112 :
Charges Q, 2Q and 4Q are uniformly distributed in three dielectric solid spheres 1, 2 and 3 of radii R/2, R and 2R respectively. If magnitudes of the electric fields at point P at a distance R from the centre of spheres 1, 2 and 3 are $E_1, E_2,$ and $ E_3$ respectively, then:<br/>

Question 113 :
The value of distance $r_m$, inside the ball at which electric field intensity maximum is given by-

Question 114 :
Let a total charge $2Q$ be distributed in a sphere of radius $R$, with the charge density given by $\rho(r) = kr$, where $r$ is the distance from the centre. Two charges $A$ and $B$, of $-Q$ each, are placed on diametrically opposite points, at equal distance, a form the centre. If $A$ and $B$ do not experience any force, then:

Question 115 :
Let there be a spherically symmetric charge distribution with charge density varying as $\displaystyle \mathrm{p}(\mathrm{r})=\mathrm{p}_{0}(\frac{5}{4}-\frac{\mathrm{r}}{\mathrm{R}})$ upto $\mathrm{r}=\mathrm{R}$, and $\mathrm{p}(\mathrm{r})=0$ for $\mathrm{r}&gt;\mathrm{R}$, where $\mathrm{r}$ is the distance from the origin. The electric field at a distance $\mathrm{r}(\mathrm{r}&lt;\mathrm{R})$ from the origin is given by :<br/>

Question 116 :
The magnitude of electric field as a function of the distance r inside the sphere is given by :

Question 117 :
A ball of radius R carries a positive charge whose volume charge density depends only on the distance r from the ball's centre as: $\rho=\rho_0(1-\dfrac {r}{R})$ where $\rho_0$ is constant. Assume $\epsilon$ as the permittivity of the ball.<br/>Then the magnitude of the electric field as a function of the distance r outside the ball is given by :

Question 118 :
A spherically symmetric charge distribution is characterised by a charge density having the following variation :<br>$p(r)=p_o(1-\frac {r}{R})$ for $r&lt;R$<br>$p(r)=0$ for $r\geqslant R$<br>Where r is the distance from the centre of the charge distribution and $p_o$ is a constant. The electric field at an internal point (r&lt;R) is :

Question 119 :
A sphere of radius R carries charge such that its volume charge density is proportional to the square of the distance from the center. What is the ratio of the magnitude of the electric field at distance 2R from the center to the magnitude of the electric field at a distance of R/2 from the center?

Question 121 :
The magnitude of the electric field as a function of the distanee r outside the ball is given by :

Question 123 :
The value of distance $r_m$ at which electric field intensity is maximum, is given by :

Question 124 :
Charges $Q_1$ and $Q_2$ lie inside and outside respectively of a closed surface S. Let E be the field at any point on S and $\phi$ be the flux of E over S.

Question 125 :
Compute the electric flux through a square surface of edges $2l$due to a charge $+Q$ whose geometric centre islocated on the x-axis at a perpendicular distance $l$ from the centre of the square.

Question 126 :
If the charge $+Q$ is now at the centre of a cube of side $2l$, what is the total flux emerging from all the six faces of the closedsurface?

Question 127 :
Electric field at the surface of a charged conductor is proportional to

Question 128 :
Given a uniform electric field $E=5 \times 10^3\hat i N/C$. Find the flux of this field through a square of side $10cm$ on a side whose plane is parallel to YZ-plane :

Question 129 :
The electric field in a region is radially outward with magnitude $E=\alpha r$. Calculate the charge contained in a sphere of radius R centered at the origin. Calculate the value of the charge if $\alpha =100 Vm^{-2}$ and R=0.30 m.

Question 130 :
Charges $Q_1$ and $Q_2$ lie inside and outside respectively of a closed surface S. Let E be the field at anypoint on S and $\phi$be the flux of E over S

Question 131 :
Charges $Q_1$ and $Q_2$ lie inside and outside respectively of a closed surface S. Let E be the field at any point on S and $\psi$ be the flux of E over S.

Question 132 :
Charges $Q_1$ &#160; and&#160; $Q_2$ lie inside and outside, respectively, of a closed surface S. Let E be the field at any point on S and $\phi$ be the flux of E over S.

Question 133 :
A point charge +q is placed at the centre of a cube of side L. The electric flux emerging from the cube is-

Question 134 :
The electric field in a region is $E = \displaystyle \frac{5\times 10^3 x}{2}\hat{i} \ NC^{-1} cm^{-1} $. The charge contained inside a cubical volume bounded by the surfaces $x = 0, x = 1, y = 0, y = 1, z = 0, z = 1$ is (where x, y, z are in cm) :

Question 135 :
A point charge $q$ is placed at a point on the axis of a non-conducting circular plate of radius $r$ at a distance $R (R &gt;&gt; r)$ from its center. The electric flux associated with the plate is :<br/>

Question 136 :
A solid sphere of radius $R$ has a charge $Q$ distributed in its volume with a charge density $\rho = kr^{a}$, where $k$ and $a$ are constants and $r$ is the distance from its centre. If the electric field at $r = \dfrac {R}{2}$ is $\dfrac {1}{8}$ times that at $r = R$, the value of $a$ is

Question 137 :
Fill in the blanks.<br/>A field normal to the plane of a circular wire n turns and radius r which carries a current I is measured on the axis of the coil at small h distance h from the centre of the coil. This is smaller than the field at the centre by a friction ____

Question 138 :
A particle of mass $m$ and charge $q$ at rest is released in a uniform electric field between parallel planes of charge $+q$ and $-q$ respectively. The particle accelerates towards the other place a distance $'d'$ away. The speed at which it strikes the opposite plane is:

Question 139 :
A long cylindrical wire carries a linear charge density of $3\times 10^{-8} Cm^{-1}$. An electron revolve around it in a circular path under the influence of the attractive force. $KE$ of the electron is :

Question 140 :
The electric field at a point $2$cm from an infinite line charge of linear charge density $10^{-7}$ $cm^{-1}$ is?

Question 141 :
Let $E_1(r), E_2(r)$ and $E_3(r)$ be the respective electric fields at a distance r from a point charge Q, an infinitely long wire with constant linear charge density $\lambda$, and an infinite plane with uniform surface charge density $&#160;\sigma $. lf $E_1(r_0) = E_2(r_0) = E_3(r_0)$ at a given distance $r_0$, then :<br/>

Question 142 :
A system consists of a thin charged wire ring of radius r and a very long uniformly charged wire oriented along the axis of the ring, with one of its ends coinciding with the center of the ring. The total charge on the ring is q, and the linear charge density on the straight wire is $\lambda$. The interaction force between the ring and the wire is :

Question 143 :
The expression for electric field intensity at a point outside uniformly charged thin place sheet is (d is the distance of point from plane sheet)

Question 144 :
Let E$_1$(r), E$_2$(r) and E$_3$(r) be the respective electric fields at a distance r from a point charge Q, an infinitely long wire with constant linear charge density $\lambda$, and an infinite plane with uniform surface charge density $\sigma$. If E$_1(r_0) = E_2 (r_0) = E_3 (r_0)$ at a given distance r$_0$, then :

Question 145 :
Two infinitely long parallel conducting plates having surface charge densities$\displaystyle +\sigma$ and$\displaystyle -\sigma$respectively, are separated by a small distance. The medium between the plates is vacuum. If$\displaystyle { \varepsilon }_{ 0 }$is the dielectric permittivity of vacuum then the electric field in the region between the plates is:

Question 146 :
Two parallel large thin metal sheets have equalsurface charge densities$\displaystyle \left( \sigma =26.4\times { 10 }^{ -12 }{ c }/{ { m }^{ 2 } } \right)$ of opposite signs.<br>The electric field between these sheets is:<br><br>

Question 147 :
Charges $Q_1$ and $Q_2$ are placed inside and outside respectively of an uncharged conducting shell. Their seperation is r.

Question 149 :
Find the electric field at a distance $x$ from the centreinside the shell.

Question 150 :
Two charged spheres having radii a and b are joined with a wire then the ratio of electric field $\dfrac{E_a}{E_b}$ on their surface is?