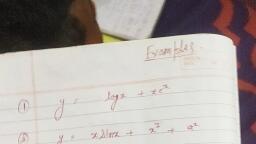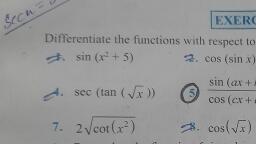Question 1 :
The set of points where the functions f given by $f(x)=|x-3|\cos x$ differentiable is
Question 2 :
The function $f(x)=x-[x]$, where $[\cdot]$ denotes the greatest integer function is?
Question 3 :
If $f(x)=\begin{cases}<br>ax &a<1&\\<br>ax^2+bx+2 &a\ge 1&.<br>\end{cases}$<br>Then the values of $a$, $b$ for which $f(x)$ is differentiable, are
Question 5 :
<span>Find the values of a and b so that the function $f(x)=\left\{\begin{matrix} x^2+3x+a, & if & x\leq 1\\ bx+2, & if & x > 1\end{matrix}\right.$ is differentiable at each $x\in R$.</span>
Question 7 :
The set of points where the function $f(x)=x|x|$ is differentiable is?
Question 10 :
<div>Consider the function $f(x)=\left\{\begin{matrix} x^2-5, & x\leq 3\\ \sqrt{x+13}, & x > 3\end{matrix}\right.$.<br/></div><div><br/></div>Consider the following statements.<br/>$1$. The function is discontinuous at $x=3$.<br/>$2$. The function is not differentiable at $x=0$.<br/>Which of the statements is$/$ are correct?
Question 11 :
If $\displaystyle f\left( x \right) =\begin{cases} \begin{matrix} { x }^{ p }\cos { \dfrac { 1 }{ x } } , & x\neq 0 \end{matrix} \\ \begin{matrix} 0, & x=0 \end{matrix} \end{cases}$, then at $x=0, f(x)$ is
Question 13 :
If $f(x)=\begin{cases} x+\left\{ x \right\} +x\sin { \left\{ x \right\};}\ x\neq 0 \\ 0;\ \quad x=0 \end{cases}$ where $\left\{ x \right\} $ denotes the fractional part function, then
Question 14 :
If $f:R \rightarrow (0, \infty)$ be a differentiable function $f(x)$ satisfying $f(x+ y) - f(x - y) = f(x) \{ f(y) - f(y) - y \}, \forall x, y \epsilon R, (f(y) \neq f(-y)$ for all $y \epsilon R)$ and $f' (0) = 2010$.<br>Now answer the following questions<br><br>Which of the following is true for $f(x)$
Question 15 :
Let $ \displaystyle f\left ( x \right )=\left | x \right |+\left | \sin x \right |in\left ( -\pi /2,3\pi /2 \right ) $. Then $ \displaystyle f $ is<br/>
Question 16 :
At point $x=1,$ the function $\displaystyle f\left( x \right) =\begin{cases} \begin{matrix} { x }^{ 3 }-1; & 1<x<\infty \end{matrix} \\ \begin{matrix} x-1; & -\infty <x\le 1 \end{matrix} \end{cases}$ is
Question 17 :
If $f(x)\begin{cases} \cfrac { x.\ln { \left( \cos { x } \right) } }{ \ln { \left( 1+{ x }^{ 2 } \right) } } \quad x\neq 0 \\ 0\quad \quad \quad \quad \quad \quad \quad \quad \quad x=0 \end{cases}$, then
Question 18 :
The domain of the derivative of the function $\displaystyle f\left ( x \right )= \left\{\begin{matrix}\tan ^{-1}x &for \left | x \right |\leq 1 \\\dfrac{1}{2}\left ( \left | x \right |-1 \right ) &for\left | x \right |> 1 \end{matrix}\right.$ is
Question 19 :
Assertion: Statement-1: $\displaystyle f(x) = \frac { 1 }{ \left\{ x \right\} } $ is discontinuous for integral values of $x$, where {} denotes the fractional part function.
Reason: Statement-2: For integral values of $x$, $f(x)$ is not defined.
Question 20 :
Let $[x]$ denote the greatest integer less than or equal to $x$. If $f(x)=\left [ x\sin\pi x \right ]$ , then $f(x)$ is :
Question 21 :
The function $ {f}( {x})=| {x}|+| {x}-1|$ is continuous at which of the following?<br/>
Question 22 :
If $ \displaystyle 0< a< 1 $ and $ \displaystyle f $ is defined as $ \displaystyle f\left ( x \right )=\left\{\begin{matrix} x^{a} & if x> 0\\ 0& if x=0\end{matrix}\right. $ then<br>
Question 23 :
<br>Let $\displaystyle \mathrm{f}(\mathrm{x})=\frac{\sin 4\pi[\mathrm{x}]}{1+[\mathrm{x}]^{2}},\mathrm{w}\mathrm{h}\mathrm{e}\mathrm{r}\mathrm{e} [\times]$ is the greatest integer less than or equal to x, then<br>
Question 25 :
Let $f\left( x \right)={ x }^{ 3 }-{ x }^{ 2 }+x+1$ and $g\left( x \right) =\begin{cases} \begin{matrix} \max { \left\{ f\left( t \right) \right\} } , & 0\le t\le x & 0\le x\le 1 \end{matrix} \\ \begin{matrix} 3-x, & 1<x\le 2 \end{matrix} \end{cases}$.<br/><br/> Then in the interval $\left[ 0,2 \right] $, $g\left( x \right) $ is
Question 26 :
If $f$ is defined by $f(x) = \left\{\begin{matrix} x,& for & 0 \leq x < 1\\ 2 - x & for &x\geq 1 \end{matrix}\right.$, then at $x = 1$, is:<br/>
Question 28 :
$ \displaystyle f\left ( x \right )=\left\{\begin{matrix}x^{2} & for \quad x \geq 1\\ ax+b & for \quad x< 1\end{matrix}\right. $ <div><br/></div><div>If $f$ is a differentiable function, then<br/></div>
Question 29 :
Assertion: The function $f\left ( x \right )=\begin{cases}<br><br>\left | x \right |+3 & \forall x\leq -3 \\ <br><br>-2x & \forall -3< x< 3 \\ <br><br>6x+2 & \forall x\geq 3 <br><br>\end{cases}$ is continuous for $-3\leq x< 3$ & $x> 3$ & non differentiable at $x=3$.
Reason: If $\lim_{x\rightarrow a}f\left ( x \right )=f\left ( a \right )$ then $f(x)$ is continuous and if L.H.D at $x=a$=R.H.D at $x=a$ then $f(x)$ is non differentiable at $x=0$.
Question 30 :
Assertion: If $f$ and $g$ are defined on $ \displaystyle \left [ 0,\infty \right ] $ by $ \displaystyle f\left ( x \right )=\lim_{n\rightarrow \infty }\frac{x^{n}-1}{x^{n}+1}$ and $g\left ( x \right )=\int_{0}^{x}f\left ( t \right )dt $ then $g$ is continuous but not differentiable at $x = 1$
Reason: $ \displaystyle f\left ( x \right )=sgn\left ( x-1 \right ) $
Question 32 :
Let $f\left( x \right) =\begin{cases} x\quad \quad \quad \quad \quad \quad \quad \quad x<1 \\ 2-x\quad \quad \quad \quad \quad \quad 1\le x\le 2 \\ -2+3x-{ x }^{ 2 }\quad \quad \quad x>2 \end{cases}$ then $f(x)$ is<br/>
Question 33 :
A function $f$ defined as $f(x)=x[x]$ for $-1\le x\le 3$ where $[x]$ defines the greatest integer $\le x$ is-
Question 34 :
Let $f(x)=x-x^2$ and $g(x)=\begin{cases}max\, f(t), 0\le t\le x, 0 \le, x \le 1\\ \sin \pi x, x > 1 \end{cases}$, then in the interval $[0, \infty)$
Question 35 :
Let $f(x)=\left [ \tan ^{2}x \right ]$, where [.] denotes the greatest integer function. Then
Question 36 :
Consider $f(x)=\begin{cases} \left[ \cfrac { 2\left( \sin { x } -\sin ^{ 3 }{ x } \right) +\left| \sin { x } -\sin ^{ 3 }{ x } \right| }{ 2\left( \sin { x } -\sin ^{ 3 }{ x } \right) -\left| \sin { x } -\sin ^{ 3 }{ x } \right| } \right] ,\quad x\neq \cfrac { \pi }{ 2 } \quad for\quad x\in \left( 0,\pi \right) \\ 3\quad \quad \quad \quad \quad \quad \quad \quad \quad \quad \quad x=\cfrac { \pi }{ 2 } \end{cases}$, where $[]$ denotes the greatest integer function, then-
Question 37 :
Let $f:(-1,1)\rightarrow R$ be a differentiable function satisfying <br> $(f'(x))^4=16(f(x))^2$ for all $x\in (-1,1)$<br> $f(0)=0$<br>The number of such functions is
Question 38 :
If f (x + y) = 2 f(x) f(y) all x, y $\in$ R where f' (0) = 3 and f (4) =2, then f'(4) is equal to
Question 39 :
Let $f(x) = \left\{\begin{matrix}2a - x, &if\ -a < x < a \\ 3x - 2a, &if\ a \leq x \end{matrix}\right.$. Then, which of the following is true?<br>
Question 41 :
Let $f(x) = [x]$ and $g(x) = \left\{\begin{matrix}0, & x \in Z\\ x^2, & x \in R - Z \end{matrix}\right.$. Then which of the following is not true ([.] represents the greatest integer function)?
Question 42 :
If $f(x) =\begin{cases} 2x^2+12x+16&, -4\le x\le -2\\2-|x|& , -2 < x \le 1\\4x-x^2-2& , 1< x \le 3 \end{cases}$. Then $f(x)$ is
Question 43 :
Let $f:\left[ {0,2} \right] \to R$ a function which is continuous on $\left[ {0,2} \right]$ and is differentiable on $\left( {0,2} \right)$ with $f\left( 0 \right) = 1$. Let $F\left( x \right) = \int\limits_0^{{x^2}} {f\left( {\sqrt t } \right)} dt,$ for $x \in \left[ {0,2} \right]$, if $F'\left( x \right) = f'\left( x \right),\forall x \in \left( {0,2} \right),$ then $F(2)$ equals to
Question 44 :
Let $\mathrm{f}:\mathrm{R}\rightarrow \mathrm{R}$ be any function. Define $\mathrm{g}:\mathrm{R}\rightarrow \mathrm{R}$ by $g(x)=|f(x)| $for all $x$. Then $g$ is<br/>
Question 45 :
Let [x] denote the greatest integer less than or equal to x.<br>If $f\left ( x \right )=\left [ x\sin \pi x \right ]$, then f(x) is<br>
Question 46 :
Given $f(x)=\cos ^{ -1 }{ \left( sgn\left( \cfrac { 2\left| x \right| }{ 3x-\left| x \right| } \right) \right) } $ where $sgn(.)$ denotes the signum function $\left[ \cdot \right] $ denotes the greatest integer function. Discuss the continuity and differentiablity at $x=\pm 1$
Question 47 :
Let $f(x)=[x]^{2}+\sqrt{\{x\}}$ where [] & {}respectively denotes the greatest integer and fractional part functions, <span>then which of the following is correct?</span>
Question 48 :
<u></u>Let $f : R \rightarrow R$ be a continuous function such that $f(x^2) = f(x^3)$ for all $ x \in R$. Consider the following statements.<br>I. f is an odd function.<br>II. f is an even function.<br>III. f is differentiable everywhere
Question 50 :
The function $f(x)=\begin{cases} ax(x-1)+b\quad \quad when\quad x<1 \\ x-1\quad \quad \quad when\quad \quad 1\le x\le 3 \\ p{ x }^{ 2 }+qx+2\quad \quad when\quad x>3 \end{cases}$ Find the values of the constants $a,b,p,q$ so that $(i)f(x)$ is continuous for all $x$ $(ii)f'(1)$ does not exist $(iii)f'(x)$ is continuous at $x=3$