Question 1 :
For the Hyperbola {tex} \frac { x ^ { 2 } } { \cos ^ { 2 } \alpha } - \frac { y ^ { 2 } } { \sin ^ { 2 } \alpha } = 1 , {/tex} which of the following remains constant when {tex} \alpha {/tex} varies?
Question 2 :
Let {tex} A ( h , k ) , B ( 1,1 ) {/tex} and {tex} C ( 2,1 ) {/tex} be the vertices of a right angled triangle with {tex} A C {/tex} as its hypotenuse. If the area of the triangle is 1 square unit, then the set of values which {tex} ^ { \prime } k ^ { \prime } {/tex} can take is given by
Question 3 :
A point on the parabola {tex} y ^ { 2 } = 18 x {/tex} at which the ordinate increases at twice the rate of the abscissa is
Question 4 :
If the sum of the slopes of the lines given by {tex} x ^ { 2 } - 2 c x y - 7 y ^ { 2 } = 0 {/tex} is four times their product, then {tex} c {/tex} has the value
Question 5 :
A parabola has the origin as its focus and the line {tex} x = 2 {/tex} as the directrix. Then the vertex of the parabola is at
Question 6 :
If <img style='object-fit:contain' src="https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5ea7bb2a6f3020298ca12b5c"> , then the points <img style='object-fit:contain' src="https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5ea7bb2ac2a2ae2953d936a4"> and <img style='object-fit:contain' src="https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5ea7ba54c2a2ae2953d9346c"> are
Question 7 :
The feet of the perpendicular drawn from <img style='object-fit:contain' src="https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5ea7ba066f3020298ca1287d"> to the sides of a <img style='object-fit:contain' src="https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5ea7ba17ab3481716f4b61cc"> are collinear, then <img style='object-fit:contain' src="https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5ea7ba066f3020298ca1287d"> is
Question 8 :
Circumcentre of triangle whose vertices are (0, 0), (3, 0) and (0, 4) is
Question 9 :
The normal to the curve {tex} x = a ( 1 + \cos \theta ) , y = a \sin \theta {/tex} at {tex} \theta {/tex} always passes through the fixed point
Question 10 :
If the vertices of a triangle have integral coordinates, the triangle cannot be
Question 11 :
If the sum of the distance of a point <img style='object-fit:contain' src="https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5ea7ba066f3020298ca1287d"> from two perpendicular lines in a plane is 1, then the locus of <img style='object-fit:contain' src="https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5ea7ba066f3020298ca1287d"> is a
Question 12 :
If {tex} \left( a , a ^ { 2 } \right) {/tex} falls inside the angle made by the lines {tex} y = \frac { x } { 2 } , x > 0 {/tex} and {tex} y = 3 x , x > 0 , {/tex} then {tex} a {/tex} belongs to
Question 13 :
If one of the lines of {tex} m y ^ { 2 } + \left( 1 - m ^ { 2 } \right) x y - m x ^ { 2 } = 0 {/tex} is a bisector of the angle between the lines {tex} x y = {/tex} {tex} 0 , {/tex} then {tex} m {/tex} is
Question 14 :
The equation of the straight line passing through the point {tex} ( 4,3 ) {/tex} and making intercepts on the coordinate axes whose sum is {tex} - 1 {/tex} is
Question 15 :
The equation of a tangent to the parabola {tex} y ^ { 2 } = 8 x {/tex} is {tex} y = x + 2 . {/tex} The point on this line from which the other tangent to the parabola is perpendicular to the given tangent is
Question 16 :
The centre of the circle passing through {tex} ( 0,0 ) {/tex} and {tex} ( 1,0 ) {/tex} and touching the circle {tex} x ^ { 2 } + y ^ { 2 } = 9 {/tex} is
Question 17 :
The equation of a circle with origin as a centre and passing through equilateral triangle whose median is of length {tex} 3 a {/tex} is
Question 18 :
The point diametrically opposite to the point {tex} P ( 1 , {/tex} {tex} 0 ) {/tex} on the circle {tex} x ^ { 2 } + y ^ { 2 } + 2 x + 4 y - 3 = 0 {/tex} is
Question 19 :
The eccentricity of an ellipse, with its centre at the origin, is {tex} 1 / 2 . {/tex} If one of the directrices is {tex} x = 4 , {/tex} then the equation of the ellipse is:
Question 20 :
Consider a family of circles which are passing through the point {tex} ( - 1,1 ) {/tex} and are tangent to {tex} x {/tex} - axis. If {tex} ( h , k ) {/tex} are the coordinate of the centre of the circles, then the set of values of {tex} k {/tex} is given by the interval
Question 21 :
An ellipse has {tex} O B {/tex} as semi minor axis, {tex} F {/tex} and {tex} F {/tex} its focii and the angle {tex} F B F {/tex} is a right angle. Then the eccentricity of the ellipse is
Question 22 :
The lines {tex} 2 x - 3 y = 5 {/tex} and {tex} 3 x - 4 y = 7 {/tex} are diameters of a circle having area as 154 sq. units. Then the equation of the circle is
Question 23 :
If the lines {tex} 3 x - 4 y - 7 = 0 {/tex} and {tex} 2 x - 3 y - 5 = 0 {/tex} are two diameters of a circle of area {tex} 49 \pi {/tex} square units, then the equation of the circle is
Question 24 :
If a circle passes through the point {tex} ( a , b ) {/tex} and cuts the circle {tex} x ^ { 2 } + y ^ { 2 } = 4 {/tex} orthogonally, then the locus of its centre is
Question 25 :
If {tex} a \neq 0 {/tex} and the line {tex} 2 b x + 3 c y + 4 d = 0 {/tex} passes through the points of intersection of the parabolas {tex} y ^ { 2 } = 4 a x {/tex} and {tex} x ^ { 2 } = 4 a y , {/tex} then
Question 26 :
A variable circle passes through the fixed point {tex} A ( p , q ) {/tex} and touches {tex} x {/tex} -axis. The locus of the other end of the diameter through {tex} A {/tex} is
Question 27 :
The normal to a curve at {tex} P ( x , y ) {/tex} meets the {tex} x {/tex} -axis at {tex} G . {/tex} If the distance of {tex} G {/tex} from the origin is twice the abscissa of {tex} P , {/tex} then the curve is a
Question 28 :
The point of lines represented by {tex} 3 a x ^ { 2 } + 5 x y + \left( a ^ { 2 } - 2 \right) y ^ { 2 } = 0 {/tex} and {tex} \perp {/tex} to each other for
Question 29 :
Locus of mid point of the portion between the axes of {tex} x \cos \alpha + y \sin \alpha = p {/tex} where {tex} p {/tex} is constant is
Question 30 :
The normal at the point {tex} \left( b t _ { 1 } ^ { 2 } , 2 b t _ { 1 } \right) {/tex} on a parabola meets the parabola again in the point {tex} \left( b t _ { 2 } ^ { 2 } , 2 b t _ { 2 } \right) , {/tex} then
Question 31 :
The line parallel to the {tex} x {/tex} -axis and passing through the intersection of the lines {tex} a x + 2 b y + 3 b = 0 {/tex} and {tex} b x - 2 a y - 3 a = 0 , {/tex} where {tex} ( a , b ) \neq ( 0,0 ) {/tex} is
Question 32 :
The point which divides the joint of ( 1, 2 ) and ( 3,4 ) externally in the ratio 1 : 1 .
Question 33 :
The centres of a set of circles, each of radius {tex} 3 , {/tex} lie on the circle {tex} x ^ { 2 } + y ^ { 2 } = 25 . {/tex} The locus of any point in the set is
Question 34 :
The intercept on the line {tex} y = x {/tex} by the circle on {tex} x ^ { 2 } + y ^ { 2 } - 2 x = 0 {/tex} is {tex} A B {/tex}. Equation of the circle on {tex} A B {/tex} as a diameter is
Question 35 :
A straight line through the point {tex} A ( 3,4 ) {/tex} is such that its intercept between the axes is bisected at {tex} A . {/tex} Its equation is,
Question 36 :
The perpendicular bisector of the line segment joining {tex} P ( 1,4 ) {/tex} and {tex} Q ( k , 3 ) {/tex} has {tex} y {/tex} -intercept {tex} - 4 . {/tex} Then a possible value of {tex} k {/tex} is
Question 37 :
If the circles {tex} x ^ { 2 } + y ^ { 2 } + 2 a x + c y + a = 0 {/tex} and {tex} x ^ { 2 } + y ^ { 2 } - 3 a x + d y - 1 = 0 {/tex} intersect in two distinct points {tex} P {/tex} and {tex} Q {/tex} then the line {tex} 5 x + b y - a = 0 {/tex} passes through {tex} P {/tex} and {tex} Q {/tex} for
Question 38 :
If the plane 2ax - 3ay + 4az + 6 = 0 passes through the mid-point of the line joining the centres of the spheres x<sup>2</sup> + y<sup>2</sup> + z<sup>2</sup> + 6x - 8y - 2z = 13 and x<sup>2</sup> + y<sup>2</sup> + z<sup>2</sup> - 10x + 4y - 2z = 8, then a equals
Question 39 :
If the equation of the locus of point equidistant from the points {tex} \left( a _ { 1 } , b _ { 1 } \right) {/tex} and {tex} \left( a _ { 2 } , b _ { 2 } \right) {/tex} is {tex} \left( a _ { 1 } - a _ { 2 } \right) x + \left( b _ { 1 } - b _ { 2 } \right) y + c = 0 , {/tex} then {tex} c = {/tex}
Question 40 :
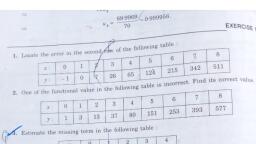
The points scored by a basketball team in a series of matches are as follows:<br>{tex} 15,3,8,10,22,5,27,11,12,19,18,21,13,14 {/tex} Its median is<br>
Question 41 :
If in a frequency distribution, the mean and median are 21 and 22 respectively, then its mode is approximately
Question 42 :
The weighted mean of first {tex} n {/tex} natural numbers whose weights are equal is given by
Question 43 :
A box contains 24 identical balls of which 12 are white and 12 are black. The balls are drawn at random from the box one at a time with replacement. The probability that a white ball is drawn for the 4th time on the 7th draw is
Question 44 :
The A.M. of {tex} n {/tex} numbers of a series is {tex} \overline { X } . {/tex} If the sum of first {tex} ( n - 1 ) {/tex} terms is {tex} k , {/tex} then the {tex} n {/tex} th number is
Question 45 :
The probability of having at least one tail in 4 throws with a coin is
Question 46 :
If a variable takes the discrete value {tex} \alpha + 4 , \alpha - 7 / 2 , \alpha - 5 / 2 , \alpha - 3 , {/tex} {tex} \alpha - 2 , \alpha + 1 / 2 , \alpha - 1 / 2 , \alpha + 5 , ( \alpha > 0 ) , {/tex} then the median is
Question 47 :
A committee of seven is chosen at random from 10 men and 10 women, then the probability that the men will have majority in the committee.
Question 48 :
In the above problem, the probability that A<sub>3</sub> reaches final is
Question 49 :
The expenditure of a family for a certain month were as follows:<br>Food - Rs.560, Rent - Rs.420, Clothes - Rs.180, Education - Rs.160, Other items - Rs.120<br>A pie graph representing this data would show the expenditure for clothes by a sector whose angle equals
Question 50 :
A letter is selected from each of the words PROBABILITY and GEOMETRY. Then the probability that both are vowels?
Question 51 :
Geometric mean of {tex} 1,2,2 ^ { 2 } , 2 ^ { 3 } , \ldots , 2 ^ { n } {/tex} is
Question 52 :
A fair die is thrown until a score of less than 5 points is obtained. The probability of obtaining not less than 2 points on the last thrown is
Question 54 :
The probability that a non-leap year has 53 Sundays is -
Question 55 :
The S.D of 15 items is 6 and if each item is decreased or increased by 1, then standard deviation will be
Question 56 :
A dice is tossed 5 time. Getting an odd number is considered a success. Then the variance of distribution of success is
Question 57 :
The standard deviation of 25 numbers is 40. If each of the numbers is increased by 5, then the new standard deviation will be
Question 59 :
If P (a) = 0.4, P (b) = x, & P(A ∪ B)= 0.7 and the event A & B are mutually exclusive then x =
Question 60 :
If mode of a data is 27 and mean is {tex} 24 , {/tex} then median is
Question 61 :
The average marks of boys in class is {tex}52{/tex} and that of girls is {tex} 42 . {/tex} The average marks of boys and girls combined is {tex} 50 . {/tex} The percentage of boys in the class is<br>
Question 62 :
A letter is known to have come from either TATANAGAR or CALCUTTA. On the envelope, just two consecutive letters TA are visible . The probability that the letter has come from CALCUTTA is
Question 63 :
A party of 23 persons take their seats at a round table. The odds against two persons sitting together are
Question 64 :
In a toss of two dice, A throws a total of 5. The probability that he will throw another 5 before he throws 10 is
Question 65 :
In order to get at least once a head with probability ≥0.9, the number of times a coin needs to be tossed is
Question 66 :
If a variate {tex} X {/tex} is expressed as a linear function of two variates {tex} U {/tex} and {tex} V {/tex} in the form {tex} X = a U + b V , {/tex} then mean {tex} \overline { X } {/tex} of {tex} X {/tex} is
Question 67 :
There are four machines and it is known that exactly two of them are faulty. They are tested, one by one, in a random order till both the faulty machines are identified. Then the probability that only two tests are needed is:
Question 69 :
The A.M. of {tex} n {/tex} numbers of a series is {tex} \overline { X } . {/tex} If the sum of first {tex} ( n - 1 ) {/tex} terms is {tex} k , {/tex} then the {tex} n {/tex} th number is
Question 70 :
A question (sum) is given to three boys whose chances of solving it are 1/3, 1/4 and 1/5 respectively. What is the probability that the question will be solved?
Question 71 :
The median of a set of {tex} 9 {/tex} distinct observations is {tex} 20.5 . {/tex} If each of the largest {tex}4{/tex} observations of the set is increased by {tex} 2 , {/tex} then the median of the new set
Question 72 :
For a set of 100 observations, taking assumed mean as {tex} 4 , {/tex} the sum of the deviations is {tex} - 11 \mathrm { cm } , {/tex} and the sum of the squares<br>of these deviations is {tex} 275 \mathrm { cm } ^ { 2 } . {/tex} The coefficient of variation is<br>
Question 73 :
In a certain population 10{tex} \% {/tex} of the people are rich, 5{tex} \% {/tex} are famous and 3{tex} \% {/tex} are rich and famous. The probability that a person picked at random from the population is either famous or rich but not both, is equal to
Question 74 :
For a set of 100 observations, taking assumed mean as {tex} 4 , {/tex} the sum of the deviations is {tex} - 11 \mathrm { cm } , {/tex} and the sum of the squares of these deviations is {tex} 275 \mathrm { cm } ^ { 2 } . {/tex} The coefficient of variation is<br>
Question 75 :
A box contains 2 blue caps, 4 red caps, 5 green caps and 1 yellow cap. <br>If four caps are picked at random, what is the probability that none is green ?
Question 76 :
Let two fair six-faced dice {tex} A {/tex} and {tex} B {/tex} be thrown simultaneously. If {tex} E _ { 1 } {/tex} is the event that dice {tex} A {/tex} shows up four, {tex} E _ { 2 } {/tex} is the event that dice {tex} B {/tex} shows up two and {tex} E _ { 3 } {/tex} is the event that the sum of numbers on both dice is odd, then which of the following statements is NOT true?
Question 77 :
The mean of following frequency table is {tex} 50 . {/tex}<br>{tex} \begin{array} { | c | c | } \hline \text { Class } & { \text { Frequency } } \\ \hline 0 - 20 & { 17 } \\ { 20 - 40 } & { f _ { 1 } } \\ { 40 - 60 } & { 32 } \\ { 60 - 80 } & { f _ { 2 } } \\ { 80 - 80 } & { 19 } \\ \hline \text { Total } & { 120 } \\ \hline \end{array} {/tex}<br>The missing frequencies are
Question 78 :
The probability that a leap year selected at random contains either 53 Sundays or 53 Mondays, is
Question 79 :
Five horses are in a race. Mr. A selects two of the horses at random and bets on them. The probability that Mr. {tex} A {/tex} selected the winning horse is
Question 80 :
Study the given information carefully and answer the questions that follow.<br>A basket contains 4 red, 5 blue and 3 green marbles. If three marbles are picked at random, what is the probability that at least one is blue?
Question 81 :
An experiment succeeds twice as often as it fails. The probability of at least five successes in the six trials of this experiment is
Question 82 :
Three boxes are labelled A, B and C and each box contains four balls numbered 1, 2, 3 and 4. The balls in each box are well mixed. A child chooses one ball at random from each of the three boxes. If a, b and c are the numbers on the balls chosen from the boxes A, B and C resepctively, the child wins a toy helicopter when a = b + c. The odds in favour of the child to receive the toy helicopter are
Question 83 :
A random variable {tex} X {/tex} has Poisson distribution with mean {tex} 2 . {/tex} The {tex} P ( X > 1.5 ) {/tex} equals
Question 84 :
The mean of following frequency table is {tex} 50 . {/tex}<br>{tex} \begin{array} { | c | c | } \hline \text { Class } & { \text { Frequency } } \\ \hline 0 - 20 & { 17 } \\ { 20 - 40 } & { f _ { 1 } } \\ { 40 - 60 } & { 32 } \\ { 60 - 80 } & { f _ { 2 } } \\ { 80 - 80 } & { 19 } \\ \hline \text { Total } & { 120 } \\ \hline \end{array} {/tex}<br>The missing frequencies are
Question 85 :
If a dice is thrown twice, then the probability of occurrence of 4 at least once is
Question 86 :
A bag contains 19 tickets numbered from 1 to 19. A ticket is drawn and then another ticket is drawn without replacement. The probability that both the tickets will show even number, is
Question 87 :
Suppose a population {tex} A {/tex} has {tex}100{/tex} observations {tex} 101 , {/tex} {tex} 102 , \ldots , 200 , {/tex} and another population {tex} B {/tex} has {tex}100{/tex} observations {tex} 151,152 , \ldots , 250 . {/tex} If {tex} V _ { A } {/tex} and {tex} V _ { B } {/tex} represent the variances of the two populations, respectively,then {tex} V _ { A } / V _ { B } {/tex} is
Question 88 :
The probability of happening an event {tex} A {/tex} is {tex}0.5 {/tex} and that of {tex} B {/tex} is {tex} 0.3 . {/tex} If {tex} A {/tex} and {tex} B {/tex} are mutually exclusive events, then the probability of happening neither {tex} A {/tex} nor {tex} B {/tex} is
Question 89 :
A box contains 5 green, 4 yellow and 3 white marbles. 3 marbles are drawn at random. What is the probability that they are not of the same colour?
Question 90 :
If the mean of five observations x, x + 2, x + 4, x + 6 and x + 8 is 11, then the mean of last three observations is
Question 91 :
If X is a poisson variate such that P(X=1) = P(X=2), then P(X = 4) is equal to
Question 92 :
There are 5 duplicate and 10 original items in an automobile shop and 3 items are brought at random by a customer. The probability that none of the items is duplicate, is
Question 93 :
For the three events A, B and C, P (exactly one of the events A or B occurs)= P(exactly one of the events B or C occurs) − P (exactly one of the events C or A occurs) = p and P ( all the three events occur simultaneously) = $p^{2},\ \text{where}\ 0 < p < \frac{1}{2}$. Then, the probability of at least one of the three events A, B and C occuring, is
Question 94 :
The correlation coefficient of two variable x and y is 0.8. The regression coefficient of y on x is 0.2, than the regression coefficient of x on y is
Question 95 :
If two dice are thrown together, then the probability that the sum of numbers appearing on them is 9, is
Question 96 :
If a variable takes values 0, 1, 2, …, n with frequencies 1, <sup>n</sup>C<sub>1</sub>, <sup>n</sup>C<sub>2</sub>, …, <sup>n</sup>C<sub>n</sub>, then the AM is
Question 97 :
<p>The probability distribution of a random variable X is given as</p> <table> <thead> <tr> <th><br/><strong>X</strong><br/></th> <th>-5</th> <th>-4</th> <th>-3</th> <th>-2</th> <th>-1</th> <th><br/>0<br/></th> <th><br/>1<br/></th> <th><br/>2<br/></th> <th><br/>3<br/></th> <th><br/>4<br/></th> <th><br/>5<br/></th> </tr> </thead> <tbody> <tr> <td><strong>P</strong><strong>(</strong><strong>X</strong><strong>)</strong></td> <td>p</td> <td>2p</td> <td>3p</td> <td>4p</td> <td>5p</td> <td>7p</td> <td>8p</td> <td>9p</td> <td>10p</td> <td>11p</td> <td>12p</td> </tr> </tbody> </table> <p>Then, the value of P is</p>
Question 98 :
The probability that a number n chosen at random from 1 to 30, to satisfy n + (50/n) > 27 is
Question 99 :
The mean of the series x<sub>1</sub>, x<sub>2</sub>, …, x<sub>n</sub> is $\overline{X}.$ If x<sub>2</sub> is replaced by λ, then the new mean is
Question 100 :
If $P\left( A \cap B \right) = \frac{1}{2},P\left( \overline{A} \cap \overline{B} \right) = \frac{1}{3},P\left( A \right) = p,P\left( B \right) = 2\ p,$ then the value of p is given by
Question 101 :
If A and B are independent events and P(C) = 0, then
Question 102 :
In a certain population 10% of the people are rich, 5% are famous and 3% are rich and famous. The probability that a person picked at random from the population is either famous or rich but not both, is equal to
Question 103 :
A binary operation is chosen at random from the set of all binary operations on a set A containing n elements. The probability that the binary operation is commutative, is
Question 104 :
Fifteen coupons are numbered 1 to 15. Seven coupons are selected at random, one at a time with replacement. The probability that the largest number appearing on a selected coupon be 9, is
Question 105 :
The average of the squares of the numbers 0, 1, 2, 3, 4, ..., n is
Question 106 :
The probability that a candidate secures a seat in Engineering through “EAMCET” is 1/10. 7 candidates are selected at random from a centre. The probability that exactly two will get seats is
Question 107 :
A coin is tossed three times. The probability of getting head and tail alternatively, is
Question 108 :
The average of the four-digit numbers that can be formed using each of the digits 3, 5, 7 and 9 exactly once in each number, is
Question 109 :
If A and B are two independent events, the probability that both A and B occur is 1/8 and the probability that neither of them occurs is 3/8. The probability of the occurrence of A, is
Question 111 :
If p + q + r = 0 = a + b + c, Then the value of the determinant <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e86f86c19f8d44d3a17ec5d' height='63' width='75' > is
Question 112 :
The number of non-zero diagonal matrices of order 4 satisfying {tex} A ^ { 2 } = A {/tex} is
Question 113 :
In a symmetric matrix of order '12' maximum number of different elements are -
Question 114 :
If A = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e86fa2675ed294f2c7c3569' height='63' width='76' > and B = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e86fa2719f8d44d3a17eecc' height='63' width='75' >, then the element of 3<sup>rd</sup> row and third column in AB will be
Question 115 :
The number of values of 'k', for which the system x = 2y + kz = 1 2x = ky + 8z = 3 does not have a solution, is -
Question 116 :
Let A be square matrix of order 3 such that |A| = 5. Then |adj(adjA)| =
Question 117 :
The cofactor of the element 4 in the determinant <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e86f8e919f8d44d3a17ed1c' height='75' width='75' > is
Question 118 :
Let {tex} f ( x ) = \left| \begin{array} { c c c } { x } & { 1 } & { 1 } \\ { \sin 2 \pi x } & { 2 x ^ { 2 } } & { 1 } \\ { x ^ { 3 } } & { 3 x ^ { 4 } } & { 1 } \end{array} \right| {/tex}. If {tex} f ( x ) {/tex} be an odd function and its odd value is equal to {tex} g ( x ) , {/tex} then find the value of {tex} \lambda {/tex}. Also {tex} f ( 1 ) {/tex} {tex} g ( 1 ) = - 4 \lambda {/tex}
Question 119 :
If <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e86f98475ed294f2c7c3495' height='63' width='85' > = x + iy, then
Question 120 :
If matrix A = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e86f8b2e6d3604eaa92def6' height='63' width='63' >where a, b, c are real positive numbers, abc = 1 and A<sup>T</sup> A = 1, then the value of a<sup>3</sup> + b<sup>3</sup> + c<sup>3</sup> is -
Question 121 :
If A<sup>2</sup> = A, then (I + A)<sup>4</sup> is equal to -
Question 124 :
The value of the determinanat <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e86f99875ed294f2c7c34b1' height='68' width='165' > is -
Question 125 :
If A<sub>r </sub>= <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e86f89e19f8d44d3a17ecac' height='41' width='72' >where r is a natural number then |A<sub>1</sub>| + |A<sub>2</sub>| + |A<sub>3</sub>| + ......+ |A<sub>2006</sub>| must be equal to
Question 126 :
If a, b, c be positive and not all equal, then the value of the determinant <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e86f866e6d3604eaa92de7b' height='63' width='60' > is -
Question 127 :
If k is a scalar and A is an n × n square matrix, then |kA| =
Question 128 :
The system of equations x + ky + 3z = 0, 3x + ky -2z = 0, 2x + 3y - 4z = 0 possess a non-trivial solution over the set of rationals, then 2k, is an integral element of the interval:
Question 129 :
If a, b, c, d, e and f are in G.P. then the value of <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e86f9ad19f8d44d3a17ee32' height='68' width='77' >depends on -
Question 130 :
Let matrix A = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e86f8d419f8d44d3a17ecfe' height='63' width='68' >where a, b, c are real positive numbers with abc = 1 if A<sup>T</sup>A = I, then a<sup>3</sup> + b<sup>3</sup> + c<sup>3</sup> =
Question 131 :
If a<sub>1</sub>, a<sub>2</sub>, a<sub>3</sub>, ............., a<sub>n</sub>, ........ are in GP, then the value of the determinant <img style='object-fit:contain' src="https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5ec8e39014bbd053c6532bee" width=148 height=57 /> is
Question 132 :
If A = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e86fa07e6d3604eaa92e0c1' height='63' width='89' >, then adj A =
Question 133 :
If B is a non-singular matrix and A is a square matrix, then det (B<sup>-1</sup> AB) is equal to -
Question 135 :
If <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e86f9c075ed294f2c7c34ee' height='68' width='99' > = (a - b) (b - c) (c - a), then n is equal to
Question 136 :
If the system of equation 2x + 5y + 8z = 0 x + 4y + 7z = 0 6x + 4y - λz = 0 has a non trivial solution then λ is equal to -
Question 137 :
The rotation through <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e86f93819f8d44d3a17ed8d' height='17' width='29' >is identical to
Question 138 :
If ω be a complex cube root of unity, then <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e86f87c19f8d44d3a17ec75' height='68' width='97' > is equal to-
Question 139 :
In a third order determinant, each element of the first column consists of sum of two terms, each element of the second column consists of sum of three terms and each element of third column consists of sum of four terms. Then, it can be decomposed in n determinants, where n has the value
Question 140 :
If {tex} A = \left[ \begin{array} { l l l } { 0 } & { 0 } & { 0 } \\ { 1 } & { 0 } & { 0 } \\ { 0 } & { 1 } & { 0 } \end{array} \right] , {/tex} then
Question 141 :
Let {tex} A {/tex} be a {tex} 3 \times 3 {/tex} matrix such that<br>{tex} A \left[ \begin{array} { l l l } { 1 } & { 2 } & { 3 } \\ { 0 } & { 2 } & { 3 } \\ { 0 } & { 1 } & { 1 } \end{array} \right] = \left[ \begin{array} { l l l } { 0 } & { 0 } & { 1 } \\ { 1 } & { 0 } & { 0 } \\ { 0 } & { 1 } & { 0 } \end{array} \right] {/tex}<br>Then {tex} A ^ { - 1 } {/tex} is
Question 142 :
Let {tex} f ( x ) = \left| \begin{array} { c c c } { 2 \cos ^ { 2 } x } & { \sin 2 x } & { - \sin x } \\ { \sin 2 x } & { 2 \sin ^ { 2 } x } & { \cos x } \\ { \sin x } & { - \cos x } & { 0 } \end{array} \right| . {/tex} Then the value of {tex} \int \limits_ { 0 } ^ { \pi / 2 } \left[ f ( x ) + f ^ { \prime } ( x ) \right] d x {/tex} is
Question 143 :
Given that matrix {tex} A = \left[ \begin{array} { l l l } { x } & { 3 } & { 2 } \\ { 1 } & { y } & { 4 } \\ { 2 } & { 2 } & { z } \end{array} \right] {/tex}. If {tex} x y z = 60 {/tex} and {tex} 8 x + 4 y + 3 z {/tex} {tex} = 20 , {/tex} then {tex} A ( \text { adj } A ) {/tex} is equal to
Question 144 :
If <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e86f98e75ed294f2c7c34a0' height='75' width='171' > = A<sub>0</sub>+A<sub>1</sub>x+A<sub>2</sub>x<sup>2</sup> + A<sub>3</sub>x<sup>3</sup>, then A<sub>0</sub> is equal to-
Question 145 :
The matrix {tex} \dot { X } {/tex} for which {tex} \left[ \begin{array} { c c } { 1 } & { - 4 } \\ { 3 } & { - 2 } \end{array} \right] X = \left[ \begin{array} { c c } { - 16 } & { - 6 } \\ { 7 } & { 2 } \end{array} \right] {/tex} is
Question 146 :
If {tex} x \neq 0 , y \neq 0 , z \neq 0 {/tex} and {tex} \left| \begin{array} { c c c } { 1 + x } & { 1 } & { 1 } \\ { 1 + y } & { 1 + 2 y } & { 1 } \\ { 1 + z } & { 1 + z } & { 1 + 3 z } \end{array} \right| = 0 , {/tex} then {tex} x ^{-1} + y^{-1}+z^{-1}{/tex} is equal to
Question 147 :
If {tex} A = \left[ \begin{array} { c c } { i } & { - i } \\ { - i } & { i } \end{array} \right] {/tex} and {tex} B = \left[ \begin{array} { c c } { 1 } & { - 1 } \\ { - 1 } & { 1 } \end{array} \right] , {/tex} then {tex} A ^ { 8 } {/tex} equals
Question 148 :
The product of matrices {tex} A = \left[ \begin{array} { c c } { \cos ^ { 2 } \theta } & { \cos \theta \sin \theta } \\ { \cos \theta \sin \theta } & { \sin ^ { 2 } \theta } \end{array} \right] {/tex} and {tex} B = \left[ \begin{array} { c c } { \cos ^ { 2 } \phi } & { \cos \phi \sin \phi } \\ { \cos \phi \sin \phi } & { \sin ^ { 2 } \phi } \end{array} \right] {/tex} is a null matrix if {tex} \theta - \phi = {/tex}
Question 149 :
Let {tex} a , b , c {/tex} be cube roots of unity and {tex} \Delta = \left| \begin{array} { c c c } { a ^ { 2 } + b ^ { 2 } } & { c ^ { 2 } } & { c ^ { 2 } } \\ { a ^ { 2 } } & { b ^ { 2 } + c ^ { 2 } } & { a ^ { 2 } } \\ { b ^ { 2 } } & { b ^ { 2 } } & { c ^ { 2 } + a ^ { 2 } } \end{array} \right| , {/tex} then
Question 150 :
If {tex} A = \left[ \begin{array} { c c } { 2 } & { 3 } \\ { 5 } & { - 2 } \end{array} \right] , {/tex} then {tex} A ^ { - 1 } {/tex} is equal to
Question 151 :
If {tex}a, b, c{/tex} are non-zero real numbers and if the equations {tex}(a - 1)x = y + z, (b - 1)y = z + x, (c - 1)z = x + y{/tex} have a non-trivial solution, then {tex}ab + bc + ca{/tex} equals
Question 152 :
{tex} A {/tex} is a {tex} 2 \times 2 {/tex} matrix such that {tex} A \left[ \begin{array} { c } { 1 } \\ { - 1 } \end{array} \right] = \left[ \begin{array} { c } { - 1 } \\ { 2 } \end{array} \right] {/tex} and {tex} A ^ { 2 } \left[ \begin{array} { c } { 1 } \\ { - 1 } \end{array} \right] = \left[ \begin{array} { l } { 1 } \\ { 0 } \end{array} \right] {/tex} The sum of the elements of {tex} A {/tex} is
Question 153 :
If A, B, C are angles of a triangle and <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e86f884e6d3604eaa92dea8' height='63' width='252' > = 0 then triangle ABC is
Question 155 :
If k<img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e86f8bee6d3604eaa92df09' height='63' width='83' >is an orthogonal matrix then k is equal to-
Question 157 :
If {tex} A {/tex} and {tex} B {/tex} are two square matrices of the same order and {tex} m {/tex} is a positive integer, then {tex} ( A + B ) ^ { m } = ^ { m } C _ { 0 } A ^ { m } + ^ { m } C _ { 1 } A ^ { m - 1 } B + ^ { m } C _ { 2 } A ^ { m - 2 } B ^ { 2 } + \cdots + ^ { m } C ^ { m - 1 } A B ^ { m - 1 } {/tex} {tex} + ^ { m } C _ { m } B ^ { m } {/tex} if<br>
Question 158 :
If p , q , r are in A.P., then the value of determinant is <img style='object-fit:contain' src="https://data-screenshots.sgp1.digitaloceanspaces.com/5de0fe963f8352053aeb035f.jpg" />
Question 160 :
If {tex} x , y , z {/tex} are different from zero and {tex} \Delta = \left| \begin{array} { c c c } { a } & { b - y } & { c - z } \\ { a - x } & { b } & { c - z } \\ { a - x } & { b - y } & { c } \end{array} \right| {/tex} {tex} = 0 , {/tex} then the value of the expression {tex} \frac { a } { x } + \frac { b } { y } + \frac { c } { z } {/tex} is
Question 161 :
If [] denotes the greatest integer less than or equal to the real number under consideration, and {tex} - 1 \leq x < 0,0 \leq y < 1 {/tex} ,{tex} 1 \leq z < 2 , {/tex} then the value of the determinant<br>{tex} \left| \begin{array} { c c c } { [ x ] + 1 } & { [ y ] } & { [ z ] } \\ { [ x ] } & { [ y ] + 1 } & { [ z ] } \\ { [ x ] } & { [ y ] } & { [ z ] + 1 } \end{array} \right| {/tex} is<br>
Question 162 :
For each real {tex} x , - 1 < x < 1 {/tex}. Let {tex} A ( x ) {/tex} be the matrix (1-x) {tex} ^ { - 1 } \left[ \begin{array} { c c } { 1 } & { - x } \\ { - x } & { 1 } \end{array} \right] {/tex} and {tex} z = \frac { x + y } { 1 + x y } {/tex}. Then
Question 163 :
Let {tex} A + 2 B = \left[ \begin{array} { c c c } { 1 } & { 2 } & { 0 } \\ { 6 } & { - 3 } & { 3 } \\ { - 5 } & { 3 } & { 1 } \end{array} \right] {/tex} and {tex} 2 A - B = \left[ \begin{array} { c c c } { 2 } & { - 1 } & { 5 } \\ { 2 } & { - 1 } & { 6 } \\ { 0 } & { 1 } & { 2 } \end{array} \right] {/tex}. Then {tex} \operatorname { tr } ( A ) - \operatorname { tr } ( B ) {/tex} has the value equal to
Question 164 :
There are three points {tex} ( a , x ) , ( b , y ) {/tex} and {tex} ( c , z ) {/tex} such that the straight lines joining any two of them are not equally<br>inclined to the coordinate axes where {tex} a , b , c , x , y , z \in R . {/tex} If<br>{tex} \left| \begin{array} { c c c } { x + a } & { y + b } & { z + c } \\ { y + b } & { z + c } & { x + a } \\ { z + c } & { x + a } & { y + b } \end{array} \right| = 0 {/tex} and {tex} a + c = - b , {/tex} then {tex} x , - \frac { y } { 2 } , z {/tex} are in
Question 165 :
The factors of $\left| \begin{matrix} x & a & b \\ a & x & b \\ a & b & x \\ \end{matrix} \right|$ are
Question 166 :
<p>Consider the following statements:</p> <p>1. A square matrix A is hermitian, if A = A<sup>′</sup></p> <p>2. Let A = [a<sub>ij</sub>] be a skew- hermitian matrix, then a<sub>ij</sub> is purely imaginary</p> <p>3. All integer powers of a symmetric matrix are symmetric. Which of these is/are correct?</p>
Question 167 :
<p>Let $\omega = - \frac{1}{2} + i\frac{\sqrt{3}}{2},$ then the value of the determinant</p> <p>$\left| \begin{matrix} 1 & 1 & 1 \\ 1 & - 1 - \omega^{2} & \omega^{2} \\ 1 & \omega^{2} & \omega^{4} \\ \end{matrix} \right|,$ is</p>
Question 168 :
<p>If A, B, C are the angles of a triangle, then the value of</p> <p>$\Delta = \left| \begin{matrix} - 1 & \cos C & \cos B \\ \cos C & - 1 & \cos A \\ \cos B & \cos A & - 1 \\ \end{matrix} \right|$ is</p>
Question 169 :
The value of $\mathrm{\Delta}\ = \left| \begin{matrix} a & a + b & a + 2b \\ a + 2b & a & a + b \\ a + b & a + 2b & a \\ \end{matrix} \right|$ is equal to
Question 170 :
If A and B are two matrices such that A + B and AB are both defined, then
Question 171 :
If $A = \begin{bmatrix} 3 & 2 \\ 0 & 1 \\ \end{bmatrix},\ $then(A<sup> − 1</sup>)<sup>3</sup> is equal to
Question 172 :
A is a square matrix of order 4 and I is a unit matrix, then it is true that
Question 173 :
<br/>$\mathrm{\Delta}\ = \left| \begin{matrix} 1/a & 1 & \text{bc} \\ 1/b & 1 & \text{ca} \\ 1/c & 1 & \text{ab} \\ \end{matrix} \right| =$<br/>
Question 174 :
$\left| \begin{matrix} b^{2}c^{2} & \text{bc} & b + c \\ c^{2}a^{2}\ & \text{ca} & c + a \\ a^{2}b^{2} & \text{ab} & a + b \\ \end{matrix} \right|$ is equal to
Question 175 :
<p>The roots of the equation</p> <p>$\left| \begin{matrix} 3x^{2} & x^{2} + x\cos{\theta + \cos^{2}{\theta\ }} & x^{2} + x\sin{\theta + \sin^{2}\theta} \\ x^{2} + x\cos{\theta + \cos^{2}\theta} & 3\cos^{2}\theta & 1 + \frac{\sin{2\theta}}{2} \\ x^{2} + x\sin{\theta + \sin^{2}\theta} & 1 + \frac{\sin{2\theta}}{2} & 3\sin^{2}\theta \\ \end{matrix} \right| = 0$</p>
Question 176 :
If $\mathrm{\Delta} = \left| \begin{matrix} 1 & 2 & 3 \\ 2 & 5 & 7 \\ 3 & 9 & 13 \\ \end{matrix} \right|$ and $\mathrm{\Delta}^{'} = \left| \begin{matrix} 7 & 20 & 29 \\ 2 & 5 & 7 \\ 3 & 9 & 13 \\ \end{matrix} \right|$ , then
Question 177 :
If $\begin{bmatrix} x + y & 2x + \mathcal{z} \\ x - y & 2\mathcal{z} + w \\ \end{bmatrix} = \begin{bmatrix} 4 & 7 \\ 0 & 10 \\ \end{bmatrix}$, then the value of x, y, 𝓏, w are
Question 178 :
If $P = \begin{bmatrix} \frac{\sqrt{3}}{2}\text{\ \ \ \ \ \ }\frac{1}{2} \\ \frac{- 1}{2\ }\ \frac{\sqrt{3}}{2} \\ \end{bmatrix},\ A = \begin{bmatrix} 1\ \ 1 \\ 0\ \ 1 \\ \end{bmatrix}\ \text{and}\ Q = \text{PA}P^{T},$ then P<sup>T</sup> Q<sup>2005</sup> P is
Question 179 :
The roots of the equation $\left| \begin{matrix} 1 & 4 & 20 \\ 1 & - 2 & 5 \\ 1 & 2x & 5x^{2} \\ \end{matrix} \right|$=0
Question 180 :
The value of $\left| \begin{matrix} 441\ \ 442\ \ 443 \\ 445\ \ 446\ \ 447 \\ 449\ \ 450\ \ 451 \\ \end{matrix} \right|$ is
Question 181 :
<p>l, m, n are the pth, qth and rth terms of an GP and all</p> <p>Positive, then$\left| \begin{matrix} \log l & p & 1 \\ \log m & q & 1 \\ \log n & r & 1 \\ \end{matrix} \right|$ equals</p>
Question 182 :
If the matrices A=$\begin{bmatrix} 2 & 1 & 3 \\ 4 & 1 & 0 \\ \end{bmatrix}$ and B=$\begin{bmatrix} 1 & - 1 \\ 0 & 2 \\ 5 & 0 \\ \end{bmatrix},\text{then\ }\text{AB}$
Question 183 :
If the matrix M<sub>r</sub> is given by $M_{r} = \begin{bmatrix} r & r - 1 \\ r - 1 & r \\ \end{bmatrix}$ r = 1, 2, 3..., then the value of det (M<sub>1</sub>) + det (M<sub>2</sub>) + ... + det(M<sub>2008</sub>) is
Question 184 :
If a square matrix A is orthogonal as well as symmetric, then
Question 185 :
Two spheres of radii {tex}3{/tex} and {tex}4{/tex} cut orthogonally. The radius of common circle is
Question 186 :
If {tex}\hat{a}, \hat{b}, \hat{c}{/tex} are three non-coplanar unit vectors, then {tex}[\hat{a} \vec{p} \vec{q}]\hat{a}{/tex} + {tex}[\hat{b} \vec{p} \vec{q}]\hat{b}{/tex} + {tex}[\hat{c} \vec{p} \vec{q}]\hat{c}{/tex} is equal to
Question 187 :
Under what condition does the equation {tex} x ^ { 2 } + y ^ { 2 } + z ^ { 2 } + 2 u x + 2 u y + 2 w z + d = 0 {/tex} represent a real sphere?
Question 188 :
The volume of the parallelopiped whose edges are represented by <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74b200f511820358e68475' height='19' width='92' > and is 546. Then <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74b20146f7eb01d9e6dd6c' height='13' width='23' >
Question 189 :
If position vectors of a point A is a + 2b and a divides AB in the ratio <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74b0c146f7eb01d9e6dbdf' height='15' width='27' >, then the position vector of B is
Question 190 :
The magnitudes of mutually perpendicular forces a, b and c are 2, 10 and 11 respectively. Then the magnitude of its resultant is
Question 193 :
Direction ratios of two lines are {tex} a , b , c {/tex} and {tex} \frac { 1 } { b c } , \frac { 1 } { c a } , \frac { 1 } { a b } , {/tex} The lines are
Question 194 :
Given that {tex} A ( 3,2 , - 4 ) , B ( 5,4 , - 6 ) {/tex} and {tex} C ( 9,8 , - 10 ) {/tex} are collinear. The ratio in which {tex} B {/tex} divides {tex} A C {/tex} is
Question 195 :
Distance between two parallel planes {tex} 2 x + y + 2 z = 8 {/tex} and {tex} 4 x + 2 y + 4 z + 5 = 0 {/tex} is
Question 196 :
The system of vectors <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74afca46f7eb01d9e6daef' height='19' width='35' > is
Question 198 :
Under what condition do {tex} \left\langle \frac { 1 } { \sqrt { 2 } } , \frac { 1 } { 2 } , \mathrm { k } \right\rangle {/tex} repressent direction cosines of a line?
Question 199 :
The locus of a point, such that the sum of the squares of its distances from the planes {tex} x + y + z = 0 , x - z = 0 {/tex} and {tex} x - 2 y + {/tex} {tex} z = 0 {/tex} is {tex} 9 , {/tex} is
Question 200 :
A variable plane remains at constant distance p from the origin. If it meets coordinate axes at points {tex} \mathrm { A } , \mathrm { B } , \mathrm { C } {/tex} then the locus of the centroid of {tex} \Delta \mathrm { ABC } {/tex} is
Question 201 :
The length of the perpendicular from the origin to the plane <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74b7a5f511820358e68888' height='17' width='103' >is
Question 202 :
The locus of a point, such that the sum of the squares of its distances from the planes {tex} x + y + z = 0 , x - z = 0 {/tex} and {tex} x - 2 y + z = 0 {/tex} is {tex} 9 , {/tex} is
Question 203 :
Distance between the points (1, 3, 2) and (2, 1, 3) is
Question 204 :
Projection of {tex} \hat { i } + 2 \hat { j } + 3 \hat { k } {/tex} on {tex} \hat { i } - 2 \hat { j } - 2 \hat { k } {/tex} is equal to
Question 205 :
If the plane {tex} 2 a x - 3 a y + 4 a z + 6 = 0 {/tex} passes through the midpoint of the line joining the centres of the spheres {tex} x ^ { 2 } + y ^ { 2 } + z ^ { 2 } + 6 x - 8 y - 2 z = 13 {/tex} and {tex} x ^ { 2 } + y ^ { 2 } + z ^ { 2 } - 10 x + 4 y - 2 z = 8 {/tex} then {tex} a {/tex} equals
Question 206 :
If a and b be unlike vectors, then a . b =
Question 207 :
If the radius of the sphere {tex} x ^ { 2 } + y ^ { 2 } + z ^ { 2 } - 6 x - 8 y + 10 z + \lambda = 0 {/tex} is unity, what is the value of {tex} \lambda ? {/tex}
Question 208 :
If equation of a sphere is {tex} 2 \left( x ^ { 2 } + y ^ { 2 } + z ^ { 2 } \right) - 4 x - 8 y + 12 z - 7 = 0 {/tex} and one extremity of its diameter is {tex} ( 2 , - 1,1 ) , {/tex} then the other extremity of diameter of the sphere will be
Question 210 :
The equation of a plane passing through the line of intersection of the planes {tex} x + y + z = 5 {/tex} and {tex} 2 x - y + 3 z = 1 {/tex} and parallel to the line {tex} y = z = 0 {/tex} is
Question 211 :
If vector equation of the line {tex} \frac { x - 2 } { 2 } = \frac { 2 y - 5 } { - 3 } = z + 1 , {/tex} is {tex} \vec { r } = \left( 2 \hat { i } + \frac { 5 } { 2 } \hat { j } - \hat { k } \right) + \lambda \left( 2 \hat { i } - \frac { 3 } { 2 } \hat { j } + p \hat { k } \right) {/tex} then {tex} p {/tex} is equal to
Question 212 :
A variable plane passes through a fixed point {tex} ( a , b , c ) {/tex} and meets the coordinate axes in {tex} A , B {/tex} and {tex} C {/tex} . The locus of the point common to the plane through {tex} A , B {/tex} and {tex} C {/tex} parallel to the coordinate planes is
Question 213 :
The plane {tex} a x + b y = 0 {/tex} is rotated through an angle {tex} \alpha {/tex} about its line of intersection with the plane {tex} z = 0 . {/tex} Then the equation to the plane in new position.
Question 214 :
Statement-1: The point A(1,0,7) is the mirror image of the point B(1,6,3) in the line: <br><img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74b3a746f7eb01d9e6df7a' height='50' width='141' ><br>Statement-2: The line <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74b3a7f511820358e68691' height='46' width='140' > bisects the line segment joining A(1,0,7) and B(1,6,3).
Question 215 :
The vector equation of the plane which is at a distance of {tex} \frac { 6 } { \sqrt { 29 } } {/tex} from the origin and its normal vector from the origin is {tex} 2 \hat { \mathrm { i } } - 3 \hat { \mathrm { j } } + 4 \hat { \mathrm { k } } , {/tex} is
Question 216 :
Let {tex} \vec { a } {/tex} and {tex} \vec { b } {/tex} be unit vectors such that {tex} | \vec { a } + \overline { b } | = \sqrt { 3 } . {/tex} Then the value of {tex} ( 2 \vec { a } + 5 \vec { b } ) \cdot ( 3 \vec { a } + \vec { b } + \vec { a } \times \vec { b } ) {/tex} is equal to
Question 217 :
If the vectors {tex} \vec { a } = \hat { i } - \hat { j } + 2 \hat { k } , \vec { b } = 2 \hat { i } + 4 \hat { j } + \hat { k } {/tex} and {tex} \vec { c } = \lambda \hat { i } + \hat { j } + \mu \hat { k } {/tex} are mutually orthogonal, then {tex} ( \lambda , \mu ) = {/tex}
Question 218 :
If the plane {tex} x - 3 y + 5 z = d {/tex} passes through the point {tex} ( 1,2,4 ) , {/tex} then the length of intercepts cut by it on the axes of {tex} X , Y , Z {/tex} are respectively, is
Question 219 :
Statement-1: The point A(3,1,6) is the mirror image of the point B(1,3,4) in the plane <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74b39b46f7eb01d9e6df6d' height='21' width='102' >.<br>Statement-2: The plane <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74b39b46f7eb01d9e6df6d' height='21' width='102' > bisects the line segment joining A(3,1,6) and B(1,3,4). [2010]
Question 220 :
The line passing through the points {tex} ( 5,1 , a ) {/tex} and {tex} ( 3 , b , 1 ) {/tex} crosses the {tex} y z {/tex}-plane at the point {tex} \left( 0 , \frac { 17 } { 2 } , \frac { - 13 } { 2 } \right) {/tex}. Then
Question 221 :
A line in the three-dimensional space makes an angle {tex} \theta \left( 0 < \theta \leq \frac { \pi } { 2 } \right) {/tex} with both the {tex} x {/tex} -and {tex} y {/tex} -axis. Then the set of all values of {tex} \theta {/tex} is the interval
Question 222 :
A vector {tex} \vec { n } {/tex} is inclinedtox-axis at {tex} 45 ^ { \circ } , {/tex} to {tex} y {/tex} -axis at {tex} 60 ^ { \circ } {/tex} and at an acute angle to {tex} z {/tex} -axis. If {tex} \vec { n } {/tex} is a normal to a plane passing through the point {tex} ( \sqrt { 2 } , - 1,1 ) {/tex} then the equation of the plane is
Question 223 :
What is the angle between the line {tex} 6 \mathrm { x } = 4 \mathrm { y } = 3 \mathrm { z } {/tex} and the plane {tex} 3 \mathrm { x } + 2 \mathrm { y } - 3 \mathrm { z } = 4 ? {/tex}
Question 224 :
Let the line {tex} \frac { x - 2 } { 3 } = \frac { y - 1 } { - 5 } = \frac { z + 2 } { 2 } {/tex} lie the plane {tex} x + 3 y - \alpha z + \beta = 0 . {/tex} Then {tex} ( \alpha , \beta ) {/tex} equals
Question 225 :
A line with direction cosines proportional to {tex} 2,1,2 {/tex} meets each of the lines {tex} x = y + a = z {/tex} and {tex} x + a = 2 y = 2 z {/tex}. The co-ordinates of each of the points of intersection are given by
Question 226 :
If {tex} r , a , b , c {/tex} are the three non-zero vectors such that {tex} \vec { r } \cdot \vec { a } = \vec { r } \cdot \vec { b } = \vec { r } \cdot \vec { c } = 0 , {/tex} then {tex} [ \vec { a } \vec { b } \vec { c } ] {/tex}
Question 227 :
If {tex} ( 2,3,5 ) {/tex} is one end of a diameter of the sphere {tex} x ^ { 2 } + y ^ { 2 } + z ^ { 2 } - 6 x - 12 y - 2 z + 20 = 0 {/tex} , then the coordinates of the other end of the diameter are
Question 228 :
The equation of the straight line through the origin parallel to the line {tex} ( b + c ) x + ( c + a ) y + ( a + b ) z = k = ( b - c ) x + ( c - a ) y {/tex} {tex} + ( a - b ) z {/tex} is
Question 229 :
Distance between the parallel planes {tex} 2 \mathrm { x } - \mathrm { y } + 3 \mathrm { z } + 4 = 0 {/tex} and {tex} 6 \mathrm { x } - 3 \mathrm { y } + 9 \mathrm { z } - 3 = 0 {/tex} is
Question 230 :
The value of {tex} | \vec { a } \times \hat { i } | ^ { 2 } + | \vec { a } \times \hat { j } | ^ { 2 } + | \vec { a } \times \hat { k } | ^ { 2 } {/tex} is
Question 231 :
If {tex} b {/tex} and {tex} c {/tex} are any two perpendicular unit vectors and {tex} a {/tex} is any vector, then \[ ( a \cdot b ) b + ( a \cdot c ) c + \frac { a \cdot ( b \times c ) } { | b \times c | } ( b \times c ) = \]
Question 232 :
The points {tex} A ( 1,2,3 ) , B ( - 1 , - 2 , - 3 ) {/tex} and {tex} C ( 2,3,2 ) {/tex} are three vertices of a parallelogram ABCD. The equation of {tex} C D {/tex} is
Question 233 :
The vector {tex} \vec { a } ( x ) = \cos x \hat { i } + \sin x \hat { j } {/tex} and {tex} \vec { b } ( x ) = x \hat { i } + \sin x \hat { j } {/tex} are collinear for
Question 234 :
Let {tex} \vec{a} = \hat{i}+\hat{j}+ \hat{k}{/tex}, {tex} \vec{b} = x_{1} \hat{i}+x_{2} \hat{j}+x_{3} \hat{k}{/tex}, where {tex} x_{1}, x_{2}, x_{3} \varepsilon [ {-3,-2,-1,0,2}]{/tex}. Then number of possible vectors {tex}\vec{b}{/tex} such that {tex}\vec{a}{/tex} and {tex}\vec{b}{/tex} are mutually perpendicular is
Question 235 :
The direction cosines l, m, n of two lines are connected by the relations l + m + n = 0, lm = 0, then the angle between them is
Question 236 :
Given that $\left| \overrightarrow{\mathbf{a}} \right| = 3,|\overrightarrow{\mathbf{b}}| = 4,|\overrightarrow{\mathbf{a}} \times \overrightarrow{\mathbf{b}}| = 10,\ $then $|\overrightarrow{\mathbf{a}} \bullet \overrightarrow{\mathbf{b}}|^{2}$ equals
Question 237 :
A unit vector coplanar with $\widehat{\mathbf{i}}\mathbf{+}\widehat{\mathbf{j}}\mathbf{+}2\widehat{\mathbf{k}}$ and $\widehat{\mathbf{i}}\mathbf{+}2\widehat{\mathbf{j}}\mathbf{+}\widehat{\mathbf{k}}$ and perpendicular to $\widehat{\mathbf{i}}\mathbf{+}\widehat{\mathbf{j}}\mathbf{+}\widehat{\mathbf{k}}$ is
Question 238 :
If from a point P(a, b, c) perpendiculars PA, PB are drown to yz and zx plane, then the equation of the plane OAB is
Question 239 :
If $\overrightarrow{\mathbf{a}}\mathbf{\bullet}\overrightarrow{\mathbf{b}}\mathbf{\bullet}\overrightarrow{\mathbf{c}}$ are unit vectors, then $\left| \ \overrightarrow{\mathbf{a}}\mathbf{-}\overrightarrow{\mathbf{b}}\ \left. \ \right|^{2} + \right|\overrightarrow{\mathbf{b}}\mathbf{-}\overrightarrow{\mathbf{c}}\ \left| \ ^{2} + |\overrightarrow{\mathbf{c}}\mathbf{-}\overrightarrow{\mathbf{a}} \right|^{2}$ does not exceed
Question 240 :
The work done in moving an object along a vector d⃗ = 3î + 2ĵ − 5k̂ if the applied force is F⃗ = 2î − ĵ − k̂ is
Question 241 :
The moment about the point M( − 2, 4, − 6) of the force represented in magnitude and position $\overrightarrow{\mathbf{\text{AB}}}$ where the points A and B have the coordinates (1, 2, − 3) and (3, − 4, 2) respectively, is
Question 242 :
A force of magnitude 5 units acting along the vector 2î − 2ĵ + k̂ displaces the point of application from the point (1, 2, 3) to the point (5, 3, 7), then the work done by the force is
Question 243 :
<p>The angle between the line</p> <p>$\frac{x}{1} = \frac{y}{0} = \frac{z}{- 1}\ \text{and\ }\frac{x}{3} = \frac{y}{4} = \frac{z}{5}\ \text{is\ equal\ to}$</p>
Question 244 :
If the constant forces 2î − 5ĵ + 6k̂ and − î + 2ĵ − k̂ act on a particle due to which it is displaced from a point A(4, −3, −2) to a point B(6, 1, −3), then the work done by the forces is
Question 245 :
If ABCDEF is a regular hexagon with $\overrightarrow{\mathbf{\text{AB}}} = \overrightarrow{\mathbf{a}}\ $and $\overrightarrow{\mathbf{\text{BC}}} = \overrightarrow{\mathbf{b}},\ $then $\overrightarrow{\mathbf{\text{CE}}}$ equals
Question 246 :
If $\overrightarrow{\mathbf{a}}\mathbf{,}\overrightarrow{\mathbf{b}}\mathbf{,}\overrightarrow{\mathbf{c}}$ are vectors such that $\overrightarrow{\mathbf{c}}\mathbf{=}\overrightarrow{\mathbf{a}}\mathbf{+}\overrightarrow{\mathbf{b}}$ and $\overrightarrow{\mathbf{a}}\mathbf{\bullet}\overrightarrow{\mathbf{b}} = 0$, then
Question 247 :
D, E and F are the mid-points of the sides BC, CA and AB respectively of ΔABC and G is the centroid of the triangle, then $\overrightarrow{\text{GD}} + \overrightarrow{\text{GE}} + \overrightarrow{\text{GF}} =$
Question 248 :
If $\left| \overrightarrow{\mathbf{A}} \right| = 3,\ \left| \overrightarrow{\mathbf{b}} \right| = 4,$ then a value of λ for which $\overrightarrow{\mathbf{a}} + \lambda\overrightarrow{\mathbf{b}}$ is perpendicular to $\overrightarrow{\mathbf{a}} - \lambda\overrightarrow{\mathbf{b}}$ is
Question 249 :
A force of magnitude 5 unit acting along the vector $2\widehat{\mathbf{i}} - 2\widehat{\mathbf{j}} + \widehat{\mathbf{k}}$ displaces the point of applications from (1,2,3) to (5,3,7) then the work done is
Question 250 :
The value of c so that for all real x, the vectors cx î − 6ĵ + 3k̂, xî + 2ĵ + 2cx k̂ make an obtuse angle are
Question 251 :
If a⃗, b⃗, c⃗ are linearly independent vectors and $\mathrm{\Delta} = \left| \begin{matrix} \overrightarrow{a} & \overrightarrow{b} & \overrightarrow{c} \\ \overrightarrow{a}.\overrightarrow{a} & \overrightarrow{a}.\overrightarrow{b} & \overrightarrow{a}.\overrightarrow{c} \\ \overrightarrow{a}.\overrightarrow{c} & \overrightarrow{b}.\overrightarrow{c} & \overrightarrow{c}.\overrightarrow{c} \\ \end{matrix} \right|$, then
Question 252 :
The moment about the point M(−2, 4, −6) of the force represented in magnitude and position by AB where the points A and B have the coordinates (1, 2, −3) and (3, −4, 2) respectively is
Question 253 :
If (a⃗×b⃗) + (a⃗.b⃗)<sup>2</sup> = 144 and |a⃗| = 4, then |b⃗|=
Question 254 :
The equation of the plane perpendicular to the line $\frac{x - 1}{1} = \frac{y - 2}{- 1} = \frac{z + 1}{2}$ and passing through the point (2, 3, 1), is
Question 255 :
The angles between two planes x + 2y + 2𝓏 = 3 and − 5x + 3y + 4𝓏 = 9 is
Question 256 :
The direction ratio of the line x − y + z − 5 = 0 = x − 3y − 6 are
Question 257 :
If the vectors $\widehat{\dot{\mathbf{i}}} - 3\widehat{\dot{\mathbf{j}}} + 2\widehat{\mathbf{k}},\ - \widehat{\dot{\mathbf{i}}} + 2\widehat{\dot{\mathbf{j}}}$ represent the diagonals of a parallelogram, them its area will be
Question 258 :
If for a plane, the intercepts on the coordinate axes are 8, 4, 4, then the length of the perpendicular from the origin to the plane is
Question 259 :
Let ABCD be the parallelogram whose sides AB and AD are represented by the vectors $2\widehat{\dot{\mathbf{i}}} + 4\widehat{\dot{\mathbf{j}}} - 5\widehat{\mathbf{k}}$ and $\widehat{\dot{\mathbf{i}}} + 2\widehat{\dot{\mathbf{j}}} + 3\widehat{\mathbf{k}}$ respectively. Then if $\overrightarrow{\mathbf{a}}\ $is a unit vector parallel to $\overrightarrow{\mathbf{\text{AC}}}$, then $\overrightarrow{\mathbf{a}}$ is equal to
Question 260 :
The set of all real values of x satisfying the inequality <br>|x<sup>2</sup> + x - 6| < 6 is :<br>
Question 261 :
The roots of the equation {tex} t ^ { 3 } + 3 a t ^ { 2 } + 3 b t + c = 0 {/tex} are {tex} z _ { 1 } , z _ { 2 } , z _ { 3 } {/tex} which represent the vertices of an equilateral triangle, then
Question 262 :
Let {tex} \left| z _ { r } - r \right| \leq r , \forall r = 1,2,3 , \ldots , n . {/tex} Then {tex} \left| \sum _ { r = 1 } ^ { n } z _ { r } \right| {/tex} is less than
Question 263 :
(x-a) (x-b) + c = 0 (c ≠ 0), then the roots of the equation <br>(x - c - α) (x - c - β) = c are -<br>
Question 264 :
If roots of the equation x<sup>3</sup> - 12x<sup>2</sup> + kx -28 = 0 are in A.P. then k is:<br>
Question 265 :
If {tex} \left| z ^ { 2 } - 1 \right| = | z | ^ { 2 } + 1 , {/tex} then {tex} z {/tex} lies on
Question 266 :
Let {tex} C _ { 1 } {/tex} and {tex} C _ { 2 } {/tex} are concentric circles of radius {tex}1{/tex} and {tex} 8 / 3 , {/tex} respectively, having centre at {tex} ( 3,0 ) {/tex} on the Argand plane. If the complex number {tex} z {/tex} satisfies the inequality<br>{tex} \log _ { 1 / 3 } \left( \frac { | z - 3 | ^ { 2 } + 2 } { 11 | z - 3 | - 2 } \right) > 1 {/tex} then<br>
Question 267 :
If a ∈ (-1, 1), then roots of the quadratic equation (a - 1) x<sup>2</sup> + ax + <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74642d4b0dee6204206930' height='25' width='45' > = 0 are -<br>
Question 268 :
If α, β are the roots of equation x<sup>2</sup> + px + q = 0 and γ, δ are roots of equation x<sup>2</sup> + rx + s = 0 then the value of (α - γ)<sup>2</sup> + (β - γ)<sup>2</sup> +(α - δ)<sup>2 </sup>+ (β - δ)<sup>2 </sup>is -<br>
Question 269 :
Locus of {tex} z {/tex} if {tex} \arg [ z - ( 1 + i ) ] {/tex}<br>{tex} = \left\{ \begin{array} { l l } { \frac { 3 \pi } { 4 } } & { \text { when } | z | \leq | z - 2 | } \\ { \frac { - \pi } { 4 } } & { \text { when } | z | > | z - 4 | } \end{array} \right. {/tex} is<br>
Question 270 :
The number of real solutions of the equation {tex} x ^ { 2 } - 3 | x | + 2 = 0 {/tex} is
Question 271 :
If {tex} \omega ( \neq 1 ) {/tex} is a cube root of unity, and {tex} ( 1 + \omega ) ^ { 7 } = A + B \omega . {/tex} Then {tex} ( A , B ) {/tex} equals
Question 272 :
If {tex} z = i \log ( 2 - \sqrt { - 3 } ) , {/tex} then {tex} \cos z = {/tex}
Question 273 :
If {tex} 8 i z ^ { 3 } + 12 z ^ { 2 } - 18 z + 27 i = 0 , {/tex} then
Question 274 :
If x be real, then the minimum value of <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e7463134b0dee62042066f4' height='19' width='68' > is<br>
Question 275 :
If one root of the equations ax<sup>2</sup> + bx + c = 0 and <br>bx<sup>2</sup> + cx + a = 0 (a, b, c ∈ R) is common, then the value of <br><img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e7463db4b0dee620420687d' height='49' width='88' >is:<br>
Question 276 :
If {tex} | z - 1 | \leq 2 {/tex} and {tex} \left| \omega z - 1 - \omega ^ { 2 } \right| = a {/tex} (where {tex} \omega {/tex} is a cube root of unity) then complete set of values of {tex} a {/tex} is
Question 277 :
If {tex} x ^ { 2 } + 2 a x + b \geq c , \forall x \in R , {/tex} then
Question 278 :
If {tex} z {/tex} and {tex} \omega {/tex} are two non-zero complex numbers such that {tex} | z \omega | = 1 , {/tex} and {tex} \mathrm { Arg } ( z ) - \mathrm { Arg } ( \omega ) = \pi / 2 , {/tex} then {tex} \overline { \mathrm { z } } \omega {/tex} is equal to
Question 279 :
Let {tex} z = 1 - t + i \sqrt { t ^ { 2 } + t + 2 } , {/tex} where {tex} t {/tex} is a real parameter. The locus of {tex} z {/tex} in the Argand plane is
Question 280 :
For all x ∈ R, if mx<sup>2</sup> - 9mx + 5m + 1 > 0, then m lies in the interval -<br>
Question 281 :
The value of a for which the equation {tex} \left( a ^ { 2 } + 4 a + 3 \right) x ^ { 2 } {/tex} {tex} + \left( a ^ { 2 } - a - 2 \right) x + ( a + 1 ) a = 0 {/tex} has more than two roots is
Question 282 :
If the product of the roots of the equation <br>αx<sup>2</sup> + bx + α<sup>2</sup> + 1 = 0 is -2, then α equals -<br>
Question 283 :
Let {tex} p ( x ) = 0 {/tex} be a polynomial equation of least possible degree, with rational coefficients having {tex} \sqrt [ 3 ] { 7 } + \sqrt [ 3 ] { 49 } {/tex} as one of its roots. Then the product of all the roots of {tex} p ( x ) = 0 {/tex} is
Question 284 :
The value of 'a' so that the sum of the squares of the roots of the equation x<sup>2</sup> - (a - 2) x - a + 1 = 0. assume the least value, is <br>
Question 285 :
Let {tex} \lambda \in R , {/tex} the origin and the non-real roots of {tex} 2 z ^ { 2 } + 2 z + \lambda = 0 {/tex} form the three vertices of an equilateral triangle in the Argand plane then {tex} \lambda {/tex} is
Question 286 :
If {tex} \alpha , \beta {/tex} be the roots of the equation {tex} u ^ { 2 } - 2 u + 2 = 0 {/tex} and if {tex} \cot \theta {/tex} {tex} = x + 1 , {/tex} then {tex} \left[ ( x + \alpha ) ^ { n } - ( x + \beta ) ^ { n } \right] / [ \alpha - \beta ] {/tex} is equal to
Question 287 :
{tex} 1 , z _ { 1 } , z _ { 2 } , z _ { 3 } , \ldots , z _ { n - 1 } {/tex} are the {tex} n ^ { \text {th } } {/tex} roots of unity, then the value of {tex} 1 / \left( 3 - z _ { 1 } \right) + 1 / \left( 3 - z _ { 2 } \right) + \cdots + 1 / \left( 3 - z _ { n - 1 } \right) {/tex} is equal to
Question 288 :
Which of the following represents a point in an Argand plane, equidistant from the roots of the equation {tex} ( z + 1 ) ^ { 4 } = 16 z ^ { 4 } ? {/tex}
Question 289 :
If {tex} \cos \alpha + 2 \cos \beta + 3 \cos \gamma = \sin \alpha + 2 \sin \beta + 3 \sin \gamma = 0 , {/tex} then the value of {tex} \sin 3 \alpha + 8 \sin 3 \beta + 27 \sin 3 \gamma {/tex} is
Question 290 :
If {tex} ( \cos \theta + i \sin \theta ) ( \cos 2 \theta + i \sin 2 \theta ) \cdots ( \cos n \theta + i \sin n \theta ) = 1 {/tex} then the value of {tex} \theta {/tex} is, {tex} m \in N {/tex}
Question 291 :
Which of the following is equal to {tex} \sqrt [ 3 ] { - 1 } ? {/tex}
Question 292 :
If {tex} \alpha {/tex} is the {tex} n ^ { \text {th } } {/tex} root of unity, then {tex} 1 + 2 \alpha + 3 \alpha ^ { 2 } + \cdots {/tex} to {tex} n {/tex} terms equal to
Question 293 :
If {tex} |2 z - 1| = | z - 2 | {/tex} and {tex} z _ { 1 } , z _ { 2 } , z _ { 3 } {/tex} are complex numbers such that {tex} \left| z _ { 1 } - \alpha \right| < \alpha , \left| z _ { 2 } - \beta \right| < \beta , {/tex} then {tex} \left| \frac { z _ { 1 } + z _ { 2 } } { \alpha + \beta } \right| {/tex}
Question 294 :
Let {tex} z , w {/tex} be complex numbers such that {tex} \bar { z } + i \bar { w } = 0 {/tex} and arg {tex} z w = {/tex} {tex} \pi . {/tex} Then arg {tex} z {/tex} equals
Question 295 :
The greatest positive argument of complex number satisfying {tex} | z - 4 | = \operatorname { Re } ( z ) {/tex} is
Question 296 :
{tex} z _ { 1 } {/tex} and {tex} z _ { 2 } {/tex} are two distinct points in an Argand plane. If {tex} a \left| z _ { 1 } \right| = b \left| z _ { 2 } \right| {/tex} (where {tex} a , b \in R ) , {/tex} then the point {tex} \left( a z _ { 1 } / b z _ { 2 } \right) + \left( b z _ { 2 } / a z _ { 1 } \right) {/tex} is a point on the
Question 297 :
Roots of the equations are {tex} ( z + 1 ) ^ { 5 } = ( z - 1 ) ^ { 5 } {/tex} are
Question 298 :
Consider the equation {tex} 10 z ^ { 2 } - 3 i z - k = 0 , {/tex} where {tex} z {/tex} is a complex variable and {tex} i ^ { 2 } = - 1 . {/tex} Which of the following statements is true?
Question 299 :
If {tex} z ( 1 + a ) = b + i c {/tex} and {tex} a ^ { 2 } + b ^ { 2 } + c ^ { 2 } = 1 , {/tex} then {tex} [ ( 1 + i z ) / ( 1 - i z )] = {/tex}
Question 300 :
The polynomial {tex} x ^ { 6 } + 4 x ^ { 5 } + 3 x ^ { 4 } + 2 x ^ { 3 } + x + 1 {/tex} is divisible by where {tex}\mathrm w {/tex} is cube root of units
Question 301 :
Suppose {tex} A {/tex} is a complex number and {tex} n \in N , {/tex} such that {tex} A ^ { n } = ( A {/tex} {tex} + 1 ) ^ { n } = 1 , {/tex} then the least value of {tex} n {/tex} is
Question 302 :
{tex} P ( z ) {/tex} be a variable point in the Argand plane such that {tex} | z | = {/tex} minimum {tex} \{ | z - 1 | , | z + 1 | \} {/tex} then {tex} z + \bar { z } {/tex} will be equal to
Question 303 :
Let {tex} a {/tex} be a complex number such that {tex} | a | < 1 {/tex} and {tex} z _ { 1 } , z _ { 2 } , z _ { 3 } , \ldots {/tex} be the vertices of a polygon such that {tex} z _ { k } = 1 + a + a ^ { 2 } + \cdots + a ^ { k - 1 } {/tex} for all {tex} k = 1,2,3 , \ldots {/tex} then {tex} z _ { 1 } , z _ { 2 } , \ldots {/tex} lie within the circle
Question 304 :
If {tex} A \left( z _ { 1 } \right) , B \left( z _ { 2 } \right) , C \left( z _ { 3 } \right) {/tex} are the vertices of the triangle {tex} A B C {/tex} such that {tex} \left( z _ { 1 } - z _ { 2 } \right) / \left( z _ { 3 } - z _ { 2 } \right) = ( 1 / \sqrt { 2 } ) + ( i / \sqrt { 2 } ) , {/tex} the triangle {tex} A B C {/tex} is
Question 305 :
If ω is a complex cube root of unity, then $\frac{\left( 1 + i \right)^{2n} - \left( 1 - i \right)^{2n}}{(1 + \omega^{4} - \omega^{2})(1 - \omega^{4} + \omega^{2})}$ is equal to
Question 306 :
The general value of θ which satisfies the equation (cosθ+isinθ)(cos3θ+isin3 θ)(cos5 θ+isin5 θ)… (cos (2n−1)θ + isin (2n − 1)θ = 1) is
Question 307 :
The complex number z = x + iy, which satisfy the equation $\left| \frac{z - 5i}{z + 5i} \right| = 1$ lies on
Question 308 :
If the equation x<sup>3</sup> − 3x + a = 0 has distinct roots between 0 and 1, then the value of a is
Question 309 :
If ax<sup>2</sup> + bx + c = 0 and 2x<sup>2</sup> + 3x + 4 = 0 have a common root where a, b, c ∈ N (set of natural numbers), the least value of a + b + c is
Question 310 :
The co0mplex number z = x + iy which satisfy the equation $\left| \frac{z - 5i}{z + 5i} \right| = 1$ lies on
Question 311 :
For any complex number z, the minimum value of |z| + |z − 1| is
Question 312 :
If $a = \cos{\frac{4\pi}{3} + i\sin{\frac{4\pi}{3},}}$ then the value of $\left( \frac{1 + a}{2} \right)^{3n}$ is
Question 315 :
If $\left| \frac{z - 25}{z - 1} \right| = 5$, find the value of |z|
Question 316 :
If z<sub>1</sub>, z<sub>2</sub> are two complex numbers satisfying $\left| \frac{z_{1} + 3z_{2}}{3 - z_{1}\overline{z_{2}}} \right| = 1,\ |z_{1}| \neq 3$, then |z<sub>2</sub>| is equal to
Question 317 :
If one root of the equation 8x<sup>2</sup> − 6x − a − 3 = 0 is the square of the other, then the values of a are
Question 318 :
If z<sup>2</sup> + (p+iq)z + (r+is) = 0, where, p, q, r, s are non-zero has real roots, then
Question 319 :
The roots of the cubic equation (z+αβ)<sup>3</sup> = α<sup>3</sup>, α ≠ 0
Question 320 :
If z is a complex number in the Argand plane such that $\arg{\left( \frac{z - 3\sqrt{3}}{z + 3\sqrt{3}} \right) = \frac{\pi}{3}}$ then the lous of z is
Question 322 :
If z is a non-real 7<sup>th</sup> root of − 1, then z<sup>86</sup> + z<sup>175</sup> + z<sup>289</sup> is equal to
Question 323 :
If (x<sup>2</sup> − 3x + 2) is a factor of x<sup>4</sup> − px<sup>2</sup> + q = 0, then the values of p and q are
Question 324 :
If α<sub>1</sub>, α<sub>2</sub>, α<sub>3</sub> respectively denote the moduli of the complex numbers $- i,\frac{1}{3}(1 + i)$ and − 1 + i, then their increasing order is
Question 325 :
If f(x) = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e870c9175ed294f2c7c41ae' height='52' width='101' >
is continuous but not <br>differentiable at x = 0 then -
Question 329 :
If function f(x) = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e870c7d19f8d44d3a17facc' height='41' width='125' >,
then the number of points at which f(x) is continuous, is :
Question 330 :
If f(x) = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e870c8419f8d44d3a17fad6' height='41' width='100' >
then the function is non differentiable at -
Question 331 :
If f(x) = |x| + |x - 1|, g(x) = e<sup>x</sup> + e<sup>-x</sup> then f(x) ÷ g(x) is -
Question 332 :
Let ƒ and g be differentiable functions satisfying g′(a) = 2, g (a) = b and ƒog = I (identity function). Then, ƒ′ (b) is equal to -
Question 333 :
A function f(x) = sin x + cos x + e<sup>|x|</sup> is non differentiable at -
Question 334 :
If f (x) = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e870beb19f8d44d3a17f901' height='44' width='57' > then -
Question 335 :
If f(x) = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e870c88e6d3604eaa92ed37' height='36' width='60' >; x ≠ 0 is continuous at x = 0 then f(0) equals -
Question 336 :
Let f:R →R be a function defined as f(x)= Min{ 1 +x, 1 + |x|} then which of the following is correct
Question 337 :
Let f(x) = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e870d0719f8d44d3a17fc80' height='89' width='124' ><br>Then f(x) is continuous at x = 4 when
Question 338 :
If f(x) = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e870cfbe6d3604eaa92eea7' height='68' width='91' >
is continuous " x ∈ R then (A, B) is-
Question 339 :
If A and B are square Matrices of order 3 such that |A| = -1 , |B| = 3 then |3AB| = ---------
Question 341 :
<img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e870cec75ed294f2c7c42db' height='27' width='57' >, where [.] is GIF, is
Question 342 :
If x is real number in [0, 1], then the value of <br><img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e870cf275ed294f2c7c42ed' height='27' width='55' >[1 + cos<sup>2m</sup> (n!πx)] is given by
Question 343 :
A function f(x) = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e870d0375ed294f2c7c431a' height='44' width='79' >, α ≠ mπ is continuous at x = α then
Question 344 :
If f(x) = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e870d0319f8d44d3a17fc72' height='55' width='96' >
then f(x) is -
Question 345 :
{tex}\underset{ x \rightarrow 0 }\lim x ^ { x } = {/tex}
Question 346 :
Let $f(x)$ be continous and differentiable function for all real values.<br/>$f(x+y)=f(x)-3xy+f(y)$. If $\displaystyle \lim_{h\to0}\dfrac{f(h)}{h}=7$, then value of $f'(x)$ is
Question 347 :
The function $f(x) = \begin{cases} 3x - 1, & if & x < 1 \\ x^2, & if & x > 2 \end{cases}$ continuous on
Question 348 :
If the function $f(x)=\left\{\begin{matrix}\dfrac{{}x^{2}-(A+2)x+A}{x-2} & for\ x\neq 2\\ 2& for\ x = 2\end{matrix}\right.$ is<br/>continuous at $x = 2$ , then<br/>
Question 349 :
The function of $f(x)=\left[ x \right] $ where $\left[ x \right] $ the greatest integer function is continuous at
Question 350 :
If the function $f(x)$ defined as<div>$\displaystyle f\left( x \right) =\begin{cases} \begin{matrix} { \left( \sin { x } +\cos { x } \right) }^{ \csc { x } }, & -\frac { \pi }{ 2 } <x<0 \end{matrix} \\ \begin{matrix} a, & x=0 \end{matrix} \\ \begin{matrix} \frac { { e }^{ 1/x }+{ e }^{ 2/x }+{ e }^{ 3/x } }{ a{ e }^{ -2+1/x }+b{ e }^{ -1+3/x } } , & 0<x<\frac { \pi }{ 2 } \end{matrix} \end{cases}$ is continuous at $x=0$, then</div>
Question 351 :
If {tex} f ( x ) = \left\{ \begin{array} { l l } { \frac { 1 - | x | } { 1 + x } , } & { x \neq - 1 } \\ { 1 , } & { x = - 1 } \end{array} , \text { } \text { } \right. {/tex}then the value of {tex}f ( [ 2 x ] ){/tex} will be<br>(where [1 shows the greatest integer function)<br>
Question 352 :
Let f : R → R be a function defined by f(x) = max {x, x<sup>3</sup>}. The set of all points where f(x) is NOT differentiable is
Question 353 :
The function $f(x)=\displaystyle \frac{x\tan 2x}{\sin 3x.\sin 5x}$ for $x\neq 0$, $f(\mathrm{0})=2/17$ at $x=0$ is:<br>
Question 354 :
The function <br> $\displaystyle f\left ( x \right )=\frac{\cos x-\sin x}{\cos 2x}$ is not defined at $\displaystyle x=\frac{\pi }{4}$. The value of $\displaystyle f\left ( \frac{\pi }{4}\right )$ so that $ f\left ( x \right)$ is continuous everywhere, is
Question 355 :
The point of discontinuity of the function $f(x)=\dfrac{1+\cos {5x}}{1-\cos {4x}}$ is-
Question 356 :
If the function ƒ(x) = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e870c6b75ed294f2c7c4144' height='47' width='61' > sin (x - 2) + a cos (x - 2), (where [.] denotes the greatest integer function) is continuous and differentiable in (4, 6), then -
Question 357 :
If the function $f:R\rightarrow R$ defined by<br>$f(x)=\begin{cases} ax,\quad \quad x<2 \\ a{ x }^{ 2 }-bx+3,\quad x\ge 2 \end{cases}$ is differentiable, then the value of $f'(-3)+f'(3)$ is equal to:
Question 358 :
$If\,f\left( x \right) = x + \left\{ { - x} \right\} + \left[ x \right]$ <br> $where\left[ . \right]$ is the integral part & $\left\{ . \right\}$ is the fractional , then the number of points of discontinuity of f in $\left[ { - 2,2} \right]$ is/are
Question 359 :
$f\left( x \right) =\begin{cases} a\tan { ^{ -1 }\left( \dfrac { 1 }{ x-4 } \right) }\quad if\quad 0\le x<4 \\ b\tan { ^{ -1 } } \left( \dfrac { 2 }{ x-4 } \right)\quad if\quad 4<x<6 \\ \sin { ^{ -1 } } \left( 7-x \right) +a\dfrac { \pi }{ 4 }\quad if\quad \sin { ^{ -1 } } \left( 7-x \right) +a\dfrac { \pi }{ 4 } \end{cases} $ and $f(4)=\pi/2$ is continuous on $(0,8)$ then
Question 360 :
Let $f$ be a continuous function on $R$ such that $\displaystyle f\left( \frac { 1 }{ 4n } \right) =\left( \sin { { e }^{ n } } \right) { e }^{ -{ n }^{ 2 } }+\frac { { n }^{ 2 } }{ { n }^{ 2 }+1 } $. Then, the value of $f(0)$ is
Question 361 :
Let $f(x)=\begin{cases} -2\sin { x } ,\quad \quad -\pi \le x\le -\cfrac { \pi }{ 2 } \\ a\sin { x+b,\quad -\cfrac { \pi }{ 2 } <x<\cfrac { \pi }{ 2 } } \\ \cos { x } ,\quad \quad \quad \quad \cfrac { \pi }{ 2 } \le x\le \pi \end{cases}$<br>If $f(x)$ is continuous on $\left[ -\pi ,\pi \right] $, then
Question 362 :
$f\left( x \right) =\begin{cases} { x }^{ 2 } \ if\ x\ is\ irrational \\ 1\ if x\ is\ rational \end{cases}$, then
Question 363 :
Let $f(x)=\begin{cases} -2\sin { x } ,\quad \quad if\quad x\le -\cfrac { \pi }{ 2 } \\ A\sin { x } +B,\quad if\quad -\cfrac { \pi }{ 2 } <x<\cfrac { \pi }{ 2 } \\ \cos { x } ,\quad if\quad x\ge \cfrac { \pi }{ 2 } \end{cases}$. Then
Question 364 :
f(x) = $\dfrac{\sin2x + 1}{\sin x - \cos x}$ is discontinuous at $x =$ ____________.
Question 365 :
The set of points where the function $f\left( x \right) = \sqrt{1 - e^{- x^{2}}}$ is differentiable is
Question 366 :
$f\left( x \right) = \left\{ \begin{matrix} 2a - x\text{\ \ }\text{in}\ –a < x < a \\ 3x - 2a\text{\ \ }\text{in}\text{\ \ }a \leq x \\ \end{matrix} \right.\ $. Then, which of the following is true?
Question 367 :
If the function {tex} f ( x ) = \frac { 1 - \cos 4 x } { 8 x ^ { 2 } } , {/tex} where {tex} x \neq 0 {/tex} and {tex} f ( x ) = k {/tex} where {tex} x = 0 {/tex} is a continuous function at {tex} x = 0 , {/tex} then the value of {tex} k {/tex} will be<br>
Question 368 :
The function<br>$f(x)=max\left\{ \left( 1-x \right) ,\left( 1+x \right) ,2 \right\} ,x\in \left( -\infty ,\infty \right) $ is
Question 369 :
If the function {tex} f ( x ) = \left\{ \begin{array} { c } { 1 + \sin \frac { \pi x } { 2 } , \text { for } - \infty < x \leq 1 } \\
{ a x + b , \text { for } 1 < x < 3 \quad \text { } } \\ { 6 \tan \frac { x \pi } { 12 } , \text { for } 3 \leq x < 6 } \end{array} \right. {/tex}<br>is continuous in the interval {tex} ( - \infty , 6 ) , {/tex} then the values of {tex} a {/tex} and {tex} b {/tex} are respectively<br>
Question 370 :
At $x = \dfrac {3}{2}$, the function $f(x) = \dfrac {|2x - 3|}{2x - 3}$ is
Question 371 :
Let $f:\:R\rightarrow R$ be a function such that $\displaystyle f\left ( \frac{x+y}{2} \right )=\frac{f(x)+f(y)}{2}$ for all x, y, and $f(0)=3$ and ${f}'(0)=3$. Then
Question 373 :
f(x) = |[x]+x| in − 1 < x ≤ 2 is
Question 375 :
<p>Let $f\left( x \right) = \left\{ \begin{matrix} \frac{1}{|x|}\ \text{for}\ |x| \geq 1 \\ ax^{2} + b\ \text{for}\ \left| x \right| < 1 \\ \end{matrix} \right.\ $</p> <p>If f(x) is continuous and differentiable at any point, then</p>
Question 377 :
If $f\left( x \right) = \left\{ \begin{matrix} \frac{|x + 2|}{\tan^{- 1}{(x + 2)}},\ x \neq - 2 \\ 2,\ \ \ \ \ \ x = - 2 \\ \end{matrix} \right.\ $, then f(x) is
Question 378 :
The set of all those points, where the function {tex} f ( x ) = \frac { x } { 1 + | x | } {/tex} is differentiable, is<br>
Question 379 :
$\lim_{h \rightarrow 0}\frac{\sin{(a + 3h)} - 3\sin\left( a + 2h \right) + 3\sin\left( a + h \right) - \sin a}{h^{3}}$ is equal to
Question 381 :
The value of f(0) so that the function $f\left( x \right) = \frac{2 - \left( 256 - 7\ x \right)^{1/8}}{\left( 5x + 32 \right)^{1/5} - 2}(x \neq 0)$ is continuous everywhere, is given by
Question 382 :
$f(x)$ is a continuous function for all real values of $x$ and satisfies $\int_{0}^{x} f(t) d t=\int_{x}^{1} t^{2} f(t) d t+\dfrac{x^{16}}{8}+\dfrac{x^{6}}{3}+a,$ then the value of $a$ is equal to
Question 383 :
The function $f\left( x \right) = \left\{ \begin{matrix} 1,\ \ \ \ \ |x| \geq 1 \\ \frac{1}{n^{2}},\frac{1}{n} < \left| x \right| < \frac{1}{n - 1},\ n = 2,\ 3,\ldots \\ 0,\ \ \ \ x = 0 \\ \end{matrix} \right.\ $
Question 384 :
<p>If the function</p> <p>$f\left( x \right) = \left\{ \begin{matrix} \left\{ 1 + \left| \sin x \right| \right\}^{\frac{a}{|\sin x|}},\ \ - \frac{\pi}{6} < x < 0 \\ b,\ \ x = 0 \\ e^{\frac{\tan{2x}}{\tan{3x}}},\ \ 0 < x < \frac{\pi}{6} \\ \end{matrix} \right.\ $</p> <p>Is continuous at x = 0</p>
Question 385 :
The differential equation of all non-horizontal lines in a plane is _____
Question 386 :
What is the general solution of the differential equation $x\, dy - y\, dx \,y^2$ ?
Question 388 :
The differential equation obtained by eliminating $A$ and $B$ from $y = A \cos wt + B \sin wt $ is
Question 391 :
If $\displaystyle x=a \left ( k\sin t+\sin kt \right ), y= a\left ( k\cos t+\cos kt \right ),$ then find $\displaystyle \frac{d^{2}y}{dx^{2}}$ in terms of t.
Question 395 :
$\displaystyle \frac{d^{2}y}{dx^{2}}+\cos x\frac{dy}{dx}+4y\cos c^{2}x=0$ it being given that $\displaystyle z=\log \tan \frac{x}{2}.$
Question 397 :
If $\dfrac{dy}{dx}=e^{x+y}$ and at $x=1;\> y=1$, then for $x=-1;\> y$ ___.<br/>
Question 398 :
The differential equation satisfied by all the circle in the x - y plane is $(1 + y_1 ^2) y_3 = \lambda \ y_1 y_2 ^2$ where $\lambda$ =
Question 404 :
<div>Differential equation $\dfrac{dy}{dx}=f(x)g(x)$ can be solved by</div><div>separating variable $\dfrac{dy}{g(y)}=f(x)dx$</div><br/>If $\dfrac{dy}{dx}=1+x+y+xy$ and $y(-1)=0$, then $y$ is equal to
Question 407 :
The D.E whose solution is $y=$a cos x + b sin x + x sin x is:<br/>
Question 408 :
Assume that a spherical rain drop evaporates at a rate proportional to its surface area. If k is a positive constant then differential equation involving the rate of change of the radius of the rain drop is<b></b><b></b>
Question 410 :
The solution of differential equation<br>$4xy\cfrac { dy }{ dx } =\cfrac { 3{ \left( 1+x \right) }^{ 2 }\left( 1+{ y }^{ 2 } \right) }{ \left( 1+{ x }^{ 2 } \right) } $ is
Question 413 :
Eliminate the arbitrary constants and obtain the differential equation satisfied by it: $y = \displaystyle \left(\frac{a}{x^{2}} \right) + bx$
Question 414 :
Find the solution of the D.E whose solution is given as $y=2\left ( x^{2}-1 \right )+ce^{-x^{2}}$ <br/>
Question 415 :
The differential equation of all circles whose centers are at the origin is:
Question 416 :
If $ x\dfrac{dy}{dx} = y(\log y - \log x + 1) $, then the solution of the equation is
Question 417 :
$y={ ae }^{ mx }+{ be }^{ -mx }$ satisfies which of the following differential equations:
Question 418 :
The differential equation of all circles passing through the origin and with centres on x- axis is:<br/>
Question 423 :
The slope of a curve at any point is the reciprocal of twice the ordinate at the point and it passes through the point (4, 3). The equation of the curve is
Question 424 :
The order and degree of the differential equation $\sqrt{\frac{\text{dy}}{\text{dx}}} - 4\frac{\text{dy}}{\text{dx}} - 7x = 0$ are
Question 425 :
The solution of $2\left( y + 3 \right) - \text{xy}\ \frac{\text{dy}}{\text{dx}} = 0$ with y = − 2, where x = 1, is
Question 426 :
The solution of $\frac{\text{dy}}{\text{dx}} + y = e^{- x},\ y\left( 0 \right) = 0$ is
Question 427 :
The solution of the differential equation $\frac{\text{dy}}{\text{dx}}\tan y = \sin{(x + y)} + \sin{(x - y)}$ is
Question 428 :
The solution of the differential equation $x\ \text{dy} - y\ \text{dx} - \sqrt{x^{2} - y^{2}}\ \text{dx} = 0$ is
Question 429 :
The solution of the differential equation $\left\{ \frac{1}{x} - \frac{y^{2}}{\left( x - y \right)^{2}} \right\}\text{dx} + \left\{ \frac{x^{2}}{\left( x - y \right)^{2}} - \frac{1}{y} \right\}\text{dy} = 0$ is
Question 432 :
The degree of the differential equation y<sub>3</sub><sup>2/3</sup> + 2 + 3y<sub>2</sub> + y<sub>1</sub> = 0, is
Question 433 :
Solution of the differential equation xdy − ydx = 0 represents a
Question 434 :
An integrating factor of the differential equation $\left( 1 - x^{2} \right)\frac{\text{dy}}{\text{dx}} - \text{xy} = 1$ is
Question 435 :
The equation of the curve through the point (1,0) and whose slope is $\frac{y - 1}{x^{2} + x}$, is
Question 436 :
The solution of the differential equation $9y\ \frac{\text{dy}}{\text{dx}} + 4x = 0$ is
Question 437 :
The differential equation obtained on eliminating A and B from the equation y = Acos ωt + Bsin ωt is
Question 438 :
The differential equation of the family y = ae<sup>x</sup> + bx e<sup>x</sup> + cx<sup>2</sup> e<sup>x</sup> of curves, where a, b, c are arbitrary constants, is
Question 439 :
The differential equation of all circles of radius a is of order
Question 440 :
If $\frac{\text{dy}}{\text{dx}} + y = 2e^{2x}$, then y is equal to
Question 441 :
The solution of dy = cos x(2−y cosec x)dx, where $y = \sqrt{2}$, when $x = \frac{\pi}{4}$ is
Question 442 :
The area bounded by y = x<sup>2 </sup>+ 2 and y = 2|x| - cosπx is equal to -
Question 443 :
The area of the region bounded by the curves {tex} y = x ^ { 2 } {/tex} and {tex} y = | x | {/tex} is
Question 444 :
{tex} \int e ^ { \ln ( \sin x ) } d x {/tex} is equal to
Question 445 :
The area of the region bounded by y=|x-1| and y = 1 is
Question 446 :
If ƒ : R→R, g : R→R are continuous functions then the value of the integral <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e870cb819f8d44d3a17fb82' height='49' width='195' >is -
Question 447 :
Let f : R →R and g : R →R be continuous functions. Then the value of <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e870b3275ed294f2c7c3d52' height='51' width='37' >{f (x) + f (-x)} {g (x) - g (-x)} dx is
Question 448 :
The value of the integral, {tex} \int \limits_ { 3 } ^ { 6 } \frac { \sqrt { x } } { \sqrt { 9 - x } + \sqrt { x } } d x {/tex} is
Question 450 :
{tex} \sum _ { k = 1 } ^ { n }{tex} \int_{0}^{1} f(k-1+x)dx \ is{/tex}
Question 451 :
{tex} \int \frac { x ^ { 2 } + \sin ^ { 2 } x } { 1 + x ^ { 2 } } \sec ^ { 2 } x d x {/tex} is equal to
Question 452 :
The area between the curve {tex} y ^ { 2 } = 4 a x , x {/tex}-axis and the ordinates {tex} x = 0 {/tex} and {tex} x = a {/tex} is
Question 453 :
The area bounded by y = ln x, the x-axis and the ordinates x = 0 and x = 1, is
Question 454 :
<img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e870b3ae6d3604eaa92e909' height='49' width='21' >[2 e<sup>-x</sup>]dx, where [.] denotes the greatest integer function, is equal to
Question 456 :
{tex} \int \frac { \cos \sqrt { x } } { \sqrt { x } } d x {/tex} is equal to
Question 457 :
<img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74c4b4d374484379aa8e4b' height='39' width='43' > {1 + (x + 2) log (x + 2)} dx =
Question 458 :
If ƒ(x) = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e870c8d19f8d44d3a17faf0' height='53' width='52' >dt, find the interval in which ƒ(x) is increasing -
Question 459 :
The area bounded by the curve y = | x | - 1 and y = - | x | + 1 is -
Question 460 :
Area bounded by {tex} y = x \sin x {/tex} and {tex} x {/tex} -axis between {tex} x = 0 {/tex} and {tex} x = 2 \pi {/tex} is
Question 461 :
The area of the curve {tex} x + | y | = 1 {/tex} and the {tex} y {/tex} -axis is
Question 462 :
The value of {tex} \int \frac { d x } { x \left( x ^ { n } + 1 \right) } {/tex} is equal to
Question 463 :
{tex} \int \frac { d x } { x \sqrt { x ^ { 4 } - 1 } } {/tex} is equal to
Question 464 :
The area of the region consisting of points {tex} ( x , y ) {/tex} satisfying {tex} | x \pm y | \leq 2 {/tex} and {tex} x ^ { 2 } + y ^ { 2 } \geq 2 {/tex} is
Question 465 :
{tex} \int \frac { \cos 2 x } { \cos x } d x {/tex} is equal to
Question 466 :
{tex} \int \{ \sin ( \log x ) + \cos ( \log x ) \} d x = {/tex}
Question 468 :
{tex} \int \frac { \tan x } { \sqrt { \cos x } } d x {/tex} is equal to
Question 469 :
If f is an odd function, then I = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e870b3919f8d44d3a17f6c4' height='52' width='113' >dx
Question 471 :
The area enclosed between the curve {tex} y = \log _ { e } ( x + e ) {/tex} and the coordinate axes is
Question 472 :
The least value of the function \[ F ( x ) = \int\limits _ { 5 \pi/ 4 } ^ { x } ( 3 \sin u + 4 \cos u ) d u \]<br>on the interval {tex} \left[ \frac { 5 \pi } { 4 } , \frac { 4 \pi } { 3 } \right] {/tex} is<br>
Question 473 :
{tex} \int e ^ { \log _ { e } x } d x = {/tex}
Question 474 :
{tex} \lim\limits _ { n \rightarrow \infty } \left\{ \frac { 1 } { 1 - n ^ { 2 } } + \frac { 2 } { 1 - n ^ { 2 } } + \frac { 3 } { 1 - n ^ { 2 } } + \cdots + \frac { n } { 1 - n ^ { 2 } } \right\} = {/tex}
Question 475 :
If {tex} f ( a + b - x ) = f ( x ) , {/tex} then {tex} \int \limits_{a}^{b}x f ( x ) d x {/tex} is equal to
Question 476 :
{tex} \int \frac { d x } { ( 2 x + 1 ) ( 1 + \sqrt { ( 2 x + 1 ) } ) } {/tex} is equal to
Question 477 :
If area bounded by the curves {tex} y ^ { 2 } = 4 a x {/tex} and {tex} y = m x {/tex} is {tex} \frac { a ^ { 2 } } { 3 } , {/tex} then the value of {tex} m {/tex} is
Question 478 :
Let {tex} \frac { d } { d x } F ( x ) = \left( \frac { e ^ { \sin x } } { x } \right) ; x > 0 . {/tex} If {tex} \int _ { 1 } ^ { 4 } \frac { 3 } { x } e ^ { \sin x ^ { 2 } } d x = F ( k ) - F ( 1 ) , {/tex} then one of the possible value of {tex} k {/tex} is
Question 479 :
If f : R → R and g : R → R are continuous, then <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e870d2f75ed294f2c7c43a3' height='36' width='203' >=
Question 480 :
If {tex} \int x \log _ { e } ( 1 + 1 / x ) d x = P ( x ) \ln \left( 1 + \frac { 1 } { x } \right) + \frac { 1 } { 2 } x - \frac { 1 } { 2 } \ln ( 1 + x ) + c {/tex} then
Question 481 :
The area bounded by the curves {tex} y ^ { 2 } - x = 0 {/tex} and {tex} y - x ^ { 2 } = 0 {/tex} is
Question 482 :
The value of is {tex} \sqrt { 2 } \int \frac { \sin x d x } { \sin \left( x - \frac { \pi } { 4 } \right) } {/tex}
Question 483 :
The value of {tex} \int\limits _ { 0 } ^ { 100 } a ^ { x - [ x ] } d x {/tex} is
Question 484 :
{tex} \int _ { 0 } ^ { \infty } \frac { x d x } { ( 1 + x ) \left( 1 + x ^ { 2 } \right) } = {/tex}
Question 485 :
{tex} \int _ { - \pi / 2 } ^ { \pi / 2 } \sin ^ { 4 } x \cos ^ { 6 } x d x = {/tex}
Question 486 :
The area bounded by the curve {tex} y = x ( 3 - x ) ^ { 2 } , {/tex} the {tex} x {/tex} -axis and the ordinates of the maximum and minimum points of the curve is
Question 487 :
The area bounded by the curves {tex} y = | x | - 1 {/tex} and {tex} y = - | x | + 1 {/tex} is
Question 488 :
If {tex} \int f ( x ) d x = \psi ( x ) , {/tex} then {tex} \int x ^ { 5 } f \left( x ^ { 3 } \right) d x {/tex} is equal to
Question 489 :
{tex} \int \tan ^ { 3 } 2\mathrm x \sec 2 \mathrm x \mathrm{d x} = {/tex}
Question 490 :
If {tex} \int \limits_ { 0 } ^ { \pi } x f ( \sin x ) d x = A \int \limits_ { 0 } ^ { \pi / 2 } f ( \sin x ) d x , {/tex} then {tex} A {/tex} is
Question 491 :
The area bounded by the circle {tex} x ^ { 2 } + y ^ { 2 } = 4 , {/tex} line {tex} x = \sqrt { 3 } y {/tex} and {tex} x {/tex} -axis lying in the first quadrant is
Question 492 :
The maximum area of a rectangle whose two vertices lie on the x-axis and two on the curve y = 3 - |x|, - 3 ≤ x ≤ 3 is
Question 493 :
If the integral {tex} \int \frac { 5 \tan x } { \tan x - 2 } d x = x + a\operatorname { ln } | \sin x - 2 \cos x | + k , {/tex} then {tex} a {/tex} is equal to
Question 494 :
The value of {tex} \int e ^ { x } \frac { \left( x ^ { 3 } + x + 1 \right) } { \left( 1 + x ^ { 2 } \right) ^ { 3 / 2 } } d x {/tex} is equal to
Question 495 :
The value of {tex} \int \frac { d x } { \left( e ^ { x } + 1 \right) \left( 2 e ^ { x } + 3 \right) } {/tex} is equal to
Question 496 :
{tex} \int \frac { d x } { \cos ( x - a ) \cos ( x - b ) } = {/tex}
Question 498 :
If I<sub>m</sub> = ∫<sub>1</sub><sup>x</sup>(logx)<sup>m</sup>dx satisfies the relation I<sub>m</sub> = k − II<sub>m − 1</sub>, then
Question 499 :
If $\int_{0}^{\pi/3}\frac{\cos x}{3 + 4\sin x}\text{dx} = k\log\left( \frac{3 + 2\sqrt{3}}{3} \right)$, then k is
Question 501 :
If P = ∫<sub>0</sub><sup>3π</sup>f(cos<sup>2</sup>x) dx and Q = ∫<sub>0</sub><sup>π</sup>f(cos<sup>2</sup>x) dx, then
Question 502 :
$\lim_{x \rightarrow \infty}\frac{\pi}{n}\left\{ \sin\frac{\pi}{n} + \sin\frac{2\pi}{n} + \ldots + \sin\frac{(n - 1)\pi}{n} \right\}$ equals
Question 503 :
<font>The area bounded by y = ln x, the x-axis and the ordinates x = 0 and x = 1, is</font></p>
Question 504 :
If $\int_{}^{}{\frac{e^{x} - 1}{e^{x} + 1}\ \text{dx} = f\left( x \right) + c}$, then f(x) is equal to
Question 505 :
If h(a) = h(b), the value of the integral ∫<sub>a</sub><sup>b</sup>[f(g (h(x)))]<sup> − 1</sup>f<sup>′</sup>(g(h(x)))g<sup>′</sup>(h(x))h<sup>′</sup>(x)dx is equal to
Question 508 :
The area of the figure bounded by the curves y<sup>2</sup> = 2x + 1 and x − y − 1 = 0 is
Question 510 :
Let f(x) be a function satisfying f<sup>′</sup>(x) = f(x) with f(0) = 1 and g(x) be a function that satisfies f(x) + g(x) = x<sup>2</sup>. Then, the value of the integral ∫<sub>0</sub><sup>1</sup>f(x) g(x) dx, is
Question 516 :
If $\int_{}^{}{\frac{\cos{4x + 1}}{\cos{x - \tan x}}\ \text{dx} = A\cos{4x + B}}$,then
Question 517 :
A triangle has vertices A(1,-1) B(2,4) and C(6,0) The length of the median from A is
Question 518 :
The section formula (internal division only) says that if point $R(X,\,Y)$ divides the join of $P(X,\,Y)$ and $Q(x_2,\,y_2)$ internally in a given ratio $(m_1:m_2)$, then coordinates of point R are
Question 520 :
The point which divides line segment joining the points (7, -6) and (3, 4 ) in ratio 1 : 2 internally lies in the
Question 521 :
The ratio in which the joining of (-3,2) and (5,6) is divided by the y-axis is
Question 522 :
The distance between $M(-1,5)$ and $N(x,5)$ is $8$ units. The value of $x$ is:
Question 523 :
A point R (2,-5) divides the line segment joining the point A (-3,5) and B (4,-9) , then the ratio is
Question 524 :
The segment from (-1,4) to (2, -2) is extended three times its own length The terminal point is
Question 525 :
The ratio in which the line joining the points $(3, 4)$ and $(5, 6)$ is divided by $x-$axis :
Question 526 :
A(3 , 2) and B(5 , 4) are the end points of a line segment . Find the co-ordinates of the mid-point of the line segment .
Question 527 :
Find the distance between the points $P(3, 2)$ and $Q(-2, -1).$
Question 528 :
Find the distance between P and Q if P is a point on the x axis with abscissa 12 and Q is (8, 3)
Question 531 :
The coordinates of $A$ and $B$ are $(1, 2) $ and $(2, 3)$. Find the coordinates of $R $, so that $A-R-B$ and $\displaystyle \frac{AR}{RB} = \frac{4}{3}$.<br/>
Question 532 :
The point P which divides the line segment joining the points A(2,-5) and B(5,2) in the ratio 2:3 lies in the quadrant<br>
Question 533 :
If $Q$ is the midpoint of $CD$ and $d(C, Q)$ $=$ $4.5$, find the length of $CD$.
Question 535 :
If $A(3, 5), B (-5, -4), C (7, 10)$ are the vertices of a parallelogram, taken in the order, then the coordinates of the fourth vertex are
Question 537 :
Given the points $A(-2, -9)$ and $B(6, 1)$, a point P bisects $AB$. Find the coordinate of P.
Question 538 :
If $A ( - 2, 5), B (3, 1)$ and $P,Q$ are the points of intersection of $AB$ then mid-point of $PQ$ is
Question 539 :
$O(0,0), A(1, 1), B(0, 3)$ are the vertices of $\triangle OAB, P$ divides $OB$ in the ratio $1 : 2, Q$ is the mid-point of $AP, R$ divides $AB$ in the ratio $2: 1$.<div>If $\displaystyle \alpha(QR)^{2} = \beta(PR)^{2}$, then<br/></div>
Question 540 :
A point which divides the joint of $(1,2)$ and $(3,4)$ externally in the ratio $1:1$
Question 541 :
<div> Find the coordinates of the point which divides the line segment joining the points (6, 3) and (-4, 5) in the ratio 3 : 2 e xternally.</div>
Question 542 :
In what ratio does y axis divide the line segment joining points $P(5,\,7)$ and $Q(-8,\,9)$?
Question 543 :
The point on the y-axis which is equidistant from $A(-5, -2)$ and $B(3, 2)$ is
Question 544 :
The point which lies on the perpendicular bisector of the line segment joining the points A $(-2 , -5) $ and B$(2 , 5) $ is
Question 546 :
The latus rectum of a conic section is the width of the function through the focus. The positive difference between the length of the latus rectum of $3y = {x^2} + 4x - 9$ and ${x^2} + 4{y^2} - 6x + 16y = 24$ is
Question 547 :
Find the value of $x$, so that the three points, $(2, 7), (6, 1), (x, 0)$ are collinear.
Question 548 :
Mid point of $A(0,0)$ and $B(1024,2048)$ is ${A}_{1}$, midpoint of ${A}_{1}$ and $B$ is ${A}_{2}$ and so on. Coordinates of ${A}_{10}$ are
Question 549 :
In $\triangle ABC$ having vertices $A(-1,3), B(1,-1)$ and $C(5,1)$, the length of the median $AD$ is
Question 550 :
The ratio in which the point $(2, y)$ divides the line segment joining the points $(-4, 3)$ and $(6, 3)$ and hence the value of $y$ is:
Question 551 :
Find the co-ordinates of the point $P$ which divides segment $JL$ externally in the ratio $m:n$ in the following example:<div>$J(5, -3), L(0, 9), m:n = 4:3$<br/></div>
Question 552 :
If the area of a Δ ABC is given by Δ = a<sup>2</sup> − (b−c)<sup>2</sup>, then $\tan\left( \frac{A}{2} \right)$ is equal to
Question 553 :
The sides of a triangle are sin αcos α and $\sqrt{1 + \sin\alpha\cos\alpha}$ for some$0 < \alpha < \frac{\pi}{2}$. Then, the greatest angle of the triangle is
Question 554 :
A spherical balloon of radius r subtends an angle α at the eye of an observer. If the angle of elevation of the centre of the balloon be β, then height of the centre of the balloon is
Question 555 :
The transformed equation of x<sup>2</sup> + 6xy + 8y<sup>2</sup> = 10 when the axes are rotated through an angle $\frac{\pi}{4}$ is
Question 556 :
On the level ground the angle of elevation of the top of a tower is 30<sup>∘</sup>. On moving 20 m nearer the tower, the angle of elevation is found to be 60<sup>∘</sup>. The height of the tower is
Question 557 :
The area (in square unit) of the triangle formed by the lines x = 0, y = 0 and 3x + 4y = 12, is
Question 558 :
<p>The orthocentre of the triangle whose vertices are</p> <p>{at<sub>1</sub> t<sub>2</sub>, a(t<sub>1</sub>+t<sub>2</sub>)},{at<sub>2</sub> t<sub>3</sub>,a(t<sub>2</sub>+t<sub>3</sub>)}, {at<sub>3</sub> t<sub>1</sub>, a(t<sub>3</sub>+t<sub>1</sub>)} is</p>
Question 559 :
From the top of a cliff h metres above sea level an observer notices that angles of depression of an object A and its image B are complementary. If the angle of depression at A is θ. The height of A above sea level is
Question 560 :
In a ΔABC, $\frac{\left( a + b + c \right)\left( b + c - a \right)\left( c + a - b \right)(a + b - c)}{4b^{2}c^{2}}$ equals
Question 561 :
If the angles of a triangle are in the ratio 4 : 1 : 1, then the ratio of the longest side to the perimeter is
Question 562 :
ABC Is a triangle with vertices A(−1, 4), B(6, − 2) andC( − 2, 4). D, E And F are the points which divide each AB, BC and CA respectively in the ratio 3:1 internally. Then, the centroid of the triangle DEF is
Question 563 :
Each side of a square subtends an angle of 60<sup>∘</sup> at the top of a tower h metres high standing in the centre of the square. If a is the length of each side of the square, then
Question 564 :
If orthocentre and circumcentre of triangle are respectively (1, 1) and (3, 2), then the coordinates of its centroid are
Question 565 :
From an aeroplane flying, vertically above a horizontal road, the angles of depression of two consecutive stones on the same side of the aeroplane are observed to be 30<sup>∘</sup>and 60<sup>∘</sup>respectively. The height at which the aeroplane is flying in km, is
Question 566 :
If points A(x<sub>1</sub>,y<sub>1</sub>), B(x<sub>2</sub>, y<sub>2</sub>) and C(x<sub>3</sub>, y<sub>3</sub>) are such that x<sub>1</sub>, x<sub>2</sub>, x<sub>3</sub> and y<sub>1</sub>, y<sub>2</sub>, y<sub>3</sub> are in AP, then
Question 567 :
The tangent to the curve y = 2x<sup>2</sup> - x + 1 is parallel to the line y = 3x + 9 at the point whose co-ordinates are :
Question 568 :
Let f : R → R be a continuous onto function satisfying f(x) + f(-x) = 0, " x ∈ R. If f(-3) = 2 and f(5) = 4 in [-5, 5], then the equation f(x) = 0 has
Question 569 :
Let f : R → R be a function satisfying f(x + y) = f(x) + λxy + 3x<sup>2</sup>y<sup>2</sup> for all x, y ∈ R. If f(3) = 4 and f(5) = 52 then f ' (x) is equal to -
Question 571 :
The tangent to the curve y = e<sup>2x</sup> at the point (0, 1) meets the x- axis at
Question 572 :
If the subtangent at any point on y = a<sup>1-n</sup> x<sup>n</sup> is of constant length, then the value of n is
Question 573 :
Let f(x) =<img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74bfdaf511820358e68fb4' height='39' width='65' >, x ∈ (-∞, ∞) then the interval for which f(x) is increasing is -
Question 574 :
Number of critical points of f (x) = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74c065d374484379aa89d3' height='39' width='51' > is
Question 575 :
If 27a + 9b + 3c + d = 0, then the equation 4ax<sup>3</sup> + 3bx<sup>2</sup> + 2cx + d = 0 has at least one real root lying between-
Question 576 :
If f(x) is differentiable in [a, b] such that f(a) = 2, f (b) = 6, then there exists at least one c, a < c < b such that (b<sup>3</sup> - a<sup>3</sup>) f ' (c) =<sup>
Question 578 :
The equation x<sup>3</sup>- 3x + [a] = 0, where [.] denotes the greatest integer function, will have real and distinct roots if
Question 579 :
If f(x) = p | sin x| + qe<sup>|x|</sup> + r|x|<sup>3</sup> and if f(x) is differentiable at x = 0, then
Question 580 :
f(x) satisfies the conditions of Rolle's theorem in [1, 2] and f(x) is continuous in [1, 2] then <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74bf6df511820358e68f36' height='49' width='41' >dx is equal to -
Question 581 :
If f(x) = x<sup>2</sup> + ax + 1 is monotonic increasing in the interval [1, 2], then the minimum value of a is -
Question 582 :
Rolle's theorem is not applicable to function ƒ(x) = |x| in the interval [-3, 3] because -
Question 583 :
f(x) = x<sup>9</sup> + 3x<sup>7</sup> + 64 is monotonic increasing for -
Question 584 :
Let g(x) = f(x + l) + f(x - l) and f(x) = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74be6a2ca8b87fce1520f8' height='57' width='123' > k > 1, l> 1then g(x) is
Question 585 :
The function f(x) = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74bfc3f511820358e68f92' height='37' width='88' >is increasing for x ∈ R when
Question 586 :
If x + 4 |y| = 6y, then y as a function of x is -
Question 587 :
In [- 1, 2] the function f(x) = | x | + | x - 1| is -
Question 588 :
If the equation a<sub>n</sub>x<sup>n</sup> + a<sub>n-1</sub>x<sup>n-1</sup> + ..... + a<sub>1 </sub>x = 0 ; a<sub>1</sub>≠ 0, n ≥ 2, has a positive root x = α, then the equation na<sub>n</sub>x<sup>n-1 </sup>+(n-1)a<sub>n-1</sub> x<sup>n-2</sup> + ...+a<sub>1</sub> = 0 has a positive root, which is -
Question 590 :
Let f(x) = x<sup>3</sup> + bx<sup>2</sup> + cx +d ; 0 < b<sup>2</sup>< c then f(x) :
Question 591 :
The surface area of a sphere when its volume is increasing at the same rate as its radius is-
Question 592 :
The tangent to the curve y = e<sup>2x</sup> at the point (0, 1) meets x-axis at the point -
Question 593 :
Let the tangent to the graph of y = f(x) at the point x = a be parallel to x-axis and let f '(a - h) > 0 and f ' (a + h) < 0 where h is a very small + ve quantity. Then, the ordinate at x = a is -
Question 594 :
Let x ∈<img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74bfab023ac44295581bd5' height='41' width='41' >, f(x) = x tan(sin x), g(x) = xsin(tanx) and h(x) = sinx tanx. Which one is greatest ?
Question 596 :
In the interval (1, 2), function f(x) = 2|x - 1| + 3| x - 2| is
Question 597 :
If f(x) = (cos x + i sin x) (cos 3x + i sin 3x) .... (cos (2n - 1) x + i sin (2n - 1)x), then f′′(x) is equal to -
Question 598 :
Let f(x) = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74be3cf511820358e68dcf' height='47' width='151' >f(x) will be differentiable at x = 1, if
Question 599 :
The point of intersection of the tangents drawn to the curve x<sup>2</sup>y = 1 - y at the points where it is met by the curve <br>xy = 1 - y, is given by -
Question 600 :
The equation of the tangent to the curve f (x) = 1 + e<sup>-2x</sup> where it cuts the line y = 2 is
Question 601 :
f(x) = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74c033023ac44295581c8e' height='49' width='56' > (t - 1)(t - 2)<sup>3</sup>(t - 3)<sup>5</sup> dt has a local minimum at x equals :
Question 602 :
The function <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74c014023ac44295581c63' height='40' width='60' > has no maximum or minimum if (k an integer) -
Question 603 :
The largest value of 2x<sup>3</sup> - 3x<sup>2</sup> - 12x + 10 for - 2 ≤ x ≤4 occurs at x =
Question 604 :
The greatest of the numbers 1, 2<sup>1/2</sup>, 3<sup>1/3</sup>, 4<sup>1/4</sup>, 5<sup>1/5</sup>, 6<sup>1/6</sup> and 7<sup>1/7</sup> is
Question 605 :
The coordinates of the point on the parabola y<sup>2</sup> = 8x, which is at minimum distance from the circle x<sup>2</sup> + (y + 6)<sup>2</sup> = 1 are-
Question 606 :
The function ƒ(x) = 1 + x (sin x) [cos x], 0 < x ≤/2
Question 607 :
Two racers start a race at the same moment and finish in a tie. Which of the following must be true?
Question 608 :
The function y = f(x) is defined by x = 2t - |t|, y = t<sup>2</sup> + t |t|, t ∈ R in the interval x ∈ [- 1, 1] then
Question 609 :
The function y = f(x) is represented parametrically by x = t<sup>5</sup> - 5t<sup>3</sup> - 20t + 7 and y = 4t<sup>3</sup> - 3t<sup>2</sup> - 18t + 3, (-2 < t < 2). The minimum of y = f(x) occurs at
Question 610 :
If the function f(x) = |x<sup>2</sup>+ a |x| + b| has exactly three points of non-differentiability, then which of the following may hold
Question 611 :
If ƒ(x) = x(x - 2) (x - 4), 1 ≤ x ≤ 4, then a number satisfying the condition of the mean value theorem is -
Question 613 :
Given f(x) = 4 -<img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74c08cf511820358e690a7' height='43' width='63' >; g(x) = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74c08c023ac44295581d05' height='55' width='96' >; h(x) = {x}, k (x) = <img style='object-fit:contain' src='https://storage.googleapis.com/teachmint/question_assets/JEE%20Main/5e74c08dd374484379aa8a09' height='20' width='52' >, Then in [0, 1] Langranges Mean value theorem is NOT applicable to:
Question 615 :
The function f(x) = sin<sup>4 </sup>x + cos <sup>4</sup> x increasing if -
Question 616 :
The function f(x) = x<sup>5</sup> - 5x<sup>4</sup> + 5x<sup>3</sup> - 1 has-
Question 617 :
If the parabola y = f(x), having axis parallel to y-axis, touches the line y = x at (1, 1) then
Question 618 :
y = f(x) is a parabola, having it's axis parallel to y-axis. If the line y = x touches this parabola at x = 1, then
Question 619 :
Given a function f : [0, 4] ―→ R is differentiable, then for some a, b ∈ (0, 4) [f(4)]<sup>2</sup> - [f(0)]<sup>2</sup> =
Question 620 :
The maximum area of the rectangle whose sides pass through the angular points of a given the rectangle is of sides a and b is
Question 621 :
The acute angles between the curves y = |x<sup>2</sup> - 1| and <br>y = |x<sup>2</sup> - 3| at their points of intersection is -
Question 622 :
A spherical balloon is being inflated so that its volume increase uniformly at the rate of 40cm<sup>3</sup>/minute. The rate of increase in its surface area when the radius is 8 cm is
Question 625 :
If the area of the triangle included between the axes and any tangent to the curve x<sup>n</sup>y = a<sup>n</sup> is constant, then n is equal to
Question 627 :
If the rate of change of sine of an angle θ is k, then the rate of change of its tangent is
Question 628 :
If y = sec (tan<sup> − 1</sup>x),then $\frac{\text{dy}}{\text{dx}}$ is equal to
Question 629 :
If {tex} y = x \log \left( \frac { x } { a + b x } \right) , {/tex} then {tex} x ^ { 3 } \frac { d ^ { 2 } y } { d x ^ { 2 } } {/tex} is equal to
Question 630 :
If $y = sin\lbrack\cos^{- 1}{\{\sin{(\cos^{- 1}{x)\}\rbrack,\ \text{then}\frac{\text{dy}}{\text{dx}}\ \text{at\ }x = \frac{1}{2}\ }}}$is equal to
Question 631 :
The minimum value of px + qy when xy = r<sup>2</sup>, is
Question 632 :
If $\sqrt{1 - x^{2}} + \sqrt{1 - y^{2}} = a(x - y)$, then $\frac{\text{dy}}{\text{dx}}$ equals
Question 633 :
If f : R → R is an even function having derivatives of all orders, then an odd function among the following is
Question 634 :
The equation x + cos x = a has exactly one positive root. Complete set of values of 'a' is
Question 636 :
<p>The function f defined by</p> <p>f(x) = x<sup>3</sup> − 6x<sup>2</sup> − 36x + 7 is increasing, if</p>
Question 637 :
f(x) is a polynomial of degree 2, f(0) = 4, f<sup>′</sup>(0) = 3 and f<sup>″</sup>(0) = 4, then f( − 1) is equal to
Question 638 :
The length of the subtangent to the curve x<sup>2</sup> + xy + y<sup>2</sup> = 7 at (1, − 3) is
Question 639 :
If$\ x = a\left( cos\theta + \theta\ sin\theta \right)\text{\ and\ }y = a(\text{sinθ} - \theta\cos{\theta),\ \text{then}\frac{\text{dy}}{\text{dx}}\ }$is equal to
Question 640 :
The minimum value of f(x) = e<sup>(x<sup>4</sup> − x<sup>3</sup> + x<sup>2</sup>)</sup> is
Question 641 :
A missile is fired from the ground level rises x metres vertically upwards in t seconds where $x = 100t - \frac{25}{2}t^{2}.$The maximum height reached is