Test Details

Triangle And It's Properties

Nov 24, 10:29 AM

120 minutes

Nov 25, 11:00 AM

52.0 marks

MCQ

33 questions

ABDULLAH Sir

I have done B.Sc Physics. I have been teaching since 2014 in the school and coaching offline. I have learnt many ways to teach student. I explain the topics in easy way so students can clear her/his concept very well.

Class Details

English Grammar

Science

Mathematics

Class VIII Science & Math

English Grammar

Science

Mathematics

In this classroom, we will cover important complete syllabus of Maths and Science. And Doubt session will be in the end of week(Saturday). If you miss your live classes , you'll get recorded class in 24 hrs of Live class. You'll get the pdf of notes. There will be periodic test of objectives in app. We'll give you assignment in chapters of important question.

More from ABDULLAH Sir

(20)

Homework

Lines and Angles

b-sc

Socialscience

0 Likes

6 Views

ABDULLAH Sir

Nov 15, 2022

Homework

Science Test

b-sc

Socialscience

0 Likes

10 Views

ABDULLAH Sir

Sep 03, 2022

Homework

Science Test

class-3rd

Maths

0 Likes

7 Views

ABDULLAH Sir

Sep 03, 2022

Study Material

COAL & PETROLEUM

class-3rd

Maths

0 Likes

8 Views

ABDULLAH Sir

Aug 26, 2022

Homework

Science Test 25 June

class-3rd

Maths

0 Likes

10 Views

ABDULLAH Sir

Jun 25, 2022

Homework

MAths Test

b-sc

Socialscience

0 Likes

21 Views

ABDULLAH Sir

Jun 22, 2022

Homework

Maths Test

class-3rd

Maths

0 Likes

7 Views

ABDULLAH Sir

Jun 22, 2022

Homework

Test Of Science

b-sc

Sciencemaths

0 Likes

12 Views

ABDULLAH Sir

Jun 12, 2022

Homework

Maths Test

class-6th

Maths

0 Likes

11 Views

ABDULLAH Sir

May 29, 2022

Homework

Science Test

b-sc

Socialscience

0 Likes

16 Views

ABDULLAH Sir

May 29, 2022

Study Material

2. Trade To Territory.pdf

b-sc

Englishgrammar

0 Likes

65 Views

ABDULLAH Sir

May 21, 2022

Homework

Test 1 Maths

b-sc

Socialscience

0 Likes

12 Views

ABDULLAH Sir

May 14, 2022

Homework

Maths Lesson 1 Test

b-sc

Sciencemaths

0 Likes

18 Views

ABDULLAH Sir

May 14, 2022

Homework

Lesson 1 maths Test

b-sc

Englishgrammar

0 Likes

11 Views

ABDULLAH Sir

May 14, 2022

Homework

Grammar

class-6th

Maths

0 Likes

12 Views

ABDULLAH Sir

May 13, 2022

Homework

Ex - 7B And 7C

class-6th

Sciencemaths

0 Likes

36 Views

ABDULLAH Sir

Jan 10, 2022

Lecture

Lecture 2022-01-07

class-7th

Sciencemaths

0 Likes

9 Views

ABDULLAH Sir

Jan 07, 2022

Homework

Mensuration Exercise 20A

class-7th

Sciencemaths

0 Likes

72 Views

ABDULLAH Sir

Dec 13, 2021

Homework

English Grammar

class-7th

Sciencemaths

0 Likes

202 Views

ABDULLAH Sir

Dec 08, 2021

Test

Congruence of Triangles

class-7th

Sciencemaths

0 Likes

23 Views

ABDULLAH Sir

Dec 07, 2021

Recommended Content

(20)

Test

Triangle And It's Properties

a³aimachieversacademy

Maths

0 Likes

16 Views

Aim achievers academy

Nov 19, 2021

Test

Triangle And It's Properties

class-7th

Mathematics

0 Likes

10 Views

Er. Neeraj Sir

Nov 11, 2021

Homework

Triangle And It's Properties

class-7th

Maths

0 Likes

13 Views

Bhagyashree Archak

Jan 22, 2022

Test

Triangle and it's properties

class-1st

Maths

0 Likes

10 Views

Harkesh Meena

Nov 14, 2021

Homework

Triangle and it's properties

class-6th

Maths

0 Likes

9 Views

H C Jha

Jan 28, 2022

Test

Triangle And Properties

class-7th

Mathematics

0 Likes

30 Views

Shruthi

Dec 14, 2021

Test

The Triangle And It's Property

class-7th

Maths

0 Likes

43 Views

Kawade V.S

Oct 17, 2021

Homework

Triangle And Its Properties

class-7th

Maths

0 Likes

14 Views

Sukhchain Singh

Jan 31, 2022

Test

Triangle And Its Properties

class-7th

Science

0 Likes

25 Views

Aim achievers academy

Nov 16, 2021

Homework

Triangle And Its Properties

class-7th

Mathematics

0 Likes

10 Views

Mukesh Kumar Sharma

Jan 24, 2022

Test

Triangle And Its Properties

class-7th

Mathematics

0 Likes

10 Views

Gourab karmakar

Nov 20, 2021

Test

The Triangles and It's Properties

class-7th

Science

0 Likes

7 Views

Dr. Rajesh Kumar

Feb 10, 2022

Homework

Properties Of Triangle

class-7th

Maths

0 Likes

227 Views

AKASH SIR

Jan 24, 2022

Homework

Properties Of Triangle

class-7th

Maths

0 Likes

5 Views

KOUSHIK KUMAR KUILA

Jan 08, 2022

Homework

Properties Of Triangle

class-7th

Mathematics

0 Likes

11 Views

prahlad sir

Jan 21, 2022

Homework

Properties Of Triangle

class-7th

Mathematics

0 Likes

8 Views

sushma rana

Jan 27, 2022

Homework

Properties Of Triangle

class-7th

Mathematics

0 Likes

8 Views

sushma rana

Jan 24, 2022

Homework

Properties Of Triangle

class-7th

Mathematics

0 Likes

15 Views

sushma rana

Jan 29, 2022

Homework

Properties Of Triangle

class-7th

Mathematics

0 Likes

19 Views

sushma rana

Jan 19, 2022

Homework

Properties Of Triangle

class-7th

Maths

0 Likes

23 Views

akhilesh choubey

Jan 10, 2022

Question Text

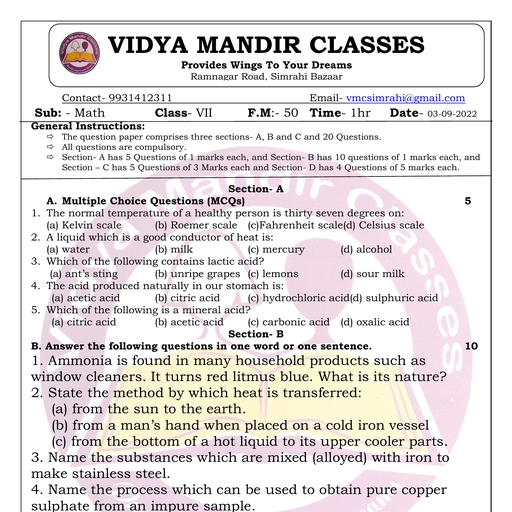
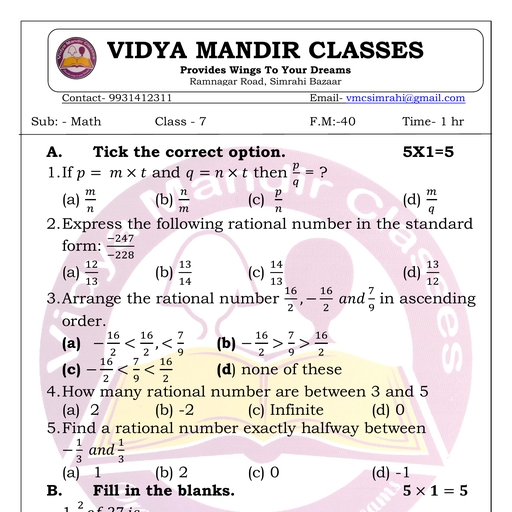
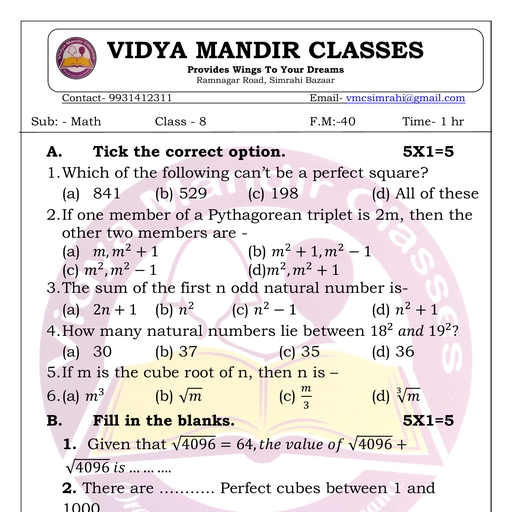
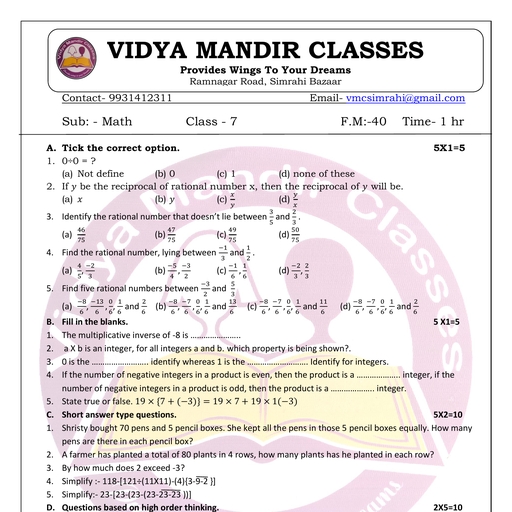
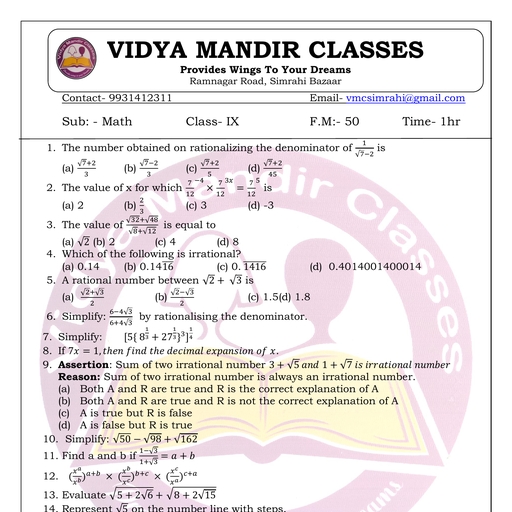
Question 1 :
The base angles of an isosceles triangle is $50^{o}$. The size of vertical angle is

Question 2 :
Two sides of a triangle are of lengths 5 cm and 1.5 cm, then the length of the third side of the triangle cannot be

Question 3 :
Which is the smallest side in the following triangle? $\displaystyle \angle P:\angle Q:\angle R=1:2:3$

Question 4 :
Which side is the hypotenuse for the sides, $89, 39$ and $80$ in a right angled triangle? (Apply Converse of Pythagoras theorem).

Question 5 :
Which of the following is true? (i) A triangle can have two obtuse angles. (ii) A triangle can have all angles equal to $60^o$. (iii) A triangle can have all angles more than $60^o$.

Question 6 :
Find the perimeter of an isosceles right triangle with each of its congruent sides is $7\,cm$.

Question 7 :
Which of the following numbers form pythagorean triplet?  i) $2, 3, 4$ ii) $6, 8, 10$ iii) $9, 10, 11$ iv) $8, 15, 17$

Question 8 :
Do the sides, $12, 37$ and $35$ form a right triangle? If so, which side is the hypotenuse?

Question 9 :
If length of the largest side of a triangle is 12 cm then other two sides of triangle can be :

Question 10 :
In any triangle, the side opposite to the larger (greater) angle is longer

Question 11 :
The incentre of a triangle coincides with the circumcentre, orthocentre and centroid in case of:

Question 12 :
What is the length of the hypotenuse formed if the two sides are of 5 cm, 12 cm?

Question 13 :
In $\Delta ABC, \angle A=100^{\circ}, \angle B=30^{\circ}$ and $\angle C= 50^{\circ}$,then

Question 14 :
If the measures of sides of a triangle are $(x^2-1) cm, (x^2 +1) cm$, and $2x cm$, then the triangle will be: 

Question 15 :
$(5, -2), (6, 4) $ and $(7, -2)$ are the vertices of an isosceles triangle.

Question 16 :
Which of the following will be the angles of a triangle?

Question 17 :
In a right angled triangle one angle is $\displaystyle 48^{\circ}$. Then the remaining angle is:

Question 18 :
In the following, state if the statement is true(T) or false(F). Each acute triangle is equilateral.

Question 19 :
A point within an equilateral triangle, where perimeter is $18$ m, is $1$ m from one side and $2$ m from another side. Its distance from the third side is:

Question 21 :
Pythagorean triple is one in which a, b and c are

Question 22 :
The base of a triangle is $80$, and one of the base angles is $60^{\circ}$. The sum of the lengths of the other two sides is $90$. The shortest side is

Question 23 :
Which of the following triplets cannot be the angles of a triangle?

Question 24 :
A and B are two fixed points in a plane. If P is a moving point in the plane such that PA = PB, then the:

Question 25 :
Which of the following sets of side lengths will not form a triangle?

Question 26 :
Which of the following statements are true (T) and which are false(F) : If the altitude from one vertex of a triangle bisects the opposite side, then the  triangle will be isosceles.

Question 27 :
In an equilateral triangle, the relative position of the centroid and the circumcenter of the triangle

Question 29 :
$(1, 0), (0, 1), (1, 1)$ are the co-ordinates of vertices of a triangle. The triangle is ............. triangle.

Question 30 :
Which statement is true about the difference of any two sides of a triangle?

Question 31 :
In an equilateral triangle if 3 times the squareof one side is equal to K times the square ofits altitude then K equals

Question 32 :
If the points $( 0,0 ) , ( 3 , \sqrt { 3 } ) , ( p , q )$ form an equilateral triangle and $q _ { 1 } , q _ { 2 }$ are the twovalues of $q$ then $q _ { 1 } + q _ { 2 } =?$

Question 33 :
In an isosceles triangle $A B C , A B = A C = 25 \mathrm { cm }$ and $B C = 14$ cm The measure of an altitude from $A$ on BC in $cm$ is

More from ABDULLAH Sir

Recommended Content

Learn from Anywhere on Any Device