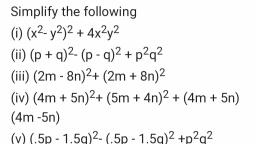Question 2 :
The pair of rational numbers lying between $\displaystyle \frac{1}{4}$ and $\displaystyle -\frac{3}{4}$ is ?

Question 3 :
$8$ can be written as a rational number with any integer as numerator.

Question 4 :
Given that $Q$ is a rational number:(i) Difference of two $Q$s is $Q$.(ii) Subtraction is commutative on $Q$.(iii) Addition is not commutative on $Q$.Which option is wrong?

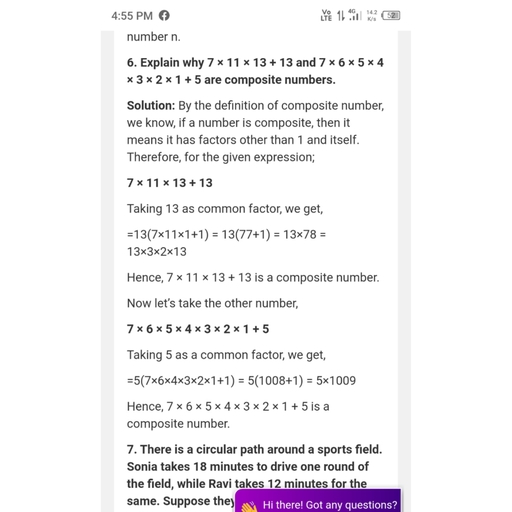
Question 6 :
$G$ is a set of all rational numbers except $-1$ and $\ast $ is defined by $a\ast b=a+b+ab$ for all $a,b\in G$, in the group $\left( G,\ast \right) $, the solution of ${ 2 }^{ -1 }\ast x\ast { 3 }^{ -1 }=5$ is

Question 10 :
Compare the size of a pen tip which is $0.000005$ m to that of the width of pen which is $ 0.0000249$ m.

Question 11 :
If $5^{k^2}(25^{2k})(625) = 25\sqrt{5}$ and $k < -1$, find the value of $k$.

Question 13 :
If the number $\overline {481x673}$ is completely divisible by  $9$. Then the smallest whole number is place of x will be

Question 15 :
Consider the number $4^n$, where $n$ is a natural number. Check whether there is any value of $n \in N$ for which $4^n$ ends with the digit zero.<br/>

Question 16 :
A number is divisible by both $5$ and $12$. By which other numbers Will that number be always divisible

Question 17 :
&#160; &#160; &#160; $\bigcirc \ \ \Diamond$ <br> $\times$ &#160;&#160;$\Box\ \ \ &#160;\Diamond$__________&#160; &#160; $ &#160; \triangle\ &#160;\Box\ \Diamond$&#160; &#160; &#160;<br/>In the multiplication above, each symbol represents a different unknown digit, and $\bigcirc&#160; \times&#160;\Box \times&#160;\Diamond = 36$.&#160;What is the three-digit integer&#160;$\bigcirc &#160;\Box &#160;\Diamond$?

Question 22 :
Find the value of cube root of the number $1290$. (Round off your number to the nearest whole number)<br/>

Question 25 :
Find the value of $\sqrt{15625}$ and the use it to find the value of $\sqrt{156.25}+\sqrt{1.5625}$.

Question 26 :
The value of $\sqrt { \sqrt [ a ]{ { 4 }^{ { a }^{ { a }^{ 2 } } }\sqrt { { 6 }^{ { a }^{ 3 } }\sqrt [ { a }^{ 3 } ]{ { 12 }^{ { a }^{ 6 } }\sqrt [ { a }^{ 4 } ]{ { 18 }^{ { a }^{ 10 } } } } } } }$ is equal to

Question 28 :
State true or falseSquare numbers can only have even number of zeros at the end.

Question 31 :
If&#160;$\displaystyle a + \dfrac{1}{a} = 4$ and&#160;$\displaystyle a \neq 0$, find :<br/>$\displaystyle a^{4} + \dfrac{1}{a^{4}}$