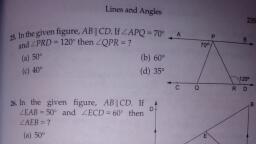Question 1 :
Angles of a triangle are in the ratio 2 : 4 : 3. The smallest angle of the triangle is ___________.
Question 2 :
How many triangles can be drawn having its angles as $45^{\circ}$ $64^{\circ}$ and $72^{\circ}$?
Question 4 :
A triangle ABC is right angled at A. L is a point on BC such that AL $⊥$ BC. Are the two angles $∠$ BAL and $∠$ ACB equal?
Question 5 :
A transversal intersects two parallel lines. The bisectors of any pair of corresponding angles so formed are ____________.
Question 7 :
Bisectors of interior $∠$B and exterior $∠$ACD of a $∆$ABC intersect at the point T. Find the relation between $ \angle BTC$ and $\angle BAC $.
Question 8 :
If one of the angles of a triangle is $130^{\circ}$, then the angle between the bisectors of the other two angles can be __________.
Question 10 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d148f59b460d7261f410.png' />
In the above figure, AB, CD and EF are three lines concurrent at O. Find the value of 'y'.
Question 11 :
If a ray stands on a line, then the adjacent angles so formed are __________ and its converse,
Question 12 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d14bf59b460d7261f414.png' />
In the picture given above, POQ is a line. The value of x is ________.
Question 13 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d14ff59b460d7261f41b.png' />
In the above figure, AP and BQ are the bisectors of the two alternate interior angles formed by the intersection of a transversal 't' with parallel lines 'l' and 'm'. Thus, AP and BQ are __________.
Question 14 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d151f59b460d7261f41d.png' />
In the above figure, BA || ED and BC || EF. Are the angles $∠ABC$ and $∠$DEF equal?
Question 15 :
Lines parallel to the same line are _________ to each other,
Question 16 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d14cf59b460d7261f416.png' />
In the above figure, for what value of x + y will ABC be a line?
Question 17 :
If one angle of a triangle is equal to the sum of the other two angles, then the triangle is _________.
Question 18 :
Let OA, OB, OC and OD are rays in the anticlockwise direction such that $∠$AOB = $∠$COD = $100^{\circ}$, $∠$BOC = $82^{\circ}$ and $∠$AOD = $78^{\circ}$. Is it true to say that AOC and BOD are lines?
Question 19 :
Two lines 'l' and 'm' are perpendicular to the same line 'n'. Are 'l' and 'm' perpendicular to each other?
Question 20 :
How many triangles can be drawn having its angles as $53^{\circ}$ $64^{\circ}$ and $63^{\circ}$?
Question 21 :
If a transversal intersects two parallel lines, then alternate interior angles are _____________.
Question 22 :
The angles of a triangle are in the ratio 2 : 3 : 4. Find the angles of the triangle.
Question 23 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d14df59b460d7261f417.png' />
In the above figure, find the value of 'x' for which the lines 'l' and 'm' are parallel.
Question 24 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d14bf59b460d7261f415.png' />
In the above given figure, if OP||RS, $∠$OPQ = $110^{\circ}$ and $∠$QRS = $130^{\circ}$, then $∠$PQR is equal to _________.
Question 25 :
A transversal intersects two lines in such a way that the two interior angles on the same side of the transversal are equal. Will the two lines always be parallel?
Question 26 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d14af59b460d7261f413.png' />
In the given figure, if AB || CD || EF, PQ || RS, $∠$RQD = $25^{\circ}$ and ∠CQP = $60^{\circ}$, then $∠$QRS is equal to _______.
Question 27 :
State true or false: Bisectors of angles B and C of a triangle ABC intersect each other at the point O. Thus, $\angle BOC=90^{\circ}+\frac{1}{2}\angle A$.
Question 28 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d14ff59b460d7261f41a.png' />
In the above figure, $∠$1 = $60^{\circ}$ and ∠6 = $120^{\circ}$. Thus, the lines 'm' and 'n' are ____________.
Question 29 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d152f59b460d7261f41f.png' />
In the above figure, DE || QR and AP and BP are bisectors of $∠$ EAB and $∠$ RBA, respectively. Find $∠$APB.
Question 30 :
Two adjacent angles are equal. Is it necessary that each of these angles will be a right angle?
Question 31 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d150f59b460d7261f41c.png' />
If in the above figure, bisectors AP and BQ of the alternate interior angles are parallel, then 'l' and 'm' are _____________.
Question 32 :
Two lines are respectively perpendicular to two parallel lines. Hence, the two perpendiculars are eventually ___________ to each other.
Question 33 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d151f59b460d7261f41e.png' />
In the above figure, BA || ED and BC || EF. Find ($∠$ABC + $∠$DEF)
Question 34 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d14ef59b460d7261f419.png' />
In the above figure, OD is the bisector of $∠$AOC, OE is the bisector of $∠$BOC and OD $⊥$ OE. Hence, the points A, O and B are ______________.
Question 35 :
State true or false: An exterior angle of a triangle is equal to the sum of the corresponding two interior opposite angles.
Question 36 :
If two interior angles on the same side of a transversal intersecting two parallel lines are in the ratio 2 : 3, then the greater of the two angles is
Question 38 :
The angles of a triangle are in the ratio 5 : 3 : 7. The triangle is _________.
Question 39 :
If a transversal intersects two parallel lines, then interior angles on the same side of the transversal are _____________.
Question 40 :
An exterior angle of a triangle is $105^{\circ}$ and its two interior opposite angles are equal. What is the value of each of these equal angles?
Question 41 :
If a transversal intersects two parallel lines, then corresponding angles are __________.