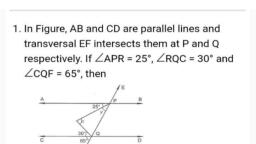
Question 1 :
If $∆ PQR ≅ ∆ EDF$, then is it true to say that PR = EF?
Question 2 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d258f59b460d7261f595.png' />
In the above fig, AD and BC are equal perpendiculars to a line segment AB. Does CD bisect AB?
Question 3 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d246f59b460d7261f57e.PNG' />
In the above fig, AB is a line segment and line $l$ is its perpendicular bisector. If a point P lies on $l$, is the point P equidistant from A and B?
Question 4 :
Two sides of a triangle are of lengths 5 cm and 1.5 cm. The length of the third side of the triangle cannot be
Question 5 :
Which of the following is not a criterion for congruence of triangles?
Question 6 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d249f59b460d7261f583.PNG' />
In the above fig, we have to prove that angles opposite to equal sides of an isosceles triangle are equal. This can be done by drawing a line AD which is a _________.
Question 7 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d245f59b460d7261f57d.PNG' />
In the above fig, AB is a line segment and line $l$ is its perpendicular bisector. Is ∆ PCA ≅ ∆ PCB?
Question 8 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d268f59b460d7261f5aa.PNG' />
In the above fig, ∆ ABC and ∆ DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC. If AD is extended to intersect BC at P, is ∆ ABD ≅ ∆ ACD?
Question 9 :
D is a point on the side BC of a $∆$ ABC such that AD bisects $∠$BAC. Then
Question 10 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d25df59b460d7261f59b.png' />
In the above fig, AC = AE, AB = AD and ∠BAD = ∠EAC. Is BC = DE?
Question 11 :
Bisectors of the angles B and C of an isosceles triangle with AB = AC intersect each other at O. BO is produced to a point M. Thus,
Question 12 :
M is a point on side BC of a triangle ABC such that AM is the bisector of $∠$BAC. Is it true to say that perimeter of the triangle is greater than 2 AM?
Question 13 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d253f59b460d7261f591.PNG' />
In the quadrilateral ACBD shown in the fig above, AC = AD and AB bisects ∠A. What can you say about BC and BD?
Question 14 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d243f59b460d7261f57a.PNG' />
Are the triangles shown in the above figure congruent?
Question 15 :
In $∆$ ABC, BC = AB and $∠$B = 80$^{\circ}$. Then $∠$A is equal to
Question 16 :
If two sides of a triangle are unequal, the angle opposite to the longer side is __________.
Question 17 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d254f59b460d7261f592.PNG' />
In the above fig, ABCD is a quadrilateral in which AD = BC and ∠DAB = ∠CBA. Is ∆ ABD ≅ ∆ BAC?
Question 18 :
Is it possible to construct a triangle with lengths of its sides as 4 cm, 3 cm and 7 cm?
Question 19 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d24ef59b460d7261f58b.PNG' />
Are the two triangles shown in the above fig congruent?
Question 20 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d259f59b460d7261f597.PNG' />
In the fig above, $l$ and $m$ are two parallel lines intersected by another pair of parallel lines p and q. ∆ ABC ≅ ∆ CDA are congruent by which rule of congruence?
Question 21 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d255f59b460d7261f593.PNG' />
In the above fig, ABCD is a quadrilateral in which AD = BC and ∠DAB = ∠CBA. Is BD = AC?
Question 23 :
In an isosceles triangle ABC, with AB = AC, the bisectors of ∠B and ∠C intersect each other at O. Join A to O. Does AO bisect ∠A?
Question 24 :
S is any point in the interior of $∆$ PQR. Hence, (SQ + SR) ___ (PQ + PR).
Question 25 :
Two or more figures that are equal in all respects or figures whose shapes and sizes are both the same are referred to as _________ figures.
Question 26 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d24af59b460d7261f584.PNG' />
In the above fig, can we say that if two angles of any triangle are equal, then the sides opposite to them are also equal?
Question 27 :
O is a point in the interior of a square ABCD such that OAB is an equilateral triangle. Thus, $∆$ OCD is (a/an) ____________ triangle.
Question 28 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d242f59b460d7261f579.PNG' />
Are the triangles shown in the above figure congruent?
Question 29 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d26cf59b460d7261f5b0.PNG' />
In the above fig, sides AB and AC of ∆ ABC are extended to points P and Q respectively. Also, ∠PBC < ∠QCB. Which of the following is true?
Question 30 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d244f59b460d7261f57b.png' />
In the above fig, OA = OB and OD = OC. Is ∆ AOD ≅ ∆ BOC?
Question 31 :
In triangles ABC and DEF, $∠A = ∠D, ∠B = ∠E$ and AB = EF. Will the two triangles be congruent?
Question 32 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d25cf59b460d7261f59a.png' />
In the above fig, AC = AE, AB = AD and ∠BAD = ∠EAC. Is ∆ ABC ≅ ∆ ADE?
Question 33 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d267f59b460d7261f5a8.PNG' />
In the above fig, ABC and DBC are two isosceles triangles on the same base BC. Is ∠ABD not equal to ∠ACD?
Question 34 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d262f59b460d7261f5a1.PNG' />
In the fig above is shown a right triangle ABC, right angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B. Is CM = $\frac{1}{2}$ AB?
Question 35 :
State true or false: ABCD is a quadrilateral such that AB = AD and CB = CD. Also, AC is the perpendicular bisector of BD.
Question 36 :
ABC is an isosceles triangle in which AC = BC. AD and BE are respectively two altitudes to sides BC and AC. So,
Question 37 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d265f59b460d7261f5a5.PNG' />
In the fig above, ABC is an isosceles triangle in which altitudes BE and CF are drawn to equal sides AC and AB respectively. Are these altitudes equal?
Question 38 :
State true or false: If in a triangle ABC, D is the mid-point of side AC such that BD = $\frac{1}{2}$ AC, then, $∠$ABC is a right angle.
Question 39 :
In triangles ABC and PQR, $∠A = ∠Q and ∠B = ∠R$. Which side of $∆$ PQR should be equal to side BC of $∆$ ABC so that the two triangles are congruent?
Question 40 :
State true or false: In a triangle, the sum of any two sides is greater than the third side.
Question 41 :
State true or false: A point equidistant from two intersecting lines lies on the bisectors of the angles formed by the two lines.
Question 42 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d263f59b460d7261f5a3.PNG' />
In the fig above is shown ∆ ABC where AD is the perpendicular bisector of BC. ∆ ABC is an isosceles triangle with _________.
Question 43 :
State true or false: If two angles and a side of one triangle are equal to two angles and a side of another triangle, then the two triangles must be congruent.
Question 44 :
In $∆$ PQR, $∠$R = $∠$P and QR = 4 cm and PR = 5 cm. Then the length of PQ is
Question 45 :
In order to show that the angles of an equilateral triangle are 60° each, how many different pairs of congruent triangles do we need?
Question 46 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d26ff59b460d7261f5b4.PNG' />
In the above fig , PR > PQ and PS bisects ∠QPR. Which of the following is true?
Question 47 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d24cf59b460d7261f588.PNG' />
In the above fig, E and F are respectively the mid-points of equal sides AB and AC of ∆ ABC. Is BF = CE?
Question 48 :
<img style='object-fit:contain' src='https://teachmint.storage.googleapis.com/question_assets/cbse_ncert/61b1d24df59b460d7261f589.PNG' />
In the above fig is shown an isosceles triangle ABC with AB = AC, D and E are points on BC such that BE = CD. Is ∆ ABD ≅ ∆ ACE?
Question 49 :
In triangles ABC and PQR, AB = AC, $∠$C = $∠$P and $∠$B = $∠$Q. The two triangles are
Question 50 :
Of all the line segments drawn from a given point not on it, the __________ line segment is the shortest.