Page 3 :
ARIHANT PRAKASHAN (School Division Series), , © Publisher, No part of this publication may be re-produced, stored in a retrieval system or by any, means, electronic, mechanical, photocopying, recording, scanning, web or otherwise, without the written permission of the publisher. Arihant has obtained all the information, in this book from the sources believed to be reliable and true. However, Arihant or its, editors or authors or illustrators don’t take any responsibility for the absolute accuracy of, any information published and the damage or loss suffered thereupon., , All disputes subject to Meerut (UP) jurisdiction only., Administrative & Production Offices, Regd. Office, ‘Ramchhaya’ 4577/15, Agarwal Road, Darya Ganj, New Delhi -110002, Tele: 011- 47630600, 43518550, , Head Office, Kalindi, TP Nagar, Meerut (UP) - 250002, Tel: 0121-7156203, 7156204, , Sales & Support Offices, Agra, Ahmedabad, Bengaluru, Bareilly, Chennai, Delhi, Guwahati,, Hyderabad, Jaipur, Jhansi, Kolkata, Lucknow, Nagpur & Pune., , ISBN : 978-93-25793-65-1, PO No : TXT-XX-XXXXXXX-X-XX, Published by Arihant Publications (India) Ltd., For further information about the books published by Arihant, log on to, www.arihantbooks.com or e-mail at info@arihantbooks.com, Follow us on, , CBSE, New Pattern
Page 4 :
Contents, Chapter, Physical World, , -, , Chapter, Units and Measurements, , -, , Chapter, Motion in a Straight Line, , -, , Chapter, Motion in a Plane, , -, , Chapter, Laws of Motion, , -, , Chapter, Work, Energy and Power, , -, , Chapter, System of Particles and Rotational Motion, , -, , Chapter, Gravitation, , -, , Practice Papers, , CBSE, New Pattern, , -, , -
Page 5 :
Syllabus (Rationalised), (Term I), Max Marks :, , Time : One and Half hours, , No. of Periods Marks, Unit I, , Physical World and Measurement, Chapter : Physical World, Chapter : Units and Measurements, , Unit II, , Kinematics, Chapter : Motion in a Straight Line, Chapter : Motion in a Plane, , Unit III, , Laws of Motion, Chapter : Laws of Motion, , Unit IV, , Work, Energy and Power, Chapter, , Unit V, , : Work, Energy and Power, , Motion of System of Particles, and Rigid Body, Chapter : System of Particles, and Rotational Motion, , Unit VI, , Gravitation, Chapter : Gravitation, Total, , UNIT-I, , Physical World and Measurement, , Chapter-, , Physical World, Physics-scope and excitement; nature of physical laws; Physics, technology, and society. To be discussed as a part of Introduction and integrated with, other topics, , CBSE, New Pattern, , Periods
Page 6 :
Chapter-, , Units and Measurements, Need for measurement: Units of measurement; systems of units; SI units,, fundamental and derived units. Length, mass and time measurements;, accuracy and precision of measuring instruments; errors in measurement;, significant figures., Dimensions of physical quantities, dimensional analysis and its applications., , UNIT-II, , Kinematics, , Chapter-, , Motion in a Straight Line, Elementary concepts of differentiation and integration for describing, motion, uniform and non- uniform motion, average speed and, instantaneous velocity, uniformly accelerated motion, velocity - time and, position-time graphs., Relations for uniformly accelerated motion graphical treatment ., , Chapter-, , Motion in a Plane, Scalar and vector quantities; position and displacement vectors, general, vectors and their notations; equality of vectors, multiplication of vectors by a, real number; addition and subtraction of vectors, relative velocity, Unit, vector; resolution of a vector in a plane, rectangular components, Scalar and, Vector product of vectors., Motion in a plane, cases of uniform velocity and uniform accelerationprojectile motion, uniform circular motion., , UNIT-III, , Laws of Motion, , Chapter-, , Laws of Motion, Intuitive concept of force, Inertia, Newton s first law of motion; momentum and, Newton s second law of motion; impulse; Newton s third law of motion., Recapitulation only, Law of conservation of linear momentum and its applications., Equilibrium of concurrent forces, Static and kinetic friction, laws of friction,, rolling friction, lubrication., Dynamics of uniform circular motion: Centripetal force, examples of circular, motion vehicle on a level circular road, vehicle on a banked road ., , CBSE, New Pattern, , Periods, , Periods
Page 7 :
UNIT-IV, , Work, Energy and Power, , Chapter-, , Work, Energy and Power, Work done by a constant force and a variable force; kinetic energy, workenergy theorem, power., Notion of potential energy, potential energy of a spring, conservative forces:, conservation of mechanical energy kinetic and potential energies ;, non-conservative forces: motion in a vertical circle; elastic and inelastic, collisions in one and two dimensions., , UNIT-V, , Motion of System of Particles and Rigid Body, , Chapter-, , System of Particles and Rotational Motion, Centre of mass of a two-particle system, momentum conservation and, centre of mass motion. Centre of mass of a rigid body; centre of mass of a, uniform rod., Moment of a force, torque, angular momentum, law of conservation of, angular momentum and its applications., Equilibrium of rigid bodies, rigid body rotation and equations of rotational, motion, comparison of linear and rotational motions., Moment of inertia, radius of gyration, values of moments of inertia for simple, geometrical objects no derivation ., , UNIT-VI, , Gravitation, , Chapter-, , Gravitation, Universal law of gravitation. Acceleration due to gravity recapitulation only, and its variation with altitude and depth., Gravitational potential energy and gravitational potential, escape velocity,, orbital velocity of a satellite, Geo-stationary satellites., , CBSE, New Pattern, , Periods, , Periods, , Periods
Page 12 :
CBSE New Pattern ~ Physics 11th (Term-I), , 01, , 01, Physical World, Quick Revision, 1. Science It is exploring, experimenting and, , predicting from what we see around us. It is, a systematic attempt to understand natural, phenomena., 2. Physics It refers to the study of the physical, world, i.e. the study of the basic laws of nature, and their manifestation in different natural, phenomena., 3. Scope and Excitement of Physics To define, the scope and excitement of Physics, it is, categorised into two groups, on the basis of, magnitude of physical quantities involved in it,, i.e. macroscopic and microscopic groups of, Physics., 4. Macroscopic Group of Physics It deals with, the subjects included in Classical Physics. It, consists of phenomena at the laboratory,, terrestrial and astronomical scales., Classical physics can be classified as, ● Mechanics It deals with the study of motion, of particles, rigid and deformable bodies and, general system of particles. It is based on the, law of gravitation and Newton’s laws of, motion., ● Electrodynamics It deals with the study of, electric and magnetic phenomena associated, with charged and magnetic bodies. It is based, on the laws given by Coulomb, Oersted,, Ampere and Faraday., , Optics It deals with the study of, phenomena related to light, working of, human eye, telescope, microscope, etc., ● Thermodynamics It deals with the study of, the system in macroscopic equilibrium, considering changes in internal energy,, temperature, entropy, etc., 5. Microscopic Group of Physics It deals with, the study of constituents and structure of, matter at minute scale of length, i.e. at the, scale of atoms and nuclei or even smaller than, these. This group of Physics can be studied, under the subject Quantum Physics., 6. Fundamental Forces in Nature There are, following four fundamental forces in nature, ● Gravitational Force The force of mutual, attraction between any two objects because, of their masses is called gravitational force., This force was discovered by Isaac Newton., ●, Electromagnetic Force The force, associated with charged particles is called, electromagnetic force., ● Strong Nuclear Force It is the force, which binds the protons and neutrons, together inside a tiny nucleus., ● Weak Nuclear Force The force which, appears only between elementary particles, involved in nuclear processes of, radioactivity like b- decay of a nucleus, etc., ●
Page 13 :
02, , CBSE New Pattern ~ Physics 11th (Term-I), , 7. Comparison between Four Fundamental, Forces, Name, Gravitational force, , Relative, strength, , Range, , 10-39, , Infinite, , -13, , Weak nuclear force, , 10, , Electromagnetic, force, , 10-2, , Strong nuclear force, , 1, , Very short,, sub-nuclear, size (~10-16 m), Infinite, , ●, , Short,, nuclear size, (~10 -15 m), , 8. Nature of Physical Laws Physicists observed, that during a physical phenomenon governed, by different forces, several quantities may, change with time but some special physical, quantities remain constant with time. They are, called conserved quantities of nature and, this is called law of conservation., There are four laws of conservation in classical, Physics, ● Law of Conservation of Energy It states, that, energy can neither be created nor be, destroyed, but it can be changed from one, form to another, i.e. the total sum of all kinds, of energy in this universe remains same., ● Law of Conservation of Mass Earlier it, was assumed that, mass is indestructible and, law of conservation of mass states that, matter, can neither be created nor be destroyed., , ●, , But Einstein’s theory of relativity, (energy-mass relation, E = mc 2, where m is, the mass and c is the speed of light in, vacuum) has modified it., In a nuclear process, mass gets converted to, energy (or vice-versa). This is the energy, which is released in a nuclear power, generation and nuclear explosion., Law of Conservation of Momentum, Momentum is the quantity of motion of a, moving body (generally measured as the, product of mass and velocity of the body)., Momentum of an isolated system is also, conserved. It can be classified into two types, (linear momentum and angular momentum), and law of conservation is valid for both of, them., (a) Law of Conservation of Linear, Momentum This law states that, if no, external force acts on a system, then its, linear momentum remains constant, i.e., When S Fext = 0, then p = constant., (b) Law of Conservation of Angular, Momentum It states that, if no external, torque acts on a system, then its angular, momentum remains constant, i.e. when, S t ext = 0, then L = constant., Law of Conservation of Charge This law, states that, the net charge of an isolated, system remains constant.
Page 14 :
CBSE New Pattern ~ Physics 11th (Term-I), , 03, , Objective Questions, Multiple Choice Questions, 1. Physics is the branch of science which, deals with the study of, (a), (b), (c), (d), , practical purposes, living things, technologies, nature and natural phenomena, , 2. In Physics, quantitative measurement is, central to the growth of science because, (a) laws of nature are expressible in precise, mathematical equations, (b) basic laws universally apply in different, contexts, (c) strategy of approximation turned out to be, very successful, (d) All of the above, , 3. With which phenomena, classical, Physics deals mainly?, (a) Macroscopic, (c) Natural, , (b) Microscopic, (d) None of these, , 4. Microscopic domain includes, (a) quantum theory, (c) thermodynamics, , (b) mechanics, (d) sound, , 5. Observable universe has range of mass, of, (a) 1020 kg, (c) 1040 kg, , (b) 1030 kg, (d) 1055 kg, , 6. Maxwell’s set of equation encapsulated, basic laws such as, (a), (b), (c), (d), , Coulomb and Oersted’s laws, Ampere and Faraday’s laws, Faraday’s and Optic laws, Both (a) and (b), , 7. The phenomena that optics deals with are, (a), (b), (c), (d), , light, working of telescopes and microscopes, colours exhibited by thin films, All of the above, , 8. Conservation laws are such that, (a), (b), (c), (d), , it cannot be proved but can be verified, it can neither be proved nor can be verified, it can be proved and verified, it can be proved but not verified, , 9. Radio and television are based on, (a) inverse square law of charges, (b) production, propagation and reception of, electromagnetic waves, (c) digital logic, (d) mechanics, , 10. The person who had been awarded the, title of the Father of Physics of 20th, century is, (a), (b), (c), (d), , Madam Curie, Sir C.V. Raman, Neils Bohr, Albert Einstein, , 11. In Physics, the range of time scales used, is ……… ., (a) 1015 s to 10- 15 s, (c) 10- 20 s to 109 s, , (b) 10- 22 s to 1018 s, (d) 10- 17 s to 1020 s, , 12. According to Einstein’s theory of, relativity, energy-mass relation is, ……… ., (a) E = mc, (c) E = mc 2, , (b) E = m/c 2, (d) E = 2 mc 2, , 13. ……… was discovered by Huygens’., (a), (b), (c), (d), , Wave theory of light, Quanta of light, Particle nature of light, None of the above, , 14. Which of the following statement is not, correct?, (a) Physics is the study of nature and natural, phenomena., (b) Physics and technology are not related to, each other.
Page 15 :
04, , CBSE New Pattern ~ Physics 11th (Term-I), , (c) Electrodynamics deals with electric and, magnetic phenomena associated with, charged and magnetic bodies., (d) The physical quantities that remain, unchanged in a process are called, conserved quantities., , 15. Study the following statements, regarding conservation law and choose, the incorrect option., (a) Conservation law is a hypothesis based on, observations and experiments., (b) Conservations laws do not have a deep, connection with symmetries of nature., (c) A conservation law cannot be proved., (d) Conservation of energy, linear momentum, and angular momentum are considered to, be fundamental laws of physics., , 16. Choose the correct statement from the, following options., (a) An axiom is self-evident truth while a model, is a theory proposed to explain observed, phenomena., (b) Wireless communication followed the, discovery of basic laws of electricity and, magnetism., (c) Bohr had dismissed the possibility of, tapping energy from atoms., (d) Both (a) and (b), , 17. Match the Column I (domains) with, Column II (relation) and select the, correct option from the codes given, below., Column I, , Column II, , A., , Mechanics, , p., , electric and, magnetic fields, , B., , Electrodynamics, , q., , macroscopic, equilibrium, , C., , Thermodynamics, , r., , Newton’s laws, of motion, , Codes, A, , B, , C, , A, , B, , C, , (a) p, , q, , r, , (b) r, , p, , q, , (c) p, , r, , r, , (d) q, , r, , p, , 18. Match the Column I (physical, quantities) with Column II (scale) and, select the correct option from the codes, given below., Column I, , Column II, , A., , Size of electron, or proton, , p. 10 -30 kg, , B., , Mass of an, electron, , q. 10 -14 m, , C., , Extent of, universe, , r., , 10 26 m, , Codes, A, (a) q, (b) q, (c) s, , B, p, r, p, , C, r, p, r, , (d) q, , p, , q, , 19. Match the Column I (name of, physicists) with Column II, (contribution/discovery) and select the, correct option from the codes given, below., Column I, , Column II, , A., , Johannes, Kepler, , p., , Nuclear model, of the atom, , B., , Tycho Brahe, , q., , Planetary, motion, , C., , Nicolaus, Copernicus, , r., , Elliptical orbit, theory, , D., , Ernest, Rutherford, , s., , Circular orbit, theory, , Codes, A, , B, , C, , D, , (a) q, , s, , r, , p, , (b) p, , q, , r, , s, , (c) q, , p, , s, , r, , (d) r, , q, , s, , p
Page 16 :
CBSE New Pattern ~ Physics 11th (Term-I), , Assertion-Reasoning MCQs, , 05, , 22. Assertion Symmetry of laws of nature, , For question numbers 20 to 25, two, statements are given-one labelled, Assertion (A) and the other labelled, Reason (R). Select the correct answer to, these questions from the codes (a), (b), (c), and (d) are as given below, (a) Both A and R are true and R is the, correct explanation of A., (b) Both A and R are true but R is not the, correct explanation of A., (c) A is true but R is false., (d) A is false and R is also false., , 20. Assertion The concept of energy is, , with respect to translation in space give, rise to conservation of linear, momentum., Reason Isotropy of space does not, underlies the law of conservation of, angular momentum., , 23. Assertion According to the principle, of conservation of energy, all heat can, be converted into mechanical work., Reason Due to various losses, it is, impossible to convert all heat into, mechanical work., , central to Physics and its expression can, be written for every physical system., , 24. Assertion Matter can neither be, , Reason Law of conservation of energy, is not valid for all forces and for any, kind of transformation between, different forms of energy., , Reason This is law of definite, proportions., , 21. Assertion Physics generates new, technology., Reason Technology give rise to new, physics., , created nor be destroyed., , 25. Assertion Electric force and magnetic, force are jointly called electromagnetic, force., Reason Electric and magnetic effects, are inseparable., , ANSWERS, Multiple Choice Questions, 1. (d), 11. (b), , 2. (d), 12. (c), , 3. (a), 13. (a), , 4. (a), 14. (b), , 5. (d), 15. (b), , 6. (d), 16. (d), , 23. (b), , 24. (c), , 25. (a), , Assertion-Reasoning MCQs, 20. (c), , 21. (b), , 22. (c), , 7. (d), 17. (b), , 8. (a), 18. (a), , 9. (b), 19. (a), , 10. (d)
Page 17 :
06, , CBSE New Pattern ~ Physics 11th (Term-I), , SOLUTIONS, 1. Nature and natural phenomena; for example, motion of the moon around the earth, etc.,, are dealt with in Physics., 2. Quantitative measurement in Physics is, central to the growth of science because all, the basic universal laws apply in different, context., Also, laws of nature are expressible in, mathematical equations and strategy of, approximation turned out to be very, successful., , 3. Classical Physics deals mainly with, , 4., , 5., 6., , 7., , 8., , 9., , 10., , macroscopic phenomena and includes, subjects like mechanics, electrodynamics,, optics and thermodynamics., Quantum theory explains microscopic, domain involving molecules, atoms, electrons, and other elementary particles., Mass of observable universe has a range of, 10 55 kg., The basic laws regarding electromagnetism, given by Oersted, Coulomb, Ampere and, Faraday. These were encapsulated by, Maxwell in his famous set of equations., Optics deals with the study of phenomena, related to light. So, the working of human eye,, telescope, microscope, colours that exhibits by, thin films etc., are all studied under this branch., Conservation laws are basically hypothesis,, based on observations and experiments., Thus, these laws cannot be proved but can, be verified or disproved by experiments., Radio and television are based on production, (generation), propagation and reception, (detection) of electromagnetic waves., Albert Einstein was awarded the title of the, Father of Physics of 20th century., Range of time scales is 10 -22 s to 10 18 s., , 11., 12. Energy-mass relation is E = mc 2., , 13. Huygens’ discovered the wave theory of light., 14. The statement given in option (b) is incorrect, and it can be corrected as,, Physics and technology are interdependent to, each other., , 15. The statement given in option (b) is incorrect, and it can be corrected as,, Conservation laws have a deep connection, with symmetries of nature. Symmetries of, space and time and other types of symmetries, play a central role in modern theories of, fundamental forces in nature., , 16. Wireless communication technology followed, the discovery of the basic laws of electricity, and magnetism in the nineteenth century., Axiom is a self-evident truth that it is, accepted without controversy while model is, a theory proposed to explain observed, phenomena., A., Mechanics is based on Newton’s laws of, 17., motion., B. Electrodynamics deals with electric and, magnetic phenomena associated with, charged and magnetic bodies., C. Thermodynamics in contrast to mechanics,, does not deal with the motion of bodies as, a whole. Rather, it deals with systems in, macroscopic equilibrium and is concerned, with changes in internal energy,, temperature, entropy, etc., of the system, through external work and transfer of heat., Hence, A ® r, B ® p and C ® q., , 18. The correct match of this question is, A ® q, B ® p and C ® r., , 19. The correct match of this question is, A ® q, B ® s, C ® r and D ® p., , 20. Law of conservation of energy is always valid, for all forces and for any kind of, transformation between different forms of, energy., Therefore, A is true but R is false., , 21. Sometimes physics generates new technology, and at others technology gives rise to new, physics. Both have desired impact on society., Therefore, both A and R are true but R is, not the correct explanation of A., , 22. Symmetry of natural laws with respect to, translation in space give rise to conservation, of linear momentum.
Page 18 :
CBSE New Pattern ~ Physics 11th (Term-I), , Isotropy of space (no intrinsically preferred, direction in space) underlies the law of, conservation of angular momentum., Therefore, A is true but R is false., , 23. According to the law of conservation of, energy, energy can neither be created nor it, can be destroyed. Thus, it is physically, possible to convert all of heat into, mechanical work. But due to various energy, losses, this cannot be achieved partically., Therefore, both A and R are true but R is, not the correct explanation of A., 24. Law of conservation of energy states that,, matter can never be created nor be, , 07, destroyed. Law of definite proportions states, that, molecules will always have elements in a, particular ratio which will also be fixed and not, dependent on the method of preparation of the, molecule., Therefore, A is true but R is false., , 25. Charges in motion produces magnetic effects,, these effects give rise to a force on a moving, charge. So, electric and magnetic effects are, inseparable., Therefore, it is named as electromagnetic, force., Therefore, both A and R are true and R is the, correct explanation of A.
Page 19 :
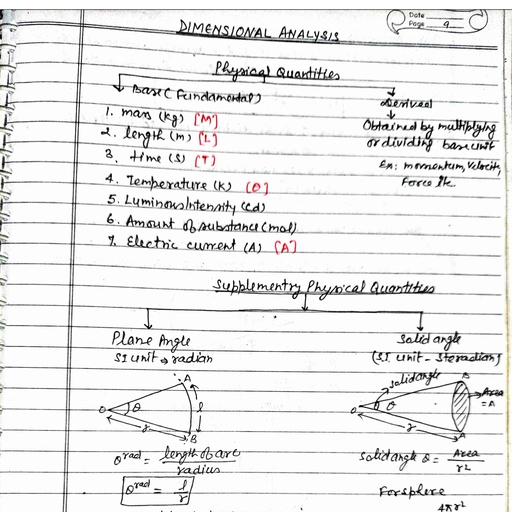
08, , CBSE New Pattern ~ Physics 11th (Term-I), , 02, Units and, Measurements, Quick Revision, 1. Physical Quantities All the quantities which, can be measured directly or indirectly and, in terms of which laws of Physics are described, are called physical quantities., These can be divided into two types, namely, fundamental and derived quantities., ● Fundamental Quantities The physical, quantities which are independent of other, physical quantities and are not defined in, terms of other physical quantities are called, fundamental or base quantities., e.g. Mass, length, time, temperature, luminous, intensity, electric current, amount of, substance, etc., ● Derived Quantities Those quantities which, can be derived from the fundamental physical, quantities are called derived quantities., e.g. Velocity, acceleration, linear momentum,, etc., 2. Physical Unit The standard amount of a, physical quantity chosen to measure the, physical quantity of same kind is called a, physical unit. The physical units can be, classified into following two types, ● Fundamental Units The units of, fundamental quantities are known as, fundamental units., , Derived Units The units of measurement, of all other physical quantities, which can, be obtained from fundamental units are, called derived units., 3. System of Units It is the complete set of, units, both fundamental and derived physical, units., The common system of units used in, mechanics are as follows, ●, The FPS System It is the British, engineering system of units. It uses foot as, the unit of length, pound as the unit of mass, and second as the unit of time., ● The CGS System It is the French system of, units, which uses centimetre, gram and, second as the units of length, mass and time,, respectively., ● The MKS System It uses metre, kilogram, and second as the fundamental units of, length, mass and time, respectively., ● The International System of Units, (SI Units) The system of units which is, accepted internationally for measurement is, the ‘Systeme International d’ Units (French, for International System of Units),, abbreviated as SI., ●
Page 20 :
CBSE New Pattern ~ Physics 11th (Term-I), , 4. Fundamental Quantities and their SI Units, Fundamental, quantity, , SI unit, , Symbol, , Length, , metre, , m, , Mass, , kilogram, , kg, , Time, , second, , s, , Electric current, , ampere, , A, , Temperature, , kelvin, , K, , Amount of, substance, , mole, , mol, , Luminous intensity, , candela, , cd, , 5. Supplementary Quantities and their SI Units, Supplementary, quantity, , SI unit, , Plane angle, , radian, , Solid angle, , steradian, , Symbol, rad, sr, , 6. Some Important Practical Units, For Length/Distance, ● Astronomical Unit It is the mean distance, of the earth from the sun., 1 AU = 1.496 ´ 10 11 m, ●, Light year It is the distance travelled by, light in vacuum in one year., 1 ly = 9.46 ´ 10 15 m, -6, ● Micro or micrometer, 1 mm = 10, m, ●, , Nanometer, 1 nm = 10 -9 m, , ●, , Angstrom, 1 Å = 10 -10 m, , Fermi This unit is used for measuring, nuclear sizes. 1 Fm = 10 -15 m, For Mass, ● Pound, 1 lb = 0.4536 kg, ●, , ●, , Slug, 1 slug = 14.59 kg, , ●, , Quintal, 1 q = 100 kg, , ●, , Tonne or metric tonne, 1 t = 1000 kg, , ●, , Atomic mass unit (It is defined as the, (1/12)th of the mass of one 12, C-atom) 1 u or, 6, amu = 1.66 ´ 10 –27 kg., , 09, For Area, -28, ● Barn, 1 barn = 10, m2, 2, ● Acre, 1 acre = 4047 m, 4, ● Hectare, 1 hectare = 10, m2, 7. Accuracy and Precision of Instruments, The accuracy of a measurement is a measure of, how close the measured value is to the true, value of the quantity. While, precision tells us, to what resolution or limit the quantity is, measured., 8. Errors in Measurement, Difference in the true value and the measured, value of a quantity is called error in, measurement., Error = True value – Measured value, In general, the errors can be further classified as, ●, Systematic Errors Those errors that tend, to be in one direction, either positive or, negative are called systematic errors. Some, of the sources of systematic errors are, (a) Instrumental errors They occur due to, imperfect design or manufacture or, calibration of the measuring instrument., (b) Imperfection in experimental, technique or procedure These types of, errors occur due to the experimental, arrangement limitations., (c) Personal errors These errors arise due, to inexperience of the observer such as lack, of proper setting of the apparatus and, taking observations without observing, proper precautions, etc., (d) Errors due to external causes Various, parameters such as change in, temperature, pressure, volume, etc. during, experiment may affect the reading of, measurement., ● Random Errors The errors which occur, irregularly and are random in magnitude, and direction are called random errors., ● Least Count Error The smallest value that, can be measured by a measuring instrument, is called the least count of the instrument, and error in its value is called least count, error.
Page 21 :
10, , CBSE New Pattern ~ Physics 11th (Term-I), , ●, , ●, , ●, , ●, , Absolute Error The magnitude of the, difference between the true value of the, quantity and the individual measured value, is called the absolute error of the, measurement. It is denoted by | D a |., Suppose, the measured values are, a 1, a 2, a 3, K , a n , then arithmetic mean of, a + a2 + K + an, these values is a Mean = 1, ., n, If we take arithmetic mean a Mean as the true, value, then the absolute errors in the, individual measured value will be, Da 1 = a Mean – a 1, Mean Absolute Error It is the arithmetic, mean of the magnitudes of absolute errors in, all the measurements of the quantity., n | Da |, i, Mean absolute error, D a Mean = S, i =1, n, Relative Error or Fractional Error It is, defined as the ratio of mean absolute error to, the mean value of the quantity measured., D a Mean, Relative error, da =, a Mean, Percentage Error When fractional error or, relative error is expressed in percentage,, then it is called percentage error., D a Mean, Percentage error, da % =, ´ 100%, a Mean, , 9. Combination of Errors, ● Error in Sum or Difference, Let X = A + B or X = A - B, where, A and B are physical quantities have, measured value A ± DA, B ± DB ,, respectively., , ●, , So, the maximum possible error in sum and, difference, DZ = DA + DB, Error in Product or Quotient, A, Let, X =, X = A ´ B or, B, So, the maximum possible error in product, DZ, DA DB, or quotient is, =, +, Z, A, B, , ●, , Error in Case of a Measured Quantity, Raised to a Power, Relative error of Z = A n B m is, DZ, DB ù, é DA, = ± ên, +m, Z, A, B úû, ë, , 10. Significant Figures, The digits that are known reliably plus the first, uncertain digit are known as significant digits, or significant figures., Rules for Determining the Number of, Significant Figures, Rule 1 All non-zero digits are significant., Rule 2 All the zeros between two non-zero, digits are significant, no matter where, the decimal point is, if at all., Rule 3 If the number is less than one, the, zero(s) on the right of decimal point, and to the left of first non-zero digit, are not significant., Rule 4 In a number without a decimal point,, the terminal or trailing zeros is not, significant., Rule 5 The trailing zero(s) in a number with a, decimal points are significant., 11. Rules for Arithmetical Operations with, Significant Figures, Some rules of arithmetical operations with, significant figures are as given below, ● Addition and Subtraction In both,, addition and subtraction, the final result, should retain as many decimal places as, are there in the number with the least, decimal places., ● Multiplication and Division In, multiplication or division, the final result, should retain as many significant figures, as, are there in the original number with the, least significant figures., 12. Rounding Off The result of computation with, approximate numbers, which contains more, than one uncertain digit, should be rounded, off. While rounding off measurements, we use, the following rules by convention
Page 22 :
CBSE New Pattern ~ Physics 11th (Term-I), , Rule 1 If the digit to be dropped is less than 5,, then the preceding digit is left, unchanged., Rule 2 If the digit to be dropped is more than, 5, then the preceding digit is raised by, one., Rule 3 If the digit to be dropped is 5 followed, by digits other than zero, then the, preceding digit is raised by one., Rule 4 If the digit to be dropped is 5 and, followed by zeros, then the preceding, digit is left unchanged, if it is even., Rule 5 If the digit to be dropped is 5 and, followed by zeros, then the preceding, digit is raised by one, if it is odd., 13. Dimensions of a Physical Quantity, The dimensions of a physical quantity are the, powers (or exponents) to which the units of, base quantities are raised to represent a, derived unit of that quantity. There are seven, base quantities represented with square brackets, [ ] such as length [L], mass [M], time [T], electric, current [A], thermodynamic temperature [K],, luminous intensity [cd] and amount of, substances [mol]., 14. Dimensional Formulae and, Dimensional Equations, The expression which shows how and which of, the fundamental quantities represent the, dimension of the physical quantity is called the, dimensional formula of the given physical, quantity., Some of the dimensional formulae are as given, below, Acceleration = [M 0 L1 T -2 ], Mass density = [ML-3 T 0 ], Volume = [M 0 L3 T 0 ], , 11, The equation obtained by equating a physical, quantity with its dimensional formula is called, the dimensional equation of the given, physical quantity., 15. Dimensional Analysis and its Applications, The dimensional analysis helps us in deducing, the relations among different physical quantities, and checking the accuracy, derivation and, dimensional consistency or homogeneity of, various numerical expressions. Its applications, are as given below, ● Checking the Dimensional Consistency, of Equations The principle of homogeneity, of dimension states that, a physical quantity, equation will be dimensionally correct, if the, dimensions of all the terms occurring on, both sides of the equation are same., ● Conversion of One System of Units into, Another If M 1, L 1 and T1 are the, fundamental units of mass, length and time, in one system and while for other system,, M 2, L 2 and T2 are the fundamental units of, mass, length and time, then n 1 = [M a1 L b1 T c1 ], and n 2 = [M a2 Lb2 T2c ]., From n 1u 1 = n 2u 2, where u 1 and u 2 are two, units of measurement of the quantity and n 1, and n 2 are their respective numerical values., n [M a L b T c ], Then, n 2 = 1 a 1 b 1 c 1, [M 2 L 2 T 2 ], a, , b, , éM ù éL ù é T ù, = n1 ê 1 ú × ê 1 ú × ê 1 ú, ë M 2 û ë L 2 û ë T2 û, ●, , c, , Deducing Relation among the Physical, Quantities The method of dimensions is, used to deduce the relation among the, physical quantities. We should know the, dependence of the physical quantity on other, quantities.
Page 23 :
012, , CBSE New Pattern ~ Physics 11th (Term-I), , Objective Questions, Multiple Choice Questions, 1. The quantity having the same unit in all, system of unit is, (a) mass, (c) length, , (b) time, (d) temperature, , 2. The SI unit of thermal conductivity is, (a) J m -1 K -1, (c) W m -1 K -1, , (b) W-m K -1, (d) Jm K -1, , directly proportional to the velocity., The unit of the constant of, proportionality is, (b) kg-ms -2, (d) kg-s, , 128 kg m -3 . In certain units in which, the unit of length is 25 cm and the unit, of mass is 50 g, the numerical value of, density of the material is, (b) 16, , (c) 640, , (d) 410, , 5. If the value of work done is, , 10 10 g-cm 2 s -2 , then its value in SI units, will be, (a) 10 kg-m 2 s -2, (c) 104 kg-m 2 s -2, , (b) 102 kg-m 2 s -2, (d) 103 kg-m 2 s -2, , 6. Amongst the following options, which, is a unit of time?, (a) Light year, (c) Year, , 8. The ratio of the volume of the atom to, the volume of the nucleus is of the, order of, (b) 1025, (d) 1010, , 9. Which of the following measurement is, most precise?, (a) 5.00 mm, (c) 5.00 m, , (NCERT Exemplar), , (b) 5.00 cm, (d) 5.00 km, , 10. A student measured the length of a rod, , 4. The density of a material in SI units is, , (a) 40, , 4.5 ´ 109 m, 3.83 ´ 108 m, 2.5 ´ 104 m, 4 ´ 107 m, , (a) 1015, (c) 10 20, , 3. The damping force on an oscillator is, , (a) kg-ms -1, (c) kgs -1, , (a), (b), (c), (d), , (b) Parsec, (d) None of these, , 7. The moon is observed from two, , diametrically opposite points A and B, on earth. The angle q subtended at the, moon by the two directions of, observation is 1°54¢; given that the, diameter of the earth to be about, 1.276×10 7 m. Compute the distance of, the moon from the earth., , and wrote it as 3.50 cm. Which, instrument did he use to measure it?, , (a) A meter scale, (b) A vernier calliper where the 10 divisions in, vernier scale matches with 9 divisions in, main scale and main scale has 10 divisions, in 1 cm, (c) A screw gauge having 100 divisions in the, circular scale and pitch as 1 mm, (d) A screw gauge having 50 divisions in the, circular scale and pitch as 1 mm, , 11. The length, breadth and height of a, rectangular block of wood were, measured to be l = 1213, . ± 0.02 cm,, b = 816, . ± 0.01 cm and, h = 3.46 ± 0.01 cm., (a) 0.88%, (c) 0.78%, , (b) 0.58%, (d) 0.68%, , 12. A student measures the time period of, , 100 oscillations of a simple pendulum, four times. The data set is 90 s, 91s, 92s, and 95s. If the minimum division in the, measuring clock is 1s, then the reported, mean time should be, , (a) (92 ± 2) s, (c) (92 ± 18, . )s, , (b) (92 ± 5 ) s, (d) (92 ± 3) s
Page 24 :
CBSE New Pattern ~ Physics 11th (Term-I), , 13. In successive experiments while, , measuring the period of oscillation of a, simple pendulum. The readings turn, out to be 2.63 s, 2.56 s, 2.42 s, 2.71s and, 2.80 s. Calculate the mean absolute, error., (a) 0.11 s, , (b) 0.42 s, , (c) 0.92 s (d) 0.10 s, , 14. The period of oscillation of a simple, pendulum is T = 2p L / g . Measured, value of L is 20 cm known to 1 mm, accuracy and time for 100 oscillations of, the pendulum is found to be 90 s using a, wrist watch of 1s resolution.What is the, percentage error in the determination, of g ?, (a) 5%, (c) 4%, , (b) 3%, (d) 7%, , 15. Calculate the mean percentage error in, five observations,, 80.0, 80.5, 81.0, 81.5 and 82., (a) 0.74%, (c) 0.38%, , (b) 1.74%, (d) 1.38%, , 16. Calculate the relative errors in, , measurement of two masses 1.02 g, ± 0.01g and 9.89g + 0.01g ., (a) ± 1% and ± 0.2%, (c) ± 2% and ± 0.3%, , (b) ± 1% and ± 0.1%, (d) ± 3% and ± 0.4%, , 17. The density of a material in the shape, , of a cube is determined by measuring, three sides of the cube and its mass. If, the relative errors in measuring the, mass and length are respectively 1.5%, and 1%, the maximum error in, determining the density is, (a) 2.5%, , (b) 3.5%, , (c) 4.5% (d) 6%, , 18. Percentage errors in the measurement, , of mass and speed are 2% and 3%,, respectively. The error in the estimation, of kinetic energy obtained by, measuring mass and speed will be, (a) 8%, (c) 12%, , (b) 2%, (d) 10%, , 13, 19. If the length of a pendulum is increased, by 2%, then in a day, the pendulum, (a) loses 764 s, (c) gains 236 s, , (b) loses 924 s, (d) loses 864 s, , 20. The length and breadth of a rectangular, , sheet are 16.2 cm and 10.1 cm,, respectively. The area of the sheet in, appropriate significant figures and error is, (NCERT Exemplar), , (a) 164 ± 3 cm, (c) 163.6 ± 2.6 cm2, 2, , (b) 163.62 ± 2.6 cm2, (d) 163.62 ± 3 cm2, , 21. In an experiment, four quantities, , a, b , c and d are measured with, percentage error 1%, 2%, 3% and 4%,, respectively. Quantity P is calculated as, a3 b2, follows P =, , percentage error in, cd, P is, (a) 14%, , (b) 10%, , (c) 7%, , (d) 4%, , 22. A physical quantity z depends on four, observables a, b , c and d, as z =, , a 2b 2/3, ., cd 3, , The percentages of error in the, measurement of a, b , c and d are 2%,, 1.5%, 4% and 2.5% respectively. The, percentage of error in z is, (a) 13 . 5%, , (b) 16 . 5%, , (c) 14 . 5% (d) 12 .25%, , 23. The respective number of significant, , figures for the numbers 23.023, 0.0003, and 2.1 ´ 10 -3 are, (a) 5, 1, 2, (c) 5, 5, 2, , (b) 5, 1, 5, (d) 4, 4, 2, , 24. If 3.8 ´ 10 - 6 is added to 42 ´ 10 -6, , giving due regard to significant figures,, then the result will be, (a) 4.58 ´ 10- 5, (c) 45 ´ 10- 5, , (b) 4.6 ´ 10- 5, (d) None of these, , 25. The numbers 5.355 and 5.345 on, , rounding off to 3 significant figures will, give, , (a) 5.35 and 5.34, (c) 5.35 and 5.35, , (b) 5.36 and 5.35, (d) 5.36 and 5.34
Page 25 :
14, , CBSE New Pattern ~ Physics 11th (Term-I), , 26. The mass and volume of a body are, , 4.237 g and 2.5 cm 3 , respectively. The, density of the material of the body in, correct significant figures is, (NCERT Exemplar), , (a) 1.6048 g cm-3, (c) 1.7 g cm-3, , (b) 1.69 g cm-3, (d) 1.695 g cm-3, , 27. If mass M , distance L and time T are, , fundamental quantities, then find the, dimensions of torque., 2, , -2, , (a) [ML T ], (c) [MLT], , -2, , (b) [MLT ], (d) [ML2T], , 28. Let l , r , c and v represent inductance,, resistance, capacitance and voltage,, respectively. The dimension of l in SI, rcv, units will be, (a) [LT2], (c) [A - 1], , (b) [LTA], (d) [LA - 2], , 29. Obtain the dimensional formula for, universal gas constant., (a), (b), (c), (d), , [M L2 T- 2 mol- 1 K - 1], [ML3 T- 1 mol- 2 K - 2], [M2 LT- 1 mol- 1 K - 1], [M 3 LT- 2 mol- 1 K - 2], , physical parameters have the same, dimensions?, I. Energy density, II. Refractive index, III. Dielectric constant, IV. Young’s modulus, V. Magnetic field, (b) III and V, (d) I and V, , 31. If P , Q , R are physical quantities,, having different dimensions, which of, the following combinations can never, be a meaningful quantity?, (a) (P - Q) / R, (c) PQ / R, , with distance x from a fixed origin as, A x, U =, , where A and B are, x +B, constants. The dimensions of AB are, (a) [ML5 /2 T- 2], (c) [M3/2L3 T- 2], , (b) [ML2 T- 2], (d) [ML7 /2 T- 2], , 33. In the formula, X = 3YZ 2 , X and Z, , have dimensions of capacitance and, magnetic induction. The dimensions of, Y in MKSQ system are, (a) [M-3L-2T4Q4], (c) [M-2L-3T2 Q4], , (b) [ML2T8 Q4], (d) [M-2L-2TQ2], , 34. If the velocity v (in cms -1 ) of a particle, , is given in terms of t (in second) by the, b, ,, relation v = at +, t +c, then the dimensions of a, b and c are, a, b, c, , (a), (b), (c), (d), , [L], [L2 ], [LT2 ], [LT-2 ], , [LT], [T], [LT], [L], , [T2 ], [LT-2 ], [L], [T], , 35. A book with many printing errors, , 30. Which two of the following five, , (a) I and IV, (c) I and II, , 32. The potential energy of a particle varies, , (b) PQ - R, (d) (PR - Q 2 ) / R, , contains four different formulae for the, displacement y of a particle under going, a certain periodic motion,, where, a = maximum displacement of, the particle, v = speed of the particle,, T = time period of motion., Which are the correct formulae on, dimensional grounds?, (a) y = a sin, , 2pt, T, , æaö, (c) y = ç ÷ sin (t /a), èT ø, , (b) y = a sin vt, (d) None of these, , 36. If speedV , area A and force F are, , chosen as fundamental units, then the, dimensional formula of Young’s, modulus will be, (a) [FA 2 V -3], (c) [FA 2 V -2], , (b) [FA -1 V 0], (d) [FA 2 V -1]
Page 26 :
CBSE New Pattern ~ Physics 11th (Term-I), , 37. If dimensions of critical velocity v c of a, liquid flowing through a tube are, expressed as [h x r y r z ], where h, r and r, are the coefficient of viscosity of liquid,, density of liquid and radius of the tube, respectively, then the values of, x , y and z are given by, (a) 1, - 1, - 1, (c) -1, - 1 , - 1, , (b) -1, - 1, 1, (d) 1, 1, 1, , 38. The density of a material in CGS, , system is 10 g cm -3 . If unit of length, becomes 10 cm and unit of mass, becomes 100 g, the new value of, density will be, (a) 10 units, (c) 1000 units, , (b) 100 units, (d) 1 unit, , 39. When 1 m, 1 kg and 1 min are taken as, , the fundamental units, the magnitude of, the force is 36 units. What will be the, value of this force in CGS system?, (a) 105 dyne, (c) 108 dyne, , (b) 103 dyne, (d) 104 dyne, , 40. The solid angle subtended by the, , periphery of an area 1 cm 2 at a point, situated symmetrically at a distance of, 5 cm from the area is ……… steradian., (a) 2 ´ 10-2 (b) 4 ´ 10-2, , (c) 6 ´ 10-2 (d) 8 ´ 10-2, , 41. Measure of two quantities along with, , the precision of respective measuring, instrument is A = 25, . ms -1 ± 0.5 ms -1 ,, B = 0.10 s ± 0.01 s. The value of AB will, (NCERT Exemplar), be ……… ., (a) (0.25 ± 0.08) m, (c) (0.25 ± 0.05) m, , (b) (0.25 ± 0.5) m, (d) (0.25 ± 0.135) m, , 42. It is claimed that two cesium clocks, if, , allowed to run for 100 yrs without any, disturbance may differ by only about, 0.02 s. Then the accuracy of the clock, in measuring a time interval of, 1 s is ……… ., , 15, (a) 10-10, (c) 10-5, , (b) 10-11, (d) 10-8, , 43. Photon is quantum of radiation with, energy E = hn, where n is frequency, and h is Planck’s constant. The, dimensions of h are the same as that, of ……… ., (a), (b), (c), (d), , linear impulse, angular impulse, linear momentum, energy, , 44. Which amongst the following statement, is incorrect regarding mass?, , (a) Its SI unit is kilogram., (b) It does not depend on the location of the, object in space., (c) It is the basic property of matter., (d) While dealing with atoms, kilogram is a, convenient unit for measuring mass., , 45. Choose the incorrect statement out of, the following., , (a) Every measurement by any measuring, instrument has some errors., (b) Every calculated physical quantity that is, based on measured values has some errors., (c) A measurement can have more accuracy, but less precision and vice-versa., (d) The percentage error is different from, relative error., , 46. Given that T stands for time period and, l stands for the length of simple, pendulum. If g is the acceleration due, to gravity, then which of the following, statements about the relation T 2 = l / g, is correct?, (a) It is correct both dimensionally as well as, numerically., (b) It is neither dimensionally correct nor, numerically., (c) It is dimensionally correct but not, numerically., (d) It is numerically correct but not, dimensionally.
Page 27 :
16, , CBSE New Pattern ~ Physics 11th (Term-I), , Codes, , 47. Match the following columns., Column I, , A, , B, , C, , D, , Column II, , (a) s, , r, , p, , q, p, , A., , Capacitance, , p., , volt (ampere)-1, , (b) r, , q, , s, , B., , Magnetic, induction, , q., , volt-sec, (ampere)-1, , (c) r, , p, , s, , q, , (d) r, , s, , p, , q, , C., , Inductance, , r., , newton, (ampere)-1, (metre)-1, , D., , Resistance, , s., , coulomb 2(joule)-1, , Assertion-Reasoning MCQs, , Codes, A, , B, , C, , D, , (a) q, , r, , s, , p, , (b) s, , r, , q, , p, , (c) r, , s, , p, , q, , (d) s, , p, , q, , r, , 48. Match the Column I (unit) with, , Column II (value) and select the correct, option from the codes given below., Column I, , For question numbers 50 to 59, two, statements are given-one labelled, Assertion (A) and the other labelled, Reason (R). Select the correct answer to, these questions from the codes (a), (b), (c), and (d) are as given below, (a) Both A and R are true and R is the, correct explanation of A., (b) Both A and R are true but R is not the, correct explanation of A., (c) A is true but R is false., (d) A is false and R is also false., , 50. Assertion Unit chosen for measuring, , physical quantities should not be easily, reproducible., , Column II, , A., , 1 are, , p., , 200 mg, , B., , 1 bar, , q. 1013, ., ´ 10 5 Pa, , C., , 1 carat, , r., , 10 2 m 2, , Reason Unit should change with the, changing physical conditions like, temperature, pressure, etc., , 51. Assertion The unit used for measuring, , Codes, A, , B, , C, , A, , B, , C, , nuclear cross-section is ‘barn’., , (a) q, , p, , r, , (b) r, , r, , p, , (c) r, , q, , p, , (d) r, , p, , q, , Reason 1 barn = 10 - 14 m 2 ., , 52. Assertion When we change the unit of, , 49. Names of units of some physical, , quantities are given in Column I and, their dimensional formulae are given in, Column II and select the correct option, from the codes given below., Column I, A., B., , Nm-K, , p., -1, , -1, , C., , J kg K, , D., , -1, , q., -1, , Wb m K, , -1, , measuring distances of nearby stars only., , [L2T -2K -1 ], -2, , -1, , -1, , [MLT A K ], -1, , -1, , r., , [ML T ], , s., , [ML2T -2K -1 ], , Reason Smaller the unit of measurement, smaller is its numerical value., , 53. Assertion Parallax method is used for, , Column II, , Pa-s, , measurement of a quantity, its, numerical value changes., , Reason With increase in the distance, of star from earth, the parallactic angle, becomes too small to be measured, accurately.
Page 28 :
CBSE New Pattern ~ Physics 11th (Term-I), , 54. Assertion Out of two measurements, , 17, , Case Based MCQs, , l = 0.7 m and l = 0.70 m, the second one, is more accurate., , Direction Answer the questions from, 60-64 on the following case., , Reason In every measurement, the last, digit is not accurately know., , Measurement of Physical Quantity, All engineering phenomena deal with definite, and measured quantities and so depend on, the making of the measurement. We must be, clear and precise in making these, measurements. To make a measurement,, magnitude of the physical quantity (unknown), is compared., The record of a measurement consists of, three parts, i.e. the dimension of the quantity,, the unit which represents a standard quantity, and a number which is the ratio of the, measured quantity to the standard quantity., , 55. Assertion Random errors arise due to, , random and unpredictable fluctuations, in experimental conditions., Reason Random errors occurred due, to irregularly with respect to sign and, size., , 56. Assertion When a quantity appears, , with a power n greater than one in an, expression, its error contribution to the, final result decreases n times., , Reason In all mathematical, operations, the errors are not additive, in nature., , 57. Assertion Special functions such as, trigonometric, logarithmic and, exponential functions are not, dimensionless., , Reason A pure number, ratio of, similar physical quantities, such as, angle and refractive index, has some, dimensions., , 58. Assertion Specific gravity of a fluid is a, dimensionless quantity., Reason It is the ratio of density of fluid, to the density of water., , 59. Assertion The method of dimensions, , analysis cannot validate the exact, relationship between physical quantities, in any equation., Reason It does not distinguish, between the physical quantities having, same dimensions., , 60. A device which is used for, , measurement of length to an accuracy, of about 10 -5 m, is, (a) screw gauge, (c) vernier callipers, , (b) spherometer, (d) Either (a) or (b), , 61. Which of the technique is not used for, measuring time intervals?, (a), (b), (c), (d), , Electrical oscillator, Atomic clock, Spring oscillator, Decay of elementary particles, , 62. The mean length of an object is 5 cm., , Which of the following measurements is, most accurate?, (a) 4.9 cm, (c) 5.25 cm, , (b) 4.805 cm, (d) 5.4 cm, , 63. If the length of rectangle l = 10.5 cm,, breadth b = 21, . cm and minimum, possible measurement by scale = 01, . cm,, then the area is, (a) 22.0 cm 2, (c) 22.5 cm 2, , (b) 21.0 cm 2, (d) 21.5 cm 2
Page 29 :
18, , CBSE New Pattern ~ Physics 11th (Term-I), , 64. Age of the universe is about 10 10 yr,, , whereas the mankind has existed for, 10 6 yr. For how many seconds would, the man have existed, if age of universe, were 1 day?, (a) 9.2 s, (c) 8.6 s, , Significant Digits, Normally, the reported result of measurement, is a number that includes all digits in the, number that are known reliably plus first digit, that is uncertain. The digits that are known, reliably plus the first uncertain digit are, known as significant digits or significant, figures., e.g. When a measured distance is reported to, be 374.5 m, it has four significant figures 3, 7,, 4 and 5. The figures 3, 7, 4 are certain and, reliable, while the digit 5 is uncertain. Clearly,, the digits beyond the significant digits, reported in any result are superfluous., , 65. In 4700 m, significant digits are, (b) 3, , (c) 4, , (d) 5, , 66. To determine the number of significant, figures, scientific notation is, (a) a b, (c) a ´ 102, , (b) a ´ 10b, (d) a ´ 104, , 67. 5.74 g of a substance occupies 1.2 cm 3 ., Express its density by keeping the, significant figures in view., (a) 4.9 g cm-3, (c) 4.8 g cm-3, , 69. Consider the following rules of, significant figures., , (b) 10.2 s, (d) 10.5 s, , Direction Answer the questions from, 65-69 on the following case., , (a) 2, , (c) 4700 m = 4.700 ´ 103 m, here there is change, in numbers of significant numbers., (d) Change in unit changes the number of, significant figure., , (b) 5.2 g cm-3, (d) 4.4 g cm-3, , 68. Choose the correct option., (a) Change in unit does not change the, significant figure., (b) 4.700 m= 4700 mm, here there is a change, of significant number from 4 to 2 due to, change in unit., , I. All the non-zero digits are significant., II. All the zeroes between two non-zero, digits are significant., III. The terminal or trailing zero(s) in a, number without a decimal point are, significant., Which of the above statement(s) is/are, correct?, (a) I and II, (c) I and III, , (b) II and III, (d) All of these, , Direction Answer the questions from, 70-74 on the following case., Combination of Errors, Maximum absolute error in the sum or, difference of two quantities is equal to sum of, the absolute error in the individual quantities,, i.e. Z = A + B , then ± DZ = ± DA ± DB, Maximum fractional error in a product or, division of quantities is equal to the sum of, fractional errors in the individual quantities, A, DZ, DA DB, i.e. AB or , then, =±, +, B, Z, A, B, Two resistors of resistances R1 = 100 ± 3 W, and R 2 = 200 ± 4W are connected (a ) in series, and ( b) in parallel., , 70. The percentage error in the value of R1, is, , (a) 3%, , (b) 4%, , (c) 6%, , (d) 0.3%, , 71. The fractional error in the value of R2 is, 1, 40, 1, (c), 100, (a), , 1, 50, 1, (d), 200, (b)
Page 30 :
CBSE New Pattern ~ Physics 11th (Term-I), , 72. Find the equivalent resistance of the, series combination., (a), (b), (c), (d), , (250 ± 7) W, (320 ± 6) W, (300 ± 7) W, (300 ± 1) W, , 73. The percentage error in equivalent, resistance in series combination is, (a) 2%, (c) 2.5%, , (b) 2.3%, (d) 3%, , 74. Find the equivalent resistance of the, , parallel combination having error of, 1.8 W., , (a) (66 ± 1) W, (c) (66.3 ± 2) W, , (b) (66.7 ± 1.8) W, (d) (67 ± 3) W, , Direction Answer the questions from, 75-79 on the following case., Dimensional analysis and its applications, The expression which shows how and which, of the base quantities represent the, dimensions of a physical quantity is called the, dimensional formula of the given physical, quantity. The recognition of concepts of, dimensions, which guide the description of, physical behaviour is of basic importance as, only those physical quantities can be added or, subtracted which have the same dimensions., A thorough understanding of dimensional, analysis helps us in deducing certain relations, among different physical quantities and, checking the derivation, accuracy and, dimensional consistency or homogeneity of, various mathematical expressions. When, magnitudes of two or more physical quantities, are multiplied, their units should be treated in, the same manner as ordinary algebraic, symbols. We can cancel identical units in the, numerator and denominator. The same is true, for dimensions of a physical quantity., Similarly, physical quantities represented by, symbols on both sides of a mathematical, equation must have the same dimensions., , 19, 75. Statement I The method of, , dimensions analysis cannot validate the, exact relationship between physical, quantities in any equation., Statement II It does not distinguish, between the physical quantities having, same dimensions., Which of the following statement(s), is/are correct?, (a) Only I, (c) Only II, , (b) I and II, (d) None of these, , 76. The quantity having same dimension, as that of Planck’s constant is, (a) work, (b) linear momentum, (c) angular momentum (d) impulse, , 77. If speed v, acceleration A and force F ,, , are considered as fundamental units,, the dimension of Young’s modulus will, be, , (a) [v -4A - 2F], (c) [v -2 A 2 F - 2], , (b) [v -2 A 2 F 2], (d) [v -4A 2 F 1], , 78. Given that, the amplitude of the, scattered light is, (i) directly proportional to amplitude of, incident light, (ii) directly proportional to the volume, of the scattering dust particle, (iii) inversely proportional to its distance, from the scattering particle and, (iv) dependent upon the wavelength l of, the light., Then, the relation of intensity of, scattered light with the wavelength is, (a), , 1, l2, , (b), , 1, l4, , (c), , 1, l6, , (d), , 1, l7, , 79. Find the value of power of 60 J/min on, a system that has 100 g, 100 cm and 1, min as the base units., (a) 2.16 ´ 104 units, (c) 3 ´ 104 units, , (b) 2.16 ´ 106 units, (d) 4 ´ 107 units
Page 31 :
020, , CBSE New Pattern ~ Physics 11th (Term-I), , ANSWERS, Multiple Choice Questions, 1. (b), 11. (b), 21. (a), , 2. (c), 12. (a), 22. (c), , 3. (c), 13. (a), 23. (a), , 4. (a), 14. (b), 24. (b), , 5. (d), 15. (a), 25. (d), , 6. (c), 16. (b), 26. (c), , 7. (b), 17. (c), 27. (a), , 8. (a), 18. (a), 28. (c), , 9. (a), 19. (d), 29. (a), , 10. (b), 20. (a), 30. (a), , 31. (a), 41. (a), , 32. (d), 42. (b), , 33. (a), 43. (b), , 34. (d), 44. (d), , 35. (a), 45. (d), , 36. (b), 46. (c), , 37. (a), 47. (b), , 38. (b), 48. (c), , 39. (b), 49. (d), , 40. (b), , 52. (c), , 53. (a), , 54. (b), , 55. (b), , 56. (d), , 57. (d), , 58. (a), , 59. (a), , 62. (a), 72. (c), , 63. (a), 73. (b), , 64. (c), 74. (b), , 65. (a), 75. (b), , 66. (b), 76. (c), , 67. (c), 77. (d), , 68. (a), 78. (b), , 69. (a), 79. (b), , Assertion-Reasoning MCQs, 50. (d), , 51. (c), , Case Based MCQs, 60. (d), 70. (a), , 61. (c), 71. (b), , SOLUTIONS, 1. Time is the quantity which has same unit in, all systems of unit, i.e. second. Other three, quantities, i.e. mass, length and temperature, have different units in different system of, units., , 2. The coefficient of thermal conductivity is, given by, K =, , L dQ, ADT dt, , where, L = length of conductor, A = area of, conductor, DT = change in temperature, dQ, and, = rate of flow of heat., dt, metre, ´ watt, \ Unit of K =, (metre) 2 ´ (kelvin), = Wm-1K -1, , 3. Given, damping force µ velocity, F µ v Þ F = kv Þ, Unit of k =, , k =, , F, v, , Unit of F, kg - ms -2, =, = kg s -1, Unit of v, ms -1, , 4. To convert a measured value from one, system to another system, we use, N 1u1 = N 2u2, where, N is numeric value and u is unit., , We get,, \, , 128 ×, , kg, 50 g, =N2, m3, (25 cm) 3, , mass ù, é, êëQ density = volume úû, 128 ´ 1000 g, N 2 ´ 50 g, Þ, =, 100 ´ 100 ´ 100 cm3 25 ´ 25 ´ 25 cm3, 128 ´ 1000 ´ 25 ´ 25 ´ 25, N2 =, = 40, Þ, 50 ´ 100 ´ 100 ´ 100, , 5. Given, work done,, W = 10 10 g-cm 2 s -2, which is in CGS system of units., g, In SI unit,W = 10 10 2 cm2, s, (10 -3 kg)(10 -4 m2 ), = 10 10, 1s 2, = 10 3 kg-m2 s -2, , 6. We know that, 1 light year = 9.46 ´ 10 11 m, = distance that light travels in 1 year with, speed 3 ´ 10 8 m/s., 1 parsec = 3.08 ´ 10 16 m, = Distance at which average radius of earth’s, orbit subtends an angle of 1 parsecond., Here, year represent time.
Page 32 :
CBSE New Pattern ~ Physics 11th (Term-I), , 7. We have, q = 1° 54 ¢ = (60 + 54)¢, = 114 ¢ = (114 ´ 60) ¢ ¢, Since, 1¢¢ = 4.85 ´ 10 - 6 rad, = (114 ´ 60 ) ¢¢ ´ (4.85 ´ 10 - 6 ) rad, = 3.33 ´ 10 - 2 rad, Also, diameter of earth, b = 1. 276 ´ 10 7 m, Hence, the earth-moon distance is given as, 1.276 ´ 10 7, D = b /q =, = 3.83 ´ 10 8 m, 3.33 ´ 10 -2, , 8. We know that, radius of atom, ra = 10, Radius of nucleus, rn = 10, \, , -15, , m, , Ratio,, , -10, , m, , ra 10 -10, =, = 10 5, rn 10 -15, , 4, 3, 3, pra, ær ö, 3, Ratio of volume =, =ç a÷, 4, 3, è rn ø, prn, 3, = (10 5 ) 3 = 10 15, , 9. All given measurements are correct upto two, decimal places. As here 5.00 mm has the, smallest unit and the error in 5.00 mm is, least (commonly taken as 0.01 mm if not, specified), hence 5.00 mm is most precise., , 21, Mean deviation of a simple pendulum, S | x - xi | 2 + 1 + 3 + 0, =, =, = 1.5, N, 4, Given, minimum division in the measuring, clock, i.e. simple pendulum = 1 s. Thus, the, reported mean time of a oscillating simple, pendulum = ( 92 ± 2) s., , 13. The mean period of oscillation of the, pendulum,, 2 . 63 + 2.56 + 2.42 + 2.71 + 2.80, 5, 13.12, =, = 2.624 = 2.62 s, 5, The absolute errors in the measurements are, DT 1 = 2.63 s - 2.62 s = 0.01 s, DT 2 = 2.56 s - 2.62 s = - 0.06 s, DT 3 = 2.42 s - 2.62 s = - 0.20 s, DT 4 = 2.71 s - 2.62 s = 0.09 s, DT 5 = 2.80 s - 2.62 s = 0.18 s, The arithmetic mean of all the absolute errors, is, 5, | DT i |, DT mean = å, 5, i =1, T mean =, , = [(0.01 + 0.06 + 0.20 + 0.09 + 0.18)] / 5, = 0.54 / 5 = 0.108 ~, - 0.11 s, , 10. If student measure 3.50 cm, it means that, there in an uncertainly of order 0.01 cm., For vernier scale with 1 MSD, = 1mm and 9 MSD = 10 VSD, \ LC of VC = 1 MSD - 1 VSD, 1 æ, 9ö, 1, =, cm, ç1 - ÷ =, 10 è 10 ø 100, , 11. Volume of block, V = lbh, The percentage error in the volume is given by, DV, Db Dh ö, æ Dl, ´ 100 = ç, +, +, ÷ ´ 100, è l, V, b, h ø, æ 0.02 0.01 0.01 ö, =ç, +, +, ÷ ´ 100, è1213, 816, 3.46 ø, ., ., æ 200 100 100 ö, =ç, +, +, ÷, è1213 816 346 ø, = 01649, ., + 01225, ., + 0.2890, = 0.58% (rounded off to two significant figures), , 12. Arithmetic mean time of a oscillating simple, S xi, 90 + 91 + 92 + 95, pendulum =, =, = 92 s, N, 4, , 14. As we know, time period of oscillation is T, = 2p, , L, g, , So,, g = 4 p 2L /T 2, Therefore, relative error in g is, ( Dg / g ) = ( DL /L ) + 2 ( DT /T ), Given, DL = 1 mm = 01, . cm, L = 20 cm,, DT = 1 s and T = 90 s, 0.1 æ 1 ö, Dg, Þ, =, +2 ç ÷ = 0.027, è 90 ø, 20, g, Thus, the percentage error in g is, Dg, =, ´ 100%, g, = 0.027 ´ 100% = 27, . %~, - 3%, , 15. Mean of the five observations,, m=, , 80.0 + 80.5 + 81.0 + 81.5 + 82, 5
Page 33 :
22, , CBSE New Pattern ~ Physics 11th (Term-I), 405.0, = 81, 5, \ Mean error, é| 80 - m | + | 80.5 - m | + | 81.0 - m |, ù, ê, ú, +, |, 81, ., 5, m, |, +, |, 82, m, |, û, =ë, 5, é| 80 - 81 | + | 80.5 - 81 | + | 81.0 - 81 |, ù, ê, ú, +, +, |, 81, ., 5, 81, |, |, 82, 81, |, û, =ë, 5, 1 + 0.5 + 0 + 0.5 + 1 3, =, = = 0.6, 5, 5, 0.6, \ Mean % error =, ´ 100% = 0.74%, 81, =, , 16. The error in the measurement of mass 1.02 g, is ± 0.01 g,, whereas that of another measurement 9.89 g, is also ± 0.01 g., \ The relative error in 1.02 g, = [ ± 0.01 /1.02) ] ´ 100% = ± 0.98% @ ± 1%, Similarly, the relative error in 9.89 g, = [ ± 0.01 / 9.89] ´ 100% = ± 0.1%, The relative errors in measurement of two, masses are ± 1% and ± 0.1%., Mass, M, M, = 3 or r = 3, Volume L, L, Dr DM, 3DL, Þ Error in density, =, +, r, M, L, , 17. Q Density, r =, , So, maximum % error in measurement of r is, Dr, DM, 3DL, ´ 100 =, ´ 100 +, ´ 100, r, M, L, or % error in density = 1.5 + 3 ´ 1, % error = 4.5%, 1, 18. Kinetic energy, K = mv 2, 2, DK, Dm, 2Dv, \, ´ 100 =, ´ 100 +, ´ 100, K, m, v, = 2% + 2 ´ 3% = 8%, l, 19. Time period, T = 2p, g, DT 1 Dl, =, T, 2 l, , or, For 1 s ,, DT =, , 1 æ Dl ö, 1, ç ÷ T = ´ 0.02 ´ T = 0.01 T, è, ø, 2 l, 2, , For a day, the pendulum loses,, DT = 24 ´ 60 ´ 60 ´ 0.01 = 864 s, , 20. Given, length, l = (16.2 ± 0.1) cm, Breadth, b = (10.1 ± 0.1) cm, Area, A = l ´ b, = (16.2 cm) ´ (10.1 cm) = 163.62 cm2, Rounding off to three significant digits,, area, A = 164 cm2, D A Dl Db 0.1 0.1, =, +, =, +, A, l, b 16.2 10.1, 1.01 + 1.62 2.63, =, =, 16.2 ´ 10.1 163.62, 2.63, 2.63, Þ, DA = A ´, = 163.62 ´, 163.62, 163.62, = 2.63 cm2 » 3 cm2, (By rounding off to one significant figure), \ Area, A = A ± DA = (164 ± 3) cm2, a 3b 2 Da, 21. Given, P =, ,, ´100% = 1%,, a, cd, Db, Dc, ´ 100% = 2%,, ´ 100% = 3%, b, c, Dd, and, ´ 100% = 4%, d, ö, æ DP, ´ 100 ÷ %, \ % error in P = ç, ø, è P, æ 3Da 2Db Dc Dd ö, =ç, +, +, +, ÷ ´ 100%, è a, b, c, d ø, Db, ö, æ Da, ´ 100% + 2, ´ 100%, ÷, ç3, a, b, ÷, =ç, Dc, Dd, ÷, ç, ç, +, ´ 100% +, ´ 100% ÷, ø, è, c, d, = 3 ´ 1% + 2 ´ 2% + 3% + 4% = 14%, a 2b 2 / 3, 22. Given, z =, c d3, According to question,, æ 2ö, % error in z = ( 2)% error in a + ç ÷ % error in, è 3ø, 1, æ ö, b, + ç ÷ % error in c + ( 3)% error in d, è 2ø, Dz, Da 2 Db 1 Dc, Dd, =2, +, +, +3, z, a, d, 3 b, 2 c, 2, 1, = 2 ´ 2 % + ´ 1.5 % + ´ 4 % + 3 ´ 2.5 %, 3, 2, = 14.5%
Page 34 :
CBSE New Pattern ~ Physics 11th (Term-I), , 23. The reliable digit plus the first uncertain digit, is known as significant figures., For the number 23.023, all the non-zero, digits are significant, hence 5., For the number 0.0003, number is less than, 1, the zero(s) on the right of decimal point, but to the left of the first non-zero digit are, not significant, hence 1., For the number 2.1 ´ 10 -3 , significant figures, are 2., , 24. By adding 3.8 ´ 10 -6 and 42 ´ 10 -6 , we get, = 45.8 ´ 10 -6 = 4.58 ´ 10 -5, As least number of decimal figures in given, values is 1, so we round off the result to, 4.6 ´ 10 -5 ., , 25. The number 5.355 rounded off to three, significant figures becomes 5.36, since, preceding digit of 5 is odd, hence it is raised, by 1., On other hand, the number 5.345 rounded off, to three significant figures becomes 5.34., Since, the preceding digit of 5 is even., , 26. In this question, density should be reported, to two significant figures., 4.237g, Density =, = 1.6948, 2.5 cm3, As rounding off the number, we get density, = 17, . g cm -3, , 27. Dimensions of torque,, t=F ´r, = [MLT-2 ] [L], = [ML2 T-2 ], , 28. Dimensions of given quantities are, l = inductance = [M 1 L2 T-2 A -2 ], r = resistance = [M 1 L2 T-3 A -2 ], c = capacitance = [M - 1 L- 2 T4 A 2 ], v = voltage = [M 1 L2 T-3 A -1 ], l, are, So, dimensions of, rcv, 2, -2, -2, é l ù [M L T A ], -1, =, 1, 2, 2, êë rcv úû [M L T A -1 ] = [A ], , 29. According to ideal gas equation, i.e. pV = nRT ,, where n is the number of moles of gases., , 23, , \ Universal gas constant, R =, , ( p ) (V ), (n )(T ), , Dimensional formula of R =, , [ML-1 T -2 ] [L3 ], [mol] [K], , = [ML2 T -2 mol -1K-1 ], [ML2 T-2 ], Energy, 30. I. Energy density =, =, [L3 ], Volume, = [ML-1 T-2 ], II. Refractive index has no dimensions., III. Dielectric constant has no dimensions., IV. Young’s modulus,, F l [MLT-2 ][L], Y =, = [ML-1 T-2 ], =, [L]2[L], ADl, V. Magnetic field,, [MLT-2 ], F, B=, = [MT-2 A -1 ], =, [A][L], I l, , 31. In this question, it is given that P , Q and R, are having different dimensions, hence they, cannot be added or subtracted, so we can say, that (a) is not meaningful. We cannot say, about the dimension of product of these, quantities, hence (b), (c) and (d) may be, meaningful., A x, 32. Given, U =, x+B, Dimensions of U = Dimensions of potential, energy = [ML2 T -2 ], According to the principle of homogeneity,, Dimensions of B = Dimensions of x = [M 0 LT 0 ], \ Dimensions of A, Dimensions of U ´ Dimensions of ( x + B ), =, Dimensions of x, =, , [ML2 T-2 ] [M 0 LT0 ], = [ML5/ 2 T-2 ], [M 0 L1/ 2 T0 ], , Hence, dimensions of AB, = [ML5/ 2 T-2 ] [M 0 LT0 ], = [ML7/ 2 T-2 ], , 33. According to question, [ X ] = Dimensions of, capacitance = [M -1L-2 T2 Q 2 ], and [Z ] = Dimensions of magnetic induction., = [MT -1Q -1 ]
Page 35 :
24, , CBSE New Pattern ~ Physics 11th (Term-I), , Given,, \, Þ, , X = 3YZ 2 ,, [X ], [Y ] = 2, [Z ], [Y ] =, , [M -1L-2 T2Q 2 ], = [M -3 L-2 T4 Q 4 ], [M 2 T -2Q -2 ], , b, 34. Given, v = at +, t +c, Since, LHS is equal to velocity, so at and, b, must have the dimensions of velocity., t +c, [LT-1 ], v, \ at = v or a = Þ [a ] =, = [LT-2 ], [T], t, Now, c = time, \, c =t, [c ] = [ T], b, Now,, =v, t +c, \, , (Q quantities are added), , b = v ´ time, [b ] = [LT-1 ][T] = [L], , 35. The dimensions of LHS of each relation is, [L],therefore the dimensions of RHS should, be [L] as per the principle of homogeneity, and the argument of the trigonometrical, function, i. e. angle should be dimensionless., 2pt, (a) As, is dimensionless, therefore, T, dimensions of RHS = [L]. Thus, this, formula is correct., (b) Dimensions of RHS, = [L]sin [LT-1 ] [T] = [L] sin [L], As angle is not dimensionless here,, therefore, this formula is incorrect., (c) Dimensions of RHS, [L], [T], =, = [LT-1 ] sin [TL-1 ], sin, [T], [L], As angle is not dimensionless here,, therefore this formula is incorrect., \ Thus, the correct formula on the, dimensional ground is option (a)., , 36. Let Young’s modulus is related to speed, area, and force, as Y = F A V, Substituting dimensions, we have, [ML-1 T-2 ] = [MLT-2 ]x [L2 ] y [LT-1 ]z, x, , y, , z, , Comparing power of similar quantities, we, have, x = 1, x + 2y + z = - 1 and -2x - z = - 2, Solving these, we get x = 1, y = -1, z = 0, So,, [Y] = [ FA -1 V 0 ], , 37. Given, critical velocity of liquid flowing, through tube is expressed as , v c µ h x r y r z, Coefficient of viscosity of liquid,, h = [ ML-1 T-1 ], Density of liquid, r = [ML-3 ], Radius of a tube, r = [L ], Critical velocity of liquid, v c = [ M 0 LT-1 ], Þ, , [M 0 LT-1 ] = [ML-1 T-1 ] x [ML-3 ]y [L]z, , [ M 0 LT-1 ] = [ M x + yL- x - 3 y + z T- x ], Comparing powers of M, L and T, we get, x + y = 0, - x - 3y + z = 1, - x = - 1, On solving above equations, we get, x = 1, y = - 1, z = -1, Mass, 38. Q Density =, Volume, [M], \ Dimensions of density = 3 = [ML-3 ], [L], Given, n1 = 10, M 1 = 1 g, L 1 = 1 cm,, In new system, n2 = ?, M 2 = 100 g, L 2 = 10 cm, So, conversion of 10g cm -3 (n1 ) into new system, é M ù éL ù, n2 = n1 ´ ê 1 ú ê 1 ú, ë M2 û ë L 2 û, , -3, , -3, , æ 1 öæ1ö, = 10 ´ ç, ÷ç ÷, è100 ø è10 ø, 1, = 10 ´, ´ 10 ´ 10 ´ 10, 100, = 100 units, , 39. As, dimensional formula of force = [ MLT -2 ], n1 = 36, M 1 = 1 kg, L 1 = 1 m, T 1 = 1 min = 60 s, n2 = ?, M 2 = 1 g, L 2 = 1 cm, T 2 = 1 s, So, conversion of 36 units into CGS system,, éM ù, i. e. n2 = n1 ê 1 ú, ë M2 û, , a, , é L1 ù, êL ú, ë 2û, 1, , b, , é T1 ù, êT ú, ë 2û, 1, , c, , -2, , é1 kg ù é 1 m ù é1 min ù, n2 = n1 ê, ú ê, ú ê, ú, ë 1g û ë1 cm û ë 1 s û, 1, -2, é1000 g ù é100 cm ù é 60 s ù, 3, = 36 ê, úê, ú = 10 dyne, ú ê, ë 1 g û ë 1 cm û ë 1 s û, Þ
Page 36 :
CBSE New Pattern ~ Physics 11th (Term-I), , 40. Solid angle, d W =, , dA, 1 cm2, =, r 2 ( 5 cm) 2, , = 0.04 steradian, = 4 ´ 10 -2 steradian, , 41. Given, A = 2.5 ms -1 ± 0.5 ms -1,, B = 0.10 s ± 0. 01 s, x = AB = ( 2.5)( 010, . ) = 0.25 m, Dx DA DB, =, +, x, A, B, 0.5 0.01 0.05 + 0.025 0.075, =, +, =, =, 2.5 010, ., 0.25, 0.25, Dx = 0.075 = 0.08 m, rounding off to two, significant figures., AB = ( 0. 25 ± 0.08 ) m, 42. 100 years in seconds = 100 ´ 365 ´ 24, ´ 60 ´ 60 s. Error that may occur in the clock, after these many seconds is 0.02 s, 0.02 s, \ Error in 1 s =, 100 ´ 365 ´ 24 ´ 60 ´ 60, = 10 -11 (approx.), , 43. We know that, energy of radiation, E = hn, [h ] =, , [ E ] [ML2 T-2 ], =, = [ML2 T-1 ], [T-1 ], [n ], , Dimensions of linear impulse = Dimension of, momentum = [MLT-1 ], As we know that, linear impulse J = DP, Þ Angular impulse = tdt = DL, = change in angular momentum, Hence, dimensions of angular impulse, = dimension of angular momentum, = [ML2 T-1 ], This is similar to the dimensions of Planck’s, constant h., Dimensions of energy is [ML2 T-2 ] ., , 44. Statement given in option (d) is incorrect and, it can be corrected as, While dealing with atoms, kilogram is an, inconvenient unit. In this case, there is an, important standard unit of mass called, unified atomic mass unit (u), which has been, established for expressing the mass of atom., Rest statements are correct., , 25, 45. When the relative error is expressed in, percentage, we call it as percentage error., Thus, statement (d) is incorrect while all, other statements regarding measurement of a, quantity are correct., , 46. The correct relation for time period of simple, pendulum is T = 2p( l/ g ) 1 / 2 ., So, the given relation is numerically incorrect, as the factor of 2p is missing., But, it is dimensionally correct., , 47. Capacitance,, C =, , Q, V, , =, , Q, , 2, , W, , = (coulomb) 2 joule-1, , Magnetic induction,, newton, F, B=, =, il ampere ´ metre, = (newton) (ampere) -1(metre) -1, e, volt, Inductance, L =, =, dl / dt ampere /second, = volt -second (ampere) -1, volt, V, Resistance, R = =, I ampere, = volt (ampere) -1, Hence, A ® s, B ® r, C ® q and D ® p., , 48. Are is also unit of area, 1 are = 10 2 m 2, Atmospheric pressure is measured in SI unit, of bar., 1 bar = 1.013 ´ 10 5 N/m 2 = 1.013 ´ 10 5 Pa, Carat is the unit of mass., i.e., 1 carat = 200 mg, Hence, A ® r, B ® q and C ® p., , 49. Dimensions of Pa-s is [ML-1 T-2 ] × [T], = [ML-1T -1 ], Dimensions of Nm K -1 is, [MLT-2 ][L][K -1 ] = [ML2 T-2K -1 ], Dimensions of J-kg -1 K -1 is, [ML2 T-2 ][M -1 ][K -1 ] = [L2 T-2K -1 ], Dimensions of Wbm -1 K -1 is, [ML2 T-2 A -1 ][L-1 ][K -1 ] = [MLT-2 A -1K -1 ], Here, W is for weber, unit of magnetic flux, BA with dimensions
Page 37 :
26, , CBSE New Pattern ~ Physics 11th (Term-I), , [MLT-2 ], [L2 ] = ML2 T-2 A -1, [AT] [LT-1 ], Hence, A ® r, B ® s, C ® p and D ® q., , 50. The unit chosen for measuring any physical, quantity, should be easily reproducible, i.e., replicas of the unit should be available easily., Also, unit should not change with changing, physical conditions like temperature,, pressure, etc., Therefore, A is false and R is also false., , 51. Barn is used in nuclear physics for measuring, the cross-sectional area of nuclei., One barn is equal to 10 -28 m2 ., Therefore, A is true but R false., , 52. Changing the unit of the measurement, the, numerical value of the quantity also changes., For example, let the length of scale be 1 m., Its value in CGS unit is 100 cm., Therefore, the numerical value changes., Also, we can say that from the above, example smaller the unit of measurement,, greater is its numerical value., Therefore, A is true but R is false., , 53. Parallax method is used for measuring, distances of nearby stars only., If D is a distance of a far away star from, b, Earth, then, D=, q, where, q is called parallactic angle and b is, the distance between the two different, positions on Earth from where the star is, being observed., \ With increase in the distance of star,, parallactic angle becomes too small to be, measured accurately., Therefore, both A and R are true and R is, the correct explanation of A., , 54. Accuracy of the measurement is the measure, of how close the measured value is to the, true value., So, the greater the significant figures in the, digit, greater will be its accuracy., , Since, 0.70 m has more significant figure, (i.e., 2) as compare to 0.7 m (i.e., 1). So, it, will be more accurate., Also, in general, the last digit is not, accurately known in every measurement., Therefore, both A and R are true but R is, not the correct explanation of A., , 55. Random errors are those errors, which occur, irregularly and hence are random with, respect to sign and size., These can arise due to random and, unpredictable fluctuations in experimental, conditions, personal (unbiased) errors by the, observer taking readings, etc., Therefore, both A and R are true but R is, not the correct explanation of A., , 56. In all mathematical operations, the errors are, of additive nature., When a quantity appears with a power n, greater than one in an expression, its error, contribution to the final result increases n, times., So, quantities with higher power in the, expression should be measured with, maximum accuracy., Therefore, A is false and R is also false., , 57. The arguments of special functions, such as, the trigonometric, logarithmic and, exponential functions must be dimensionless., A pure number, ratio of similar physical, quantities, such as angle as the ratio, (length/length), refractive index as the ratio, of (speed of light in vacuum/speed of light in, medium), etc. has no dimensions., Therefore, A is false and R is also false., Density of fluid, 58. Relative density of a fluid =, Density of water, As the density of any substance has same, units, hence relative density is dimensionless., Therefore, both A and R are true and R is, the correct explanation of A., , 59. The method of dimensions can only test the, dimensional validity but not the exact, relationship between physical quantities in, any equation.
Page 38 :
CBSE New Pattern ~ Physics 11th (Term-I), , This is because it does not distinguish, between the physical quantities having same, dimensions., Therefore, both A and R are true and R is, the correct explanation of A., 60. A screw gauge and a spherometer can be, used to measure length accurately as less as, 10 - 5 m., , 61. Spring oscillator cannot be used to measure, time intervals., , 62. Given, length, l = 5 cm, Now, checking the errors with each options, one-by-one, we get, D l 1 = 5 - 4.9 = 0.1 cm, D l 2 = 5 - 4.805 = 0.195 cm, D l 3 = 5 . 25 - 5 = 0 . 25 cm, D l 4 = 5 . 4 - 5 = 0 . 4 cm, Error D l 1 is least., Hence, 4.9 cm is most precise or accurate., , 63. Area of rectangle, A = Length ´ Breadth, So, A = lb = 10.5 ´ 21, . = 22.05 cm 2, Minimum possible measurement of, scale = 01, . cm., So, area measured by scale = 22.0 cm 2 ., Age of mankind, 64. Magnification in time =, Age of universe, 10 6, = 10 - 4, 10 10, Apparent age of mankind = 10 - 4 ´ 1 day, =, , = 10 - 4 ´ 86400 s, = 8.64 s » 8.6 s, 65. As, we know that, the terminal or trailing, zero(s) in a number without a decimal point, are not significant. So, 4700 m has two, significant figures., 66. Every number is expressed as a ´ 10 b , where a, is a number between 1 & 10 and b is any, positive or negative exponent (or power) of 10., 67. There are 3 significant figures in the, measured mass whereas there are only 2, significant figures in the measured volume., Hence, the density should be expressed to, only 2 significant figures., 5.74, Density =, = 4.8 g cm-3, 1. 2, , 27, 68. There is no change in number of significant, figures on changing the units. For it, the, convention is that we write,, 4700 m = 4.700 ´ 10 3 m, This convention ensures no change in, number of significant numbers., , 69. Following rules of significant figures are, I. All the non-zero digits are significant., II. All the zeroes between two non-zero, digits are significant, no matter where the, decimal point is, if at all., III. The terminal or trailing zero(s) in a, number without a decimal point are not, significant. Thus, 123 m = 12300 cm, = 123000 mm has three significant figures,, the trailing zero(s) being not significant., , 70. Given, R1 = (100 ± 3) W, \, , 3, DR1, ´ 100 =, ´ 100 = 3%, 100, R1, , 71. Given, R2 = ( 200 ± 4 )W, \, , DR2, 4, 1, =, =, R2, 200 50, , 72. The equivalent resistance of series, combination,, i.e. R S = R1 + R2 = (100 ± 3) W + ( 200 ± 4 ) W, = ( 300 ± 7 ) W, , 73. As, R S = ( 300 ± 7 ) W, \, , 7, DR S, ´ 100 =, ´ 100 = 2.3%, 300, RS, , 74. The equivalent resistance of parallel, combination,, R¢ =, , R1 R2, R1 + R2, , 200, = 66.7 W, 3, DR ¢ = 18, . W, R ¢ = ( 66.7 ± 18, . )W, =, , Given,, \, , 75. The method of dimensions can only test the, dimensional validity but not the exact, relationship between physical quantities in, any equation., This is because, it does not distinguish, between the physical quantities having same, dimensions.
Page 39 :
28, , CBSE New Pattern ~ Physics 11th (Term-I), , E, n, é ML2 T-2 ù, 2 -1, So, dimensions of h = ê, ú = [ML T ], -1, ë T, û, , 76. Planck’s constant, h =, , Angular momentum, L = mvr, Dimensions of L = [M] [LT-1 ] [L] = [ML2 T-1 ], Work, W = Force ´ Displacement, \ Dimensions of W = [MLT-2 ] ´ [L] = [ML2 T-2 ], Linear momentum, p = Mass ´ Velocity, Dimensions of p = [M][LT-1 ] = [MLT-1 ], Force, Impulse, I =, Time, [MLT-2 ], Dimensions of I =, = [MLT-3 ], [T], Hence, only angular momentum has same, dimensions as that of Planck’s constant., , 77. Dimensions of speed [ v ] = [LT-1 ], Dimensions of acceleration [A] = [LT-2 ], Dimensions of force [F] = [MLT-2 ], Dimensions of Young modulus [Y] =, [ML-1 T-2 ], Let dimensions of Young’s modulus is, expressed in terms of speed, acceleration and, force as, …(i), [ Y ] = [ v ] a [ A ] b [F ] g, Then substituting dimensions in terms of M,, L and T, we get, [ML-1 T-2 ] = [LT-1 ]a [LT-2 ]b[MLT-2 ]g, = [ M gLa + b+ g T- a - 2b - 2g ], Now comparing powers of basic quantities on, both sides, we get, g =1, a + b + g = -1, and - a - 2b - 2g = -2, Solving these, we get, a = -4, b = 2, g = 1, Substituting the values of a, b and g in Eq. (i),, we get, [Y] = [v -4 A 2F 1 ], , 78. According to the question, the expression for, the scattered amplitude of light ( A s ) in terms, of amplitude of incident light ( A i ), volume, (V ) , distance from scattering particle (x) and, wavelength ( l ) can be given as, \, A s = kA 1i V 1 x -1 ld, where, k is the constant of proportionality., Writing the dimensions on both sides of the, above equation, we get, [L] = [L] [L3 ] [L-1 ] [Ld ] = [L3 + d ], Comparing the powers of L on both sides, we, get, or, 1=3+d, or, d = -2, 1, i. e., As µ 2, l, But, intensity ( I s ) µ [amplitude ( A s )] 2, 1, \, Is µ 4, l, Work done, 79. Given, power, P1 =, Time taken, 60 J 60 J, =, =, = 1 W or kg-m 2 s -3, 1min 60 s, which is the SI unit of power., Given, P1 = 1 W, M 1 = 1kg = 1000 g, L 1 =1 m = 100 cm , T 1 = 1 s, In new system, P2 = ?, M 2 = 100 g, L 2 = 100 cm,, T 2 = 1 min = 60 s, \ Conversion of 60 J per min or 1W in a new, system, i.e., a, , b, , c, , 2, , -3, , éM ù éL ù éTù, P2 = P1 ê 1 ú ê 1 ú ê 1 ú, ë M 2 û ë L 2 û ë T2 û, Now, [power] = [ML2 T -3 ], So,, a = 1, b = 2, and, c = -3, 1, , Þ, , é 1000 ù é 100 ù é 1 ù, P2 = 1 ê, ë 100 úû êë 100 úû êë 60 úû, = 216, . ´ 10 6 units, , \ 60 J min, , -1, , = 2.16 ´ 10 6 new units of power
Page 40 :
CBSE New Pattern ~ Physics 11th (Term-I), , 29, , 03, Motion in a, Straight Line, Quick Revision, 1. Rest If the position of an object does not change, w.r.t. its surrounding with the passage of time, it is, said to be at rest. e.g. Book lying on the table, a, person sitting on a chair, etc., 2. Motion If the position of an object is continuously, changing w.r.t. its surrounding w.r.t time, then it is said, to be in the state of motion. e.g. The crawling insects,, water flowing down a dam, etc., 3. Types of Motion, On the basis of the nature of path followed, motion, is classified as, ● Rectilinear Motion The motion in which a, particle moves along a straight line is called, rectilinear motion. e.g. Motion of a sliding body, on an inclined plane., ● Circular Motion The motion in which a particle, moves in a circular path is called circular motion., e.g. A string whirled in a circular loop., ● Oscillatory Motion The motion in which a, particle moves to and fro about a given point is, known as oscillatory motion. e.g. Simple, pendulum., On the basis of the number of coordinates required, to define the motion of an object, motion is, classified as, ● One-dimensional Motion (1-D) The motion of, an object is considered as 1-D, if only one, coordinate is needed to specify the position of the, object., , Two-dimensional Motion (2-D) The, motion of an object is considered as 2-D,, if two coordinates are needed to specify, the position of the object. In 2-D motion,, the object moves in a plane. e.g. A, satellite revolving around the earth., ● Three-dimensional Motion (3-D) The, motion of an object is considered as 3-D,, if all the three coordinates are needed to, specify the position of the object., This type of motion takes place in, three-dimensional space., e.g. Butterfly flying in garden, the, motion of water molecules and motion, of kite in the sky., 4. Point Object An object is considered as, point object, if the size of the object is, much smaller than the distance travelled, by it in a reasonable duration of time., e.g. Earth can be considered as a point, object in its orbit., 5. Position It is defined as the point where, an object is situated., 6. Path Length or Distance The length of, the path covered by the object in a given, time-interval is known as its path length or, distance travelled. It is a scalar quantity,, i.e. it has only magnitude but no direction., ●
Page 41 :
30, , CBSE New Pattern ~ Physics 11th (Term-I), , 7. Displacement The change in position of an, object in a particular direction is termed as, displacement, i.e. the difference between the, final and initial positions of the object in a, given time. It is denoted by Dx., Mathematically, it is represented by, Dx = x2 - x1, where, x 1 and x 2 are the initial and final, positions of the object, respectively., Cases, ● If x, 2 > x 1, then Dx is positive., ● If x, 1 > x 2, then Dx is negative., ● If x, 1 = x 2, then Dx is zero., It is a vector quantity as it possesses both, the, magnitude and direction., 8. Uniform Motion in a Straight Line A body, is said to be in a uniform motion, if it travels, equal distances in equal intervals of time along, a straight line. A distance (x)-time (t) graph for, uniform motion is a straight line passing, through the origin., 9. Non-uniform Motion A body is said to be in, non-uniform motion, if it travels unequal, displacements in equal intervals of time., 10. Speed The path length or the distance, covered by an object divided by the time taken, to cover that distance is called its speed., Distance travelled, Speed =, Time taken, It is a scalar quantity. The speed of the object, for a given interval of time is always positive., Unit of speed In SI (MKS) system, the unit of, speed is ms –1 and in CGS, it is cms -1., Dimensional formula [M 0 LT -1], ● Average Speed Average speed of an, object is defined as the total distance, travelled divided by the total time taken., Total distance travelled, Average speed, v av =, Total time taken, ●, , Instantaneous Speed Speed at an instant is, defined as the limit of the average speed as, the time interval ( Dt ) becomes infinitesimally, small or approaches to zero., Mathematically, instantaneous speed (v i ) at, any instant of time (t) is expressed as, , Ds, v i = Dlim, t ®0, Dt, ds, or, vi =, dt, where, ds is the distance covered in time dt., 11. Velocity The rate of change in position or, displacement of an object with time is called, the velocity of that object., Displacement, i.e. Velocity =, Time, It is a vector quantity., The velocity of an object can be positive, zero, and negative according to its displacement., Unit of velocity In CGS, the unit of velocity, is cms -1 and in MKS or SI, it is ms -1., Dimensional formula [M 0LT -1 ], ●, , ●, , Average Velocity Average velocity of a, body is defined as the change in position or, displacement ( D x ) divided by the time, interval ( Dt ) in which that displacement, occurs., Average velocity,, Total displacement ( Dx ), v av =, Total time taken ( Dt ), Instantaneous Velocity Velocity at an, instant is defined as the limit of average, velocity as the time interval ( Dt ) becomes, infinitesimally small or approaches to zero., Mathematically, instantaneous velocity (v i ) at, an instant of time (t) is given by, Dx, v i = lim, Dt ® 0 D t, dx, or, vi =, dt, where, dx is displacement for time dt., , 12. Acceleration Acceleration of a body can be, expressed as the rate of change of velocity with, time., Change in velocity, Acceleration =, Time taken, It is a vector quantity. The SI unit of, acceleration is ms –2 and in CGS system, its unit, is cm s –2. Its dimensional formula is [M 0LT –2 ] .
Page 42 :
CBSE New Pattern ~ Physics 11th (Term-I), , 13. Types of Acceleration, ● Uniform Acceleration If an object is, moving with uniform acceleration, it means, that the change in velocity is equal in equal, intervals of time., ● Non-uniform Acceleration If an object has, variable or non-uniform acceleration, it, means that, the change in velocity is unequal, in equal intervals of time., ● Average Acceleration The average, acceleration over a time interval is defined as, the change in velocity divided by the time, interval., Average acceleration,, Dv v 2 - v 1, =, a av =, Dt, t2 - t1, ●, , Instantaneous Acceleration It is defined, as the acceleration of a body at a certain, instant or the limiting value of average, acceleration when time interval becomes, very small or tends to zero. So, instantaneous, acceleration,, Dv d v, =, a inst = lim, Dt ® 0 D t, dt, , dv, is the differential coefficient of v, dt, w.r.t. t., 14. Kinematic Equations for, Uniformly Accelerated Motion If the, change in velocity of an object in each unit of, time is constant, then the object is said to be, moving with constant acceleration and such a, motion is called uniformly accelerated, motion. An object moves along a straight line, with a constant acceleration a and u be the, initial velocity at t = 0 and v be the final velocity, of the object after time (t), then, ● Velocity-Time Relation v = u + at, 1 2, ● Position-Time Relation x = ut +, at, 2, where, x is the position of the object at time t., 2, ● Position-Velocity Relation v, = u 2 + 2ax, ● Displacement of the Object in, a, nth Second s (nth ) = u + ( 2n - 1), 2, where,, , 31, 15. Non-uniformly Accelerated Motion, When acceleration of an object is not constant, or acceleration is a function of time, then, following relations hold for one-dimensional, motion, dx, ● v =, dt, ● dx = v dt, dv, dv, ● a =, =v, dx, dt, ● dv = a dt or vdv = adx, 16. Equations of Motion for the Motion of an, Object under Gravity, When an object is thrown upwards or fall, towards the earth under the effect of gravity, only, then its motion is called motion under, gravity., In this case, the equations of motion are given, below, v = u + (+ g ) t, Upward, motion, , g, 1, (+ g ) t2, g, 2, Downward, v 2 = u2 + 2(+ g )h, motion, In case of upward motion, acceleration due to, gravity, g is taken as negative and for, downward motion, g is taken as positive., , h = ut +, , 17. Stopping Distance for a Vehicle When, brakes are applied to a moving vehicle, the, distance it travels before stopping is called, stopping distance., u2, Stopping distance, ds =, 2a, where, u = initial velocity of the vehicle, and a = retardation., 18. Relative Velocity in 1-D It is defined as the, time rate of change of relative position of one, object w.r.t. to another., If an object A is moving with velocity v A and an, object B is moving with velocity v B , then the, velocity of object A relative to object B is, given as v AB = v A - v B, The relative velocity of object B relative to, object A is v BA = v B - v A
Page 43 :
32, , CBSE New Pattern ~ Physics 11th (Term-I), , 19. Different Graphs related to Motion are as, follows, Displacement-Time Graph, Condition, For a stationary body, , Graph, , Graph, , Body moving with a, constant retardation and, its initial velocity is, non-zero, , B, , Time, , Body moving with a, constant retardation with, zero initial velocity, , Time, , O, , Displacement, , O, , Velocity, , Time, , Displacement, O, , Time, , O, , Body moving with a, constant retardation, , A, , O, , Body moving with, increasing acceleration, , Body moving with a, constant acceleration, , Velocity, , Displacement, , O, , Body moving with a, constant velocity, , Condition, , Body moving with, decreasing acceleration, , Time, , Velocity, , Displacement, , O, Time, , O, , Body moving with, infinite velocity, but such, motion of a body is never, possible., , Displacement, B, , Note Slope of velocity-time graph gives average, acceleration., , Acceleration-Time Graph, Condition, , O, , A, , Time, , Time, , Body moving with a, constant acceleration, , Graph, Acceleration, , Note Slope of displacement-time graph gives average, velocity., , Velocity-Time Graph, Condition, Body moving with a, constant velocity, , Velocity, , O, , Body moving with a, constant acceleration, having zero initial, velocity, , O, , Graph, , Body moving with, constant decreasing, acceleration, , Time, , Acceleration, , O, , Time, , Time, , Acceleration, , O, Time, , Velocity, , O, , Body moving with, constant increasing, acceleration, , Time
Page 44 :
CBSE New Pattern ~ Physics 11th (Term-I), , 33, , Objective Questions, Multiple Choice Questions, , 5. The displacement of a car is given as, , 1. Which of the following is an example of, one-dimensional motion?, (a), (b), (c), (d), , Landing of an aircraft, Earth revolving around the sun, Motion of wheels of moving train, Train running on a straight track, , 2. The coordinates of object with respect, to a frame of reference at t = 0 s are, ( - 1, 0 , 3). If t = 5 s, its coordinates are, ( - 1, 0 , 4 ), then the object is in, (a), (b), (c), (d), , motion along Z-axis, motion along X-axis, motion along Y-axis, rest position between t = 0 s and t = 5 s, , 3. A person moves towards east for 3 m,, then towards north for 4 m and then, moves vertically up by 5 m. What is his, distance now from the starting point?, (a) 5 2 m (b) 5 m, , (c) 10 m, , (d) 20 m, , 4. For a stationary object at x = 40 m, the, position-time graph is, , - 240 m, here negative sign indicates, (a), (b), (c), (d), , direction of displacement, negative path length, position of car at that point, no significance of negative sign, , 6. Snehit starts from his home and walks, 50 m towards north, then he turns, towards east and walks 40 m and then, reaches his school after moving 20 m, towards south. Then, his displacement, from his home to school is, (a) 50 m, , (b) 110 m, , (c) 80 m, , (d) 40 m, , 7. A vehicle travels half the distance l with, speed v 1 and the other half with speed, v 2 , then its average speed is, , (NCERT Exemplar), , (a), , v1 + v2, 2, , (b), , 2v 1 + v 2, v1 + v2, , (c), , 2v 1v 2, v1 + v2, , (d), , l (v 1 + v 2 ), v 1v 2, , 8. A runner starts from O and comes back, to O following path OQRO in 1h. What, is his net displacement and average, speed?, , x (m), 40, , R, , (a), , 20, 0, , 10, , 20 30 40, , t (s), O, , x (m), , (b), , Q, , 40, , 0, , t (s), , 20, , x (m), , (c), , 1km, , (a) 0,3.57 km/h, , (b) 0,0 km/h, , (c) 0,2.57 km/h, , (d) 0,1 km/h, , 9. The sign ( + ve or - ve) of the average, , 40, , velocity depends only upon, 30, , (d) None of the above, , t (s), , (a), (b), (c), (d), , the sign of displacement, the initial position of the object, the final position of the object, None of the above
Page 45 :
34, , CBSE New Pattern ~ Physics 11th (Term-I), , 10. Find the average velocity, when a, particle completes the circle of radius, 1m in 10 s., (b) 3.14 m/s (c) 6.28 m/s (d) zero, , 11. The displacement-time graph of two, , Displacement (x), , moving particles make angles of 30°, and 45° with the X -axis. The ratio of, their velocities is, , 14. For the x-t graph given below, the v - t, graph is shown correctly in, x(m), , (a), 30°, , 45°, , (b) 1 :2, , (c) 1 :1, , (d), , 3 :2, , (b), , given below, the average velocity, between time t = 5 s and t = 7 s is, (c), , P1, 0, , 1, , 2, , 3, , 4, , 5, , 6 7, t (s), , (a) 8 ms-1, , (b) 8.7 ms-1, , (c) 7.8 ms-1, , (d) 13.7 ms-1, , 8, , 13. Figure shows the x-t plot of a particle in, one-dimensional motion. Two different, equal intervals of time show speed in, time intervals 1 and 2 respectively, then, , t(s), , 0, , t(s), , 0, , t(s), , 0, , t(s), , v (ms–1), , P2, , (d), , 0, , v (ms–1), , Time (t), , 12. In figure, displacement-time (x - t ) graph, , 35, 30, x (m) 27.4, 25, 20, 15, 10, 5, 0, , v (ms–1), , t(s), , 0, , (a) 1 : 3, , v1 >v2, v2 >v1, v1 =v2, Data insufficient, , v (ms–1), , (a) 2 m/s, , (a), (b), (c), (d), , 15. The speed-time graph of a particle, moving along a fixed direction is as, shown in the figure. The distance, traversed by the particle between t = 0 s, to t = 10 s is, , v (ms–1), , 12, , A, , x, , C, 2, 1, , t, , O, , (a) 20 m, , 5, , (b) 40 m, , B, 10, , t (s), , (c) 60 m (d) 80 m
Page 46 :
CBSE New Pattern ~ Physics 11th (Term-I), , 35, , 16. If an object is moving in a straight line,, then, , x (m), , (a) the directional aspect of vector can be, specified by + ve and - ve signs, (b) instantaneous speed at an instant is equal, to the magnitude of the instantaneous, velocity at that instant, (c) Both (a) and (b), (d) Neither (a) nor (b), , 17. In one dimensional motion,, instantaneous speed v satisfies, (NCERT Exemplar), 0 £ v < v 0 . Then, (a) displacement in timeT must always take, non-negative values, (b) displacement x in timeT satisfies, - v 0 T < x < v 0T, (c) acceleration is always a non-negative, number, (d) motion has no turning points, , 18. The x-t equation is given as x = 2t + 1., , t1, t (s), , (a), (b), (c), (d), , 22. A particle moves in a straight line. It, can be accelerated, (a) only, if its speed changes by keeping its, direction same, (b) only, if its direction changes by keeping its, speed same, (c) Either by changing its speed or direction, (d) None of the above, , 23. An object is moving along the path, OABO with constant speed, then, , The corresponding v-t graph is, (a), (b), (c), (d), , B, , a straight line passing through origin, a straight line not passing through origin, a parabola, None of the above, , 19. The displacement x of an object is given, as a function of time, x = 2t + 3t 2 . The, instantaneous velocity of the object at, t = 2 s is, (a) 16 ms -1, (c) 10 ms -1, , (b) 14 ms -1, (d) 12 ms -1, , from rest (at t = 0) is given by, s = 6t 2 - t 3 . The time in seconds at, which the particle will attain zero, velocity again is, (b) 4, , (c) 6, , O, , A, , (a) the acceleration of the object while moving, along to path OABO is zero, (b) the acceleration of the object along the, path OA and BO is zero, (c) there must be some acceleration along the, path AB, (d) Both (b) and (c), , 20. The displacement of a particle starting, , (a) 2, , zero, positive, Data insufficient, Cannot be determined, , (d) 8, , 21. A car moves along a straight line, according to the x-t graph given below., The instantaneous velocity of the car at, t = t 1 is, , 24. The average velocity of a body moving, with uniform acceleration travelling a, distance of 3.06 m is 0.34 ms -1 . If the, change in velocity of the body is, 0.18 ms -1 during this time, its uniform, acceleration is, (a), (b), (c), (d), , 0.01 ms-2, 0.02 ms-2, 0.03 ms-2, 0.04 ms-2
Page 47 :
36, , CBSE New Pattern ~ Physics 11th (Term-I), , 30. The resulting a-t graph for the given v-t, , 25. The slope of the straight line, connecting the points corresponding to, (v 2 , t 2 ) and (v 1 , t 1 ) on a plot of velocity, versus time gives, (a), (b), (c), (d), , average velocity, average acceleration, instantaneous velocity, None of the above, , graph is correctly represented in, , v(m/s), , 26. The displacement x of a particle at time, , (c) 2 g, , (d) -2 g, , (a), , 27. The displacement (in metre) of a, particle moving along X -axis is given, by x = 18 t + 5t 2 . The average, acceleration during the interval t 1 = 2 s, and t 2 = 4 s is, (a) 13 ms-2, (c) 27 ms-2, , (b), , (b) 10 ms-2, (d) 37 ms-2, , 28. The relation between time and distance, is t = ax + bx , where a and b are, constants. The retardation is, 2, , (a) 2 av 3, (c) 2 abv 3, , (c), , a (ms–2), , (b) - b + 2 g, , 4.8, 2.4, 0, – 2.4, – 4.8, – 7.2, – 9.6, –12.0, , a (ms–2), , (a) -b, , 2 4 6 8 10 12 1416 18 20 t(s), , 4.8, 2.4, 0, – 2.4, – 4.8, , a (ms–2), , t along a straight line is given by, x = a - bt + gt 2 . The acceleration of the, particle is, , 30, 25, 24, 20, 15, 10, 5, 0, , (b) 2bv 3, (d) 2b 2v 3, , 2 4 6 8 10 12 14 16 18 20 22, , t (s), , 2 4 6 8 10 12 14 16 18 20 22, , t (s), , 9.6, 4.8, 2.4, 0, , 2, , 4, , 6, , 8, , 10 12, , t (s), , Velocity (cms−1), , shown in the figure. The maximum, acceleration is, , 0, , 80, , 2 4 6 8 10 12 14 16 18 20 22, , t (s), , 60, , 31. The kinematic equations of rectilinear, , 40, 20, 10 20 30 40 50 60 70 80, Time (s), , (a) 1 cms, , (d), , a(ms–2), , 29. The v -t graph of a moving object is, , -2, , (b) 2 cms, , -2, , (d) 6 cms-2, , (c) 3 cms, , -2, , motion for constant acceleration for a, general situation, where the position, coordinate at t = 0 is non-zero, say x 0 is, (a) v = v 0 + at, 1 2, at, 2, 2, 2, (c) v = v 0 + 2a (x - x0 ), (d) All of the above, (b) x = x0 + v 0 t +
Page 48 :
CBSE New Pattern ~ Physics 11th (Term-I), , 37, 36. A particle is situated at x = 3 units at, , 32. The given acceleration-time graph, , t = 0. It starts moving from rest with a, constant acceleration of 4 ms -2 . The, position of the particle at t = 3 s is, , represents which of the following, physical situations?, a, , (a) x = + 21 units, , (b) x = + 18 units, , (c) x = -21 units, , (d) None of these, , 37. Consider the relation for relative, t, , (a) A cricket ball moving with a uniform speed, is hit with a bat for a very short time, interval., (b) A ball is falling freely from the top of a, tower., (c) A car moving with constant velocity on a, straight road., (d) A football is kicked into the air vertically, upwards., , velocities between two objects A and B,, v BA = - v AB, The above equation is valid, if, (a), (b), (c), (d), , v A and v B are average velocities, v A and v B are instantaneous velocities, v A and v B are average speed, Both (a) and (b), , 38. A person is moving with a velocity of, , 10 ms -1 . A constant force acts for 4 s on, the object and gives it a speed of 2 ms -1, in opposite direction. The acceleration, produced is, , 10 m s -1 towards north. A car moving, with a velocity of 20 ms -1 towards south, crosses the person., The velocity of car relative to the, person is, , (a) 3 ms-2, (c) 6 ms-2, , (a) - 30 ms-1, (c) 10 ms-1, , 33. An object is moving with velocity, , (b) - 3 ms-2, (d) - 6 ms-2, , 34. All the graphs below are intended to, represent the same motion. One of, them does it incorrectly. Pick it up., Distance, , Velocity, , (a), , (b), , Position, Position, , (c), , Time, , 39. A motion of a body is said to be ……, if, it moves along a straight line in any, direction., (a), (b), (c), (d), , one-dimensional, two dimensional, three-dimensional, All of the above, , 40. The numerical ratio of displacement to, , Velocity, , (d), , Time, , Time, , the distance covered by an object is, always equal to or less than …… ., (a) 1, (c) Both (a) and (b), , 35. Velocity of a body moving along a, straight line with uniform acceleration a, reduces by (3/4)th of its initial velocity, in time t 0 . The total time of motion of, the body till its velocity becomes zero is, (a), , 4, t0, 3, , (b), , 3, t0, 2, , (c), , 5, t0, 3, , (d), , (b) + 20ms-1, (d) - 10 ms-1, , 8, t0, 3, , (b) zero, (d) infinity, , 41. The time taken by a 150 m long train to, cross a bridge of length 850 m is 80 s. It, is moving with a uniform velocity of, …… km/h., (a) 45, (c) 60, , (b) 90, (d) 70
Page 49 :
38, , CBSE New Pattern ~ Physics 11th (Term-I), , 42. The distance-time graph of …… is a, straight line., (a), (b), (c), (d), , uniform motion, non-uniform motion, uniform acceleration, None of the above, , 43. Which of the following statement is, correct?, (a) The magnitude of average velocity is the, average speed., (b) Average velocity is the displacement, divided by time interval., (c) When acceleration of particle is constant,, then motion is called as non-uniformly, accelerated motion., (d) When a particle returns to its starting point,, its displacement is non-zero., , With reference to the graph, which of, the given statement(s) is/are incorrect?, (a) The instantaneous speed during the, interval t = 5 s to t = 10 s is negative at all, time instants during the interval., (b) The velocity and the average velocity for, the interval t = 0 s to t = 5 s are equal and, positive., (c) The car changes its direction of motion at, t = 5 s., (d) The instantaneous speed and the, instantaneous velocity are positive at all, time instants during the interval t = 0 s to, t = 5 s., , 46. A graph of x versus t is shown in figure., Choose correct statement given below., x, , 44. For motion of the car between t = 18 s, , A, , and t = 20 s, which of the given, statement is correct?, , B, , E, C, , 296, , D, , x (m) 250, 100, O, , 10, , 18, , 20 t(s), , (a) The car is moving in a positive direction, with a positive acceleration., (b) The car is moving in a negative direction, with a positive acceleration., (c) The car is moving in positive direction with, a negative acceleration., (d) The car is moving in negative direction with, a negative acceleration., , 45. The x-t graph for motion of a car is, , 47. Match the Column I with Column II, and select the correct option from the, codes given below, Column I, , Column II, , A., , d v / dt, , p., , Acceleration, , B., , d | v |/dt, , q., , Rate of, change of, speed, , C., , dr, dt, , r., , Velocity, , D., , d | r|, dt, , s., , Magnitude, of velocity, , given below, 10, x (m), , O, , 5, , 10, t(s), , t, , (a) The particle having some initial velocity at, t = 0., (b) At point B, the acceleration a > 0., (c) At point C, the velocity and the acceleration, vanish., (d) The speed at E exceeds that at D.
Page 50 :
CBSE New Pattern ~ Physics 11th (Term-I), , 39, , Codes, , Column I, , A, , B, , C, , D, , (a) p, , q, , r, , s, , (b) p, , r, , s, , q, , (c) q, , p, , r, , s, , (d) s, , r, , p, , q, , Position-time, graph of two, objects with, equal, velocities., , A., , 48. Given x-t graph represents the motion, of an object. Match the Column I, (parts of graph) with Column II, (representation) and select the correct, option from the codes given below., , Position-time, graph of two, objects with, unequal, velocities but, in same, direction., , B., , A, x, C, , B, O, , t, , Column I, A., , Position-time, graph of two, objects with, velocities in, opposite, direction., , C., , Column II, , Part OA of, graph, , p., , Positive, velocity, , B., , Part AB of, graph, , q., , Object at rest, , C., , Part BC of, graph, , r., , Negative, velocity, , D., , Point A in the, graph, , s., , Change in, direction of, motion, , Codes, A, , B, , C, , D, , (a) p, , q, , r, , s, , (b) p, , r, , q, , s, , (c) q, , p, , r, , s, , (d) s, , r, , q, , p, , 49. Match the Column I (position-time, graph) with Column II (representation), and select the correct option from the, codes given below., , Column II, p., , x (m), , O, , q., , t(s), , x (m), O, , r., , t(s), , x (m), , O, , t(s), , Codes, A, , B, , C, , A, , B, , C, , (a) p, , q, , r, , (b) q, , p, , r, , (c) p, , r, , q, , (d) q, , r, , p, , Assertion-Reasoning MCQs, For question numbers 50 to 63, two, statements are given-one labelled, Assertion (A) and the other labelled, Reason (R). Select the correct answer to, these questions from the codes (a), (b), (c), and (d) are as given below, (a) Both A and R are true and R is the, correct explanation of A., (b) Both A and R are true but R is not the, correct explanation of A., (c) A is true but R is false., (d) A is false and R is also false., , 50. Assertion In real-life, in a number of, situations, the object is treated as a, point object.
Page 51 :
40, , CBSE New Pattern ~ Physics 11th (Term-I), , Reason An object is treated as point, object, as far as its size is much smaller, than the distance, it moves in a, reasonable duration of time., , 51. Assertion If the displacement of the, body is zero, the distance covered by it, may not be zero., Reason Displacement is a vector, quantity and distance is a scalar quantity., , 52. Assertion An object can have constant, speed but variable velocity., Reason SI unit of speed is m/s., , 53. Assertion The speed of a body can be, negative., Reason If the body is moving in the, opposite direction of positive motion,, then its speed is negative., , 54. Assertion For motion along a straight, line and in the same direction, the, magnitude of average velocity is equal, to the average speed., Reason For motion along a straight line, and in the same direction, the, magnitude of displacement is not equal, to the path length., , 55. Assertion An object may have varying, speed without having varying velocity., Reason If the velocity is zero at an, instant, the acceleration is zero at that, instant., , Reason Infinite acceleration cannot be, realised in practice., , 58. Assertion In realistic situation, the x-t,, v-t and a-t graphs will be smooth., Reason Physically acceleration and, velocity cannot change values abruptly, at an instant., , 59. Assertion A body cannot be, accelerated, when it is moving, uniformly., Reason When direction of motion of, the body changes, then body does not, have acceleration., , 60. Assertion For uniform motion, velocity, is the same as the average velocity at all, instants., Reason In uniform motion along a, straight line, the object covers equal, distances in equal intervals of time., , 61. Assertion A body is momentarily at, rest at the instant, if it reverse the, direction., Reason A body cannot have, acceleration, if its velocity is zero at a, given instant of time., , 62. Assertion In the s-t diagram as shown, in figure, the body starts moving in, positive direction but not from s = 0., s, , 56. Assertion Acceleration of a moving, particle can change its direction without, any change in direction of velocity., Reason If the direction of change in, velocity vector changes, direction of, acceleration vector does not changes., , 57. Assertion The v-t graph perpendicular, to time axis is not possible in practice., , t0, , t, , Reason At t = t 0 , velocity of body, changes its direction of motion.
Page 52 :
CBSE New Pattern ~ Physics 11th (Term-I), , 63. Assertion If acceleration of a particle, , 41, , 67. If the car goes from O to P and returns, , moving in a straight line varies, as a µ t n , then s µ t n + 2 ., , back to O, the displacement of the, journey is, , Reason If a-t graph is a straight, line,then s-t graph may be a parabola., , (a) zero, (c) 420 m, , 68. The path length of journey from O to P, , Case Based MCQs, , and back to O is, , Direction Answer the questions from, 64-68 on the following case., Motion in a Straight Line, If the position of an object is continuously, changing w.r.t. its surrounding, then it is said, to be in the state of motion. Thus, motion can, be defined as a change in position of an object, with time. It is common to everything in the, universe., In the given figure, let P, Q and R represent, the position of a car at different instants of, time., R, , O, , –160 –120 –80 –40, , 0, , Q, 40, , P, , 80 120 160 200 240 280 320 360 400 (m), X-axis, , 64. With reference to the given figure, the, position coordinates of points P and R, are, (a) P º (+ 360, 0, 0); R º (- 120, 0, 0), (b) P º (- 360, 0, 0); R º (+ 120, 0 , 0), (c) P º (0, + 360, 0); R º (- 120, 0, 0), (d) P º (0, 0, + 360); R º (0, 0, - 120), , 65. Displacement of an object can be, (a), (b), (c), (d), , positive, negative, zero, All of the above, , 66. The displacement of a car in moving, from O to P and its displacement in, moving from P to Q are, (a), (b), (c), (d), , + 360 m and - 120 m, - 120 m and + 360 m, + 360 m and + 120 m, + 360 m and - 600 m, , (b) 720 m, (d) 340 m, , (a) 0 m, (c) 360 m, , (b) 720 m, (d) 480 m, , Direction Answer the questions from, 69-73 on the following case., Average Speed and Average Velocity, When an object is in motion, its position, changes with time. So, the quantity that, describes how fast is the position changing, w.r.t. time and in what direction is given by, average velocity., It is defined as the change in position or, displacement (Dx ) divided by the time interval, (Dt ) in which that displacement occur., However, the quantity used to describe the, rate of motion over the actual path, is average, speed. It defined as the total distance travelled, by the object divided by the total time taken., , 69. A 250 m long train is moving with a, , uniform velocity of 45 kmh - 1 . The time, taken by the train to cross a bridge of, length 750 m is, (a) 56 s, (c) 80 s, , (b) 68 s, (d) 92 s, , 70. A truck requires 3 hr to complete a, journey of 150 km. What is average, speed?, (a) 50 km/h, (c) 15 km/h, , (b) 25 km/h, (d) 10 km/h, , 71. Average speed of a car between points, A and B is 20 m/s, between B and C is, 15 m/s and between C and D is 10 m/s., What is the average speed between A, and D, if the time taken in the
Page 53 :
42, , CBSE New Pattern ~ Physics 11th (Term-I), , mentioned sections is 20s, 10s and 5s,, respectively?, (a) 17.14 m/s, (c) 10 m/s, , (b) 15 m/s, (d) 45 m/s, , 72. A cyclist is moving on a circular track, of radius 40 m completes half a, revolution in 40 s. Its average velocity, is, (b) 2 ms -1, (d) 8 p ms-1, , (a) zero, (c) 4 p ms-1, , 74. The displacement of a body in 8 s, , 73. In the following graph, average velocity, is geometrically represented by, 35, 30, x (m) 27.4, 25, 20, 15, 10, 5, 0, , (a), (b), (c), (d), , The motion in which the acceleration remains, constant is known as to be uniformly, accelerated motion. There are certain, equations which are used to relate the, displacement (x), time taken (t ), initial velocity, (u ), final velocity (v ) and acceleration (a ) for, such a motion and are known as kinematics, equations for uniformly accelerated motion., starting from rest with an acceleration, of 20 cms -2 is, (a) 64 m, (c) 64 cm, , (b) 640 m, (d) 0.064 m, , 75. A particle starts with a velocity of, , 2 ms -1 and moves in a straight line with, a retardation of 01, . ms -2 . The first time, at which the particle is 15 m from the, starting point is, , P2, , P1, 0, , 1, , 2, , 3, , 4, , 5, , 6 7, t (s), , 8, , length of the line P1 P2, slope of the straight line P1 P2, slope of the tangent to the curve at P1, slope of the tangent to the curve at P2, , Direction Answer the questions from, 74-78 on the following case., Uniformly Accelerated Motion, The velocity of an object, in general, changes, during its course of motion. Initially, at the, time of Galileo, it was thought that, this, change could be described by the rate of, change of velocity with distance. But, through, his studies of motion of freely falling objects, and motion of objects on an inclined plane,, Galileo concluded that, the rate of change of, velocity with time is a constant of motion for, all objects in free fall., This led to the concept of acceleration as the, rate of change of velocity with time., , (a) 10 s, (c) 30 s, , (b) 20 s, (d) 40 s, , 76. If a body starts from rest and travels, 120 cm in 6th second, then what is its, acceleration?, (a) 0.20 ms- 2, (c) 0218, . ms- 2, , (b) 0027, ., ms- 2, (d) 003, . ms- 2, , 77. An object starts from rest and moves, with uniform acceleration a. The final, velocity of the particle in terms of the, distance x covered by it is given as, (a), (c), , 2ax, ax, 2, , (b) 2ax, (d), , ax, , 78. A body travelling with uniform, acceleration crosses two points A and B, with velocities 20 ms -1 and 30 ms -1 ,, respectively. The speed of the body at, mid-point of A and B is, (a) 25 ms-1, (c) 24 ms-1, , (b) 25.5 ms-1, (d) 10 6 ms-1
Page 54 :
CBSE New Pattern ~ Physics 11th (Term-I), , 43, , ANSWERS, Multiple Choice Questions, 1. (d), 11. (a), 21. (a), , 2. (a), 12. (b), 22. (c), , 3. (a), 13. (b), 23. (d), , 4. (a), 14. (a), 24. (b), , 5. (a), 15. (c), 25. (b), , 6. (a), 16. (c), 26. (c), , 7. (c), 17. (b), 27. (b), , 8. (a), 18. (b), 28. (a), , 9. (a), 19. (b), 29. (d), , 10. (d), 20. (b), 30. (a), , 31. (d), 41. (a), , 32. (a), 42. (a), , 33. (b), 43. (b), , 34. (b), 44. (a), , 35. (a), 45. (a), , 36. (a), 46. (c), , 37. (d), 47. (a), , 38. (a), 48. (b), , 39. (a), 49. (b), , 40. (a), , 52. (b), 62. (c), , 53. (d), 63. (b), , 54. (c), , 55. (d), , 56. (d), , 57. (a), , 58. (a), , 59. (d), , 66. (a), 76. (c), , 67. (a), 77. (a), , 68. (b), 78. (b), , 69. (c), , 70. (a), , 71. (a), , 72. (b), , 73. (b), , Assertion-Reasoning MCQs, 50. (a), 60. (b), , 51. (b), 61. (c), , Case Based MCQs, 64. (a), 74. (c), , 65. (d), 75. (a), , SOLUTIONS, 1. In one-dimensional motion, only one, coordinate is required to specify the position, of the object. So, a train running on a straight, track is an example of one-dimensional, motion., , 2. Given, at t = 0 s, position of an object is, ( -1 , 0 , 3) and at t = 5 s, its coordinate is, ( -1 , 0 , 4 ). So, there is no change in x and, y-coordinates, while z -coordinate changes, from 3 to 4. So,the object is in motion along, Z-axis., , 3. Distance from starting point, = ( 3) 2 + ( 4 ) 2 + ( 5) 2 = 5 2 m, , 4. For a stationary object, the position-time, graph is a straight line parallel to the time, axis, so for the given object at x = 40 m,, x-t graph is correctly shown in option (a)., , 5. In I-D motion, positive and negative signs are, used to specify the direction of motion., Since, displacement is a vector quantity, so, negative sign in -240 m indicates the, direction of displacement., , 6. Let O be the starting point, i.e. home. So,, according to the question, Snehit moves from, O to A (50 m) towards north, then from A to, , B (40 m) towards east and from B to C (20 m), towards south as shown in the figure below., 40 m, , A, , 40 m, , D, 50 m, , N, , B, 20 m, C, , E, , W, S, , 30 m, O, , θ, , P, , Displacement of Snehit is OC, which can be, calculated by Pythagoras theorem, i.e., In DODC, OC 2 = OD 2 + CD 2 = ( 30 ) 2 + ( 40 ) 2, = 900 + 1600 = 2500, Þ, , OC = 50 m, , 7. Time taken to travel first half distance,, t1 =, , l/ 2, l, =, 2v 1, v1, , Time taken to travel second half distance,, l, t2 =, 2v 2, Total time = t 1 + t 2 =, , l, l, lé1, 1ù, +, = ê + ú, 2v 1 2v 2 2 ë v 1 v 2 û
Page 55 :
44, , CBSE New Pattern ~ Physics 11th (Term-I), , We know that, v av = average speed, total distance, =, total time, l, 2v 1v 2, =, =, lé1, 1 ù v1 + v2, ê + ú, 2 ëv1 v2 û, , 8. As runner starts from O and comes back, to O, so net displacement is zero., Average speed, Total distance OQ + QR + RO, =, =, Total time, Total time, æ 90° ö, 1 km + ( 2pr ) ç, ÷ km + 1 km, è 360° ø, =, 1h, (Q angle of sector OQR is 90°), æ1ö, 1 + 2p ´ 1 ç ÷ + 1, è 4ø, =, 1, p, = 2 + = 3.57 km/h, 2, , 9. Since, average velocity,, v =, , D x Displacement, =, D t Time interval, , So, average velocity depends on the, displacement and hence it depends on the, sign of the displacement., , 10. When a particle completes one revolution in, circular motion, then average displacement, travelled by particle is zero., Hence, average velocity, average displacement, 0, =, =, =0, Dt, Dt, , 11. In case x - t graph is a straight line, the slope, of this line gives velocity of the particle., As slope = tan q, where q is the angle which, the tangent to the curve makes with the, horizontal in anti-clockwise direction., The velocities of two particles A and B are, 1, v A = tan 30° =, 3, v B = tan 45° = 1, The ratio of velocities,, 1, vA :vB =, :1 = 1 : 3, 3, , 12. Given, x 2 = 27.4 m, x 1 = 10 m, t 2 = 7 s and, t 1 = 5 s., Average velocity between 5 s and 7s,, x - x 1 27.4 -10, v = 2, =, t2 - t1, 7-5, =, , 17.4, = 8.7 ms -1, 2, , 13. Slope of x-t graph in a small interval, = Average speed in that interval, As, slope for interval 2 > slope for interval 1., \, v2 > v1, , 14. The x - t graph shown, is parallel to time axis., This means that, the object is at rest. So, the, velocity of the object is zero for all time, instants. Hence, v -t graph coincides with the, time axis as shown in graph (a)., , 15. Distance travelled by the particle between time, interval t = 0 s to t = 10 s, = Area of triangle OAB, 1, = ´ Base ´ Height, 2, 1, = ´ OB ´ AC, 2, 1, = ´ 10 ´ 12 = 60 m, 2, , 16. In one-dimensional motion, i.e. motion along, a straight line, there are only two directions, in which an object can move and these two, directions can be easily specified by + ve and, - ve signs., Also, in this motion instantaneous speed or, simply speed at an instant is equal to the, magnitude of instantaneous velocity at the, given instant., , 17. For maximum and minimum displacements,, we have to keep in mind the magnitude and, direction of maximum velocity., As, maximum velocity in positive direction is, v 0 and maximum velocity in opposite, direction is also - v 0 ., Maximum displacement in one direction = v 0T, Maximum displacement in opposite, directions = - v 0T, Hence, the range of displacement will be, -v 0T < x < v 0T .
Page 56 :
CBSE New Pattern ~ Physics 11th (Term-I), , 18. v =, , dx, = 2 ms -1 = constant, dt, , Since, the direction of velocity is changing,, i.e. there must be some acceleration along the, path AB., Distance, 3.06, 24. Time =, =, =9 s, Average velocity 0.34, , v ms–1, 2, t (s), , Hence, option (b) is correct., , 19. Given, x = 2t + 3t 2, dx, = 2+ 6t, dt, For t = 2 s, v = 2 + 6 ( 2) = 14 ms -1, v =, , 20. Displacement of the particle,, s = 6t 2 - t 3, Velocity of the particle,, ds d, v =, = ( 6t 2 - t 3 ), dt dt, v = 12t - 3t 2, For, v = 0 Þ 12t = 3 t 2 Þ t = 4 s, , 21. The instantaneous velocity is the slope of the, tangent to the x -t graph at that instant of, time., , x (m), , P, , t = t1, , 45, , Tangent at point P, corresponding to, t = t1, , t(s), , At t = t 1, the tangent is parallel to time axis as, shown above and hence its slope is zero., Thus, instantaneous velocity at t = t 1 is zero., , 22. Since velocity is a vector quantity, having, both magnitude and direction. So, a change, in velocity may involve change in either or, both of these factors. Therefore, acceleration, may result from a change in speed, (magnitude), a change in direction or changes, in both., , 23. For paths OA and BO, the magnitude of, velocity (speed) and direction is constant,, hence acceleration is zero. For path AB, since, this path is a curve, so the direction of the, velocity changes at every moment but the, magnitude of velocity (speed) remains, constant., , Acceleration, Change in velocity, =, Time, 0.18, =, = 0.02 ms -2, 9, , 25. Average acceleration is defined as the, average change of velocity per unit time. On, a plot of v -t, the average acceleration is the, slope of the straight line connecting the, points corresponding to ( v 2, t 2 ) and ( v 1, t 1 )., , 26. Given, x = a - bt + g t 2, dx d, = ( a - bt + g t 2 ) = - b + 2 g t, dt dt, dv d, a =, = ( - b + 2g t ) = 2g, dt dt, v =, , 27. Given, x = 18t + 5t 2, dx d, = (18t + 5t 2 ) = 18 + 10t, dt dt, \ v = 10t + 18, At t 1 = 2 s, v 1 = 10 ( 2) + 18 = 38 m/s, At t 2 = 4 s, v 2 = 10 ( 4 ) + 18 = 58 m/s, 58 - 38 20, v -v, \ a = 2 1 =, =, = 10 ms -2, 2, 2, t, 28. Given, t = ax 2 + bx, dt, = 2a x + b, dx, dx, 1, Þ, =v =, dt, 2ax + b, v =, , dv dv dx, =, dt dx dt, æ - v 2a ö, dv, 1, =, Þa =v ×, ç, ÷, dx 2ax + b è 2ax + b ø, As, acceleration, a =, , = - 2a v × v 2 = - 2av 3, \ Retardation = 2av 3, 29. Maximum acceleration means maximum, change in velocity in minimum time interval., In time interval t = 30 s to t = 40 s,, Dv 80 - 20 60, a =, =, =, = 6 cms -2, Dt 40 - 30 10
Page 57 :
46, , CBSE New Pattern ~ Physics 11th (Term-I), , 30. Average acceleration for different time, intervals is the slope of v-t graph, which are, as follows, ( 24 - 0 ) ms -1, For 0 s -10 s, a =, = 2.4 ms -2, (10 - 0 ) s, ( 24 - 24 ) ms -1, For 10 s -18 s, a =, = 0 ms -2, (18 - 10 ) s, ( 0 - 24 ) ms -1, For 18 s - 20 s, a =, = - 12 ms -2, ( 20 - 18 ) s, So, the corresponding a -t graph for the given, v -t graph is shown correctly in graph (a)., , 31. All the equations given in options (a), (b) and, (c) are the kinematic equations of rectilinear, motion for constant acceleration., , 32. The acceleration-time graph represents the, motion of a uniformly moving cricket ball, turned back by hitting it with a bat for a very, short time interval., , 33. Given, v = - 2 ms -1 (opposite direction),, t = 4 s and u = 10 ms -1, \ v = u + at or - 2 = 10 + 4a or a = - 3 ms -2, , 34. If velocity versus time graph is a straight line, with negative slope, then acceleration is, constant and negative., With a negative slope, distance-time graph, 1, æ, ö, will be parabolic çs = ut - at 2 ÷ ., è, 2 ø, , 37. Given,, , v BA = - v AB, , The above relation is true for both average, velocities of particles and instantaneous, velocities of particles., As speed is scalar quantity, ignorant of, direction, so average speed may not be equal., , 38. Let south to north direction be positive., Velocity of car, vC = - 20 ms -1, Velocity of person, v P = + 10 ms -1, vCP = vC - v P = ( - 20 ) - (10 ) = - 30 ms -1, , 39. One-dimensional motion is a motion along, a straight line in any direction. e.g. A train is, moving on a platform., Hence, option (a) is correct., , 40. Since, displacement d is always less than or, equal to the distance D but never greater, than it, i.e. d £ D. So, numerical ratio of, displacement to the distance covered by an, object is always equal to or less than one., , 41. Total distance = Length of train + Length of, bridge, = (150 + 850 ) m = 1000 m, Distance, 1000, Time =, Þ 80 =, v, Velocity, v =, , 1000, 1000 18, m/s Þ v =, ´, = 45 km/h, 80, 80, 5, , Hence, options (a), (c) and (d) are correct, so, , 42. In uniform motion, the velocity of an object, , option (b) will be incorrect., , does not change or it remains constant with, time., So, the graph of distance-time is a straight, line., 43. Statement given in option (b) is correct but, the rest are incorrect and these can be, corrected as,, In general, average speed is not equal to, magnitude of average velocity. It can be so, if, the motion is along a straight line without, change in direction., When acceleration of particle is not constant,, then motion is called as non-uniformly, accelerated motion., Displacement is zero, when a particle returns, to its starting point., , 35. According to kinematic equation of motion,, v = u - at, 3u, u, where, v = u = u /4 Þ, = u - at 0, 4, 4, Negatve sign signifies that the body will, decelerate, since the final velocity is, decreasing., u 4, or, = t0, a 3, u 4, Now, 0 = u - at or, t = = t0, a 3, , 36. Given, x 0 = 3 units, a = 4 ms -2, t = 3 s, Using relation, x = x 0 + v 0 t +, =3+, , 1 2, at, 2, , 1, ´ 4 ´ ( 3) 2 = + 21 units, 2
Page 58 :
CBSE New Pattern ~ Physics 11th (Term-I), , 44. For negative acceleration, the x - t graph, moves downward. But the car is moving in, positive direction as the position coordinate is, increasing in the positive direction., Thus, the statement given in option (a) is, correct, rest are incorrect., , 45. The instantaneous speed is always positive as, it is the magnitude of the velocity at an, instant, so it is positive during t = 5 s, to t = 10 s., For t = 0 s to t = 5 s, the motion is uniform, and x-t graph has positive slope. So, the, velocity and average velocity, instantaneous, velocity and instantaneous speed are equal, and positive., During t = 0 s to t = 5 s, the slope of the, graph is positive, hence the average velocity, and the velocity both are positive., During t = 5 s to t = 10 s, the slope of the, graph is negative, hence the velocity is, negative. Since, there is a change in sign of, velocity at t = 5 s, so the car changes its, direction at this instant., Hence, option (a) is incorrect, while all others, are correct., , 47, dr, is the magnitude of rate of change of, dt, position of particle. This means it represents, magnitude of velocity., Hence, A ® p, B ® q, C ® r and D ® s., 48. In x -t graph, OA ® Positive slope ® Positive, velocity, AB ® Negative slope ® Negative velocity, BC ® Zero slope ® Object is at rest, At point A, there is a change in sign of, velocity, hence the direction of motion must, have changed at A., Hence, A ® p, B ® r, C ® q and D ® s., , 49., , A. For equal velocities, the slope of the, straight lines must be same as shown below, x, , θ, θ (Equal) same slope, , O, , t(s), , B. For unequal velocity, slope is different, but, since, the objects are moving in the same, direction, the slope for both the graphs, must be of same sign (positive or negative), and they meet at a point as shown below, , 46. As, point A is the starting point, therefore, particle is starting from rest., , x, θ2, , At point B, the graph is parallel to time axis,, so the velocity is constant here. Thus,, acceleration is zero., Also point C, the graph changes slope, hence, velocity also changes., After graph at C is almost parallel to time, axis, hence we can say that velocity and, acceleration vanishes., From the graph, it is clear that, slope at D > slope at E, Hence, speed at D will be more than at E ., dv, 47., is the rate of change of velocity, so it, dt, represents acceleration., d | v|, is rate of change of speed of the particle., dt, dr, is the rate by which distance of particle, dt, from the origin is changing., , θ1, , C. For velocities in opposite direction, slopes, must be of opposite sign. Slope = tan q,, where q is the angle of the straight line with, horizontal in anti-clockwise direction. As,, we know, tan q1 > 0, tan q2 < 0., Hence, slopes are of opposite sign., This condition is shown below, , x, , O, , θ1, , θ2, t(s), , Hence A ® q, B ® p and C ® r., , 50. The approximation of an object as point, object is valid only, when the size of the
Page 59 :
48, , CBSE New Pattern ~ Physics 11th (Term-I), , object is much smaller than the distance it, moves in a reasonable duration of time., Therefore, both A and R are true and R is, the correct explanation of A., , 51. Distance is the total path length travelled by, the object. But displacement the shortest, distance between the initial and final, positions of the object. So, distance can never, be negative or zero. But displacement can be, zero, positive and negative., Also, distance is a scalar quantity. It means, that, it is always positive but however, displacement is a vector quantity. So, it may, be positive, zero or negative depending on, given situation., Therefore, both A and R are true but R is, not the correct explanation of A., , 52. Velocity is a vector quantity, so it has both, direction and magnitude. Hence, an object, can have variable velocity by keeping its, magnitude constant, i.e. speed and by, changing direction only., The SI unit of speed is m/s., Therefore, both A and R are true but R is, not the correct explanation of A., , 53. Speed can never be negative because it is a, scalar quantity. So, if a body is moving in, negative direction, then also the speed will be, positive., , dv, = Slope of v-t graph, dt, It v-t graph is perpendicular to t-axis, slope, =¥, , 57. Acceleration, a =, , \, , a =¥, , Therefore, both A and R are true and R is, the correct explanation of A., , 58. In realistic situation, the x - t , v - t and a - t, graphs will be smooth, as the values of, acceleration and velocity cannot change, abruptly since changes are always continuous., Therefore, both A and R are true and R is, the correct explanation of A., , 59. The uniform motion of a body means that,, the body is moving with constant velocity., But if the direction of motion is changing, (such as in uniform circular motion), its, velocity changes and thus uniform, acceleration is produced in the body., Therefore, A is false and R is also false., , 60. In uniform motion along a straight line, the, object covers equal distances in equal, intervals of time., For uniform motion, x - t graph is represented, as a straight line inclined to time axis. The, average velocity during any time interval, t = t 1 to t = t 2 is the slope of the line PQ, which coincides with the graph., , Therefore, A is false and R is also false., , Q, , 54. For motion in a straight line and in the same, direction,, Displacement = Total path length, Þ Average velocity = Average speed, Therefore, A is true but R is false., , 55. If speed varies, then velocity will definitely, vary., When a particle is thrown upwards, at, highest point a ¹ 0 but v = 0., Therefore, A is false and R is also false., v - v i dv, 56. Accleration, a = f, = , i.e. direction of, Dt, dt, acceleration is same as that of change in, velocity vector or in the direction of Dv., Therefore, A is false and R is also false., , P, x, , t1, , t2, , t, , Also, velocity at any instant say t = t 1 is the, slope of the tangent at point P which again, coincides with PQ or with the graph. Hence,, velocity is same as the average velocity at all, instants., Therefore, both A and R are true but R is, not the correct explanation of A., , 61. When a particle is released from rest position, under gravity, then v = 0 but a ¹ 0.
Page 60 :
CBSE New Pattern ~ Physics 11th (Term-I), , Also, a body is momentarily at rest at the, instant, if it reverse the direction., Therefore, A is true but R is false., , 71. Total distance ( d = vt ), , 62. Slope of s-t graph = velocity = positive, At t = 0, s ¹ 0, further at t = t 0 : s = 0, v ¹ 0., Therefore, A is true but R is false., , 63. By differentiating a-t equation two times, we, will get s-t equation., Further, a, , 49, , = 20 ´ 20 + 15 ´ 10 + 10 ´ 5 = 600 m, Total time = 20 + 10 + 5 = 35 s, Therefore, average speed, = 600 / 35 = 1714, . m/s, , 72. Given, R = 40 m and t = 40 s, Displacement, Time taken, 2R 2 ´ 40, =, =, = 2 ms -1, t, 40, , Average velocity =, , s, , 73. From the position-time graph, average, t, Straight line, , t, Parabola, , Therefore, both A and R are true but R is, not the correct explanation of A., , 64. The position coordinates of point, P = ( +360, 0, 0 ) and point R = ( -120, 0, 0 )., , 65. Displacement is a vector quantity, it can be, positive, negative and zero., , 66. Displacement, Dx = x 2 - x 1, For journey of car in moving from O to P ,, x 2 = + 360 m, x1 = 0, Þ, Dx = x 2 - x 1 = 360 - 0 = + 360 m, For journey, of car in moving from P to Q ,, x 2 = + 240 m, x 1 = + 360 m, Þ, Dx = x 2 - x 1 = 240 - 360 = - 120 m, Here, -ve sign implies that the displacement, is in –ve direction, i.e. towards left., , 67. Displacement, D x = x 2 - x 1 = 0 - 0 = 0, 68. Path length of the journey, = OP + PO = + 360 m + ( + 360 ) m = 720 m, Total distance, 69. Total time taken =, Speed, 250 + 750, = 80 s, 5, 45 ´, 18, Total distance, 70. Average speed =, Total time, 150, =, = 50 km/h, 3, t=, , velocity is geometrically represented by the, slope of curve, i.e. slope of straight line P1 P2 ., 1, 74. Displacement, s = ´ (0.2) (64) = 64 cm, 2, 1, 75. From equation of motion, s = ut - at 2, 2, 1, 2, 15 = 2t - ´ ( 0.1)t Þ t = 10 s, 2, , 76. From equation of motion,, a, ( 2n - 1), 2, a, 1.2 = 0 + ( 2 ´ 6 - 1), 2, 1.2 ´ 2, a =, = 0.218 ms - 2, 11, sn = u +, , Þ, Þ, , 77. Given,, , v0 = 0, , Using relation, v 2 = v 02 + 2ax, v 2 = 2ax, \, , v = 2ax, , 78. Let the acceleration of the car = a, and distance between A and B = d, Given, v = 30 ms -1 and u = 20 ms -1, 2ad = ( 30 ) 2 - ( 20 ) 2, 900 - 400, ad =, = 250, 2, When the car is at the mid-point of AB, then, speed of car is v 1 ., v 12 - ( 20 ) 2 = 2a ( d / 2), v 12 = ad + 400, = 250 + 400 = 650, Therefore, v 1 = 25.5 ms -1
Page 61 :
50, , CBSE New Pattern ~ Physics 11th (Term-I), , 04, Motion in a Plane, Quick Revision, 1. Scalar Quantity is the physical quantity which, has only magnitude but no direction. It is, specified completely by a single number,, alongwith the proper unit., e.g. Temperature, mass, length, time, work, etc., 2. Vector Quantity is the physical quantity, which has both magnitude and direction and, obeys the triangle/ parallelogram laws of vector, addition and subtraction., e.g. Displacement, acceleration, velocity,, momentum, force, etc., 3. Representation of Vector A vector is, represented by a bold face type or by, an arrow placed over a letter,, ®, , ® ®, , i.e. F , a, b or F , a , b ., The length of the line gives the magnitude and, the arrowhead gives the direction., 4. Types of Vectors Vectors are classified into, two types polar and axial vectors., ● Polar Vectors Vectors which have a starting, point or a point of application are called polar, vectors. e.g. Force, displacement, etc., ● Axial Vectors Vectors which represent, the rotational effect and act along the axis of, rotation are called axial vectors., e.g. Angular velocity, angular momentum,, torque, etc., , 5. Modulus of a Vector The magnitude of a, vector is called modulus of vector. For a, vector A, it is represented by | A | or A., 6. Unit Vector It is a vector having unit, $, magnitude. A unit vector of A is written as A., It is expressed as, $ = A =A, A, | A| A, $, or, A = AA, In cartesian coordinates, $i, $j and k$ are the unit, vectors along X -axis, Y -axis and Z -axis., It has no unit or dimensions., 7. Equal Vectors Two vectors are said to be, equal, if they have equal magnitude and same, direction., 8. Resultant Vector It is the combination of, two or more vectors and it produces the same, effect as two or more vectors collectively, produce., Two cases for resultant vectors are as follows, Case I When two vectors are acting in, the same direction, A, B, , Resultant vector, R = A + B
Page 62 :
CBSE New Pattern ~ Physics 11th (Term-I), , 51, , Case II When two vectors are acting in, mutually opposite directions, A, ●, , B, , Resultant vector, R = A – B, (i) If B > A, then direction of R is along B., (ii) If A > B, then direction of R is along A., 9. Addition of Two Vectors (Graphical Method), Two vectors can be added, if both of them are, of same nature. Graphical method of addition, of vectors helps us in visualising the vectors, and the resultant vector., This method contains following laws, ●, Triangle Law of Vector Addition This, law states that, if two vectors can be, represented both in magnitude and direction, by two sides of a triangle taken in the same, order, then their resultant is represented, completely, both in magnitude and direction,, by the third side of the triangle taken in the, opposite order., Addition, , R=, , B, A, , ●, , N, , θ, , O, , B, , B, Addition, , A, , O, , θ, , T, , R, , A, , Q, , B, A+, P, , A, , According to this law,, OT = OP + PQ + QS + ST, \ Resultant vector, R = A + B + C + D, 10. Properties of Addition of Vectors, ● It follows commutative law,, i.e. A + B = B + A, It follows associative law,, , ●, , It follows distributive law,, l( A + B ) = l A + l B, , A+0=A, 11. Subtraction of Two Vectors, (Graphical Method) If a vector B is to be, subtracted from vector A, then we have to, invert the vector B and then add it with vector, A, according to laws of addition of two vectors., Hence, the subtraction of vector B from a vector, A is expressed as R = A + ( - B ) = A - B, ●, , B, , B, Subtraction, , B, A+, , A, , B, , C, , C, , B, O, , B, , R=, α, , +, , +, , ( A + B) + C = A + ( B + C ), , According to triangle law of vector addition,, ON = OM + MN, Resultant vector, R = A + B, Parallelogram Law of Addition of Two, Vectors This law states that, if two vectors, are acting on a particle at the same time be, represented in magnitude and direction by, two adjacent sides of a parallelogram drawn, from a point, their resultant vector is, represented in magnitude and direction by, the diagonal of the parallelogram drawn, from the same point., C, , S, , D, , ●, , M, , A, , B, , O, , B, A+, , The resultant vector formed in this method is, also same as that formed in triangle law of, addition. i.e. Resultant vector, R = A + B, Polygon Law of Addition of Vectors This, law states that, when the number of vectors, are represented in both magnitude and, direction by the sides of an open polygon, taken in an order, then their resultant is, represented in both magnitude and direction, by the closing side of the polygon taken in, opposite order., Consider a number of vectors A, B, C and D, be acting in different directions as shown, , A, A, , –B, , R=, , A, , A, , –B
Page 63 :
52, , CBSE New Pattern ~ Physics 11th (Term-I), , 12. Properties of Subtraction of Vectors, ● Subtraction of vectors does not follow, commutative law, A-B¹B-A, ● It does not follow associative law, A - ( B - C ) ¹ ( A - B) - C, ● It follows distributive law, l( A - B ) = l A - l B, 13. Resolution of Vectors in Plane, (In Two-Dimensions) The process of splitting, a single vector into two or more vectors in, different directions which collectively produce, the same effect as produced by the single, vector alone is known as resolution of a vector., The various vectors into which the single, vector is splitted are known as component, vectors., Any vector r can be expressed as a linear, combination of two unit vectors $i and $j at right, angle, i.e. r = x i$ + y $j., P (x,y), , j, , O, , Y, , Ay, γ, , β, , Ay, , A, α, , Ax, , X, Az, , Az, Ax, l2 +m2+n2=1, , Z, , Remember that, cos a =, cos b =, cos g =, , Ax, A + A 2y + Az2, 2, x, , Ay, A + A 2y + Az2, 2, x, , Az, Ax2 + A 2y + Az2, , =n, , Q, R, , i, , \Magnitude of resultant vector = | r | = x 2 + y 2, If q is the inclination of r with X-axis, then, æy ö, angle, q = tan -1 ç ÷., èx ø, 14. Resolution of a Space Vector, (In Three-Dimensions) We can resolve a, general vector A into three components along, X, Y and Z-axes in three dimensions (i.e., space). While resolving we have,, Ax = A cos a ,, A y = A cos b, Az = A cos g, \ Resultant vector,, A = A $i + A $j + A k$, x, , =m, , Here, l, m and n are known as direction, cosines of A., 15. Addition of Vectors (Analytical Method), According to triangle law of vector addition,, the resultant ( R ) is given by OQ but in opposite, order., , r, , θ, , =l, , y, , z, , Magnitude of vector A is A = Ax2 + A y2 + Az2, , O, , B, θ, , β, A, , P, , N, , Resultant, R = A 2 + B 2 + 2 AB cos q, and direction of resultant R,, B sin q, tan b =, A + B cos q, Regarding the Magnitude of R, ● When q = 0 °, then R = A + B (maximum), ●, , When q = 90 ° , then R = A 2 + B 2, , When q = 180 °, then R = A - B (minimum), 16. Subtraction of Vectors (Analytical Method), There are two vectors A and B at an angle q. If, we have to subtract B from A, then first invert, the vector B and then add with A as shown in, figure., ●
Page 64 :
CBSE New Pattern ~ Physics 11th (Term-I), B, , Addition, of inverted, B, B, , θ, , A, , –B, , A, R, =, π –θ, A, , –, , B, , The resultant vector is R = A + ( - B ) = A - B, The magnitude of resultant in this case is, R = | R | = A 2 + B 2 + 2 AB cos ( p - q ), or, , R = A 2 + B 2 - 2 AB cos q, , Regarding the magnitude of R, ● When q = 0 °, then R = A - B (minimum), ●, , 53, 19. Vector Product or Cross Product, It is defined as the product of the magnitudes, of vectors A and B and the sine angle between, them., It is represented as, A ´ B = AB sin q n$, where, n$ is a unit vector in the direction of, A ´ B., Cross Product of Two Vectors in Terms of, Their Components, If a = a i$ + a $j + a k$ and b = b $i + b $j + b k$ ,, 1, , then, , 2, , 3, , 1, , $j, , k$, , a ´ b = a1, , a2, , a3, , b1, , b2, , b3, , When q = 90 °, then R = A 2 + B 2, , When q = 180 °, then R = A + B (maximum), 17. Dot Product or Scalar Product It is defined, as the product of the magnitudes of vectors A, and B and the cosine angle between them. It is, represented by, A × B = AB cos q, Case I When the two vectors are parallel,, then q = 0°. We have, ●, , A × B = AB cos 0 ° = AB, , A × B = AB cos 90 ° = 0, Case III When the two vectors are anti-parallel,, then q = 180° . We have, A × B = AB cos 180 ° = - AB, 18. Properties of Dot Product, 2, ● a × a = (a), ● a × b = b× a, ● a × ( b + c) = a × b + a × c, ● a × b = | a | | b | cos q, = a 1b 1 + a 2b 2 + a 3b 3, where, a = a i$ + a $j + a k$ ,, 1, , and, , 2, , 3, , b = b 1$i + b 2$j + b 3k$, , Here, $i × $i = $j × $j = k$ × k$ = 1, i$ × $j = $j × k$ = k$ × i$ = 0, , 3, , = (a 2b 3 - a 3b 2 )$i - (a 1b 3 - a 3b 1 ) $j + (a 1b 2 - a 2b 1 ) k$, where, $i ´ i$ = $j ´ $j = k$ ´ k$ = 0, and, , $i ´ $j = k$ , $j ´ k$ = i$, k$ ´ $i = $j,, $j ´ i$ = - k$ , k$ ´ $j = - i$, i$ ´ k$ = - $j, , 20. Properties of Cross Product, ● a ´ b = - b ´ a, ● a ´ ( b + c) = a ´ b + a ´ c, ●, , Case II When the two vectors are mutually, perpendicular, then, q = 90 °. We have, , 2, , $i, , (a ´ b ) + (c ´ d ) = (a ´ c ) + (a ´ d ) + ( b ´ c ), + ( b ´ d), , ma ´ b = a ´ mb, ( b + c) a = b ´ a + c ´ a, ● a ´ a = 0, ● a ´ ( b - c) = a ´ b - a ´ c, 2, 2, 2, 2, ● | a ´ b| = | a | |b| - | a × b|, ● a ´ ( b ´ c) = ( c × a) b - ( b × a) c, 21. Position Vector A vector that extends from a, reference point to the point at which particle is, located is called position vector., ●, ●, , y, , B, j, y^, O, , P, r, x^, i, , A, , x, , For a particle at point P , its position vector,, r = x i$ + y $j
Page 65 :
54, , CBSE New Pattern ~ Physics 11th (Term-I), , In three-dimensions, the position vector is, represented as r = x i$ + y $j + z k$, , ●, , 22. Displacement Vector This vector represent, the straight line joining the initial and final, positions of a particle., It does not depends on the actual path, undertaken by the particle between the two, positions., y, y2, y1, , A ∆r, r2, x1, , O, , 24. Acceleration The rate of change of velocity, of a body w.r.t. time is called acceleration. It is, of two types as given below, ● Average Acceleration It is defined as the, ratio of change in velocity ( Dv ) and the, corresponding time interval ( Dt ). It can be, expressed as, , B, , r1, , x2, , x, , Displacement vector, AB, Dr = ( x 2 - x 1 ) i$ + ( y 2 - y 1 ) $j, , a av =, , Similarly, in three-dimensions, the, displacement vector can be represented as, Dr = ( x - x ) i$ + ( y - y )$j + (z - z ) k$, 2, , 1, , 2, , 1, , 2, , 1, , 23. Velocity Rate of change of displacement of a, body w.r.t. time is called velocity. It is of two, types as given below, ● Average Velocity It is defined as the ratio, of the net displacement and the, corresponding time interval., net displacement, Thus, average velocity =, time taken, Average velocity,, Dr r2 - r1 Dx $ Dy $, =, i+, j, v av =, =, Dt, t2 - t1, Dt, Dt, Velocity can be expressed in the component, form as, v = v i$ + v $j, x, , Instantaneous Velocity The velocity at an, instant of time (t ) is known as instantaneous, velocity., The average velocity will become, instantaneous, if Dt approaches to zero. The, instantaneous velocity is expressed as, Dr d r d, v i = lim, =, = ( x i$ + y $j ), Dt ® 0 D t, dt dt, , y, , where, v x and v y are the components of, velocity along x-direction and y-direction,, respectively., The magnitude of v is given by, | v | = v x2 + v 2y, and the direction of v is given by angle q, æv y ö, = tan -1 ç ÷, èvx ø, , ●, , change in velocity Dv v 2 - v 1, =, =, t2 - t1, time taken, Dt, , Instantaneous Acceleration It is defined, as the limiting value of the average, acceleration as the time interval approaches, to zero., Dv d v, It can be expressed as, a i = lim, =, Dt ® 0 D t, dt, Instantaneous acceleration, a = a i$ + a $j, i, , x, , y, , In terms of x and y, a x and a y can be, expressed as, dv, ax = x, dt, dv y, and, ay =, dt, The magnitude of instantaneous acceleration is, given by, a i = a x2 + a 2y, æa y ö, Direction of acceleration, q = tan –1 ç ÷, èax ø, 25. Motion in a Plane with Uniform, Velocity Consider an object moving with, uniform velocity v in xy-plane. Let r (0 ) and, r (t ) be its position vectors at t = 0 and t = t,, respectively., r (t ) - r (0 ), Then ,, v=, t -0
Page 66 :
CBSE New Pattern ~ Physics 11th (Term-I), Þ, r ( t ) = r ( 0 ) + vt, 26. Motion in a Plane with Constant, Acceleration For a body moving with, uniform acceleration, we have, v - v0, Þ v = v0 + a t, a=, t -0, In terms of rectangular components, we can, express it as, v x = v 0x + a x t, and, v y = v0y + a yt, 1, Also, r (t ) = r (0 ) + v 0 + at, 2, 27. Relative Velocity in Two-Dimensions The, relative velocity of an object A w.r.t. object B,, when both are in motion, is the rate of change, of position of object A w.r.t. object B., Suppose two objects A and B are moving with, velocities v A and v B w.r.t. ground or the earth., Then, relative velocity of object A w.r.t. object, B,, v AB = v A - v B, Relative velocity of object B w.r.t. object A,, v BA = v B - v A, Clearly, v AB = - v BA, and, | v AB | = | v BA |, 28. Projectile Motion It is a form of motion in, which an object or a particle is thrown with, some initial velocity near the earth’s surface, and it moves along a curved path under the, action of gravity alone. The path followed by a, projectile is called its trajectory. e.g.,, ● A tennis ball or a baseball in a flight., ● A bullet fired from a rifle., 29. Equation of Path of a Projectile Suppose at, any time t 1, the object reaches at point P ( x , y )., Y, u cos θ, , u, u sin θ, , P(x, y), , x, , y, O, , H, , θ, , u cos θ, Angle of projection, R, , B, , X, , 55, , ●, , ●, , Position of the object at time t along, horizontal direction is given by, 1, x = x 0 + ux t + ax t2, 2, Position of the object at any time t along the, vertical direction i.e. OY is, ö 2, æ1, g, y = x tan q - ç, ÷x, 2, 2, è 2 u cos q ø, , This equation represents a parabola and is, known as equation of trajectory of a, projectile., 30. Time of Flight It is defined as the total time, for which projectile is in flight, i.e. time during, the motion of projectile from O to B. It is, denoted by T ., 2 u sin q, Time of flight, T =, g, Time of flight consist of two parts such as, ●, Time taken by an object to go from point O, to H . It is also known as time of ascent (t)., ● Time taken by an object to go from point H, to B. It is also known as time of descent (t)., 31. Maximum Height of a Projectile It is, defined as the maximum vertical height, attained by an object above the point of, projection during its flight. It is denoted by H., Maximum height,, u 2 sin 2 q, H =, 2g, 32. Horizontal Range of a Projectile The, horizontal range of the projectile is defined as, the horizontal distance covered by the, projectile during its time of flight. It is denoted, by R and is given as, R = u cos q ´ T, u 2 sin 2q, or, R=, g, The horizontal range will be maximum, if, q = 45°., \ Maximum horizontal range,, u2, Rm =, g
Page 67 :
56, 33. Uniform Circular Motion When an object, follows a circular path at a constant speed,, the motion of the object is called uniform, circular motion., e.g.,, ● Motion of the tip of the second hand of, a clock., ● Motion of a point on the rim of a wheel, rotating uniformly., 34. Terms Related to Circular Motion, ●, Angular Displacement It is defined as, the angle traced out by the radius vector at, the centre of the circular path in the given, time. It is denoted by Dq and expressed in, radians. It is a dimensionless quantity., ●, Angular Velocity It is defined as the, time rate of change of its angular, displacement. It is denoted by w and is, measured in radians per second. Its, dimensional formula is [M 0 L0 T -1 ]. It is a, vector quantity., Dq, It is expressed as w =, ., Dt, ● Angular Acceleration It is defined as, the time rate of change of angular velocity, of a particle. It is measured in radian per, second square and has dimensions, [M 0 L0 T -2 ]., , CBSE New Pattern ~ Physics 11th (Term-I), , ●, , ●, , ●, , ●, , ●, , Time Period It is defined as the time taken, by a particle to complete one revolution along, its circular path. It is denoted by T and is, measured in second., Frequency It is defined as the number of, revolutions completed per unit time. It is, denoted by f and is measured in Hz., Relation between Time Period and, Frequency, 1, Time period, T =, f, Relation between Angular Velocity,, Frequency and Time Period, q 2p, Angular velocity, w = =, = 2 pf, t, T, Relation between Linear Velocity (v ) and, Angular velocity ( w), Linear velocity, v = r, , Dq, =r w, Dt, , 35. Centripetal Acceleration The acceleration, associated with a uniform circular motion and, whose direction is towards the centre of the path, is called centripetal acceleration., v2, Centripetal acceleration, a =, r
Page 68 :
CBSE New Pattern ~ Physics 11th (Term-I), , 57, , Objective Questions, Multiple Choice Questions, 1. In order to describe motion in two or, three dimensions, we use, (a) positive sign, (c) negative sign, , (b) vectors, (d) Both (b) and (c), , 7. A and B are two inclined vectors. R is, their sum., Choose the correct figure for the given, description., , 2. If length and breadth of a rectangle are, 1 m and 0.5 m respectively, then its, perimeter will be a, (a) free vector, (c) localised vector, , (a) O, , B, R=, , (b) scalar quantity, (d) Neither (a) nor (b), , A+, , 3. Consider the quantities, pressure,, power, energy, impulse, gravitational, potential, electrical charge,, temperature, area. Out of these, the, only vector quantities are, , P, , A, , P, , (c) O, , B, A+, , B, , impulse, pressure and area, impulse and area, area and gravitational potential, impulse and pressure, , 4. Suppose an object is at point P at time t, moves to P ¢ and then comes back to P ., Then, displacement is a, (a) unit vector, (c) scalar, , (b) null vector, (d) None of these, , 5. The relation between the vectors A and, - lA is that,, (a), (b), (c), (d), , both have same magnitude, both have same direction, they have opposite directions, None of the above, , 6. Choose the correct option regarding the, given figure., , B, , Q, P, , (d) O, , B, A+, , Q, , B, , Q, , 8. Find the correct option about vector, subtraction., (a) A - B = A + B, (c) A - B = A + (- B), , (b) A + B = B - A, (d) None of these, , 9. A is a vector with magnitude A, then, the unit vector a$ in the direction of, vector A is, (b) A× A, , (a) AA, , (c) A ´ A (d), , A, A, , 10. Unit vector in the direction of the, , resultant of vectors A = - 3$i - 2$j - 3k$, and B = 2$i + 4 $j + 6 k$ is, (a), , (c), , - 3$i + 2 $j - 3k$, 14, $, - i + 2 $j + 3 k$, 14, , (b) - $i + 2$j + 3 k$, (d) - 2 $i - 4 $j + 8 k$, , 11. If A = B + C have scalar magnitudes of, , A, =, , B, , 5, 4, 3 units respectively, then the angle, between A and C is, , –2, A, , (a) B = A, (c) | B | = | A |, , A+, , A, R=, , (NCERT Exemplar), , (a), (b), (c), (d), , B, , Q, , A, , R=, , (b) O, R=, , B, , P, , A, , (b) B = - A, (d) | B | ¹ | A |, , (a) cos- 1 (3/5), (c) p /2, , (b) cos- 1 (4 /5), (d) sin- 1 (4 /5)
Page 69 :
58, , CBSE New Pattern ~ Physics 11th (Term-I), , 12. For two vectors A and B,, , | A + B | = | A - B | is always true, when, (a) | A | = | B | ¹ 0, (b) | A | = | B | ¹ 0 and A and B are parallel or, anti-parallel, (c) either | A | or | B | is zero, (d) None of the above, , 13. Two equal vectors have a resultant, equal to either of the two. The angle, between them is, (a) 90°, (c) 120°, , (b) 60°, (d) 0°, , 14. Consider a vector A that lies in, xy-plane. If A x and A y are the, magnitudes of its x and y -components, respectively, then the correct, representation of A can be given by, y, A, , (a) A sin θ j, , θ, A cos θ î, , O, , x, , 16. Magnitude of a vector Q is 5 and, magnitude of its y-component is 4. So,, the magnitude of the x-component of, this vector is, (a) 8, (c) 6, , (b) 3, (d) 9, , 17. Three vectors are given as, , P = 3$i - 4 $j, Q = 6 $i - 8 $j and, R = ( 3/ 4 ) $i - $j, then which of the, following is correct?, (a) P, Q and R are equal vectors, (b) P and Q are parallel but R is not parallel, (c) P, Q and R are parallel, (d) None of the above, , 18. A vector is inclined at an angle 60° to, the horizontal. If its rectangular, component in the horizontal direction, is 50 N, then its magnitude in the, vertical direction is, (a) 25 N, (c) 87 N, , (b) 75 N, (d) 100 N, , 19. The angle q between the vector, , y, , p = $i + $j + k$ and unit vector along, (b) A sin θ j, O, , A, , X -axis is, , θ, A cos θ î, , x, , y, , (c) A cos θ j, , A, , θ, O, A sin θ î, , æ 1 ö, (a) cos- 1 ç, ÷, è 3ø, æ 3ö, (c) cos- 1 ç, ÷, è 2 ø, , æ 1 ö, (b) cos- 1 ç, ÷, è 2ø, æ1ö, (d) cos- 1 ç ÷, è2ø, , 20. Two vectors P and Q are inclined at an, x, , angle q and R is their resultant as, shown in the figure., , (d) None of the above, , 15. The component of a vector r along, , Q, R, , X-axis will have maximum value if, , q, , (NCERT Exemplar), , (a), (b), (c), (d), , r is along positive Y-axis, r is along positive X-axis, r makes an angle of 45° with the X-axis, r is along negative Y-axis, , O, , a, P, , Keeping the magnitude and the angle of, the vectors same, if the direction of P, and Q is interchanged, then their is a
Page 70 :
CBSE New Pattern ~ Physics 11th (Term-I), , 59, y, , change in which of the following with, regard to R?, (a), (b), (c), (d), , Magnitude, Direction, Both magnitude and direction, None of the above, , P′, , ∆y, , P, , ∆r, r′, , r, , 21. It is found that A + B = A . This, (a), (b), (c), (d), , | B| =0, A, B are parallel, A, B are perpendicular, A; B £ 0, , where, coordinates of P is (4, 3) and P ¢, is (7, 6). Net displacement of the, particle will be, , 22. The sides of a parallelogram are, , represented by vectors p = 5$i - 4 $j + 3k$, $ Then, the area of, and q = 3$i + 2$j - k., the parallelogram is, , (a) 684 sq. units, (c) 171 sq. units, , (b) 72 sq. units, (d) 72 sq. units, , 23. If a × b = | a ´ b | , then the angle q, between a and b will be, (a) 60°, , (b) 45°, , (c) 75°, , (b) 7$i + 9 $j, (d) 3$i + 3$j, , (a) zero, (c) 10$i + 18 $j, , 26. A particle moves in xy-plane from, positions ( 2 m, 4 m) to (6 m, 8 m) is 2 s., Magnitude and direction of average, velocity is, (a) 2 ms-1 and 45°, (c) 4 2 ms-1 and 30°, , (b) 2 2 ms-1 and 45°, (d) 3 2 ms-1 and 60°, , 27. The direction of instantaneous velocity, (d) 90°, , is shown by, , 24. Position vector r of a particle P located, in a plane with reference to the origin, of an xy-plane as shown in the figure, below is given by, , n of, ctio, Dire, P2, , Y, , P, , (a), , v, , (b), , ∆r2, , r, , O, , X, , Y, , P, , c, Dire, P, , (c), , tion, , fv, tion o, Direc, ∆r3, P3, P, , Y, , r2, , r, , y, , 4 units, , x, , ∆x, , O, , necessarily implies, , r1, , O, , X, , of v, , (d) None of these, , r, , r, O, , (a) 2 $i + 4 $j, (c) 6 k$, , 2 units, , O, , x, , (b) 4 $i + 2 $j, (d) $i + $j + 4 k$, , 25. Suppose a particle moves along a curve, shown by the thick line and the, positions of particle are represented by, P at t and P ¢ at t ¢., , X, , 28. The position of a particle is given by, , r = 3t $i + 2t 2 $j + 5k$ , then the direction of, v (t) at t = 1s in, , (a), (b), (c), (d), , 45° with X-axis, 63° with Y-axis, 30° with Y-axis, 53° with X-axis
Page 71 :
60, , CBSE New Pattern ~ Physics 11th (Term-I), , 29. In three dimensional system, the, position coordinates of a particle (in, motion) are given below, x = a cos wt, y = a sin wt, z = awt, The velocity of particle will be, (b) 2 aw, (d) 3 aw, , (a) 2 aw, (c) aw, , 30. The coordinates of a moving particle at, any time t are given by, x = 2t 3 and, y = 3t 3 . Acceleration of the particle is, given by, (a) 468 t, , (b) t 468, , (c) 234 t 2 (d) t 234, , 31. A particle starts from origin at t = 0, , with a velocity 5.0 $i ms -1 and moves in, xy-plane under action of force which, produces a constant acceleration of, ( 3.0 $i + 2.0 $j) ms -2 . What is the, y-coordinate of the particle at the, instant, if x-coordinate is 84 m?, (a) 36 m, (c) 39 m, , (b) 24 m, (d) 18 m, , 32. A car driver is moving towards a fired, , rocket with a velocity of 8$i ms -1 . He, observed the rocket to be moving with, a speed of 10 ms -1 . A stationary, observer will see the rocket to be, moving with a speed of, (a) 5 ms -1, (c) 7 ms -1, , (b) 6 ms -1, (d) 8 ms -1, , 33. A man standing on a road has to hold, his umbrella at 30° with the vertical to, keep the rain away. He throws the, umbrella and starts running at, 10 kmh - 1 ., He finds that raindrops are hitting his, head vertically. The actual speed of, raindrops is, (a) 20 kmh-1, (c) 20 3 kmh-1, , (b) 10 3 kmh-1, (d) 10 kmh-1, , 34. A girl can swim with speed 5 kmh -1 in, still water. She crosses a river 2 km, wide, where the river flows steadily at, 2 kmh -1 and she makes strokes normal, to the river current. Find how far down, the river she go when she reaches the, other bank., (a) 1 km, (c) 800 m, , (b) 2 km, (d) 750 m, , 35. When a ball is thrown obliquely from, the ground level, then the x-component, of the velocity, (a), (b), (c), (d), , decreases with time, increases with time, remains constant, zero, , 36. The motion of an object that is in flight, after being projected is a result of two, simultaneously occurring components, of motion, which are the components in, (a) horizontal direction with constant, acceleration, (b) vertical direction with constant, acceleration, (c) horizontal direction without acceleration, (d) Both (b) and (c), , 37. At the top of the trajectory of a, projectile, the directions of its velocity, and acceleration are, (a), (b), (c), (d), , parallel to each other, antiparallel to each other, inclined to each other at an angle of 45°, perpendicular to each other, , 38. A projectile is given an initial velocity, of ( $i + 2$j) ms -1 , where $i is along the, ground and $j is along vertical. If g is, 10 ms -2 , then the equation of its, trajectory is, (a), (b), (c), (d), , y = x - 5 x2, y = 2x - 5 x2, 4y = 2x - 5 x2, 4 y = 2 x - 25 x 2
Page 72 :
CBSE New Pattern ~ Physics 11th (Term-I), , 39. The equations of motion of a projectile, , are given by x = 18t and 2y = 54t - 9.8t 2 ., The angle q of projection is, (a) tan-1 (3), æ2ö, (c) sin-1 ç ÷, è 3ø, , (b) tan-1 (15, .), -1 æ 2 ö, (d) cos ç ÷, è 3ø, , 40. A football player throws a ball with a, , 61, , 45. Given below figure show three paths of, a rock with different initial velocities., The correct increasing order for the, respective initial horizontal velocity, component (ignoring the effect of air, resistance) is, Y, , -1, , velocity of 50 ms at an angle 30°, from the horizontal. The ball remains in, the air for (Take,g = 10 ms -2 ), (a) 2.5 s, (c) 5 s, , (b) 1.25 s, (d) 0.625 s, , 1, , 41. The ceiling of a hall is 30 m high. A, , ball is thrown with 60 ms -1 at an angle, q, so that it could reach the ceiling of, the hall and come back to the ground., The angle of projection q that the ball, was projected is given by, 1, 8, 1, (c) sinq =, 3, (a) sinq =, , (b) sinq =, , 1, 6, , (d) None of these, , 42. Two projectiles A and B are projected, with same speed at angles 30° and 60° to, be horizontal then, which amongst the, following relation between their range,, maximum height and time of flight is, wrong?, (a) RA = RB, (c) TB = 3TA, , (b) H B = 3H A, (d) None of these, , 43. A man can throw a stone to a, maximum distance of 80 m. The, maximum height to which it will rise, is, (a) 30 m, (c) 10 m, , (b) 20 m, (d) 40 m, , 44. Two stones were projected, simultaneously in the same vertical, plane from same point obliquely, with, different speeds and angles with the, horizontal. The trajectory of path, followed by one, as seen by the other, is, (a) parabola, (c) circle, , (b) straight line, (d) hyperbola, , 2, , 3, , O, , (a) 1 < 2 < 3, (c) 2 < 1 < 3, , X, , (b) 3 < 2 < 1, (d) 3 < 1 < 2, , 46. What is the centripetal acceleration of a, point mass which is moving on a, circular path of radius 5m with speed, 25 ms -1 ?, (a) 125 ms -2, (c) 60 ms -2, , (b) 90 ms -2, (d) None of these, , 47. The displacement of a particle moving, on a circular path, when it makes 60° at, the centre is, (a) 2 r, (c) 2r, , (b) r, (d) None of these, , 48. If a car is executing a uniform circular, motion, then its centripetal acceleration, represents, (a) a scalar quantity, (b) constant vector, (c) not a constant vector (d) None of these, , 49. A car revolves uniformly in a circle, of diameter 0.80 m and completes, 100 rev min -1 . Its angular velocity is, (a) 10.467 rads -1, (c) 46.26 rads -1, , (b) 0.6 rads -1, (d) 8 rads -1, , 50. If 2 balls are projected at angles 45° and, 60° and the maximum heights reached, are same, then the ratio of their initial, velocities is ……… ., (a) 2 : 3, (c) 3 :2, , (b) 3 : 2, (d) 2 : 3
Page 73 :
62, , CBSE New Pattern ~ Physics 11th (Term-I), , 51. Two projectiles having different masses, , m 1 and m 2 are projected at an angle a, and (90° - a ) with the same speed from, some point. The ratio of their, maximum heights is ……… ., (a) cot a :sin a, (c) tan2 a :1, , (d) None of the above, , 56. Figure shows the orientation of two, vectors u and v in the xy-plane., If u = a $i + b $j and v = p $i + q $j, y, , (b) 1 : 1, (d) 1:tana, , 52. A projectile fired with initial velocity u, , at some angle q has a range R. If the, initial velocity be doubled at the same, angle of projection, then the range will, be ……… ., (a) 2R, (c) R, , (b) R /2, (d) 4R, , 53. Two cars of masses m 1 and m 2 are, moving in circles of radii r1 and r2 ,, respectively. Their speeds are such that, they make complete circles in the same, time t . The ratio of their centripetal, accelerations is …… ., (a) m1 r1 : m2 r2, (c) r1 : r2, , (b) m1 : m2, (d) 1 :1, , 54. Which one of the following statement is, correct?, , (NCERT Exemplar), , (a) A scalar quantity is the one that is, conserved in a process., (b) A scalar quantity is the one that can never, take negative values., (c) A scalar quantity is the one that does not, vary from one point to another in space., (d) A scalar quantity has the same value for, observers with different orientation of the, axes., , 55. For two vectors A and B which lie in a, plane. Which of the following statement, is correct?, (a) If magnitude of A and B vector is 3 and 4 and, they add upto give vector having magnitude, of 5, then they must be perpendicular, vector., (b) If they add up to give more than 5, then they, must be inclined at obtuse angle., (c) If they add upto give less than 5, then they, must be inclined at acute angle., , u, , v, , x, , O, , Which of the following statement is, (NCERT Exemplar), correct?, (a) a and p are positive, while b and q are, negative., (b) a, p and b are positive, while q is negative., (c) a, q and b are positive, while p is negative., (d) a, b, p and q are all positive., , 57. Match the Column I (example of, motion) with Column II (type of, motion) and select the correct answer, from the codes given below., Column I, , Column II, , A., , Free fall, , p., , One-dimensional, motion, , B., , Projectile, motion, , q., , Two-dimensional, motion, , C., , Circular motion, , r., , Three-dimensional, motion, , D., , Motion along a, straight road, , Codes, A, (a) q, (b) p, (c) p, (d) p, , B, p, q, q, r, , C, r, r, q, q, , D, p, q, p, p, , 58. Match the Column I (magnitude of, vectors A and B) with Column II, (relationship between A and B) and, select the correct answer from the codes, given below.
Page 74 :
CBSE New Pattern ~ Physics 11th (Term-I), , Column I, |A| = l, A. ¾ ¾¾¾, ¾®, B | = 2l, ¾|¾¾¾, ¾®, |A| = l, ¾ ¾¾¾, ¾®, B. | B | = l, ¬¾¾¾, |A | = 2 l, C. ¾ ¾¾¾, ¾®, , Column II, p., , A= -B, , q., , A= B, , r., , 2A = B, , D., , s., , A=-2B, , Codes, A B, (a) q s, (c) r, p, , Reason Vector addition is commutative., , 61. Assertion Vector addition of two vectors, , D, q, s, , A, (b) r, (d) q, , B, p, r, , C D, s q, s p, , where a, b and g are the angles made, by A with coordinate axes. Then,, match the Column I with Column II, (respective values) and select the, correct option from the codes given, below., Column I, , Column II, p. cos -1 (1 / 2 ), , a, , -1, , B., , b, , q. cos, , C., , g, , r. cos -1 ( 3 / 5 2 ), , Codes, A B, (a) p q, (c) r, q, , is always greater than their vector, subtraction., Reason At q = 90º, addition and, subtraction of vectors are unequal., , 62. Assertion In case of projectile motion,, C, p, q, , 59. A vector is given by A = 4 $i + 3$j + 5k$ ,, , A., , 60. Assertion Magnitude of resultant of two, vectors may be less than the magnitude of, either vector., , B =l, , ¬¾¾, |A|= l, ¾ ¾¾¾®, | B| = l, ¾ ¾¾¾, ®, , 63, , C, r, p, , A, (b) q, (d) p, , ( 4 / 5 2), , B, r, p, , C, p, q, , Assertion-Reasoning MCQs, For question numbers 60 to 69, two, statements are given-one labelled, Assertion (A) and the other labelled, Reason (R). Select the correct answer to, these questions from the codes (a), (b), (c), and (d) are as given below, (a) Both A and R are true and R is the, correct explanation of A., (b) Both A and R are true but R is not the, correct explanation of A., (c) A is true but R is false., (d) A is false and R is also false., , the magnitude of rate of change of, velocity is variable., Reason In projectile motion, magnitude, of velocity first increases and then, decreases during the motion., , 63. Assertion At highest point of a, projectile, dot product of velocity and, acceleration is zero., Reason At highest point, velocity and, acceleration are mutually perpendicular., , 64. Assertion A particle is projected with, , speed u at an angle q with the horizontal., At any time during motion, speed of, particle is v at angle a with the vertical,, then v sin a is always constant throughout, the motion., Reason In case of projectile motion,, magnitude of radial acceleration at, topmost point is minimum., , 65. Assertion For projection angle tan -1 ( 4 ),, the horizontal and maximum height of a, projectile are equal., Reason The maximum range of projectile, is directly proportional to square of, velocity and inversely proportional to, acceleration due to gravity., , 66. Assertion The range of a projectile is, maximum at 45°., Reason At q = 45°, the value of sin q is, maximum.
Page 75 :
64, , CBSE New Pattern ~ Physics 11th (Term-I), , 67. Assertion Sum of maximum height, , for angles a and 90°-a is independent, of the angle of projection., Reason For angles a and 90°-a, the, , 68. Assertion The maximum height of, projectile is always 25% of the, maximum range., Reason For maximum range, projectile, should be projected at 90°., , 69. Assertion Uniform circular motion is, uniformly accelerated motion., Reason Kinematic equations for, uniform acceleration motion can be, applied in the case of uniform circular, motion., , Case Based MCQs, Direction Answer the questions from, 70-74 on the following case., Vectors, Vectors are the physical quantities which have, both magnitudes and directions and obey the, triangle/parallelogram laws of addition and, subtraction. It is specified by giving its, magnitude by a number and its direction., e.g. Displacement, acceleration, velocity,, momentum, force, etc. A vector is, represented by a bold face type and also by, an arrow placed over a letter, i.e., ® ® ®, , F, a, b or F , a , b., The length of the line gives the magnitude, and the arrowhead gives the direction., The point P is called head or terminal point, and point O is called tail or initial point of the, vector OP., N, E, S, , which is not a vector quantity?, (a) Force, (c) Temperature, , (b) Acceleration, (d) Velocity, , 71. Set of vectors A and B, P and Q are as, , horizontal range R is different., , W, , 70. Amongst the following quantities,, , Tail, , Head, , O, , P, , shown below, A, , X¢, , X, Y, , B, O, O, , P, Q, O, , Y¢, , O, , Length of A and B is equal, similarly, length of P and Q is equal. Then, the, vectors which are equal, are, (a) A and P, (c) A and B, , (b) P and Q, (d) B and Q, , 72. | l A| = l |A| , if, (a) l > 0, (c) l = 0, , (b) l < 0, (d) l ¹ 0, , 73. Among the following properties, regarding null vector which is, incorrect?, (a) A + 0 = A, (c) 0A = 0, , (b) l 0 = l, (d) A - A = 0, , 74. The x and y components of a position, vector P have numerical values 5 and 6,, respectively. Direction and magnitude, of vector P are, æ6ö, (a) tan-1 ç ÷ and 61, è5ø, , æ5ö, (b) tan-1 ç ÷ and 61, è6ø, , (c) 60° and 8, , (d) 30° and 9, , Direction Answer the questions from, 75-79 on the following case., Relative Velocity, , Every motion is relative as it has to be, observed with respect to an observer. Relative, velocity is a measurement of velocity of an, object with respect to other observer. It is, defined as the time rate of change of relative, position of one object with respect to another.
Page 76 :
CBSE New Pattern ~ Physics 11th (Term-I), , For example, if rain is falling vertically with a, velocity v r and a man is moving horizontally, with v m , the man can protect himself from the, rain if he holds his umbrella in the direction, of relative velocity of rain w.r.t. man., , 75. Two bodies are held separated by 9.8 m, vertically one above the other. They are, released simultaneously to fall freely, under gravity. After 2 s, the relative, distance between them is, (a) 4.9 m, , (b) 19.6 m, , (c) 9.8 m (d) 39.2 m, , 76. If two objects P and Q move along, parallel straight lines in opposite, direction with velocities v P and v Q, respectively, then relative velocity of P, w.r.t. Q ,, (a) v PQ = v P = v Q, (c) v P + v Q, , (b) v P - v Q, (d) v Q - v P, , 77. A train is moving towards East and a, car is along North, both with same, speed. The observed direction of car to, the passenger in the train is, , 78. Buses A and B are moving in the same, direction with velocities 20 $i ms - 1 and, 15$i ms - 1 , respectively. Then, relative, velocity of A w.r.t. B is, (b) 5 $i ms- 1, (d) 35 $jms- 1, , 79. A girl riding a bicycle with a speed of, -1, , 5 ms towards east direction sees, raindrops falling vertically downwards., On increasing the speed to 15 ms -1 ,, rain appears to fall making an angle of, 45° of the vertical. Find the magnitude, of velocity of rain., (a) 5 ms- 1, (c) 25 ms- 1, , Direction Answer the questions from, 80-84 on the following case., Projectile Motion, , Projectile motion is a form of motion in which, an object or particle is thrown with some, initial velocity near the earth’s surface and it, moves along a curved path under the action, of gravity alone. The path followed by a, projectile is called its trajectory, which is, shown below. When a projectile is projected, obliquely, then its trajectory is as shown in, the figure below, Y, , H, P (x, y), , x, u sin θ, u, O, , θ, u cos θ, , u cos θ, g, , y, A, , B, , X, , Here velocity u is resolved into two, components, we get (a) u cos q along OX and, (b) u sin q along OY ., , 80. The example of such type of motion is, , (a) East-North direction, (b) West-North direction, (c) South-East direction, (d) None of the above, , (a) 35 $i ms- 1, (c) 5 $jms- 1, , 65, , (b) 5 5 ms- 1, (d) 10 ms- 1, , (a) motion of car on a banked road, (b) motion of boat in sea, (c) a javelin thrown by an athlete, (d) motion of ball thrown vertically upward, , 81. The acceleration of the object in, horizontal direction is, (a) constant, (b) decreasing, (c) increasing, (d) zero, , 82. The vertical component of velocity at, point H is, (a) maximum, (b) zero, (c) double to that at O, (d) equal to horizontal component, , 83. A cricket ball is thrown at a speed of, 28 m/s in a direction 30° with the, horizontal.
Page 77 :
66, , CBSE New Pattern ~ Physics 11th (Term-I), , The time taken by the ball to return to, the same level will be, (a) 2.0 s, (c) 4.0 s, , (b) 3.0 s, (d) 2.9 s, , 84. In above case, the distance from the, thrower to the point where the ball, returns to the same level will be, (a) 39 m, (c) 68 m, , (b) 69 m, (d) 72 m, , Direction Answer the questions from, 85-89 on the following case., Uniform Circular Motion, When an object follows a circular path at a, constant speed, the motion of the object is, called uniform circular motion. The word, uniform refers to the speed which is uniform, (constant) throughout the motion. Although, the speed does not vary, the particle is, accelerating because the velocity changes its, direction at every point on the circular track., The figure shows a particle P which moves, along a circular track of radius r with a, uniform speed u., O, , a, r, , u, P, , 85. A circular motion, (a) is one-dimensional motion, (b) is two-dimensional motion, (c) it is represented by combination of two, variable vectors, (d) Both (b) and (c), , 86. For a particle performing uniform, circular motion, choose the incorrect, statement from the following., (a) Magnitude of particle velocity (speed), remains constant., (b) Particle velocity remains directed, perpendicular to radius vector., (c) Direction of acceleration keeps changing as, particle moves., (d) Angular momentum is constant in, magnitude but direction keeps changing., , 87. Two cars A and B move along a, concentric circular path of radius rA and, rB with velocities v A and v B maintaining, v, constant distance, then A is equal to, vB, (a), (c), , rB, rA, r, r, , 2, A, 2, B, , (b), (d), , rA, rB, r 2B, r A2, , 88. A car runs at a constant speed on a, circular track of radius 100 m, taking, 62.8 s for every circular lap. The, average velocity and average speed for, each circular lap, respectively is, (a), (b), (c), (d), , 0, 0, 0, 10 ms -1, 10 ms-1 , 10 ms-1, 10 ms-1 , 0, , 89. A particle is revolving at 1200 rpm in a, circle of radius 30 cm. Then, its, acceleration is, (a) 1600 ms-2, (c) 2370 ms-2, , (b) 4740 ms-2, (d) 5055 ms-2
Page 78 :
CBSE New Pattern ~ Physics 11th (Term-I), , 67, , ANSWERS, Multiple Choice Questions, 1. (b), 11. (a), 21. (a), , 2. (b), 12. (c), 22. (a), , 3. (b), 13. (c), 23. (b), , 4. (b), 14. (a), 24. (a), , 5. (c), 15. (b), 25. (d), , 6. (d), 16. (b), 26. (b), , 7. (d), 17. (c), 27. (c), , 8. (c), 18. (c), 28. (d), , 9. (d), 19. (a), 29. (a), , 10. (c), 20. (b), , 31. (a), 41. (b), , 32. (b), 42. (d), , 33. (a), 43. (b), , 34. (c), 44. (b), , 35. (c), 45. (a), , 36. (d), 46. (a), , 37. (d), 47. (b), , 38. (b), 48. (c), , 39. (b), 49. (a), , 51. (c), , 52. (d), , 53. (c), , 54. (d), , 55. (a), , 56. (b), , 57. (c), , 58. (b), , 59. (b), , 62. (d), , 63. (a), , 64. (c), , 65. (b), , 66. (c), , 67. (c), , 68. (c), , 69. (d), , 72. (a), 82. (b), , 73. (b), 83. (d), , 74. (a), 84. (b), , 75. (c), 85. (d), , 76. (c), 86. (c), , 77. (b), 87. (b), , 78. (b), 88. (b), , 79. (b), 89. (b), , 30. (b), 40. (c), 50. (b), , Assertion-Reasoning MCQs, 60. (b), , 61. (d), , Case Based MCQs, 70. (c), 80. (c), , 71. (c), 81. (d), , SOLUTIONS, 1. In order to describe two dimensional or three, dimensional motions, we use vectors., However, direction of the motion of an, object along a straight line is shown by, positive and negative signs., , 2. The perimeter of the rectangle would be the, sum of the lengths of the four sides, i.e. 1.0 m, + 0.5 m + 1.0 m + 0.5 m = 3.0 m., Since, length of each side is a scalar, thus the, perimeter is also a scalar., , 7. Vectors obey the triangle law of addition,, according to which, if vector B is placed with, its tail at the head of vector A. Then, when, we join the tail of A to the head of B. The, line OQ represents a vector R, i.e. the sum of, the vectors A and B. Thus, figure given in, option (d) is correct., , 8. To subtract B from A, we can add – B and A., So, A + ( - B) = A - B = R2 . This is as shown, below, , 3. We know that, impulse J = F × Dt = Dp, where, , 5. Multiplying a vector A by a negative number, l gives a vector lA, whose direction is, opposite to the direction of A and its, magnitude is - l times | A |., , 6. | B | = - 2 | A |. So, when A is multiplied by, - 2 , then its direction gets reversed and, magnitude would be 2 times | A|., Thus, | B | ¹ | A|., , B, , (a), , B, , positions coincides, so the displacement will, be zero. Thus, it is a null vector., , –B, , –B, , A+, , 4. In the given case, the initial and final, , –B, =A, R2, A, , A, B, =, R1, , F is force, Dt is time duration and Dp is, change in momentum. As Dp is a vector, quantity, hence impulse is also a vector, quantity. Sometimes area can also be treated, as vector., , (b), , Hence, option (c) is correct about vector, subtraction., A, A, 9. Unit vector of A is a$ =, =, | A| A, , 10. Resultant vector of A and B is, R = A + B = ( - 3$i - 2$j - 3k$ ), + ( 2$i + 4 $j + 6 k$ ), = - $i + 2$j + 3k$, | R | = ( - 1) 2 + ( 2) 2 + ( 3) 2
Page 79 :
68, , CBSE New Pattern ~ Physics 11th (Term-I), , Þ, Þ, Þ, Þ, , = 1 + 4 + 9 = 14, Unit vector in the direction of R is, R, - $i + 2$j + 3k$, R$ =, =, | R|, 14, , 11. Here, triangle OMN with its adjacent sides as, , cos q = - 1/ 2, - cos q = 1/ 2, cos (180° - q) = cos 60°, q = 120°, , 14. Vector along X-axis (x-component), = A x i$ = | A| cos q$i, = A cos qi$, , vectors A, B and C are shown below, N, θ, , 5, , =, |A|, , O, , Vector along Y-axis (y-component), = A $j = | A| sin q$j, , |C|=3, , y, , = A sin qj$, , M, , |B|=4, , 15. Let r makes an angle q with positive x-axis,, so the component of r along X-axis, rx = r cos q, ( rx ) maximum = r (cos q) maximum, = r cos 0° = r, (Q cos q is maximum of q = 0°), At q = 0°, r will be along positive X-axis., , MN, As,, cos q =, ON, | C|, æ 3ö, = cos -1 ç ÷, Þ q = cos - 1, è 5ø, | A|, , 12. Given, A + B = A - B, Þ, , 2, , A, , =, Þ, , A, , 2, , 2, , + B, , A, , + B, = A, , 2, 2, , +2 A, 2, , + B, , +2 A, + B, , 2, , B cos q, 2, , -2 A, , 16. Given, | Q | = 5, Q y =4, Q x =?, , B cos q, , B cos q, -2 A, , As,, , B cos q, , Þ 4 A B cos q = 0, Þ, A B cos q = 0, Þ, A =0, or, B =0, or, cos q = 0, Þ, q = 90°, Thus, | A + B | = | A - B | is always true, when, either | A| or | B | is zero or A and B are, perpendicular to each other., , 13. Let two vectors are A and B, inclined at an, angle q., Resultant of the two vectors A and B,, | R | = | A |2 + | B |2 + 2 | A | | B | cos q, , |Q| = Q, , 2, x, , + Q, , 2, y, , Þ, | Q |2 = Q x2 + Q y2, Substituting the given values, we get, ( 5) 2 = Q x2 + 4 2, Þ, , Q, , x, , =, , 9 = 3, , 17. Given, P = 3$i - 4 $j, and Q = 6 i$ - 8 $j = 2( 3i$ - 4 $j) = 2P, 3, 1, P, Also, R = $i - $j = ( 3$i - 4 $j) =, 4, 4, 4, So, P, Q and R are parallel with unequal, magnitude., Thus, they are not equals vectors., , …(i), , 18. Given, vector can be shown below as, Y, , Let, | A | = | B | = a, According to the question, | R | = a, From Eq. (i),, Þ, Þ, , A, , Ay, , a = a + a + 2aa cos q, 2, , 2, , a 2 = a 2 + a 2 + 2a 2 cos q, 2a 2 cos q = - a 2, , θ, 0, , Ax, , X
Page 80 :
CBSE New Pattern ~ Physics 11th (Term-I), , 69, , q = 60°, Ay, Then, tan q =, Ax, or, A y = A x tan q, Þ, A y = 50 tan 60° = 50 ´ 3, ), (Q 3 = 1732, ., = 86.6 ~, - 87 N, where,, , 19. Given,, , p = i$ + $j + k$, , æ 1 ö, q = cos - 1 ç, ÷, è 3ø, , vectors is unchanged, so the magnitude of the, resultant R will be same. However, the, direction of R will get changed., 2, , 21. Given that, | A + B| = | A| or A + B = A, 2, , + B, , 2, , +2 A, , B cos q = A, , a ´ b = $i ( 4 - 6 ) - $j ( - 5 - 9 ) + k$ (10 + 12), a ´ b = - 2i$ + 14 $j + 22k$, , Thus, | a ´ b| = ( 2) 2 + (14 ) 2 + ( 22) 2, = 684 sq. units, , 23. Given, a × b = | a ´ b|, , Þ, Þ, Þ, , ab cos q = ab sin q, (Q a × b = ab cos q and a ´ b = ab sin q), sin q ab, =, =1, cos q ab, tan q = 1, tan q = tan 45°, , \, , q = 45°, , 24. Position vector r of an object in xy-plane at, , 20. Since, the magnitude and angle between the, , Þ A, , Þ, , Þ, , and unit vector along X-axis, x = i$., So, the angle q between them can be, determine by, p× x, ( i$ + $j + k$ ) × ( i$ ), 1, cos q =, =, =, 2, 2, 2, 2, | p | | x|, 3, 1 +1 +1 × 1, \, , Þ, , 2, , Given, x = 2 units and y = 4 units., So, position vector at P will be r = 2$i + 4 $j., , 25. Position vector of the particle at P ,, , 2, , where, q is angle between A and B., Þ B ( B + 2 A cos q) = 0, B = 0 or B + 2 A cos q = 0, Þ, B, Þ cos q = 2 A, , point P with its components along X and, Y-axes as x and y, respectively is given as, r = xi$ + y$j., , r = 4 i$ + 3$j, Position vector of the particle at P ¢,, r ¢ = 7 $i + 6 $j, ...(i), , \ Displacement of the particle is Dr = r ¢- r, Þ, Dr = ( 7 $i + 6 $j) - ( 4 i$ + 3$j), , If A and B are anti-parallel, then q = 180°., Hence, from Eq. (i),, B, cos 180° = - 1 = Þ B =2A, 2A, Hence, the given condition can only be, implied of either B = 0 or A and B are, anti-parallel provided B = 2 A ., , 22. Area of a parallelogram = | a ´ b|, where, a and b are sides of parallelogram., Given, a = p = 5$i - 4 $j + 3k$, and, \, , b = q = 3$i + 2$j - k$, $i, $j, a ´ b= 5 - 4, 3, , 2, , k$, 3, -1, , = ( 7 - 4 ) $i + ( 6 - 3) $j = 3$i + 3$j, , 26. Displacement,, Dr = r2 - r1 = 4 i$ + 4 $j, Dr 4 i$ + 4 $j, vav =, =, = 2( i$ + $j) ms -1, \, Dt, 2, Þ Magnitude of velocity,, | vav | = 2 12 + 12 = 2 2 ms -1, Direction,, æ Dv y ö, -1 æ 2 ö, -1, q = tan -1 ç, ÷ = tan ç ÷ = tan 1 = 45°, è 2ø, è Dv x ø, , 27. The direction of instantaneous velocity at any, point on the path of an object is tangential to, the path at that point and is in the direction, of motion. Also, direction of average velocity, is same as that of Dr.
Page 81 :
70, , CBSE New Pattern ~ Physics 11th (Term-I), , So, amongst the given figures we can say, that, options (a) and (b) are depicting the, direction of averge velocity but option (c) is, correctly depicting the direction of, instantaneous velocity., 28. Given, r = 3t i$ + 2t 2$j + 5k$, dr d, = ( 3t $i + 2t 2 $j + 5k$ ) = 3$i + 4t $j, dt dt, At t = 1 s,, v = 3$i + 4 $j, , \ v( t ) =, , æv y ö, æ 4ö, Thus, its direction is q = tan -1 ç ÷ = tan -1 ç ÷, è 3ø, èvx ø, @ 53° withX -axis, , 29. Given that the position coordinates of a, particle, x = a cos wt ü, ï, y = a sin wt ý, ï, z = awt, þ, , …(i), , So, the position vector of the particle is, $r = x $i + y $j + z k$, Þ, , r$ = a cos wt $i + a sin wt $j + a wt k$, r$ = a [cos wt $i + sin wt $j + wt k$ ], , The magnitude of velocity is, | v | = v x2 + v 2y + v z2, or | v | = ( -aw sin wt ) 2 + ( aw cos wt ) 2 + ( aw) 2, = wa ( - sin wt ) + (cos wt ) + (1), = 2 wa, , 30. Given, x = 2t, , 3, , dx, \, vx =, = 6t 2, dt, dv, Þ, a x = x = 12t, dt, Also, y = 3t 3, dy, Þ, vy =, = 9t 2, dt, dv y, Þ, ay =, = 18t, dt, , 2, , 31. Given, initial velocity of the particle at, t = 0 s,, v0 = 5.0 i$ ms-1 , acceleration,, a = ( 3.0 i$ + 2.0 $j) ms-2, The position of the particle is given by, 1, r( t ) = v0 t + at 2, 2, = 5.0 $i t + (1/ 2)( 3.0 $i + 2.0 $j)t 2, = ( 5.0t + 1.5t 2 ) $i + 1.0t 2 $j, , …(i), , As, r( t ) = x ( t ) $i + y( t ) $j, , …(ii), , Comparing Eqs. (i) and (ii), we get, x ( t ) = 5.0t + 1.5t 2 and y ( t ) = + 1.0t 2, Given,, x ( t ) = 84m, Þ, 5.0t + 1.50 t 2 = 84, or 1.50 t 2 + 5.0t - 84 = 0, Solving the above quadratic equation, the, value of t is given as, t=, , Therefore, the velocity of the particle is, d r d [a ] [cos wt $i + sin wt $j + wt k$ ], Q, v$ =, =, dt, dt, Þ, v$ = - aw sin wt $i + aw co swt $j + aw k$ ), , 2, , \ Acceleration, a = a x2 + a 2y = t 468, , 2, , =, , -b ± b 2 - 4ac, 2a, -5 ±, , 52 - 4(1.50 ) ( -84 ), 2(1.50 ), , 25 + 504 -5 ± 529 -5 ± 23, =, =, 3, 3, 3, or, =6, - 9.33, (Neglecting the negative values as t can never, be negative), Þ t =6 s, =, , -5 ±, , At t = 6 s,, , y = 1.0( 6 ) 2 = 36 m, , 32. The velocity of car driver = 8 $i ms -1, Velocity of rocket = v y $j ms -1, Relative velocity of rocket w.r.t. car = 8 $i - v y $j, Since, the speed of the rocket observed by, the car driver is 10 ms -1 ., \ ( v y ) 2 + ( 8 ) 2 = (10 ) 2, v 2y = 100 - 64 = 36, Þ, v y = 6 ms -1, Velocity of rocket, v y $j = (6$j) ms -1, \ Relative speed of rocket w.r.t. a stationary, observer = 6 – 0 = 6 ms –1
Page 82 :
CBSE New Pattern ~ Physics 11th (Term-I), , 33. When the man is at rest with respect to the, ground, the rain comes to him at an angle, 30° with the vertical. This is the direction of, the velocity of raindrops with respect to the, ground., vm, g, , 30°, , vr, m, , vr, g, , Here, vr, g = velocity of the rain with, respect to the ground,, vm, g = velocity of the man with, respect to the ground = 10 kmh -1, and vr, m = velocity of the rain with respect, to the man., 10, vr, g =, = 20 kmh -1, \, sin30°, , 34. Given, speed of girl, v g = 5 km h -1, Speed of river, v r = 2 km h -1, Width of river, d = 2 km, The given condition is as shown in the figure, below, B vr, , d, , vg, , C, , α, A, , Since, the girl dive the river normal to the, flow of the river, time taken by the girl to, cross the river, so, d, 2 km, 2, t=, =, = h, v g 5 kmh -1 5, In this time, the girl will go down the river, by the distance AC due to river current., \ Distance travelled along the river, 2, = vr ´ t = 2 ´, 5, 4, 4000, = km =, m = 800 m, 5, 5, 35. After the object has been projected, the, x-component of the velocity remains constant, throughout the motion and only the, , 71, y-component changes, like an object in, free-fall in vertical direction., 36. An object that is in flight after being thrown, or projected is called a projectile. The motion, of projectile may be thought of as the result, of two separate, simultaneously occurring, components of motions. One component, along a horizontal direction without any, acceleration and the other along the vertical, direction with constant acceleration due to, the force of gravity., 37. Velocity is in horizontal direction and, acceleration is vertical downwards. Therefore,, the direction of velocity and acceleration of, the projectile are perpendicular to each other., , 38. Given, initial velocity,, u = ( $i + 2$j ) ms -1, Magnitude of velocity,, u = (1) 2 + ( 2) 2 = 5 ms -1, Equation of trajectory of projectile,, gx 2, y = x tan q - 2, 2u cos 2 q, gx 2 sec 2 q, = x tan q 2u 2, 2, gx, = x tan q - 2 (1 + tan 2 q), 2u, [Q sec 2 q = 1 + tan 2 q], Substituting the given values, we get, 10( x ) 2, y =x ´2\, [1 + ( 2) 2 ], 2( 5 ) 2, uy 2, é, ù, êQ tan q = u = 1 = 2ú, ë, û, x, = 2x -, , 10( x 2 ), (1 + 4 ) = 2x - 5x 2, 2´5, , 39. Given, equations of motion are, x = 18t , 2y = 54t - 9.8t 2, General equations of projectile are, x = u cos q × t and y = u sin q × t -, , 1 2, gt, 2, , where, q is the angle of projection., Comparing it with given equation, we have, 54, u cos q = 18 and u sin q =, 2
Page 83 :
72, , CBSE New Pattern ~ Physics 11th (Term-I), u sin q 54 / 2, =, u cos q, 18, 54, = 1.5 Þ q = tan -1(1.5), \ tan q =, 2 ´ 18, Þ, , 40. Time of flight,, T =, , 2u sin q 2 ´ 50 ´ sin 30°, =, =5s, g, 10, , 41. Given, u = 60 ms -1, Maximum height H that the ball will achieve, = height of ceiling of the hall, = 30 m, u 2 sin 2 q, As, maximum height, H =, 2g, ( 60 ) 2 sin 2 q, Þ, 30 =, 2g, 30 ´ 2g 10, [Q g = 10 ms -2 ], =, 60 ´ 60 60, 1, Þ, sin q =, 6, 1, T A sin 30°, 42. T µ sin q,, or T B = 3 T A, =, =, sin 60°, TB, 3, Þ, , sin 2 q =, , H µ sin 2 q ,, , HB = 3 HA, Rq = R90 ° - q, , \, , RA = RB, , R=, , u 2 sin 2q 2u 2 sin q cos q, =, g, g, , =, , 2( u sin q) ( u cos q) 2ux u y, =, g, g, , Þ R µ horizontal initial velocity component, ( ux ), \ From the given plot, we can see that for, path 3, range is maximum. This implies that, the rock has the maximum horizontal, velocity component in this path. Thus, the, correct order will be 1 < 2 < 3., , 46. Given, speed, v = 25 ms -1, v 2 25 ´ 25, =, 5, r, , 47. In the figure, AB is the required displacement, , As, range of a projectile, R =, , u sin 2q, g, , 44. Velocities of the stones at some instant t can, be given as, v1 = u1 cos q1 i$ + ( u1 sin q1 - gt ) $j, and v = u cos q i$ + ( u sin q - gt ) $j, 2, , 2, , of the particle., In triangle OAB, OA = OB and Ð AOB = 60°, A, , 2, , and it is maximum q = 45°, u2, = 80 m, \, g, u 2 sin 2 q, Maximum height, h =, 2g, 80, =, (sin 2 45° ), 2, 1, = 40 ´ = 20 m, 2, , 2, , 45. Range of a projectile,, , = 125 ms -2, , Rmax = 80 m, , 2, , = constant, Since, their relative velocity is constant., So, the trajectory of path followed by one as, seen by other will be straight line, making a, constant angle with horizontal., , Centripetal acceleration, a c =, , 43. Given, maximum horizontal range,, , 2, , + ( u1 sin q1 - u2 cos q2 ) $j, , and radius, r = 5 m, , H A sin 2 30° 1, =, =, HB, sin 2 60° 3, , or, As,, , Relative velocity,, v1 - v2 = ( u1 cos q1 - u2 cos q2 ) $i, , r, 60°, r, O, , Displacement, B, , Therefore, DAOB is an equilateral triangle, so, OA = OB = r = AB, , 48. For a uniform circular motion,, centripetal acceleration, a c =, , v2, R, , Since, v and R are constants, the magnitude, of the centripetal acceleration of the car is, also constant.
Page 84 :
CBSE New Pattern ~ Physics 11th (Term-I), , However, the direction changes pointing, towards the centre. Therefore, a centripetal, acceleration is not a constant vector., diameter 0.80 m, 49. Radius, r =, =, = 0.40 m, 2, 2, 100, rev s -1, Frequency, n = 100 rev min -1 =, 60, 1 60, Time period, T = =, = 0.60, n 100, 2p 2 ´ 314, ., =, \ Angular velocity, w =, T, 0.60, = 10.467 rad s, , -1, , 50. Given, H 1 = H 2, Þ, , v 1 r1, …(i), =, v 2 r2, v2 r, r2 r r, i.e. a c1 : a c 2 = 1 ´ 22 = 12 ´ 2 = 1 = r1 : r2, r1 v 2, r2 r1 r2, [from Eq. (i)], Þ, , 54. A scalar quantity is independent of direction, hence has the same value for observers with, different orientations of the axes., Hence, the statement given in option (d) is, correct, rest are incorrect., , 55. Since, ( 5) 2 = ( 3) 2 + ( 4 ) 2, which is in, accordance to Pythagoras theorem. So, the, vectors can be shown in the figure, , u12 sin 2 45° u22 sin 60°, =, 2g, 2g, (4) B, , 3 /2, u, sin 60°, \ 1 =, =, = 3: 2, u2 sin 45° (1/ 2 ), , 51. Maximum height, H =, , u 2 sin 2 a, 2g, , So, H 1 : H 2 = tan 2 a : 1, u 2 sin 2q, g, , \ A and B are perpendicular., However, if the length of A + B vector is, more than or less than 5, then they should be, inclined at acute and obtuse angle,, respectively., Thus, the statement given in option (a) is, correct, rest are incorrect., , 56. Clearly from the given figure,, , \ R µ u2, If initial velocity be doubled at same angle of, projection, then range will become four, times., , 53. As, centripetal acceleration is given as, v2, r, v2, For the first body of mass m 1 , a c1 = 1, r1, ac =, , For the second body of mass m 2, a c 2, , A+B (5), , A (3), , For same speed of projection,, H µ sin 2 a, H1, sin 2 a, sin 2 a, = tan 2 a, \, =, =, 2, H 2 sin ( 90° - a ), cos 2 a, , 52. R =, , 73, , v2, = 2, r2, , Also, time taken by both the cars to complete, one revolution is same., Hence, T 1 = T 2, 2pr1 2pr2, Þ, =, v1, v2, , u is in the first quadrant, hence both, components a and b will be positive., For v = p i$ + q $j, as it is in positive x-direction, and located downward hence x-component p, will be positive and y-component q will be, negative., , 57. The correct sequence is, Hence, A ® p, B ® q, C ® q and D ® p., , 58. A. As, | B | = 2 | A | and they both are in the, same direction, so 2A = B., B. As, | A | = | B | but both are in opposite, directions, so A = - B., C. As, | A | = 2 | B | but both are in opposite, directions, so A = - 2B., D. As, | A| = | B | and both are in same, direction, so A = B., , Hence, A ® r, B ® p, C ® s and D ® q.
Page 85 :
74, , CBSE New Pattern ~ Physics 11th (Term-I), , 59. Magnitude of A = | A| = A x2 + A 2y + A z2, = ( 4 ) 2 + ( 3) 2 + ( 5) 2, = 16 + 9 + 25 = 5 2, Angles made by A with coordinate axes,, 4, A, cos a = x =, | A| 5 2, æ 4 ö, a = cos -1 ç, ÷, è 5 2ø, , or, , cos b =, , Ay, | A|, , =, , cos g =, , Az, | A|, , 5, 5 2, , =, , velocity,, 1, 2, , æ 1 ö, g = cos -1 ç, ÷, è 2ø, , or, , 60. Resultant of two vectors A and B is given as, R = A 2 + B 2 + 2AB cos q, \ We can say that, (i) If q is an obtuse angle, then magnitude of, R will be less than magnitude of the either, vectors A or B., e.g. if | A| = 4, | B | = 3 and q = 120°, then, | R| = 4 + 3 + 2 ´ 4 ´ 3 cos (120° ), 2, , = 25 - 12 = 13, 1ö, æ, çQ cos 120° = - ÷, è, 2ø, \, | R| < | A|, (ii) If the vectors are in opposite direction and, are equal in magnitude, then also the, magnitude of R will be less than the, magnitude of either vectors A or B., e.g. If | A | = | B | = a (say) and q = 180°, then,, , | R| = a 2 + a 2 - 2a 2 cos(180° ), = 2a 2 - 2a 2, , dv, = | a | = 9.8 ms -2 = constant, dt, , Also, in case of projectile motion, the, magnitude of velocity first decreases and then, increases during the motion., Therefore, A is false and R is also false., , 63. Velocity is horizontal and acceleration is, , Hence, A ® q, B ® r and C ® p., , 2, , So, for example, when 90° < q < 270°,, | A + B| < | A - B|, Thus, vector addition of two vectors is not, always greater than their vector subtraction., Also, at q = 90°, | A + B | = | A - B |, = A2 + B2, , 62. In projectile motion, rate of change of, , 5 2, , =, , | A - B | = A 2 + B 2 - 2AB cos q, , Therefore, A is false and R is also false., , 3, , æ 3 ö, b = cos -1 ç, ÷, è 5 2ø, , or, , 61. | A + B | = A 2 + B 2 + 2AB cos q, , [Q cos 180° = - 1], , \, | R | < | A| or | B|, Also, vector addition is commutative in, nature., A+ B=B+ A, Therefore, both A and R are true but R is, not the correct explanation of A., , vertical. i.e. both are perpendicular to each, other, hence their dot product is zero., Therefore, both A and R are true and R is, the correct explanation of A., 64. Horizontal component of velocity = v sin a =, constant, a r = g 2 - a t2, At highest point a t = 0. Therefore, a r is, maximum., Therefore, A is true but R is false., u 2 sin 2 q 2u 2 sin q cos q, 65. H = R or, =, g, 2g, Þ, , tan q = 4, , Maximum horizontal range (at q = 45°) is, u2, given by Rmax =, g, Therefore, both A and R are true but R is, not the correct explanation of A., u 2 sin 2q, 66. Horizontal range, R =, g, At q = 45°, sin 2 q = 1, u2, \ Rmax =, = maximum range, g, Q sin q = 1 (maximum), at q = 90°, Therefore, A is true but R is false.
Page 86 :
CBSE New Pattern ~ Physics 11th (Term-I), , u 2 sin 2 a, 2g, u 2 sin 2( 90° - a ) u 2 cos 2 a, =, H2 =, 2g, 2g, , 67. Maximum height, H 1 =, and, , Þ H1 + H2 =, , u2, u2, (sin 2 a + cos 2 a ) =, 2g, 2g, , Thus, the sum of height for angles a and, 90° - a is independent of the angle of, projection., u 2 sin 2q, As, horizontal range, R =, g, So, for same value of initial velocity,, horizontal range of projectile is same for, complementary angles., Therefore, A is true but R false., , 68. To obtain maximum range, angle of, projection must be 45°, i.e. q = 45°., u 2 sin 2 ´ 45° u 2 sin 90° u 2, …(i), So, Rmax =, =, =, g, g, g, 2, , u 2 sin 2 45° u 2 æ 1 ö u 2 Rmax, =, =, ÷ =, ç, 2g, 2g è 2 ø 4 g, 4, [from Eq. (i)], is 25% of Rmax ., , \ H max =, So, H max, , Therefore, A is true but R is false., , 69. In uniform circular motion the velocity of the, object is changing continuously in direction,, the object undergoes uniform acceleration, which is not a constant vector. However, for, a uniformly accelerated motion, the, acceleration of the object should be constant., Hence, it is not an example of uniformly, accelerated motion., Kinematic equations for constant acceleration, is not applicable for uniform circular motion., Since, in this case the magnitude of, acceleration is constant but its direction is, changing., Therefore, A is false and R is also false., 70. Temperature is not a vector quantity because, it has magnitude only., However, force, acceleration and velocity, have both a magnitude and a direction. So,, these are vectors in nature., 71. Two vectors are said to be equal, if and only, if they have the same magnitude and, direction., , 75, Among the given vectors A and B are equal, vectors as they have same magnitude (length), and direction., However, P and Q are not equal even though, they are of same magnitude because their, directions are different., , 72. | l A | = l | A | , if l > 0, as multiplication of, vector A with a positive number l gives a, vector whose magnitude is changed by the, factor l but the direction is same as that of A., , 73. Null vector 0 is a vector, whose magnitude is, zero and its direction cannot be specified. So,, it means, |0| = 0., Thus, l0 = 0., Hence, property given in option (b) is, incorrect., 74. Let P be as shown in the y, figure, then according to, the given information, Py P, Px = 5, P y = 6, \, , θ, , | P | = Px2 + P y2, , Px, , x, , = 25 + 36, Þ, , | P | = 61, , and tan q =, , Py, Px, , =, , 6, Þ q = tan -1, 5, , æ6ö, ç ÷, è 5ø, , 75. Since, they are following freely, so both the, bodies will fall same distance in same time, interval., So, the relative separation between them will, remain unchanged., , 76. Relative velocity of P w.r.t. Q is given by, v PQ = v P - ( - vQ ) = v P + vQ, , 77. Velocity of car w.r.t. train, v ct = v c - v t, Þ, , v ct = v c + ( - v t ), vct, , N, , vc, , 45°, W, , −vt, , vt, , E, , Velocity of car w.r.t. train ( v ct ) is towards, West-North.
Page 87 :
76, , CBSE New Pattern ~ Physics 11th (Term-I), , 78. Given, vA = 20 i$ ms -1, vB = 15i$ ms -1, Relative velocity of A w.r.t. B,, vAB = vA - vB = 20 i$ - 15i$ = 5$i ms -1, , 79. Given, velocity of girl, v g = 5$i ms -1, Let velocity of rain, v r = v x $i + v y $j ms -1, Relative velocity of rain, = v r - v g = ( v x - 5) $i + v y $j, , r = a cos wt $i + a sin wt $j, Both the components a cos wt i$ and a sin wt $j, are perpendicular to each other., , 86. If a particle is performing uniform circular, motion, then its, (a) speed will be constant throughout the, motion., (b) velocity will be tangential in the direction, of motion at a particular point., C, , v -5, Now, it is vertical, so tan q = x, =0, vy, …(i), Þ vx - 5 = 0 Þ vx = 5, On increasing the speed of the girl, relative, velocity becomes ( v x - 15) $i + v y $j, tan q = tan 45° =, , v x - 15, =1, vy, , [using Eq. (i)], Þ v x - 15 = v y Þ v y = -10, -1, $, $, \ Velocity of rain = ( 5i - 10 j) ms, Þ Magnitude of velocity of rain, = ( 5) 2 + (10 ) 2, = 125 = 5 5 ms-1, , 80. A javelin thrown by an athlete is an example, of projectile motion., , 81. The horizontal component of velocity, (u cos q) is constant throughout the motion, so, there will be no acceleration in horizontal, direction., , 82. As the vertical components of velocity, (u sin q) decreases continuously with height,, from O to H, due to downward force of, gravity and becomes zero at H ., , 83. The time taken by the ball to return to the, same level,, 2v sin q 2 ´ 28 ´ sin 30°, T = 0, =, » 2.9 s, g, 9.8, , 84. The distance from the thrower to the point, where the ball returns to the same level is, v 2 sin 2q 28 ´ 28 ´ sin 60°, R= 0, =, » 69 m, g, 9.8, , 85. Circular motion is an example of, two-dimensional motion with radius vector as, , B, D, , O, r, A, , v, , v2, (c) acceleration, a =, will always be towards, r, centre of the circular path., (d) angular momentum (mvr) is constant in, magnitude but direction keeps on, changing., , 87. Angular velocity w is constant., v =rw, vA, r, = A, \ v µ r or, vB, rB, , 88. On a circular path in completing one turn,the, distance travelled is 2pr, while displacement, is zero., Hence, average velocity, displacement 0, =, = =0, time interval t, Distance, Average speed =, Time interval, 2pr 2 ´ 3.14 ´ 100, =, =, = 10 ms -1, t, 62.8, 1200, rps, 89. Given, n = 1200 rpm =, 60, 30, m, r = 30 cm =, 100, Acceleration of the particle = Centripetal, acceleration = w2r = ( 2pn ) 2r, 2, , 22 1200 ö, 30, æ, » 4740 ms -2, = ç2 ´, ´, ÷ ´, ø, è, 7, 60, 100
Page 88 :
CBSE New Pattern ~ Physics 11th (Term-I), , 77, , 05, Laws of Motion, Quick Revision, 1. Momentum Momentum of a body is the, quantity of motion possessed by the body. It, is defined as the product of its mass m and, velocity v and is denoted by p., Momentum, p = m v, 2. Conservation of Momentum According, to this principle, “In the absence of an, external force, the total momentum of a, system remains constant or conserved and, does not change with time”., If S Fext = 0, then momentum p = constant., 3. Equilibrium of a Particle The forces, acting at the same point or on a particle are, called concurrent forces., These forces are said to be in equilibrium,, n, , when their resultant is zero, i.e., , åF, , i, , = 0., , i = 1, , 4. Lami’s Theorem According to this, theorem, when three concurrent forces F1,, F2 and F3 acting on a body are in, equilibrium, then, F1, F, F, = 2 = 3, sin a sin b sin g, F1, , F2, , γ, β, , α, F3, , 5. Tension When a body of mass m is fastened with, the string, then the weight of the body acts, downwards while a force acting just opposite to, the downward force for balancing it is called, tension., T, mg, , T = mg, where, g = acceleration due to gravity, and, T = tension in the string., 6. Friction Whenever a body moves or tends to, move over the surface of another body, a force, comes into play which acts parallel to the surface, of contact and opposes the relative motion., This opposing force is called friction., 7. Types of Friction, ● Static Friction Force of friction which comes, into play between two bodies, before one body, actually starts moving over the other is called, static friction and it is denoted by f s ., ●, , Limiting Friction Maximum value of static, friction which comes into play when a body just, starts moving over the surface of another body is, called limiting friction., Thus, f s £ f s (max), The value of limiting static friction f s (max), between two given surfaces is directly
Page 89 :
78, , CBSE New Pattern ~ Physics 11th (Term-I), , N, θ, Applied, force, F, , fs, , Mg, , 9. Angle of Repose The minimum angle of, inclination of a plane with the horizontal, such, , m, ax, , that the body placed on the plane just starts to, slide down is known as angle of repose., (f, s), , R, , θ, Mg cosθ, , θ, s in, M, g, , proportional to the normal reaction (R ), between the two surfaces., i.e., f s (max) µ R, f s (max), Þ, f s (max) = m s R Þ m s =, R, The proportionality constant m s is called, coefficient of static friction., ● Kinetic Friction Kinetic friction or, dynamic friction is the opposing force that, comes into play when one body is actually, moving over the surface of another body., Thus, kinetic friction opposes the relative, motion. The value of kinetic friction f k is, given as, f, or, f k = mk R Þ mk = k, R, The proportionality constant mk is called, coefficient of kinetic friction., When the relative motion has begun, the, acceleration of the body on the rough, surface is given by, F - fk, a=, m, where, F = applied force and f k = kinetic, friction., ● Rolling Friction Friction which comes into, play when a body like a ring or a sphere rolls, without slipping over a horizontal surface, is, known as rolling friction., 8. Angle of Friction The angle between the, resultant of limiting friction f s and normal, reaction N with the direction of N is called, angle of friction q., , Mg, , θ, Angle of repose, , 10. Centripetal Force When an object moves on, a circular path, a force acts on it, whose, direction is towards the centre of the path, this, force is called centripetal force., Centripetal force acting on a particle of mass m, on a circular path of radius r is given by, mv 2, F =, r, 11. Motion of a Car on Level Road When a car, of mass m is turning on the level road without, skidding, centripetal force on the car must be, equal or less than static friction., 2, mv max, i.e., F ³, r, 2, mv max, or, mg ³, r, (m = coefficient of friction), or, , v max £ m × rg, , \ Maximum velocity on a curved road to, avoid skidding is v max = mrg ., 12. Motion of a Car on Banked, Road Maximum velocity of a car on banked, road is given by, æ m + tan q ö, v max = rg ç, ÷, è1 - m tan q ø, where, q = inclination of road, and, r = radius of turn., If m = 0, then v = rg tan q .
Page 90 :
CBSE New Pattern ~ Physics 11th (Term-I), , 79, , Objective Questions, Multiple Choice Questions, 1. According to Galileo’s experiment for, a double inclined plane that are, smooth, when a ball is released from, rest on one of the planes rolls down and, climb up the other of decreased slope,, the final height of the ball is, (a), (b), (c), (d), , less than the initial height, more than the initial height, equal to the initial height, more or less than the initial height, , (a) bigger ball transfers greater momentum, than smaller, (b) bigger ball transfers lesser momentum than, smaller, (c) bigger ball transfer equal momentum as, smaller, (d) None of the above, , 7. A rocket is going upwards with, , explain the concept of inertia?, , accelerated motion. A man sitting in it, feels his weight increased 5 times his, own weight. If the mass of the rocket, including that of the man is 1. 0 ´ 10 4 kg,, how much force is being applied by, rocket engine? (Take, g = 10 ms -2 )., , (a) First law, (c) Third law, , (a) 5 ´ 104N, (c) 5 ´ 108 N, , 2. Which of the Newton’s laws of motion, (b) Second law, (d) All of these, , 3. If a running bus stops suddenly, our, feet stop due to friction, but the rest of, the body continues to move forward, due to, (a) momentum, , (b) force, , (c) inertia, , (d) impulse, , 4. Suppose the earth suddenly stops, attracting objects placed near surface. A, person standing on the surface of the, earth will, (a) remain standing, (c) sink into earth, , (b) fly up, (d) either (b) or (c), , 5. When a car is stationary, there is no net, force acting on it. During pick-up, it, accelerates. This happens due to, (a), (b), (c), (d), , net external force, net internal force, may be external or internal force, None of the above, , 6. A smaller and a bigger iron balls are, dropped from a small height on a glass, pane placed on a table. Only bigger, ball breakes the glass pane, because, , (b) 5 ´ 105 N, (d) 2 ´ 104N, , 8. The motion of a particle of mass m is, , described by y = ut + gt 2 , find the force, acting on the particle., (a) Zero, (c) 2 mg, , (b) mg, (d) 3 mg, , 9. A bullet of mass 0.04 kg moving with a, speed of 90 ms -1 enters a heavy, wooden block and stopped after 3s., What is the average resistive force, exerted by the block on the bullet?, (a) 1 N, (c) 2 N, , (b) 1.2 N, (d) 3 N, , 10. A body of mass 6 kg is acted on by a, force so that its velocity changes from, 3 ms -1 to 5 ms -1 , then change in, momentum is, (a) 48 N-s, (c) 30 N-s, , (b) 24 N-s, (d) 12 N-s, , 11. A meter scale is moving with uniform, velocity. This implies, , (NCERT Exemplar), , (a) the force acting on the scale is zero, but a, torque about the centre of mass can act on, the scale
Page 91 :
80, , CBSE New Pattern ~ Physics 11th (Term-I), , (b) the force acting on the scale is zero and the, torque acting about centre of mass of the, scale is also zero, (c) the total force acting on it need not be zero, but the torque on it is zero, (d) Neither the force nor the torque need to be, zero, , 12. While launching a satellite of mass, , 10 kg, a force of 5 ´ 10 5 N is applied for, 20s. The velocity attained by the, satellite at the end of 20s, is, 4, , (a) 4 km/s, (c) 1 km/s, , (b) 3 km/s, (d) 2 km/s, , 13. The momentum p (in kg-ms -1 ) of a, particle is varying with time t (in, second) as p = 2 + 3t 2 . The force acting, on the particle at t = 3 s will be, (a) 18 N, (c) 9 N, , (b) 54 N, (d) 15 N, , 14. A machine gun fires a bullet of mass, , 40 g with a velocity of 1200 ms -1 . The, man holding it can exert a maximum, force of 144 N on the gun., How many bullets can be fired per, second at the most?, (a), (b), (c), (d), , Only one, Three, Can fire any number of bullets, 144 ´ 48, , 15. A cricket ball of mass 150 g has an, , initial velocity u = ( 3i$ + 4$j ) ms -1 and a, final velocity v = - ( 3i$ + 4$j ) ms -1 , after, being hit. The change in momentum, (final momentum – initial momentum), is (in kgms –1 ), (NCERT Exemplar), (a) zero, (c) - (09, . $i + 12, . $j), , (b) - (045, . $i + 0.6 $j), (d) - 5($i + $j) $i, , 16. The force F acting on a particle of mass, m is indicated by the force-time graph, shown below. The change in, momentum of the particle over the time, interval from 0 to 8s is, , 6, 3, F(N), , 0, , 2, , 4, , 6, , 8, , –3, t(s), , (a) 24 N-s, (c) 12 N-s, , (b) 20 N-s, (d) 6 N-s, , 17. A particle of mass m is moving in a, straight line with momentum p. Starting, at time t = 0, a force F = kt acts in the, same direction on the moving particle, during time interval T , so that its, momentum changes from p to 3p., Here, k is a constant. The value of T is, (a), , 2p, k, , (b) 2, , p, k, , (c), , 2k, p, , (d) 2, , k, p, , 18. A constant retarding force of 50 N is, applied to a body of mass 20 kg moving, initially with a speed of 15 ms -1 . How, long time does the body take to stop?, (a) 6 s, , (b) 8 s, , (c) 9 s, , (d) 10 s, , 19. A batsman hits back at ball straight in, the direction of the bowler without, changing its initial speed of 12 ms -1 . If, the mass of the ball is 0.15 kg, find the, impulse imparted to the ball. (Assume, linear motion of the ball), (a) 1.8 N-s (b) 3.6 N-s (c) 3.6 N-m (d) 1.8 N-m, , 20. The force-time ( F -t ) graph for linear, motion of a body initially at rest is, shown in figure. The segments shown, are circular, the linear momentum, gained in 4 s is, F (N), , 2, 0, , 4, 2, , –2, , (a) 8 N-s, , 6, , 8, , t (s), , (b) 4p N-s, , (c) 2p N-s (d) 8p N-s
Page 92 :
CBSE New Pattern ~ Physics 11th (Term-I), , 21. Every action has an equal and opposite, reaction, which suggests that, (a) action and reaction always act on different, bodies, (b) the forces of action and reaction cancel to, each other, (c) the forces of action and reaction cannot, cancel to each other, (d) Both (a) and (c), , 22. An initially stationary device lying on a, frictionless floor explodes into two, pieces and slides across the floor. One, piece is moving in positive x-direction, then other piece is moving in, (a), (b), (c), (d), , positive y-direction, negative y- direction, negative x-direction, at angle from x-direction, , of mass 100 kg. If the muzzle speed of, the shell is 80 m/s, calculate the recoil, speed of the gun., (c) 4 m/s (d) 16 m/s, , 24. In equilibrium of particle when net, external force of the particle is zero., Then, the particle is, (a), (b), (c), (d), , 27. A hockey player is moving northward, and suddenly turns westward with the, same speed to avoid an opponent. The, force that acts on the player is, (NCERT Exemplar), , (a), (b), (c), (d), , frictional force along westward, muscle force along southward, frictional force along south-west, muscle force along south-west, , 28. Three concurrent coplanar forces 1 N,, 2 N and 3 N are acting along different, directions on a body can keep the body, in equilibrium, if, (a), (b), (c), (d), , 2 N and 3 N act at right angle, 1 N and 2 N act at acute angle, 1 N and 2 N act at right angle, Cannot be possible, , 29. Three blocks with masses m , 2m and 3m, , 23. A shell of mass 200 g is fired by a gun, , (a) 16 cm/s (b) 18 m/s, , 81, , are connected by strings, as shown in, the figure. After an upward force F is, applied on block m, the masses move, upward at constant speed v. What is the, net force on the block of mass 2m?, (Take, g is the acceleration due to, gravity), F, m, , at rest, moving with uniform velocity, moving with uniform acceleration, Both (a) and (b), , 2m, , 25. Two forces F1 = 3 $i - 4 $j and, , 3m, , F2 = 2 $i - 3 $j are acting upon a body of, mass 2 kg. Find the force F3 , which, when acts on the body will make it, stable., (a) 5 $i + 7$j, (c) -5 $i + 7 $j, , (b) -5 $i - 7$j, (d) 5 $i - 7$j, , (a) Zero, , (b) 2 mg, , with an angle of 60° between them. If, the resultant force is equal to 40 3 N,, the magnitude of each force is, (b) 20 N, , (c) 80 N, , (d) 30 N, , (c) 3 mg, , (d) 6 mg, , 30. A ball of mass 1 kg hangs in equilibrium, from a two strings OA and OB as shown, in figure. What are the tensions in, strings OA and OB? (Take, g = 10 ms -2 ), A, , B, 30º, , 60º, , 26. Two equals forces are acting at a point, , (a) 40 N, , v, , T1, , 90º T, 2, , 120º O 150º, w = 10 N
Page 93 :
82, , CBSE New Pattern ~ Physics 11th (Term-I), , (a) 5 N, 5 N, (c) 5 N, 5 3 N, , (b) 5 3 N, 5 3 N, (d) 5 3 N, 5 N, , 31. Given figure is the part of a, horizontally stretched structure. Section, AB is stretched with a force of 10 N., The tension in the sections BC and BF ,, are, E, D, 120°, G, , 34. A system consists of three masses, m 1 , m 2 and m 3 connected by a string, passing over a pulley P . The mass, m 1 hangs freely and m 2 and m 3 are on a, rough horizontal table (the coefficient, of friction = m)., The pulley is frictionless and of, negligible mass. The downward, acceleration of mass m 1 is (Assume,, m1 = m 2 = m 3 = m), P, , F, , 90°, , m3, , C, B, 120°, 120°, m1, , A, , (a), (b), (c), (d), , m2, , 10 N, 11 N, 10 N, 6 N, 10 N, 10 N, Cannot be calculated due to insufficient, data, , 32. Find the force exerted by 5 kg block on, floor of lift, as shown in figure., (Take, g = 10 ms -2 ), , g (1 - gm), 9, g(1 - 2 m), (c), 3, , (a), , 2 gm, 3, g(1 - 2 m), (d), 2, (b), , 35. Two masses m 1 = 1 kg and m 2 = 2 kg, , are connected by a light inextensible, string and suspended by means of a, weightless pulley as shown in figure., , 5 ms–2, , 2 kg, , 1 kg m1, , 5 kg, , m2 2 kg, , (a) 100 N, (c) 105 N, , (b) 115 N, (d) 135 N, , 33. Three blocks A, B and C of masses 4 kg,, 2 kg and 1 kg respectively, are in, contact on a frictionless surface, as, shown in the figure. If a force of 14 N is, applied on the 4 kg block, then the, contact force between A and B is, A, , (a) 2 N, , (b) 6 N, , B, , C, , (c) 8 N, , (d) 18 N, , Assuming that both the masses start, from rest, the distance travelled by 2 kg, mass in 2 s is, 20, m, 9, 20, (c), m, 3, , (a), , 40, m, 9, 1, (d) m, 3, (b), , 36. If a box is lying in the compartment of, an accelerating train and box is, stationary relative to the train. What, force cause the acceleration of the box?
Page 94 :
CBSE New Pattern ~ Physics 11th (Term-I), , (a) Frictional force in the direction of train, (b) Frictional force in the opposite direction of, train, (c) Force applied by air, (d) None of the above, , 37. A box of mass 2 kg is placed on the, roof of a car. The box would remain, stationary until the car attains a, maximum acceleration. Coefficient of, static friction between the box and the, roof of the car is 0.2 and g = 10 ms -2 ., The maximum acceleration of the car,, for the box to remain stationary, is, (a) 8 ms-2, (c) 4 ms-2, , (b) 6 ms-2, (d) 2 ms-2, , 38. A car of mass m starts from rest and, acquires a velocity along east,, v = v $i (v > 0 ) in two seconds. Assuming, the car moves with uniform, acceleration, the force exerted on the, (NCERT Exemplar), car is, mv, eastward and is exerted by the car, 2, engine, mv, eastward and is due to the friction on, (b), 2, the tyres exerted by the road, mv, eastward exerted due to the, (c) more than, 2, engine and overcomes the friction of the, road, mv, (d), exerted by the engine, 2, (a), , 39. A particle of mass 2 kg is moving on a, circular path of radius 10 m with a, speed of 5 ms –1 and its speed is, increasing at a rate of 3 ms –1 . Find the, force acting on the particle., (a) 5 N, , (b) 10 N, , (c) 12 N, , (d) 14 N, , 40. Two stones of masses m and 2m are, whirled in horizontal circles, the, r, heavier one in a radius and the lighter, 2, one in a radius r. The tangential speed, , 83, of lighter stone is n times that of the, value of heavier stone, when they, experience same centripetal forces. The, value of n is, (a) 2, , (b) 3, , (c) 4, , (d) 1, , 41. If a car is moving in uniform circular, motion, then what should be the value, of velocity of a car, so that car will not, moving away from the circle?, (a) v < m s Rg, , (b) v £ m s Rg, , (c) v < m k Rg, , (d) None of these, , 42. A person is driving a vehicle at a, , uniform speed of 5 ms -1 on a level, curved track of radius 5 m. The, coefficient of static friction between, tyres and road is 0.1. Will the person, slip while taking the turn with the same, speed? (Take, g = 10 ms -2 ), (a), (b), (c), (d), , A person will slip, if v 2 = 5 m2s-2, A person will slip, if v 2 > 5 m2s-2, A person will slip, if v 2 < 5 m2s-2, A person will not slip, if v 2 > 5 m2s-2, , 43. A circular racetrack of radius 300 m is, banked at an angle of 15°. If the, coefficient of friction between the, wheels of the race car and the road is, 0.2. Find optimum speed of the race car, to avoid wear and tear on its tyres and, maximum permissible speed to avoid, slipping. (Take, g = 9.8 ms -2 and, tan 15° = 0.27), (a), (b), (c), (d), , v o = 48 ms-1 , v max = 60 ms-1, v o = 28.1 ms-1 , v max = 38.1 ms-1, v o = 62.2 ms-1 , v max = 734, . ms-1, None of the above, , 44. A car is moving in a circular horizontal, track of radius 10.0 m with a constant, speed of 10.0 ms - 1 . A plumb bob is, suspended from the roof of the car by a, light rigid rod of length 10.0 m. The, angle made by the rod with the track is, (Take, g = 10 ms - 2 ), (a) zero, , (b) 30°, , (c) 45°, , (d) 60°
Page 95 :
84, , CBSE New Pattern ~ Physics 11th (Term-I), , 45. Inertia of an object is directly, dependent on ……… ., (a) impulse, (c) mass, , (b) momentum, (d) density, , 46. A body with mass 5 kg is acted upon by, a force F = ( -3$i + 4 $j ) N. If its initial, velocity at t = 0 is u = ( 6$i - 12$j )ms -1 , the, time at which it will just have a velocity, along the Y-axis is ……… ., , (NCERT Exemplar), , (a) never, (c) 2 s, , (b) 10 s, (d) 15 s, , 47. If impulse I varies with time t as, , F (kg ms - 1 ) = 20t 2 - 20t . The change, in momentum is minimum at ……… ., , (a) t = 2s, 1, (c) t = s, 2, , (b) t = 1s, 3, (d) t = s, 2, , 48. The force which is dissipative in nature, is ……… ., (a) electrostatic force, (c) gravitational force, , (b) magnetic force, (d) frictional force, , 49. Suppose a light-weight vehicle (say, a, small car) and a heavy weight vehicle, (say, a loaded truck) are parked on a, horizontal road. Then, which of the, following statement is correct?, (a) Much greater force is needed to push the, truck., (b) Equal force is needed to push the truck and, car., (c) No force is required to move the vehicles., (d) None of the above, , 50. Which one of the following statement is, incorrect?, (a) Frictional force opposes the relative, motion., (b) Limiting value of static friction is directly, proportional to normal reaction., (c) Rolling friction is smaller than sliding, friction., (d) Coefficient of sliding friction has dimensions, of length., , 51. If no external force acts on particle,, then which of the following statement is, incorrect about particle?, (a) Particle may be at rest., (b) Particle moves with uniform velocity on, linear path., (c) Particle moves with uniform speed on circle., (d) None of the above, , 52. Match the Column I (type of friction), , with Column II (value of m) and select, the correct option from the codes given, below., Column I, A., , Column II, , Static friction, , m is highest, , p., , B., , Rolling friction, , q., , m is moderate, , C., , Kinetic friction, , r., , m is lowest, , Codes, A, , B, , C, , A, , B, , C, , (a) r, , q, , p, , (b) p, , q, , r, , (c) p, , r, , q, , (d) q, , r, , p, , 53. In the diagram shown in figure, match, the Column I with Column II and, select the correct option from the codes, given below. (Take, g = 10 ms -2 ), F2 = 18 N, , 1 kg, 2 kg, 3 kg, , F1 = 60 N, , Smooth, , θ = 30º, , Column II, , Column I, A., , Acceleration of, 2 kg block, , p., , 8 (SI unit ), , B., , Net force on, 3 kg block, , q., , 25 (SI unit ), , C., , Normal reaction, between 2 kg, and 1 kg, , r., , 2 (SI unit ), , D., , Normal reaction, between 3 kg, and 2 kg, , s., , None
Page 96 :
CBSE New Pattern ~ Physics 11th (Term-I), , Codes, A, , B, , C, , D, , (a) r, , s, , q, , s, , (b) r, , q, , s, , p, , (c) p, , q, , r, , s, , (d) p, , q, , q, , s, , Assertion-Reasoning MCQs, For question numbers 54 to 64, two, statements are given-one labelled, Assertion (A) and the other labelled, Reason (R). Select the correct answer to, these questions from the codes (a), (b), (c), and (d) are as given below, (a) Both A and R are true and R is the, correct explanation of A., (b) Both A and R are true but R is not the, correct explanation of A., (c) A is true but R is false., (d) A is false and R is also false., , 54. Assertion Aristotle stated that an, external force is required to keep a, body in motion., Reason Opposing forces are always, present in the natural world., , 55. Assertion A body is momentarily at, rest but no force is acting on it at that, time., , 85, angle with it, it changes the component, of velocity along the direction of force., Reason The component of velocity, parallel to the force remains, unchanged., , 58. Assertion If we consider system of two, bodies A and B as a whole, F AB and F BA, are internal forces of the system, ( A + B ). They add to give a null force., Reason Internal forces in a body or a, system of particles cancel away in pairs., , 59. Assertion It is not always necessary, that external agency of force is in, contact with the object while applying, force on object., Reason A stone released from top of a, building accelerates downward due to, gravitational pull of the earth., , 60. Assertion A seasoned cricketer allows, a longer time for his hands to stop the, ball, while catching the ball. His hand is, not hurt., Reason The novice (new player) keeps, his hand fixed and tries to catch the ball, almost instantly. He needs to provide a, much greater force to stop the ball, instantly and this hurts., , Reason When a force acts on a body,, it may not have some acceleration., , 56. Assertion At the microscopic level, all, bodies are made up of charged, constituents (like nuclei and electrons), and various contact forces exist, between them., Reason These forces are due to, elasticity of bodies, molecular collisions, and impacts, etc., , 57. Assertion If force is not parallel to the, velocity of the body, but makes some, , 61. Assertion Product of distance and, velocity (i.e. momentum) is basic to the, effect of force on motion., Reason Same force for same time, causes the same change in momentum, for different bodies.
Page 97 :
86, , CBSE New Pattern ~ Physics 11th (Term-I), , 62. Assertion Newton’s third law of, motion is applicable only when bodies, are in motion., Reason Newton’s third law does not, applies to all types of forces, e.g., gravitational, electric or magnetic, forces, etc., , 63. Assertion Angle of repose is equal to, angle of limiting friction., Reason When a body is just at the, point of motion, the force of friction of, this stage is called as limiting friction., , 64. Assertion A body of mass 1 kg is, making 1 rps in a circle of radius 1 m., Centrifugal force acting on it is 4 p 2 N., Reason Centrifugal force is given by, mv, ., F =, r, , Case Based MCQs, Direction Answer the questions from, 65-69 on the following case., Momentum and Newton’s Second Law of, Motion, Momentum of a body is the quantity of, motion possessed by the body. It depends on, the mass of the body and the velocity with, which it moves., When a bullet is fired by a gun, it can easily, pierce human tissue before coming to rest, resulting in casualty. The same bullet fired, with moderate speed will not cause much, damage. The greater the change in, momentum in a given time, the greater is the, force that needs to be applied., The second law of motion refers to the, general situation, where there is a net external, force rating on the body., , 65. A satellite in force-free space sweeps, stationary interplanetary dust at a rate, dM, = av , where M is the mass, v is the, dt, velocity of satellite and a is a constant., What is the deceleration of the satellite?, (a), , - 2 av 2, M, , (c) - av 2, , - av 2, M, av 2, (d), M, (b), , 66. A body of mass 5 kg is moving with, , velocity of v = ( 2$i + 6 $j ) ms - 1 at t = 0 s., After time t = 2 s, velocity of body is, (10 $i + 6 $j ) ms -1 , then change in, momentum of body is, (a) 40 $i kg-ms -1, (b) 20$i kg-ms -1, (c) 30$i kg-ms -1, (d) (50$i + 30$j) kg-ms -1, , 67. A cricket ball of mass 0.25 kg with, speed 10 m/s collides with a bat and, returns with same speed with in 0.01s., The force acted on bat is, (a) 25 N, (c) 250N, , (b) 50N, (d) 500N, , 68. A stationary bomb explodes into three, pieces. One piece of 2 kg mass moves, with a velocity of 8 ms -1 at right angles, to the other piece of mass 1 kg moving, with a velocity of 12 ms -1 . If the mass of, the third piece is 0.5 kg, then its, velocity is, (a) 10 m s-1, (c) 30 m s-1, , (b) 20 ms-1, (d) 40 ms-1, , 69. A force of 10 N acts on a body of mass, 0.5 kg for 0.25s starting from rest. What, is its momentum now?, (a) 0.25 N/s, (c) 0.5 N/s, , (b) 2.5 N/s, (d) 0.75 N/s
Page 98 :
CBSE New Pattern ~ Physics 11th (Term-I), , 87, 2T . Then, the velocity acquired by the, body is, , Direction Answer the questions from, 70-74 on the following case., Conservation of Momentum, This principle is a consequence of Newton’s, second and third laws of motion., In an isolated system (i.e. a system having no, external force), mutual forces (called internal, forces) between pairs of particles in the, system causes momentum change in, individual particles., Let a bomb be at rest, then its momentum will, be zero. If the bomb explodes into two equal, parts, then the parts fly off in exactly opposite, directions with same speed, so that the total, momentum is still zero. Here, no external, force is applied on the system of particles, (bomb)., , 74. Two masses of M and 4M are moving, , 70. A bullet of mass 10 g is fired from a gun, , with equal kinetic energy. The ratio of, their linear momenta is, , of mass 1 kg with recoil velocity of gun, 5 m/s. The muzzle velocity will be, (a), (b), (c), (d), , 30 km/min, 60 km/min, 30 m/s, 500 m/s, , 0, , A, T, , B, 2T, , – F0, , p F0 T, 4m, F0 T, (c), 4m, (a), , (b), , p F0 T, 2m, , (d) zero, , (a) 1 :8, (c) 1 :2, , (b) 1 :4, (d) 4 :1, , Direction Answer the questions from, 75-79 on the following case., , 71. A shell of mass 10 kg is moving with a, , velocity of 10 ms - 1 when it blasts and, forms two parts of mass 9 kg and 1 kg, respectively. If the first mass is, stationary, the velocity of the second is, (a), (b), (c), (d), , F0, , 1 m s -1, 10 m s-1, 100 m s-1, 1000 m s-1, , Force of Friction on Connected Bodies, When bodies are in contact, there are mutual, contact forces satisfying the third law of, motion. The component of contact force, normal to the surfaces in contact is called, normal reaction. The component parallel to, the surfaces in contact is called friction., , 72. A bullet of mass 0.1 kg is fired with a, , speed of 100 ms -1 . The mass of gun, being 50 kg, then the velocity of recoil, becomes, (a) 0.05 m s-1, (c) 0.1 m s-1, , (b) 0.5 m s-1, (d) 0.2 m s-1, , 73. A unidirectional force F varying with, time T as shown in the figure acts on a, body initially at rest for a short duration, , 8 kg, , 6 kg, , In the above figure, 8 kg and 6 kg are hanging, stationary from a rough pulley and are about, to move. They are stationary due to, roughness of the pulley.
Page 99 :
88, , CBSE New Pattern ~ Physics 11th (Term-I), , 75. Which force is acting between pulley, and rope?, (a), (b), (c), (d), , Gravitational force, Tension force, Frictional force, Buoyant force, , 78. The force of friction acting on the rope, is, (a) 20 N, (c) 40 N, , 76. The normal reaction acting on the, system is, (a) 8 g, (c) 2 g, , (a) 8 kg, (b) 6 kg, (c) Same on both, (d) Nothing can be said, , (b) 6 g, (d) 14 g, , (b) 30 N, (d) 50 N, , 79. Coefficient of friction of the pulley is, 1, 6, 1, (c), 5, , 1, 7, 1, (d), 4, , (a), , 77. The tension is more on side having, mass of, , (b), , ANSWERS, Multiple Choice Questions, 1. (c), 11. (b), 21. (d), 31. (c), , 2. (a), 12. (c), 22. (c), 32. (c), , 3. (c), 13. (a), 23. (a), 33. (b), , 4. (a), 14. (b), 24. (d), 34. (c), , 5. (a), 15. (c), 25. (c), 35. (c), , 6. (a), 16. (c), 26. (a), 36. (a), , 7. (b), 17. (b), 27. (c), 37. (d), , 8. (c), 18. (a), 28. (d), 38. (b), , 9. (b), 19. (b), 29. (a), 39. (a), , 10. (d), 20. (c), 30. (c), 40. (a), , 41. (b), 51. (c), , 42. (b), 52. (c), , 43. (b), 53. (a), , 44. (c), , 45. (c), , 46. (b), , 47. (c), , 48. (d), , 49. (a), , 50. (d), , 56. (a), , 57. (c), , 58. (c), , 59. (c), , 60. (b), , 61. (d), , 62. (d), , 63. (a), , 67. (d), 77. (a), , 68. (d), 78. (a), , 69. (b), 79. (b), , 70. (d), , 71. (c), , 72. (d), , 73. (d), , 74. (c), , Assertion-Reasoning MCQs, 54. (a), 64. (c), , 55. (d), , Case Based MCQs, 65. (d), 75. (c), , 66. (a), 76. (d)
Page 100 :
CBSE New Pattern ~ Physics 11th (Term-I), , 89, , SOLUTIONS, 1. Galileo conducted an experiment using a, double inclined plane. In this experiment,, two inclined planes are arranged facing each, other., When an object rolls down one of the, inclined planes, it climbs up the other. It, almost reaches the same height but not, completely because of friction. In ideal case,, when there is no friction the final height of, the object is same as the initial height as, shown in figure., Initial, position, , Final, position, , Both planes are inclined at same angle, , 2. According to Newton’s first law of motion,, everybody continues in its state of rest or, uniform motion. Unless an external force acts, upon it. This depicts that a body by itself, cannot change its state of rest or of uniform, motion along a straight line., This law is known as law of inertia., , 3. This is because the feet of the passenger, comes to rest along with the bus, but the, upper part of his body, due to inertia of, motion, tends to remain in motion., , 4. If downward force on the earth stops, so, upward self-adjusting force also stop. In, vertical direction, there is no force. Due to, inertia, person resists any change to its state, of rest. So, person will remain standing., 5. During pick-up, the car accelerates. This must, happens due to a net external force. This is, because, the acceleration of the car cannot be, accounted for by any internal force. The only, conceivable external force along the road is, the force of friction. It is the frictional force, that accelerates the car as a whole., 6. Since, momentum is directly proportional to, mass of the body. Hence, when both iron, balls are dropped from same height, then, bigger ball gain greater momentum than, smaller ball at the time of striking the glass, pane. Hence, it can transfer greater, , momentum to the glass pane and so it, breaks., , 7. Given, m = 1.0 ´ 10 4 kg, As the weight of the man is increased, 5 times, so acceleration of the rocket, also, increase to 5 times., i.e., a = 5g = 5 ´ 10 = 50 ms -2, Force applied by rocket engine,, F = ma = 1.0 ´ 10 4 ´ 50 = 5 ´ 10 5 N, 1, 2, , 8. From equation of motion, y = ut + at 2, where, a is the acceleration., , …(i), , 1, × 2gt 2 …(ii), 2, Comparing Eqs. (i) and (ii), we get, Acceleration, a = 2 g, Force = m ´ a = m × 2g = 2mg, , Given equation, y = ut + gt 2 = ut +, , 9. Given, mass of bullet, m = 0.04 kg, Initial speed of bullet, u = 90 ms -1, Time, t = 3 s, Final velocity of bullet, v = 0, If a be the retardation in the bullet in the, wooden block, then, From equation of motion, v = u - at, 0 = 90 - a ´ 3, Þ, 3a = 90 Þ a = 30 m/s 2, \ Average resistive force,, F = m × a = 0.04 ´ 30 = 1.2 N, 10. Given, mass, m = 6 kg, Velocity, v = v 2 - v 1 = 5 - 3 = 2, \ Momentum, p = mv = 6 ´ 2 = 12 N-s, , 11. To solve this question we have to apply, Newton’s second law of motion, in terms of, force and change in momentum., dp, We know that, F =, dt, Given that, meter scale is moving with, uniform velocity, hence dp = 0., Force, F = 0., As all parts of the scale is moving with, uniform velocity and total force is zero,, hence torque will also be zero.
Page 101 :
90, , CBSE New Pattern ~ Physics 11th (Term-I), , 16. The area under F-t graph gives change in, , 12. Given, mass of satellite, m = 10 4 kg, F = 5 ´ 10 N, t = 20 s, u = 0, v = ?, Impulse applied on the satellite is equal to, the change in momentum., i.e., F × t = m (v - u ), 5 ´ 10 5 ´ 20 = 10 4 ( v - 0 ), 5, , Þ, , v =, , 5 ´ 10 5 ´ 20, = 1000 m/s = 1 km/s, 10 4, , momentum., So, for the F-t graph as shown below, , F(N) 0, A 2, , –3, , F, , 4, D, , I, , 6, 8, t(s), E, , 1, ´ 2 ´ 6 = 6 kg-ms -1, 2, For 2 to 4s, Dp 2 = Area under the rectangle, CFEDC, = 2 ´ -3 = - 6 kg-ms -1, For 4 to 8s, Dp 3 = Area under the rectangle, =, , \ Force acting on the particle = 18 N, Dp ö, ÷, è Dt ø, , 14. From Newton’s second law, F = n × æç, , where, F = force, n = number of bullets fired, Dp, per second and, = rate of change of, Dt, momentum of one bullet., æmv - 0 ö, Þ, F =n ç, ÷, è Dt ø, Given, F = 144 N, m = 40 g = 40 ´ 10-3 kg,, v = 1200 ms-1 and Dt = 1 s, 40 ´ 10 - 3 ´ 1200, \, 144 = n ´, 1, 144, n=, Þ, 4 ´ 12, n=3, , 15. Given, u = ( 3$i + 4 $j ) m/s, and v = - ( 3i$ + 4 $j ) m/s, Mass of the ball, m = 150 g = 0.15 kg, Dp = Change in momentum, = Final momentum – Initial momentum, = mv - mu, = m ( v - u ) = ( 015, . ) [-( 3i$ + 4 $j ) - ( 3$i + 4 $j )], = ( 015, . ) [ -6 i$ - 8 $j ], , Hence, Dp = - [ 0. 9 i$ + 1 . 2$j ], , C, , H, , For 0 to 2s, Dp 1 = Area under the triangle ABC, , Differentiate w.r.t. t, we get, dp, = 0 + 3 ´ 2t = 6t, dt, dp, If t = 3 s, then, = 6 ´ 3 = 18 N, dt, , = - [ 015, . ´ 6 i$ + 015, . ´ 8 $j ], $, $, = - [ 0. 9 i + 1. 20 j ], , G, , 3, , 13. Given, p = 2 + 3t 2, , Þ, , B, , 6, , FIHGF, = 4 ´ 3 = 12 kg-ms -1, So, total change in momentum for 0 to 8s,, Dp net = Dp 1 + Dp 2 + Dp 3, = ( + 6 - 6 + 12) = 12 kgms -1 = 12 N-s, , 17. Here, F = kt, When t = 0, then linear momentum = p, When t = T , then linear momentum = 3p, According to Newton’s second law of motion,, dp, Applied force, F =, dt, or, dp = F × dt, or, dp = kt × dt, Now, integrate both side with proper limit, 3p, , T, , T, , ét2 ù, 3p, or, dp, k, t, dt, =, [, p, ], =, k, p, ê 2ú, òp, ò0, ë û0, 1, or ( 3 p - p ) = k (T 2 - 0 ), 2, p, 4, p, or, or T = 2, T2=, k, k, , 18. Given, F = 50 N, m = 20 kg, v = 15 ms -1, mv, Dt, mv, Dt =, F, 20 ´ 15, Dt =, =6 s, 50, , Impulse, F =, Time,
Page 102 :
CBSE New Pattern ~ Physics 11th (Term-I), , 19. The situation is as depicted below, , 91, 24. In equilibrium, net force is zero, therefore, acceleration is zero, hence particle is either at, rest or in motion with uniform velocity., , 25. For stable condition,, m= 0.15 kg, , Initial momentum = mv = 0 .15 ´ 12, = 18, . N-s to right, Final momentum = mv = 0.15 ´ 12, = 1.8 N-s to left, Impulse = Change in momentum, = Final momentum - Initial momentum, = (1.8 N-s ) - ( - 1.8 N-s ), = (1.8 N-s ) + (1.8 N-s ) = 3.6 N-s, = 3.6 N-s towards left, , 20. According to figure, radius of semi-circle,, r =2, Linear momentum gained, = Impulse from 0 to 4 s, = Area enclosed by graph from 0 to 4 s, pr 2 p ( 2) 2, =, =, = 2p N-s, 2, 2, , F1 + F2 + F3 = 0, ( 3$i - 4 $j) + ( 2$i - 3$j) + F3 = 0, Þ, F = -5 $i + 7 $j, , (given), , 3, , 26. Let equal forces F 1 = F 2 = F newton, Angle between the forces, q = 60°, Resultant force, R = 40 3 N, Now, R = F 12 + F 22 + 2F 1 F 2 cos q, \ 40 3 = F 2 + F 2 + 2FF cos 60°, or, , F = 40 N, , 27. Consider the adjacent diagram, N, B, , A, , W, , E, , O, , 21. Action and reaction forces always act on, different bodies, because if they work on, same body, then net force on the body is, zero and there could never be accelerated, motion., So, they cannot balance or cancel each other., , S, , Let, OA = p 1, = initial momentum of player northward, and AB = p 2 = final momentum of player, towards west., , Hence, options (a) and (c) are correct., , B, , A, , R, , O, , 22. From law of conservation of momentum,, pi = p f, and initial momentum, p i = mu = m ( 0 ) = 0, \ p f should also be zero., Hence, other piece will move in negative, x-direction., , 23. From conservation of linear momentum,, m 2v 2 = m 1v 1, 200, 100 v 2 =, ´ 80, 1000, 200 ´ 80, v2 =, 1000 ´ 100, Þ, or, , v 2 = 0.16 m/s, v 2 = 16 cm/s, , Clearly,, OB = OA + AB, Change in momentum = p 2 - p 1, = AB - OA = AB + ( - OA ), = Clearly resultant AR will be along, , south-west., 28. From the given forces, we can say that first, two forces 1 N and 2 N, if are in the same, direction, then it would be equal to third, force 3 N. But it is given that, all the three, forces are in different directions., So, there is no possibility that these three, forces, are in equilibrium.
Page 103 :
92, , CBSE New Pattern ~ Physics 11th (Term-I), , 29. Since, all the blocks are moving with constant, , 32., , N, , velocity and we know that, if velocity is, constant, acceleration of the body becomes, zero., Hence, the net force on all the blocks will be, zero., , 2 kg, , 70 N, , 30. Apply Lami’s theorem at O,, , N - 70 = 7 ´ 5, , T1, T2, =, sin 150° sin 120°, 10, 10, =, =, = 10, sin 90° 1, \, , –2, , 5 ms, , 5 kg, , \, , N = 105 N, m A = 4 kg,, , 33. Given,, , m B = 2 kg, m C = 1 kg and F = 14 N, , T 1 = 10 sin 150°, 1, = 10 ´ = 5 N, 2, T 2 = 10 sin 120°, 3, = 10 ´, =5 3N, 2, , a, F, , 31. T 1 and T 2 are the tensions in the sections BC, and BF , then resolution of all forces at B in, two perpendicular directions are shown, below, , A, , B, , C, , So, total mass, M = 4 + 2 + 1 = 7 kg, Now, F = Ma Þ 14 = 7a Þ a = 2 ms -2, FBD of block A,, a, F, , F′, , 4 kg, , T1 sin 30°, C, , T2 sin 30°, T1, B, , 30°, T1 cos 30°, , 90°, , 30°, 90°, , F, , T2, T2 cos 30°, , Þ F ¢ = F - 4a = 14 - 4 ´ 2 Þ F ¢ = 6 N, Hence, the contact force between A and, B is 6 N., , 34. First of all consider the forces on the blocks, as shown below, , a, , 10 N, T1, , A, , For equilibrium along horizontal direction,, T 1 cos 30° = T 2 cos 30°, Let,, T1 =T2 =T, Again, for equilibrium along vertical, direction., T 1 sin 30° + T 2 sin 30° = 10, Þ, 2T sin 30° = 10, 1, 2T ´ = 10 Þ T = 10 N, 2, So, the tension in both sections BC and, BF is 10 N., , m, , T2 T3, , µmg, , a T1, m, , 2, , 3, m, µmg, , 1, , mg, , For the Ist block, mg - T 1 = m ´ a, …(i), Let us consider 2nd and 3rd blocks as a system,, so, …(ii), T 1 - 2 mmg = 2m ´ a, Adding Eqs. (i) and (ii), we get, g, mg (1 - 2 m ) = 3m ´ a Þ a = (1 - 2 m ), 3
Page 104 :
CBSE New Pattern ~ Physics 11th (Term-I), , 93, , 35. Given, m 1 = 1 kg , m 2 = 2 kg and g = 10 ms -2, , one in a radius r / 2 and lighter one in, radius r., As, lighter stone is n times that of the value of, heavier stone when they experience same, centripetal forces, we get, ( F c ) heavier = ( F c ) lighter, 2m ( v ) 2 m (nv ) 2, Þ, =, ( r / 2), r, , æm - m 1 ö, Acceleration, a = ç 2, ÷g, èm 1 + m 2 ø, æ 2 -1ö, 10, =ç, ÷ 10 =, è1 + 2 ø, 3, 1 2, é, -1 ù, êëQ s = ut + 2 at and u = 0 ms úû, 1, Distance, s = ´ a ´ t 2, 2, 1 10, 20, = ´ ´4=, m, 2 3, 3, , 36. Frictional force in the direction of train, causes the acceleration of the box lying in, the compartment of an accelerating train., , 37. Given, m = 2 kg, m = 0 . 2, and, g = 10 m/s2, Here, ma = mmg, Þ, a = mg = 0.2 ´ 10 = 2 ms-2, , 38. Given, mass of the car = m, As car starts from rest, u = 0, Velocity acquired along east = v $i, Duration, t = 2s., We know that, v = u + at, Þ, v $i = 0 + a ´ 2, Þ, Force,, , a=, , v$, i, 2, , mv $, F = ma =, i, 2, , mv, towards, 2, east. As external force on the system is only, mv, is by friction., friction, hence the force, 2, Hence, force by engine is internal force., Hence, force acting on the car is, , 39. Given, m = 2 kg, r = 10 m and v = 5 ms -1, Radial acceleration (centripetal acceleration), v2 5 ´ 5, =, =, = 2.5 ms -2, r, 10, Force = Mass ´ Acceleration = 2 ´ 2.5 = 5 N, , 40. Given that, two stones of masses m and 2m, are whirled in horizontal circles, the heavier, , Þ, Þ, , n2 = 4, n=2, , 41. For car moving in circle of radius R, with, velocity v and mass = m ,, Centripetal force required, = Frictional force £ m s N, mv 2, (Q N = mg ), £ m smg, R, v £ m s Rg, , 42. We know that, F =, , 2, mv max, r, , …(i), , and, …(ii), F = m smg, From Eqs. (i) and (ii) for maximum speed of, vehicle, mv 2, m smg ³ max, r, where, v max = maximum velocity of vehicle., Given, m s = 01, . , r = 5 m and g = 10 ms -2, \, v max = m s rg, 2, v max, = 01, . ´ 5 ´ 10 = 5 m 2 s -2, So, person or vehicle will slip, if v 2 > 5 m 2 s -2 ., , 43. Given, m s = 0.2, R = 300 m and q = 15°, Optimum speed, v o = gR tan q, = 9.8 ´ 300 ´ tan 15°, = 2940 ´ 0.27 = 28.1 ms -1, and v max =, =, , gR ( m s + tan 15° ), 1 - m s tan 15°, 9.8 ´ 300 (0.2 + 0.27), 1 - 0.2 (0.27), , = 38.1 ms -1, Thus, the optimum speed and maximum, permissible speed are 28.1 ms -1 and, 38.1 ms -1 , respectively.
Page 105 :
94, , CBSE New Pattern ~ Physics 11th (Term-I), , 44. If angle of banking is q, then, tan q =, , 2, , 50. The opposing force that comes into play, when one body is actually sliding over the, surface of the other body is called sliding, friction., , 2, , mv /r, v, Þ tan q =, mg, rg, , Given, v = 10 ms -1, r = 10 m, and g = 10 ms- 2, (10 ) 2, So, tan q =, =1, 10 ´ 10, , The coefficient of sliding is given as, m S = N / F sliding, where, N is the normal reaction and F sliding is, the sliding force., As, the dimensions of N and F sliding are same., Thus, m S is a dimensionless quantity. When, body is rolling, then it reduces the area of, contact of surfaces, hence rolling friction is, smaller than sliding friction., , \, q = 45°, 45. The term inertia means resistance of any, physical object. It is defined as the tendency, of a body to remain in its position of rest or, uniform motion. So, it is dependent on mass, of the body., , 46. Given, mass, m = 5 kg, , Hence, statement (d) is incorrect., , 51. When particle moves in a circle even with, , Acting force, F = ( -3$i + 4 $j ) N, Initial velocity at t = 0, u = ( 6 $i - 12$j ) m/s, Retardation, a$ =, , F æ 3i$ 4 $j ö, = ç+, ÷ m/s 2, m è 5, 5ø, , As final velocity is along Y-axis only, its, x-component must be zero., From v = u + at, for x-component only,, 3$i, 0 = 6 $i - t, 5, 5´6, t=, = 10 s, 3, , 47. Impulse is defined as rate of change of, momentum. For change in momentum to be, minimum., d, ( 20t 2 - 20t ) = 0, dt, 40t - 20 = 0, 1, t= s, 2, , 48. Frictional force is a non-conservative force, because work done by it is dissipated, (wasted) as heat energy. This is not the case, with other forces., , 49. Due to inertia, greater force is needed to, push the truck than the car, to bring them to, the same speed in same time., Thus, the statement given in option (a) is, correct, rest are incorrect., , 52., , uniform or constant speed, it faces an, external force towards its centre called, centripetal force. Hence, the statement given, in option (c) is incorrect., A. Static friction is the frictional force, between the surfaces of two objects when, they are not in motion with respect to each, other., Due to this reason, static friction has the, highest value of frictional force and hence, m is highest., B. Rolling friction takes place when one body, rolls over the surface of another body due, to which the value of friction is less in case, of rolling friction and hence m is lowest., C. Kinetic friction takes place when one body, slides over the surface of the another body., Value of friction is moderate and lie in, between the friction value of rolling and, static friction and hence m is moderate., Hence, A ® p, B ® r and C ® q., , 53. Acceleration of system,, a =, , 60 - 18 - (m 1 + m 2 + m 3 ) g sin 30°, = 2 ms -2, (m 1 + m 2 + m 3 ), , Net force on 3 kg block = m 3a = 6 N, From free body diagram of 3 kg block, we, have, N 12 - m 1 g sin 30° - 18 = m 1 a, Þ, N 12 = 25 N, From free body diagram of 3 kg block, we, have
Page 106 :
CBSE New Pattern ~ Physics 11th (Term-I), , 60 - m 3 g sin 30° - N 32 = m 3a, \, , N 32 = 39 N, , Hence, A ® r, B ® s, C ® q and D ® s., , 54. Aristotle stated that an external force is, required to keep a body in motion as it can, be observed in our surrounding, i.e. to move, a body, we need to push or pull an object., But Aristotle didn’t give any reason behind, this fact., The reason behind this fact is that, there are, number of opposing forces like friction,, viscosity, etc., are always present in the, natural world. To counter these opposing, forces, some external force is required to, keep a body in motion., Therefore, both A and R are true and R is, the correct explanation of A., , 55. A stationary body ( v = 0 ) may still have some, acceleration, e.g. when a body is thrown in, upward direction, it comes to rest at highest, position, but at that time, it still have, acceleration equal to acceleration due to, gravity g ., Hence, gravitational force is acting at highest, position and when a force acts on a body,, then its accelerates., Therefore, A is false and R is also false., , 56. At the microscopic level, all bodies are made, up of charged constituents and various, contact forces exist between them., These forces are due to elasticity of bodies,, molecular collisions and impacts etc., Therefore, both A and R are true and R is, the correct explanation of A., , 57. Force is a vector quantity. Thus, if force is, not parallel to the velocity of the body, but, makes some angle with it, it changes the, component of velocity along the direction of, force., The component of velocity normal to the, force remains unchanged, e.g. in projectile, motion, horizontal component of velocity, does not change under the effect of vertical, gravitational force., Therefore, A is true but R is false., , 95, , 58. If force on A by B = FAB and force on B by, A = FBA ., These forces add to give a null force when, FAB = – FBA ., Here FAB and FBA are internal forces of ( A + B ), system., Internal forces in a body do not cancel away,, as they do not act on the same particle., Therefore, A is true but R is false., , 59. It is not always necessary that external, agency of force is in contact with the object,, while applying force on object., Force can be applied on a body/particle, without contact or with contact, it depends, on the agency, applying force. e.g., earth pulls (exerts force) from distance., A stone without any physical contact falls, due to gravitational pull of the earth., Therefore, A is true but R is false., Change in momentum Dp, 60. Force =, =, Time interval, Dt, If time interval is increased, then force will, get decreased (for constant Dp). Therefore,, reaction force on the hand is small, i.e. he, experience less hurt., This is what seasoned cricketer does., New player make Dt small, so force is more,, which hurt new player’s hand., Therefore, both A and R are true but R is, not the correct explanation of A., , 61. As we know, momentum, p = mv, Change in p can be brought by changing, force F i.e., dp, F=, = rate of change of momentum with, dt, time., Þ, md v = Fdt, So, in order to keep, Fdt constant, mdv should, be constant, here m and dv can change from, one body to another body., Thus, same force for same time can cause, different change in momentum for different, bodies., Therefore, A is false and R is also false.
Page 107 :
96, , CBSE New Pattern ~ Physics 11th (Term-I), , 62. According to Newton’s third law of motion, it, is impossible to have a single force out of, mutual interaction between two bodies,, whether they are moving or at rest., It means, third law of motion is applicable to, all the bodies either at rest or in motion and, this law is also applicable to all types of, forces., Therefore, A is false and R is also false., , 63. Angle of repose is equal to angle of limiting, friction and maximum value of static friction, is called the limiting friction., Maximum force of static friction which, comes into play when a body just starts, moving. Over the surface of another body is, called limiting friction., Therefore, both A and R are true and R is, the correct explanation of A., , 64. From relation, the centrifugal force,, mv 2 m ( r w ) 2, =, = mrw2, r, r, = mr ( 2pn ) 2 = 4 p 2 mr n 2, = 4 p 2 ´ 1 ´ 1 ´ 12 = 4 p 2 N, mv 2, Centripetal force, F =, r, F =, , Therefore, A is true but R is false., dp, é dM ù, 65. Force, F =, =v ê, = av 2, dt, ë dt úû, Þ, , a =, , F, av 2, =, M, M, , 66. Given, mass, m = 5 kg, Change in velocity, Dv =, v f - v i = [(10 - 2) i$ + ( 6 - 6 ) $j ], Change in momentum, = mDv = 5 [8 $i ] = 40 $i kg -ms - 1, , 67. Momentum,, Dp = 2mv = 2 ´ 0.25 ´ 10 = 5 kg-m/s, Dp, 5, Force, F =, =, = 500 N, Dt, 0.01, , 68. Momentum of third piece,, p=, , p x2 + p 2y = (16 ) 2 + (12) 2, , = 20 kg-m/s, , v =, , p 20, =, = 40 m/s, m 0.5, px = 2×8 = 16, , py = 1×12 = 12, , 69. Given, F = 10 N, v i = 0,, m = 0.5 kg, Dt = 0 .25 s, Q Change in momentum, Dp = p f - p i, Also, Dp = F × Dt, From Eqs. (i) and (ii), we get, F × Dt = p f - p i, or 10 ´ 0.25 = p f - mv i, 2.5 = p f - 0.5 ´ 0, Þ, p f = 2.5 N/s, , …(i), …(ii), , 70. Conservation of linear momentum gives, m 1v 1 + m 2v 2 = 0, m 1v 1 = - m 2v 2, - m 2v 2, v1 =, Þ, m1, æ 10 ö, Given, m 1 = 10 g = ç, ÷ kg, è1000 ø, m 2 = 1 kg and v 2 = -5 m/s, \ Velocity of muzzle,, +1´ 5, v1 =, = 500 m/s, 10 /1000, , 71. Given that, v 1 = 10 m s -1,, m 1 = 10 kg, v 2 = 0 ,, m 2 = 9 kg, v 3 = v ,, m 3 = 1 kg, According to conservation of momentum,, m 1v 1 = m 2v 2 + m 3v 3, 10 ´ 10 = 9 ´ 0 + 1 ´ v Þ v = 100 ms -1, , 72. From the law of conservation of momentum,, Initial momentum = Final momentum, Þ m 1u1 + m 2u2 = m 1v 1 + m 2v 2, \ 0.1 ´ 0 + 50 ´ 0 = 0.1 ´ 100 + 50 (- v 2 ), Þ, 0 = 10 - 50v 2, 10, v2 =, \, = 0.2 ms -1, 50
Page 108 :
CBSE New Pattern ~ Physics 11th (Term-I), , 73. From 0 to T , area is positive and from T to, , 97, , 77. As, tension, T = mg Þ T µ m, , 2T , area is negative, so net area is zero., Hence, there is no change in momentum., , 74. Two masses are moving with equal kinetic, energy., 1, 1, M v 12 = 4 M v 22, 2, 2, v1, or, =2, v2, The ratio of linear momentum is, p1, M v1, =, p2 4 M v 2, or, , p1 1 æ v 1 ö, = ç ÷, p2 4 è v 2 ø, , or, , p1 2 1, = =, p2 4 2, , Þ, , p1 : p2 = 1 : 2, , 75. Frictional force acts between pulley and rope., 76. The reaction force is, R = T 1 + T 2 = ( 8 + 6 ) g = 14 g, , So, the side having 8 kg mass will have more, tension., , 78., f (force of friction), , T2, T1, , 6 kg, , 8 kg, , Due to friction, tension at all points of the, thread is not alike., T 1 -T 2 = f, Þ, f = 8 g -6 g = 2g, (Q g = 10 ms -2 ), = 20 N, , 79. As, mR = f = 20 N, m=, , 20, 20, 1, =, =, R 14 ´ 10 7, , (Q R = mg )
Page 109 :
98, , CBSE New Pattern ~ Physics 11th (Term-I), , 06, Work, Energy, and Power, Quick Revision, 1. Work Work is said to be done by a force, when, the body is displaced actually through some, distance in the direction of the applied force., Thus, work is done on a body only if the, following two conditions are satisfied, ● A force acts on the body., ● The point of application of the force moves, in the direction of the force., 2. Work Done by a Constant Force Work done, by the force (constant force) is the product of, component of force in the direction of the, displacement and the magnitude of the, displacement. Then, the work done on the body by, the force is given by, Work done,W = F × s, SI unit of work is joule( J )., Its dimensions are [M 1L2T -2]., 3. Work Done when Force and Displacement, are Inclined to Each Other, Fy, , Fy, F, θ, , F, , Fx, , θ, , Fx, , s, , Work done, W = F × s = ( F cos q ) × s = Fs cos q, Two cases can be considered as given below for, the maximum and minimum work, Case I When F and s are in the same, direction, i.e. q = 0° , then work done is, W = Fs cos 0° = Fs (1) = Fs, , i.e. maximum work done by the, force., Case II When F and s are perpendicular to, each other, i.e. then, W = F × s = Fs cos 90° = Fs (0 ) = 0,, i.e. no work done by the force, when a, body moves in a direction, perpendicular to the force acting., 4. Work Done by a Variable Force Work, done by variable force is given as,, Wxi, , ®x, , f, , =, , ò, , x, , xi, , f, , F ×d x =, , ò, , x, , f, , xi, , (F cos q ) dx, , = Area under force-displacement curve, When the magnitude and direction of a force, vary in three dimensions, then it can be, expressed in terms of rectangular, components., So, work done from x i to x f ,, W =, , ò, , x, , xi, , f, , F x dx +, , ò, , x, , xi, , f, , F y dy +, , ò, , x, , xi, , f, , F z dz, , where, F x , F y and F z are the rectangular, components of force in x, y and z-directions,, respectively., 5. Conservative Force If the work done by the, force in displacing an object depends only on, the initial and final positions of the object and, not on the nature of the path followed, between the initial and final positions, such a, force is known as conservative force. e.g., Gravitational force is a conservative force.
Page 110 :
CBSE New Pattern ~ Physics 11th (Term-I), , 6. Non-Conservative Force If the work done, by a force in displacing an object from one, position to another depends upon the path, between the two positions. Such a force is, known as non-conservative force. e.g. Friction, is a non-conservative force., 7. Energy The energy of a body is defined as its, capacity or ability for doing work., The dimensions of energy are the same as, the dimensions of work, i.e. [M 1 L2 T -2 ]., ● It is measured in the same unit as work, i.e., joule in SI system and erg in CGS system., 8. Kinetic Energy The energy possessed by a, body by virtue of its motion is called kinetic, energy. In other words, the amount of work, done, by a moving object before coming to rest, is equal to its kinetic energy., 1, \ Kinetic energy, KE = mv 2, 2, where, m is a mass and v is the velocity of a, body., ● Relation between Kinetic Energy and, Linear Momentum, ●, , p = 2m K, 9. Work Energy Theorem or Work Energy, Principle It states that, work done by the net, force acting on a body is equal to the change, produced in the kinetic energies of the body., f, , 99, 12. Potential Energy of a Spring For a small, stretch or compression, spring obeys Hooke’s, law, i.e. restoring force µ stretch or compression, - Fs µ x Þ Fs = - k x, where, k is called spring constant. Its SI unit, is Nm –1.The negative sign shows F s acts in the, opposite direction of displacement x., If the block is moved from an initial, displacement x i to final displacement x f , then, work done by spring force is, 1, 1, W s = kx i2 - kx f 2, 2, 2, \ Change in potential energy of a spring, 1, DU = – W s = k ( x 2f – x i2 ), 2, 1, If, x i = 0, then DU = kx 2f, 2, 13. Conservation of Mechanical Energy This, principle states that, if only the conservative, forces are doing work on a body, then its, mechanical energy (KE + PE) remains constant., i.e. K + U = constant = E, \ K i +Ui = K f +U f, The quantity K + U , is called the total, mechanical energy of the system., 14. Motion in a Vertical Circle A particle of, mass m is attached to an inextensible string of, length L and is moving in a vertical circle, about fixed point O (as shown), , K f – K i = ò Fnet × dx, i, , \ K f - K i =W, where, K f and K i are the final and initial, kinetic energies of the body., 10. Potential Energy The potential energy of a, body is defined as the energy possessed by the, body by virtue of its position or configuration., So, if configuration of the system changes, then, its potential energy changes., Dimensions = [ML2T –2 ], SI unit = Joule, 11. Gravitational Potential Energy, Gravitational potential energy of a body is the, energy possessed by the body by virtue of its, position above the surface of the earth., Gravitational potential energy, U = mgh, , O, , h, , L, , θ, , A, ●, , ●, , ●, , T, , u, , v, , B, mg cos θ, mg sin θ, , Minimum velocity at highest point, so that, particle complete the circle, v min = gL ,, at this velocity, tension in the string is zero., Minimum velocity at lowest point, so that, particle complete the circle, v min = 5 gL ,, at this velocity, tension in the string is 6 mg., When string is horizontal, then minimum, velocity is 3Rg and tension in this, condition is 3 mg.
Page 111 :
100, , CBSE New Pattern ~ Physics 11th (Term-I), , 15. Power Power of a person or machine is, defined as the rate at which work is done or, energy is transferred., Average power (Pav ) = rate of doing work, work done (W ), =, time taken (t ), Thus, the average power of a force is defined, as the ratio of the work (W) to the total time (t)., 16. The instantaneous power of an agent at any, instant is equal to the dot product of its force, and velocity vectors at that instant., P = F×v, 17. Power is a scalar quantity and its dimensional, formula is [ML2T –3 ]., The SI unit of power is watt (W)., 1 joule, 1 watt =, = 1 Js - 1, 1 second, Another popular units of power are kilowatt and, horse power., 1 kilowatt = 1000 watt or 1 kW = 10 3 W, 1 horse power = 746 watt or 1 HP = 746 W, This unit is used to describe the output of, automobiles, motorbikes, engines, etc., 18. Collision A collision is an isolated event in, which two or more colliding bodies exert, strong forces on each other for a relatively, short time. For a collision to take place, the, actual physical contact is not necessary., Collision between particles have been divided, into two types which can be differentiated as, Elastic Collision, , Inelastic Collision, , A collision in which, there is absolutely no, loss of kinetic energy., , A collision in which there, occurs some loss of, kinetic energy., , Forces involved during, elastic collision must be, conserved in nature., , Some or all forces, involved during collision, may be non- conservative, in nature., , The mechanical energy, is not converted into, heat, light, sound, etc., , A part of the mechanical, energy is converted into, heat, light, sound, etc., , e.g. Collision between, subatomic particles,, collision between glass, balls, etc., , e.g. Collision between, two vehicles, collision, between a ball and floor,, etc., , 19. Conservation of Linear Momentum in, Collision Total linear momentum is, conserved at each instant during collision., \ p 1 + p 2 = constant, 20. Elastic Collision in One Dimension, In one-dimensional elastic collision, relative, velocity of separation after collision is equal to, relative velocity of approach before collision., u1 - u2 = v2 - v1, Velocities of the Bodies After the Collision, Velocity of Ist body after collision,, æ 2m 2 ö, æm - m2 ö, v1 = ç 1, ÷ u2, ÷ u1 + ç, èm1 + m2 ø, èm1 + m2 ø, , …(i), , Velocity of IInd body after collision,, æ 2m 1 ö, æm - m1 ö, v2 = ç 2, ÷u 1, ÷ u2 + ç, m, m, +, èm1 + m2 ø, è 1, 2ø, , …(ii), , Eqs. (i) and (ii) give the final velocities of the, colliding bodies in terms of their initial, velocities., The two cases under the action of same and, different masses can be considered as given, below, Case I, , When two bodies of equal masses, collide., i.e., , m 1 = m 2 = m (say), , From Eq. (i), we get, 2mu 2, = u 2 = velocity of body of, v1 =, 2m, mass m 2 before collision, From Eq. (ii), we get, 2mu 1, = u 1 = velocity of body of, v2 =, 2m, mass m 1 before collision., Case II When a light body collides against a, massive stationary body., Here, m 1 << m 2 and u 2 = 0, Neglecting m 1 in Eq. (i), we get, m 2u 1, v1 = = - u1, m2, From Eq. (ii), we get, v2 0
Page 112 :
CBSE New Pattern ~ Physics 11th (Term-I), , 21. Perfectly Inelastic Collision in One, Dimension, When the two colliding bodies together move, as a single body with a common velocity after, the collision, then the collision is perfectly, inelastic., In perfectly inelastic collision between two, bodies of masses m 1 and m 2, the body of mass, m 2 happens to be initially at rest (u 2 = 0 ). After, the collision, the two bodies move together, with common velocity v. The change in their, m 1 m 2u 12, kinetic energies is KE =, 2 (m 1 + m 2 ), \ DKE is a positive quantity., Therefore, kinetic energy is lost mainly in the, form of light, sound and heat., 22. Elastic Collision in Two Dimensions When, the collision between two bodies is not, head-on (the force during the collision is not, along the initial velocity). The bodies move, along different lines, then the collision is called, elastic collision in two dimensions., The three cases can be considered as given, below, Case I Glancing Collision In a glancing, collision, the incident particle does not lose, any kinetic energy and is scattered almost, undeflected. Thus, for such collision, when, q = 0°, f = 90°, u 1 = v 1 and v 2 = 0., 1, KE of the target particle = m 2v 22 = 0, 2, , 101, Case II Head-on Collision In this type of, collision, the target particle moves in the, direction of the incident particle, i.e. f = 0°., m 1u 1 = m 1v 1 cos q + m 2v 2 and 0 = m 1v 1 sin q, So, the kinetic energy remains unchanged., Case III Elastic Collision of Two Identical, Particles When two particles of same mass, undergo perfectly elastic collision in two, dimensions, i.e. m 1 = m 2., \, q + f = 90°, Thus, after collision the two particles will move, at right angle to each other., 23. Inelastic Collision in Two Dimensions, When two bodies travelling initially along the, same straight line collide involving some loss of, kinetic energy and move after collision along, different directions in a plane, then it is called, inelastic collision in two dimensions., 24. Coefficient of Restitution or, Coefficient of Resilience, It is defined as the ratio of relative velocity of, separation after collision to the relative velocity, of approach before collision. It is denoted by e ., e =, , Relative velocity of separation (after collision), Relative velocity of approach (before collision), e =, , |v2 - v1 |, |u 2 - u 1 |, , where, u 1 & u 2 are velocities of two bodies, before collision and v 1 & v 2 are their respective, velocities after collision., , 25. Comparison between Different Types of Collisions, Collision, , Kinetic Energy, , Coefficient of Restitution, , Main Domain, , Elastic, , Conserved, , e =1, , Between atomic particles, , Inelastic, , Non-conserved, , 0 < e <1, , Between ordinary objects, , Perfectly inelastic, , Maximum loss of KE, , e =0, , During shooting, , Super elastic, , KE increases, , e >1, , In explosions
Page 113 :
102, , CBSE New Pattern ~ Physics 11th (Term-I), , Objective Questions, Multiple Choice Questions, 1. A bicyclist comes to a skidding stop in, 10 m. During this process, the force on, the bicycle due to the road is 200N and, is directly opposed to the motion. The, work done by the cycle on the road is, , 5. A body moves from point A to B under, the action of a force varying in, magnitude as shown in figure, then the, work done is (force is expressed in, newton and displacement in metre), 20, 15, 10, 5, F 0, , (NCERT Exemplar), , (a) + 2000 J, (c) zero, , (b) - 200 J, (d) - 20, 000 J, , 2. Force of 50 N acting on a body at an, , angle q with horizontal. If 150 J work is, done by displacing it 3 m, then q is, (a) 60°, (c) 0°, , –5, –10, –15, , (b) 30°, (d) 45°, , 3. A particle is pushed by forces, , 2$i + 3$j - 2k$ and 5$i - $j - 3k$, simultaneously and it is displaced from, point $i + $j + k$ to point 2$i - $j + 3k$ . The, work done is, (b) - 7 units, (d) -10 units, , (a) 7 units, (c) 10 units, , 4. Consider a force F = - x$i + y$j. The, work done by this force in moving a, particle from point A(1, 0 ) to B(0, 1), along the line segment is (all quantities, are in SI units), Y, B (0, 1), , (a), , 3, 2, , (c) 1, , (b) 2, (d), , 1, 2, , X, , 1, , 2, , R, 3, , 5, , 4, , s, , B, , (b) 22.5 J, (d) 27 J, , 6. A string of length L and force constant, k is stretched to obtain extension l. It is, further stretched to obtain extension l 1 ., The work done in second stretching is, 1, kl 1 (2l + l 1 ), 2, 1, (c) k (l 2 + l 12 ), 2, (a), , 1 2, kl 1, 2, 1, (d) k (l 12 - l 2 ), 2, (b), , 7. A uniform chain of length l and mass m, is lying on a smooth table and one-third, of its length is hanging vertically down, over the edge of the table. If g is, acceleration due to gravity, work, required to pull the hanging part on to, the table is, , (c), A (1, 0), , P, , (a) 30 J, (c) 25 J, , (a) mgl, , (0, 0), , Q, A, , mgl, 9, , mgl, 3, mgl, (d), 18, (b), , 8. IfW 1 , W 2 andW 3 are the work done in, moving a particle from A and B along, three different paths 1, 2 and 3, respectively (as shown) in the
Page 114 :
CBSE New Pattern ~ Physics 11th (Term-I), , gravitational field of a point mass m, the, relation betweenW 1 , W 2 andW 3 is, B, , 1, , m, , circular path of radius 1 m. If the mass, moves with 300 rev/min, its kinetic, energy (in J) would be, , 3, A, , (b) W1 = W2 = W3, (d) W2 > W1 > W3, , 9. Amongst the given graphs which one, correctly represents the variation of the, kinetic energy (K ) of a body with, velocity (v )?, K, , K, , (b), , (a), , v, , v, , K, , K, , (d), , (c), v, , v, , 10. The kinetic energy of a body of mass, 4 kg and momentum 6 N-s will be, (a) 3.5 J, (c) 2.5 J, , (b) 5.5 J, (d) 4.5 J, , having a momentum p, which one of, the following correctly describes the, kinetic energy of the particle?, p2, 2m, , (b), , p, 2m, , (c), , v2, 2m, , (d), , (b) 100p 2, , (c) 5 p 2, , (d) zero, , 15. Two moving objects (m 1 > m 2 ) having, same kinetic energy are stopped by, application of equal retarding force., Which object will come to rest at, shorter distance?, (a), (b), (c), (d), , Bigger, Smaller, Both at same distance, Cannot say, , 16. A particle which is experiencing a, , force, is given by F = 3$i - 12$j,, undergoes a displacement of d = 4 $i., If the particle had a kinetic energy of, 3 J at the beginning of the, displacement, what is its kinetic energy, at the end of the displacement ?, (a) 9 J, , (b) 15 J, , (c) 12 J, , (d) 10 J, , from rest under the influence of a force, that varies with the distance travelled by, the particle as shown in the figure., The kinetic energy of the particle after, it has travelled 3 m is, , v, 2m, , 12. Two bodies of masses 4 kg and 5 kg are, moving with equal momentum. Then,, the ratio of their respective kinetic, energies is, (a) 4 : 5, (c) 1 : 3, , (a) 250p 2, , 17. A particle moves in one dimension, , 11. For a moving particle (mass m, velocity v), , (a), , (a) Heavy body, (b) Light body, (c) Both have same linear momenta, (d) None of the above, , 14. A mass of 5 kg is moving along a, , 2, , (a) W1 > W2 > W3, (c) W1 < W2 < W3, , 103, , Force, (in N), , same kinetic energy. Which will have, larger linear momentum?, , 2, , A, , B, , D, , 1, F, O, , (b) 2 : 1, (d) 5 : 4, , 13. A heavy body and a light body have, , C, , 3, , (a) 4 J, (c) 6.5 J, , 1, , 2, Distance, (in m), , E, 3, , (b) 2.5 J, (d) 5 J
Page 115 :
104, , CBSE New Pattern ~ Physics 11th (Term-I), , surface of the earth, then its potential, energy, , The kinetic friction force is 15 N and, spring constant is 10000 N/m. The, spring compresses by, , (a) increases, (c) remains same, , (a) 5.5 cm, (c) 11.0 cm, , 18. When a person lifts a brick above the, (b) decreases, (d) None of these, , 19. A massless spring of spring constant k,, has extension y and potential energy E., It is now stretched from y to 2y. The, increase in its potential energy is, (a) 3E, (c) E, , (b) 2E, (d) 4E, , 20. A bread gives 5 kcal of energy to a boy., How much height he can climbs by, using this energy, if his efficiency is, 28% and mass is 60 kg?, (a) 15m, (c) 2.5 m, , (b) 5m, (d) 10 m, , 25. 300 J of work is done in sliding a 2 kg, block up an inclined plane of height, 10 m (taking, g = 10 ms -2 ). Work done, against friction is, (a) 200 J, (c) zero, , potential energy U as a function of, position r for a particle of mass m . If, the particle is released from rest at, position r0 , what will its speed be at, position 3r0 ?, U(r), 3 U0, , of gravity alone in vaccum. Which of, the following quantities remain constant, during the fall?, (NCERT Exemplar), , 2 U0, U0, , Kinetic energy, Potential energy, Total mechanical energy, Total linear momentum, , 22. A stone is projected vertically up to, reach maximum height h. The ratio of, its kinetic energy to its potential energy, 4, at a height h, will be, 5, (a) 5 : 4, (c) 1 : 4, , (b) 4 : 5, (d) 4 : 1, , 23. A spring of force constant 800 N/m has, an extension of 5 cm. The work done in, extending it from 5 cm to 15 cm is, (a) 16 J, (c) 32 J, , (b) 100 J, (d) 1000 J, , 26. The graph below represents the, , 21. A body is falling freely under the action, , (a), (b), (c), (d), , (b) 2.5 cm, (d) 8.5 cm, , O, , (a), (c), , 1r0, , 2r0, , 3U0, m, 2 U0, m, , 3r0, , 4r0, , r, , 4 U0, m, 6 U0, m, , (b), (d), , 27. A pebble is attached to one end of a, string and rotated in a vertical circle. If, string breaks at the position of, maximum tension, so from the figure, shown below, it will break at, A, , (b) 8 J, (d) 24 J, , C, , D, , 24. A 2 kg block slides on a horizontal floor, with a speed of 4 m/s. It strikes a, uncompressed spring and compresses it, till the block is motionless., , B, , (a) A, , (b) B, , (c) C, , (d) D
Page 116 :
CBSE New Pattern ~ Physics 11th (Term-I), , 28. What is the ratio of kinetic energy of a, particle at the bottom to the kinetic, energy at the top, when it just loops a, vertical loop of radius r?, (a) 5 :1, (c) 5 :2, , (b) 2 : 3, (d) 7 :2, , 29. A man can do work of 600 J in 2 min,, then man’s power is, (a) 7.5 W, (c) 5 W, , (b) 10 W, (d) 15 W, , 30. A particle is acted by a constant power., Then, which of the following physical, quantity remains constant?, (a), (b), (c), (d), , Speed, Rate of change of acceleration, Kinetic energy, Rate of change of kinetic energy, , 31. An object of mass m moves, horizontally, increasing in speed from, 0 to v in a time t . The power necessary, to accelerate the object during this time, period is, (a), , mv 2 t, 2, , (c) 2 mv 2, , mv 2, 2, mv 2, (d), 2t, (b), , 32. A 60 HP electric motor lifts an elevator, having a maximum total load capacity, of 2000 kg. If the frictional force on the, elevator is 4000 N, the speed of the, elevator at full load is close to (take,, 1 HP = 746 W and g = 10 ms -2 ), (a) 2.0 ms -1, (c) 1.9 ms -1, , (b) 1.5 ms -1, (d) 1.7 ms -1, , 33. A car of mass m starts from rest and, accelerates, so that the instantaneous, power delivered to the car has a, constant magnitude P 0 . The, instantaneous velocity of this car is, proportional to, (a) t 2 P0, (c) t -1 / 2, , (b) t 1 / 2, (d) t / m, , 105, , 34. For a system to follow the law of, conservation of linear momentum, during a collision, the condition is, I. total external force acting on the, system is zero, II. total external force acting on the, system is finite and time of collision, is negligible, III. total internal force acting on the, system is zero, (a) Only I, , (b) Only II, , (c) Only III (d) I or II, , 35. Two identical balls A and B having, , velocities of 0.5 ms -1 and -0.3 ms -1, respectively, collide elastically in one, dimension. The velocities of B and A, after the collision respectively will be, (a), (b), (c), (d), , -05, . ms -1 and 0.3 ms -1, 0.5 m/s -1 and -0.3 ms -1, -0.3 ms -1 and 0.5 ms -1, 0.3 ms -1 and 0.5 ms -1, , 36. A particle of mass 1g moving with a, , velocity v 1 = ( 3$i - 2$j) ms -1 experiences, a perfectly elastic collision with another, particle of mass 2 g and velocity, v 2 = ( 4 $j - 6 k$ ) ms -1 . The velocity of the, particle is, (a) 2.3 ms-1, (c) 9.2 ms-1, , (b) 4.6 ms-1, (d) 6 ms-1, , 37. A particle of mass m 1 moves with, velocity v 1 collides with another particle, at rest of equal mass. The velocity of, second particle after the elastic collision, is, (a) 2v 1, , (b) v 1, , (c) - v 1, , (d) zero, , 38. During inelastic collision between two, bodies, which of the following, quantities always remain conserved?, (NCERT Exemplar), , (a), (b), (c), (d), , Total kinetic energy, Total mechanical energy, Total linear momentum, Speed of each body
Page 117 :
106, , CBSE New Pattern ~ Physics 11th (Term-I), , 39. Two objects of mass m each moving, -1, , with speed u ms collide at 90°, then, final momentum is (assume collision is, inelastic), (a) mu, (c) 2 mu, , (b) 2 mu, (d) 2 2 mu, , 40. A body of mass 5 ´ 10 3 kg moving with, speed 2 ms -1 collides with a body of, mass 15 ´ 10 3 kg inelastically and sticks, to it. Then, loss in kinetic energy of the, system will be, (a) 7.5 kJ (b) 15 kJ, , (c) 10 kJ (d) 5 kJ, , 41. If the linear momentum of a body is, increased by 50%, then the kinetic, energy of that body increases by, ……… ., (a) 100%, , (b) 125%, , (c) 225% (d) 25%, , 42. A ball of mass m moves with speed v, and strikes a wall having infinite mass, and it returns with same speed, then, the work done by the ball on the wall, is ……… ., (a) zero, (c) m / v J, , (b) mv J, (d) v/mJ, , 43. A body of mass 5 kg is thrown, vertically up with a kinetic energy of, 490 J. The height at which the kinetic, energy of the body becomes half of the, original value is ……… ., (a) 12.5 m, (c) 2.5 m, , (b) 10 m, (d) 5 m, , 44. If two persons A and B take 2 s and 4 s,, respectively to lift an object to the same, height h, then the ratio of their powers, is ……… ., (a) 1 :2, (c) 2 :1, , (b) 1 :1, (d) 1 : 3, , 45. At time t = 0, particle starts moving, along the x-axis. If its kinetic energy, increases uniformly with time t , the, net force acting on it must be, , proportional to t n , where the value of, n is ……… ., (a) 1, (c) 2, , (b) - 1/2, (d) 1/2, , 46. A man of mass m, standing at the, bottom of the staircase, of height L, climbs it and stands at its top. Which, amongst the following statement is, (NCERT Exemplar), correct?, (a) Work done by all forces on man is equal to, the rise in potential energy mgL., (b) Work done by all forces on man is zero., (c) Work done by the gravitational force on, man is mgL., (d) The reaction force from a step does some, work because the point of application of, the force does not move while the force, exists., , 47. Which of the following statement is, correct about non-conservative force?, (a) It depends on velocity of the object., (b) It depends on the particular path taken by, the object., (c) It depend on the initial and final positions of, the object., (d) Both (a) and (b), , 48. Which of the following statement is, correct?, (a) Conservation of mechanical energy does, not consider only conservative force., (b) Conservation of energy consider both, conservative and non-conservative forces., (c) Conservation of energy consider only, conservative force., (d) Mass converted into energy in nuclear, reaction is called mass-defect., , 49. Which of the following statement does, not specify an example of perfectly, inelastic collision?, (a) A bullet fired into a block if bullet gets, embedded into block., (b) Capture of electrons by an atom., (c) A man jumping on to moving boat., (d) A ball bearing striking another ball bearing.
Page 118 :
CBSE New Pattern ~ Physics 11th (Term-I), , 50. Match the Column I (angle) with, Column II (work done) and select the, correct option from the codes given, below., Column I, , Column II, , A., , q < 90°, , p., , Friction, , B., , q = 90°, , q., , Satellite rotating, around the earth, , C., , q > 90°, , r., , Coolie is lifting a, luggage, , Codes, A, , B, , C, , (a) p, , q, , r, , (b) r, , q, , p, , (c) p, , r, , q, , (d) r, , p, , q, , Column I, , Column II, , A., , Velocity is, proportional to, , p., , t, , B., , Displacement is, proportional to, , q., , t2, , C., , Work done is, proportional to, , r., , t3, , A, , B, , C, , q, , r, , (b) r, , q, , p, , (c) p, , q, , q, , (d) r, , p, , p, , For question numbers 52 to 60, two, statements are given-one labelled, Assertion (A) and the other labelled, Reason (R). Select the correct answer to, these questions from the codes (a), (b), (c), and (d) are as given below, (a) Both A and R are true and R is the, correct explanation of A., (b) Both A and R are true but R is not the, correct explanation of A., (c) A is true but R is false., (d) A is false and R is also false., , =, , by a machine delivering a power, proportional to time (P µ t ). Then,, match the Column I with Column II, and select the correct option from the, codes given below., , (a) p, , Assertion-Reasoning MCQs, , 52. Assertion Stopping distance, , 51. A body is moved along a straight line, , Codes, , 107, , Kinetic energy, , Stopping force, Reason Work done in stopping a body, is equal to change in kinetic energy of, the body., , 53. Assertion A spring of force constant k, is cut into two pieces having lengths in, the ratio 1 : 2. The force constant of, series combination of the two parts is, 2k / 3., Reason The spring connected in series, are represented by k = k1 + k 2 ., , 54. Assertion According to the law of, conservation of mechanical energy,, change in potential energy is equal and, opposite to the change in kinetic, energy., Reason Mechanical energy is not, conserved., , 55. Assertion Decrease in mechanical, energy is more in case of an object, sliding up a relatively less inclined, plane due to friction.
Page 119 :
108, , CBSE New Pattern ~ Physics 11th (Term-I), , Reason The coefficient of friction, between the block and the surface, decreases with the increase in the angle, of inclination., , 56. Assertion For looping a vertical loop of, , the force and the displacement over which it, acts. Consider a constant force F acting on, an object of mass m. The object undergoes a, displacement d in the positive x-direction as, shown in figure., , radius r, the minimum velocity at lowest, point should be 5gr ., , F, θ, , Reason In this event, the velocity at the, highest point will be zero., , 57. Assertion Kilowatt-hour is the unit of, energy., Reason One kilowatt hour is equal to, . ´ 10 6 J., 36, , 58. Assertion There is no loss in energy in, elastic collision., Reason Linear momentum is conserved, in elastic collision., , 59. Assertion Quick collision between two, bodies is more violent than a slow, collision; even when the initial and final, velocities are identical., Reason The momentum is greater in, first case., , 60. Assertion Two particles are moving in, the same direction do not lose all their, energy in completely inelastic collision., Reason Principle of conservation of, momentum does not holds true for all, kinds of collisions., , Case Based MCQs, Direction Answer the questions from, 61-65 on the following case., Work, A farmer ploughing the field, a construction, worker carrying bricks, a student studying for a, competitive examination, an artist painting a, beautiful landscape, all are said to be working., In physics, however, the word ‘Work’ covers a, definite and precise meaning. Work refers to, , x, d, , The work done by the force is defined to be, the product of component of the force in the, direction of the displacement and the, magnitude of this displacement, thus, W = ( F cos q ) d = F × d., , 61. The earth is moving around the sun in, a circular orbit, is acted upon by a, force and hence work done on the, earth by the force is, (a), (b), (c), (d), , zero, positive, negative, None of the above, , 62. In which case, work done will be, zero?, (a) A weight-lifter while holding a weight of, 100 kg on his shoulders for 1 min, (b) A locomotive against gravity is running on, a level plane with a speed of 60 kmh - 1, (c) A person holding a suitcase on his head, and standing at a bus terminal, (d) All of the above, , 63. Find the angle between force, , F = ( 3$i + 4 $j - 5k$ ) unit and, displacement d = (5$i + 4 $j + 3k$ ) unit., (a) cos-1 (0.49), (c) cos-1 (0.60), , (b) cos-1 (0.32), (d) cos-1 (0.90), , 64. Which of the following statement(s), is/are correct for work done to be, zero?, I. If the displacement is zero., II. If force applied is zero.
Page 120 :
CBSE New Pattern ~ Physics 11th (Term-I), , 109, , (a) Only I, (c) Only II, , (b), , (a), , (b) I and II, (d) I, II and III, , t, , 65. A proton is kept at rest. A positively, charged particle is released from rest at, a distance d in its field. Consider two, experiments; one in which the charged, particle is also a proton and in another,, a positron. In same time t , the work, done on the two moving charged, particles is, (a) same as the same force law is involved in, the two experiments, (b) less for the case of a positron, as the, positron moves away more rapidly and the, force on it weakens, (c) more for the case of a positron, as the, positron moves away a larger distance, (d) same as the work is done by charged, particle on the stationary proton, , Direction Answer the questions from, 66-70 on the following case., Kinetic Energy, The energy possessed by a body by virtue of, its motion is called kinetic energy. In other, words, the amount of work done, a moving, object can do before coming to rest is equal to, its kinetic energy., 1, \ Kinetic energy, KE = mv 2, 2, where, m is a mass and v is the velocity of a, body., The units and dimensions of KE are Joule (in, SI) and [ML 2 T -2 ], respectively., Kinetic energy of a body is always positive. It, can never be negative., , 66. Which of the diagrams shown in figure, most closely shows the variation in, kinetic energy of the earth as it moves, once around the sun in its elliptical, orbit?, , KE, , KE, , III. If force and displacement are, mutually perpendicular to each other., , KE, , (c), , KE, , (d), , 67. A force which is inversely proportional, to the speed is acting on a body. The, kinetic energy of the body starting from, rest is, (a) a constant, (b) inversely proportional to time, (c) directly proportional to time, (d) directly proportional to square of time, , 68. The kinetic energy of an air molecule, (10 -21 J) in eV is, (a) 6.2 meV, (c) 10.4 meV, , (b) 4.2 meV, (d) 9.7 meV, , 69. Two masses of 1 g and 4 g are moving, with equal kinetic energy. The ratio of, the magnitudes of their momentum is, (a) 4 : 1, (c) 1 : 2, , (b) 2 :1, (d) 1 : 16, , 70. An object of mass 10 kg is moving with, velocity of 10 ms -1 . Due to a force, its, velocity become 20 ms -1 . Percentage, increase in its KE is, (a) 25%, (c) 75%, , (b) 50%, (d) 300%, , Direction Answer the questions from, 71-75 on the following case., PE of Spring, There are many types of spring. Important, among these are helical and spiral springs as, shown in figure., (a), , (b)
Page 121 :
110, , CBSE New Pattern ~ Physics 11th (Term-I), , Usually, we assume that the springs are, massless. Therefore, work done is stored in, the spring in the form of elastic potential, energy of the spring. Thus, potential energy, of a spring is the energy associated with the, state of compression or expansion of an, elastic spring., , 71. The potential energy of a body is, increases in which of the following, cases?, (a), (b), (c), (d), , If work is done by conservative force, If work is done against conservative force, If work is done by non-conservative force, If work is done against non- conservative, force, , 72. The potential energy, i.e. U (x ) can be, assumed zero when, (a) x = 0, (b) gravitational force is constant, (c) infinite distance from the gravitational, source, (d) All of the above, , 73. The ratio of spring constants of two, springs is 2 : 3. What is the ratio of their, potential energy, if they are stretched, by the same force?, (a) 2 : 3, (b) 3 : 2, (c) 4 : 9, (d) 9 : 4, , Direction Answer the questions from, 76-80 on the following case., Principle of Conservation of Energy, Total energy of an isolated system always, remains constant. Since, the universe as a, whole may be viewed as an isolated system,, total energy of the universe is constant. If one, part of the universe loses energy, then other, part must gain an equal amount of energy., The principle of conservation of energy, cannot be proved as such. However, no, violation of this principle has been observed., , 76. When we rub two flint stones together,, got them to heat up and to ignite a heap, of dry leaves in the form of, (a) chemical energy, (c) heat energy, , 77. Which graph represents conservation of, total mechanical energy?, Energy, , –xm, , U, xm, , E=K+U, , X, , Energy, U, , (b), –xm, , increases by 15 J when stretched by, 3 cm. If it is stretched by 4 cm, the, increase in potential energy is, (b) 30 J, (d) 36 J, , stretched through a distance x is 10 J., What is the amount of work done on, the same spring to stretch it through an, additional distance x?, (b) 20 J, (d) 40 J, , K, xm, Energy, U, , (c), –xm, , 75. The potential energy of a spring when, , (a) 10 J, (c) 30 J, , K, , (a), , 74. The potential energy of a spring, , (a) 27 J, (c) 33 J, , (b) sound energy, (d) electrical energy, , K, xm, , K=E, , X, , E=K+U, , X, , Energy, U, , (d), –xm, , K, xm, , E=K+U, , X
Page 122 :
CBSE New Pattern ~ Physics 11th (Term-I), , 78. In the given curved road, if particle is, released from A, then, , 111, , 79. U is the potential energy, K is the, kinetic energy and E is the mechanical, energy. Which of the following is not, possible for a stable system?, , m A, , (a) U > E, h, , (b) U < E, , (c) E > K, , (d) K > E, , 80. A body of mass 5 kg is thrown, , B, , (a) kinetic energy at B must be mgh, (b) kinetic energy at B must be zero, (c) kinetic energy at B must be less than mgh, (d) kinetic energy at B must not be equal to, potential energy, , vertically up with a kinetic energy of, 490 J. The height at which the kinetic, energy of the body becomes half of the, original value is, (a) 12.5 m, (c) 2.5 m, , (b) 10, (d) 5 m, , ANSWERS, Multiple Choice Questions, 1. (c), 11. (a), 21. (c), , 2. (c), 12. (d), 22. (c), , 3. (b), 13. (a), 23. (b), , 4. (c), 14. (a), 24. (a), , 5. (b), 15. (c), 25. (b), , 6. (d), 16. (b), 26. (c), , 7. (d), 17. (c), 27. (b), , 8. (b), 18. (a), 28. (a), , 9. (c), 19. (a), 29. (c), , 10. (d), 20. (d), 30. (d), , 31. (d), 41. (b), 51. (c), , 32. (c), 42. (a), , 33. (b), 43. (d), , 34. (a), 44. (c), , 35. (b), 45. (b), , 36. (b), 46. (b), , 37. (b), 47. (d), , 38. (c), 48. (b), , 39. (c), 49. (d), , 40. (a), 50. (b), , 54. (d), , 55. (c), , 56. (c), , 57. (b), , 58. (b), , 59. (a), , 60. (c), , 63. (b), 73. (b), , 64. (d), 74. (a), , 65. (c), 75. (c), , 66. (d), 76. (a), , 67. (c), 77. (c), , 68. (a), 78. (a), , 69. (c), 79. (a), , Assertion-Reasoning MCQs, 52. (a), , 53. (c), , Case Based MCQs, 61. (a), 71. (b), , 62. (d), 72. (d), , 70. (d), 80. (d), , SOLUTIONS, 1. Here, work is done by the frictional force on, the cycle = - 200 ´ 10 = - 2000 J., As the road is not moving, hence work done, by the cycle on the road is zero., , 2. Given, F = 50 N, W = 150 J, and s = 3 m, Work done, W = Fs cos q, 150 = 50 ´ 3 ´ cos q, 150, cos q =, =1, 150, Þ, q = 0°, , 3. Net force, F = 2$i + 3$j - 2k$ + 5$i - $j - 3k$, = 7 $i + 2$j - 5k$, Displacement, d = 2i$ - $j + 3k$ - $i - $j - k$, = i$ - 2$j + 2k$, Work done = F × d = ( 7 i$ + 2$j - 5k$ ) × ( $i - 2$j + 2k$ ), = 7 - 4 - 10 = - 7 units, , 4. Work done by a variable force on the, particle,, W = ò F × dr = ò F × ( dxi$ + dy$j)
Page 123 :
112, , CBSE New Pattern ~ Physics 11th (Term-I), , \ In two dimension, dr = dx$i + dy$j, and it is given F = - xi$ + y$j, W = ò ( - x$i + y$j) × ( dxi$ + dy$j), , \, , = ò - x dx + y dy, = ò - x dx +, , ò y dy, , As particle is displaced from A(1, 0 ) to B( 0, 1),, so x varies from 1 to 0 and y varies from 0 to, 1., So, with limits, work will be, 0, , W = ò - x dx +, 1, , 0, , ò, , 1, , 0, , y dy, 1, , é - x2 ù, é y2 ù, =ê, +ê ú, ú, ë 2 û1 ë 2 û0, 1, = [ 0 - ( - 1) 2 + (1) 2 - 0 ] = 1 J, 2, , 5. Work done = Area under F -s curve, WAB = W12 + W23 + W34 + W45, = Area under AP + Area under PQ, + Area under QR - Area above RB, 1, = 10 ´ 1 + (10 + 15), 2, 1, 1, ´ 1 + ´ 1 ´ 15 - ´ 1 ´ 15, 2, 2, = 10 + 12.5 = 22.5 J, , 6. Work done in stretching a string to obtain an, extension l,, 1 2, kl, 2, Similarly, work done in stretching a string to, obtain an extension l 1 is, 1, W2 = kl 12, 2, \ Work done in second case,, 1, W = W2 - W1 = k ( l 12 - l 2 ), 2, l, 7. The weight of hanging part æç ö÷ of chain is, è 3ø, æ1 ö, ç mg ÷ . This weight acts at the centre of, è3 ø, W1 =, , gravity of the hanging part, which is at a, æl ö, distance of ç ÷ from the table., è6ø, Hence, work required to pull hanging part,, , \, , W = force ´ displacement, mg l mgl, W =, ´ =, 3 6 18, , 8. Gravitational force is a conservative force, and work done by or against the force in, moving a body depends only on the initial, and final positions of the body and not on, the nature of path followed by it., So, W1 = W2 = W3, 1, 9. As we know that, KE = mv 2, 2, So, kinetic energy is directly proportional to, the square of velocity., K µv 2, As this equation resembles equation of, parabola as m is constant, hence option (c), represents a parabola., , 10. The kinetic energy K and momentum p of a, body are related as, p2, , where m is the mass of the body, K =, 2m, Here, p = 6 N-s and m = 4 kg, (6 )2, K =, = 4.5 J, 2´4, , 11. The kinetic energy of the particle is, 1, K = mv 2, 2, As, momentum, p = mv, or, p 2 = m 2v 2, p2, or, v2 = 2, m, 1 æ p2 ö p2, \, K = mç 2÷ =, 2 èm ø 2m, p2, 2m, where, p is the momentum and m is the mass, of the body., As,, (given), p1 = p2, K1 m2 5, \, =, =, K2 m1 4, , 12. Kinetic energy of a body, K =, , 13. Kinetic energy of a body, K =, or, , p = 2mK, , p2, 2m
Page 124 :
CBSE New Pattern ~ Physics 11th (Term-I), , (given), Since,, KH =KL, where, subscripts H and L represents for, heavy and light bodies., pH, mH, \, =, pL, mL, So,, and, , mH >mL, pH > pL, , 14. Given, mass, m = 5 kg, Radius, R = 1 m, Revolution per minute, w = 300 rev/min, = ( 300 ´ 2p ) rad/min, 300 ´ 2 ´ p, rad/s = 10 p rad/s, =, 60, Linear speed, v = w R = 10 p ´ 1, = 10p m/s, 1, 2, \ KE = mv, 2, 1, = ´ 5 ´ (10 p ) 2, 2, 1, = 100 p 2 ´ 5 ´, 2, = 250 p 2 J, , 15. Applying work-energy theorem on both, moving objects,, 1, m 1v 12 = Fx 1, 2, 1, and, m 2v 22 = Fx 2, 2, Since, both moving objects have same kinetic, energy,, 1, 1, i.e., m 1v 12 = m 2v 22 Þ Fx 1 = Fx 2, 2, 2, Þ, x1 = x2, Therefore, both the objects will come to rest, at the same distance., , 16. Work done in F is given by DW = F × d, By substituting given values, we get, Þ, DW = ( 3i$ - 12$j ) × ( 4 $i ), … (i), Þ, DW = 12 J, Now, using work-energy theorem, we get, work done, DW = change in kinetic, energy, DK, or, … (ii), DW = K 2 - K 1, , 113, Comparing Eqs. (i) and (ii), we get, K 2 - K 1 = 12 J or K 2 = K 1 + 12 J, Given, initial kinetic energy, K 1 = 3 J, \ Final kinetic energy, K 2 = 3 J + 12 J = 15 J, , 17. \ Work done on the particle, = Area under the curve ABC, W = Area of square ABFO + Area of DBCD, + Area of rectangle BDEF, 1, = 2 ´ 2 + ´ 1 ´ 1 + 2 ´ 1 = 6.5 J, 2, Now, from work-energy theorem,, DW = K f - K i, (Q K i = 0), Þ K f = DW = 6.5 J, , 18. Potential energy of brick above the earth’s, surface is given by, U = mgh, i.e., U µh, Hence, when a brick is lifted above the, surface of the earth, then its potential energy, increases., , 19. The potential energy of the spring is, 1 2, …(i), ky, 2, Now, it is stretched from y to 2y, so its, potential energy becomes, 1, E ¢ = k ( 2y ) 2, 2, [using Eq. (i)], = 2ky 2 = 4 E, \ The increase in its potential energy is, DE = E ¢ - E = 4 E - E = 3E, E =, , 20. Energy received by the boys from bread, = 5000 cal = 5000 ´ 4.2, = 21 ´ 10 3 J, According to law of conservation of, mechanical energy,, 28, mgh =, ´ 21 ´ 10 3, 100, 28 ´ 21 ´ 10 3, \, h =, = 10 m, 100 ´ 9.8 ´ 60, , 21. As the body is falling freely under gravity,, the potential energy decreases and kinetic, energy increases but total mechanical energy, (PE + KE) of the body and earth system will, be constant as external force on the system is, zero.
Page 125 :
114, , CBSE New Pattern ~ Physics 11th (Term-I), , 22. At a height, , 4, h,, 5, , 26. According to the law of conservation of, , 4, 4, Potential energy = mg ´ h = mgh, 5, 5, Total energy = mgh, \ Kinetic energy at that height, 4, 1, =mgh - mgh = mgh, 5, 5, 4, \ At a height h, the ratio of, 5, 1, mgh, KE 5, 1, =, =, 4, PE, mgh 4, 5, , 23. The work done on the spring is stored as the, PE of the body and is given by, U =ò, or, , x2, x1, , U =ò, , x2, x1, , F ext dx, kx dx, , 1, k ( x 22 - x 12 ), 2, 800, =, [( 015, . ) 2 - ( 0.05) 2 ], 2, = 400 (0.2 ´ 0.1) = 8 J, =, , 24. According to work-energy theorem,, loss in kinetic energy = work done against, friction + potential energy of spring, 1, 1, mv 2 = f × x + kx 2, 2, 2, 1, 1, 4, ´ 2 ( 4 ) = 15 x + ´ 10000 x 2, Þ, 2, 2, Þ 5000 x 2 + 15x - 16 = 0, \, x = 0.055 m = 5.5 cm, , 25. Net work done in sliding a body up to a, height h on an inclined plane, = Work done against the gravitational, force + Work done against the frictional force, …(i), Þ, W = Wg + W f, But, W = 300 J, Given, m = 2 kg and h = 10 m, Wg = mgh = 2 ´ 10 ´ 10 = 200 J, Putting these values in Eq. (i), we get, 300 = 200 + W f, Þ, W f = 300 - 200 = 100 J, , energy, U i + K i = U f + K f, So, by putting the values in Eq. (i),, 1, 3U 0 + 0 = 2U 0 + mv 2, 2, 2U 0, Þ, v =, m, , ...(i), , 27. FBD of pebble,, A, , C, , D Pebble, mg cosθ, B, mg sinθ, mg, , mv 2, mv 2, \ T - mg cos q =, Þ T = mg cos q +, l, l, Tension is maximum, when cos q = 1 and, velocity is maximum. Both conditions are, satisfied at q = 0°, i.e. at lowest point B., , 28. At top point, the tension (T H ) in string, becomes zero, so velocity of the particle is, v H = gr, At the bottom, the velocity of the particle is, v L = 5gr, Therefore, the ratio of kinetic energies at, bottom and top is, 1, mv L2 æ ö 2, KL, v, = 2, =ç L÷, K H 1 mv 2 è v H ø, H, 2, 5 gr 5, =, = = 5 :1, 1, gr, Hence, the ratio of kinetic energies is 5 : 1., , 29. Given, W = 600 J, and t = 2 min = 2 ´ 60 = 120 s, W 600, \ Power, P =, =, =5W, t, 120, dW, 30. By definition, P =, dt, Q Work done = Kinetic energy, dW d (KE), P=, =, = constant, Þ, dt, dt
Page 126 :
CBSE New Pattern ~ Physics 11th (Term-I), , W, t, Since, K i = initial KE = 0, 1, and, K f = final KE = mv 2, 2, From work-energy theorem,, work done = change in KE, 1 2, mv - 0, K f -K i, \, P =, = 2, t, t, mv 2, Þ, P =, 2t, , 36. From conservation of momentum,, , 31. Power, P =, , m 1 v1 + m 2 v2 = (m 1 + m 2 ) v, 1 ´ ( 3i$ - 2$j) + 2 ´ ( 4 $j - 6 k$ ) = (1 + 2) v, Þ 3$i + 6 $j - 12k$ = 3 v, \ Velocity,, , For elastic collision,, æm - m 1 ö, 2m 1v 1, v2 = ç 2, ÷ v2 +, èm 1 + m 2 ø, m1 + m2, , Þ P0 × dt = mvdv, Integrating both sides, we get, , Þ, Þ, Þ, , P0 dt = ò mv dv, mv 2, 2, 2P0 t, 2, v =, m, v µ t 1/ 2, , P0 t =, , 34. From Newton’s second law, F =, , After putting given values, we will get, 2m 1v 1, v2 =, Þ v2 =v1, 2m 1, , 38. When we are considering the two bodies as, system, the total external force on the system, will be zero., Hence, total linear momentum of the system, remain conserved., , 39. Speed of objects = u ms -1, Since, both objects collide with 90°., According to the law of conservation of, momentum,, Total moment before collision, = Total momentum after collision, |mui$ + mu$j| = p, f, , m u + m u = p f Þ p f = 2 mu, 2 2, , 2 2, , 40. Given, mass of body, m 1 = 5 ´ 10 3 kg, dp, dt, , dp, =0, dt, Þ p = constant, Thus, if total external force acting on the, system is zero during collision, then the, linear momentum of the system remains, conserved., , If, , v = | v| = 1 + 4 + 16 = 4.6 ms -1, , 37. Given, mass, m 1= m 2 = m and velocity, v = v 1, , to pull the lift,, F = weight carried + friction = mg + f, = ( 2000 ´ 10 ) + 4000 = 24000 N, Power delivered by motor at speed v of load,, P = F ´v, P 60 ´ 746, Þ, v =, =, = 1865, ., = 1.9 ms -1, F, 24000, dv, dv, 33. As, P0 = Fv = æçm ö÷ v = mv, è dt ø, dt, , 0, , v = $i + 2$j - 4 k$, , Þ, , 32. At maximum load, force provided by motor, , ò, , 115, , F = 0, then, , and mass of another body, m 2 = 15 ´ 10 3 kg, Velocity, v 1 = 2 ms -1, For perfectly inelastic collision ( e = 0 ),, Loss in kinetic energy of system,, 1 m 1m 2, DE K =, ´ v 12, 2m1 + m2, =, , 35. As, we know in a elastical collision of two, identical bodies, i.e. m A = m B , the particles, mutually exchange their velocities., So, ( v i ) A = 0.5 ms -1 and ( v i ) B = -0.3 ms -1 ., After collision,, ( v f ) A = - 0.3 ms -1 and ( v f ) B = 0.5 ms -1 ., , 1 5 ´ 10 3 ´ 15 ´ 10 3, ´, ´ 22, 2 5 ´ 10 3 + 15 ´ 10 3, , = 7.5 ´ 10 3 J = 7.5 kJ, , 41. Kinetic energy of the body,, , p2, 2m, Since, the mass remains constant, so K µ p 2 ., K =
Page 127 :
116, , CBSE New Pattern ~ Physics 11th (Term-I), , 2, , Hence, total work done = - mgL + mgL = 0., As the point of application of the contact, forces does not move, hence work done by, reaction forces will be zero., , K 2 p 22 é150 ù, 9, =, =, =, K 1 p 12 êë100 úû, 4, , \, , æK, ö, ö, æ9, Thus, ç 2 - 1÷ ´ 100 = ç - 1÷ ´ 100, ø, è4, èK 1, ø, = 125%, , 47. If the work done or the kinetic energy, , 42. As, work done = force ´ displacement, As, there is no displacement produced in the, wall, so work done by the ball on the wall is, zero., Alternative Method As, there is no change, in kinetic energy of the ball, so according to, work-energy theorem, work done should be, zero., , 43. According to the law of conservation of, energy,, 1, 1 æ1, ö, mv 2 = ç mv 2 ÷ + mgh, ø, 2, 2è2, Þ, , 490 = 245 + 5 ´ 9.8 ´ h ,, 245, h =, = 5m, 49, , ÞPA : PB =, , …(i), , mgh 1 /t 1 æ h 1 ö æ t 2 ö t 2 4 2, =ç ÷ç ÷= = =, mgh 2 /t 2 è h 1 ø è t 1 ø t 1 2 1, , PA : PB = 2 : 1, dk, 45. Given, k µ t Þ, = constant, dt, Þ, K µt, 1 2, mv µ t Þ v µ t, 2, dK, Also, P = Fv =, = constant, dt, 1, 1, F µ Þ, F µ, Þ, v, t, Þ, , Þ, , 48. In elastic collision, the conservation of, mechanical energy consider only, conservative force while conservation of, energy consider both conservative and, non-conservative force., Mass converted into energy in nuclear, reaction is called nuclear energy., Thus, the statement given in option (b) is, correct, rest are incorrect., , 49. Whenever there is a collision between two, , 44. Given, t 1 = 2 s, t 2 = 4 s, and h 1 = h 2 = h, mgh 1, mgh 2, and PB =, As, PA =, t1, t2, , depend on other factors such as the velocity, or the particular path taken by the object, the, force would be called non-conservative., Thus, the statements given in options (a) and, (b) are correct, rest is incorrect., , F µ t -1 / 2, , 46. When a man of mass m climbs up the, staircase of height L, work done by the, gravitational force on the man is mgl, work, done by internal muscular forces will be mgL, as the change in kinetic energy is almost, zero., , bodies, the total momentum of the bodies, remains conserved. If after the collision of, two bodies, the total kinetic energy of the, bodies remains the same as it was before, collision, then it a perfectly elastic collision., A ball bearing striking another ball bearing is, an example of elastic collision. If two bodies, strick together after the collision, then the, collision is said to be perfectly inelastic, collision., Options (a), (b) and (c) are examples of, perfectly inelastic collisions., , 50. Work done by an agent is given by, W = F × s = Fs cos q, where, F is the applied force, s is the, displacement and q is the smaller angle, between F and s ., A. If q < 90° , i.e. acute angle, then work done, is positive, as in case of coolie lifting, luggage., B. If q = 90° , i.e. right angle, then work done, is zero, as in case of satellite rotation, around the earth., C. If q > 90° , i.e. obtuse angle, work done is, negative, as in case of friction., Hence, A ® r, B ® q and C ® p.
Page 128 :
CBSE New Pattern ~ Physics 11th (Term-I), , 51. As, power, P µ t, So,, , W = ò Pdt = ò at dt, , or, W µt2, Since, work done is equal to change is KE., Hence, v 2 µ t 2 or v µ t, ds, Further, v =, dt, ds, \, µ t or ds µ t dt, dt, (by integration), or, s µt2, Hence, A ® p, B ® q and C ® q., , 52. According to work-energy theorem, work, done by a body is equal to change in kinetic, energy of the body., 1, …(i), Þ, W = DKE = mv 2, 2, But, W = stopping force ´ stopping distance, …(ii), W = F ×d, From Eqs. (i) and (ii), we have, Stopping distance (d), ö, æ1, Kinetic energy ç mv 2 ÷, ø, è2, =, Stopping force ( F ), Therefore, both A and R are true and R is, the correct explanation of A., F, 1, 53. As we know, k =, Þ k µ, l, l, k 2 l1 1, = =, Þ, k 1 l2 2, , In series,, , k 1 = 2k , k 2 = k, 1 1, 3, 1, 1, 1, =, +, =, + =, k ¢ k 1 k 2 2k k 2k, , 2k, 3, Therefore, A is true but R is false., , Q, , k¢=, , 54. According to the law of conservation of, mechanical energy, for conservative forces,, the sum of kinetic energy and potential, energy remains constant and throughout the, motion it is independent of time., This is the law of conservation of mechanical, energy, i.e. KE + PE = total energy =, constant., Therefore, A is false and R is also false., , 117, 55. Mechanical energy consists of both PE and, KE. In the given cases, some of the, mechanical energy is converted into heat, energy and it is more in the case when, inclination is less due to increased (as q, decreases, value of cos q will increases), friction force on an inclined plane., f r = mmg cos q, The coefficient of friction does not depend, on the angle of inclination of the plane. It, depends only on the nature of surfaces in, contact., Therefore, A is true but R is false., , 56. At the lowest point of a vertical circle, the, minimum velocity at bottom,, v min = 5gr, Velocity at highest point, v = gr, Therefore, A is true but R is false., Work done (or energy), 57. Power =, Time, Þ Work done = Power ´ Time, W = P ´t, If, P = 1 kilowatt, t = 1 hour, then, W = 1 kilowatt ´ 1 hour, = 1 kilowatt-hour, = 10 3 watt ´ 60 ´ 60 s, . ´ 10 6 J, = 36, Therefore, both A and R are true but R is, not the correct explanation of A., , 58. In elastic collision, total energy, kinetic energy, and momentum remain conserved, therefore, no loss in energy occurs in elastic collision., Therefore, both A and R are true but R is, not the correct explanation of A., , 59. As momentum, p = mv or p µ v , i. e., momentum is directly proportional to its, velocity, so the momentum is greater in a, quicker collision between two bodies than in, slower one., Hence, due to greater momentum quicker, collision between two bodies will be more, violent even initial and final velocities are, identical., Therefore, both A and R are true and R is, the correct explanation of A.
Page 129 :
118, , CBSE New Pattern ~ Physics 11th (Term-I), , 60. If two particles are initially moving in the, same direction, then their resultant, momentum will not be zero. Therefore, their, resultant momentum cannot be zero after a, completely inelastic collision., As, kinetic energy is directly proportional to, the square of the momentum, hence kinetic, energy cannot be zero. This implies, not all, the energy in inelastic collision is lost., Therefore, A is true but R is false., , 61. When earth is moving around the sun in a, circular orbit, then gravitational attraction on, earth due to the sun provides required, centripetal force, which is in radially inward, direction, i. e. in a direction perpendicular to, the direction of motion of the earth in its, circular orbit around the sun., As a result, the work done on the earth by, the force will be zero. i.e. W = Fd cos 90° = 0., , 62. Work done by weight-lifter is zero, because, there is no displacement., In a locomotive, work done is zero because, force due to gravity and displacement are, mutually perpendicular to each other., In case of a person holding a suitcase on his, head and standing at a bus terminal, work, done is zero because there is no, displacement., Hence, options (a), (b) and (c) are correct., 63. Given, F = ( 3$i + 4 $j - 5k$ ) unit, and, d = ( 5i$ + 4 $j + 3k$ ) unit, \, , F × d = F x d x + F yd y + F z d z, = 3 ( 5) + 4 ( 4 ) + ( -5) ( 3), = 16 units, , Now,, , F × F = F 2 = F x2 + F y2 + F z2, = 9 + 16 + 25, = 50 units, , Þ, and, , F = 50 units, d × d = d 2 = d x2 + d 2y + d z2, = 25 + 16 + 9, , Þ, \, , = 50 units, d = 50 units, 16, cos q =, 50 50, , =, , 16, = 0.32, 50, , F × dö, æ, çQ cos q =, ÷, è, Fd ø, , q = cos -1 ( 0.32), , Þ, , 64. The work done in displacing an object by, applying force F is given by, W = F × s = Fs cos q, So, work done will be zero, when, (i) either applied force F or displacement s is, zero., (ii) the force and displacement are mutually, perpendicular to each other. i.e. q = 90°., So, all statements are correct., , 65. Force between two protons is same as that of, between proton and a positron., As positron is much lighter than proton, it, moves away through much larger distance, compared to proton., We know that, work done = force ´ distance., As, forces are same in case of proton and, positron but distance moved by positron is, larger, hence work done will be more in case, of positron., , 66. When the earth is closest to the sun, speed, of the earth is maximum, hence KE is, maximum. When the earth is farthest from, the sun, speed is minimum, hence KE is, minimum but never zero and negative., This variation is correctly represented by, option (d)., K, 67. F =, (given), v, dv K, m, =, dt, v, Þ, Þ, Þ, , ò mv dv = ò K dt, m, , v2, = Kt, 2, KE µ t, , 68. The kinetic energy of an air molecule is, =, , 10 -21 J, ~, - 0.0062 eV, 16, . ´ 10 -19 J/eV, , This is the same as 6.2 meV., , 69. As we know that, linear momentum, p, = 2mK, , æ, p2 ö, çQ K =, ÷, è, 2m ø
Page 130 :
CBSE New Pattern ~ Physics 11th (Term-I), , For same kinetic energy, p µ m, p1, m1, 1 1, =, =, = =1: 2, p2, m2, 4 2, , 70. Initial velocity = 10 ms -1, Final velocity = 20 ms -1, 1, Initial KE = ´ 10 ´ 10 ´ 10 = 5 ´ 10 2 J, 2, 1, Final KE = ´ 10 ´ 20 ´ 20 = 20 ´ 10 2 J, 2, ( 20 - 5) ´ 10 2, ´ 100 = 300%, % increase =, 5 ´ 10 2, , 71. Potential energy of a body increases when, work is done against a conservative force, e.g., if we raise the height of an object, its, potential energy increases because work is, done against gravitational force which is a, conservative force., , 72. The zero of the potential energy is arbitrary., It is set according to convenience. For the, spring force, we took U ( x ) = 0, at x = 0, i.e., the unstretched spring had zero potential, energy., For the constant gravitational force mg , we, took U = 0 on the earth’s surface., Also, for the force due to the universal law of, gravitation, the zero is best defined at an, infinite distance from the gravitational source., , 73. F = k 1x 1, F = k 2x 2, k 1 x2, =, k 2 x1, , k 1x 1 = k 2x 2 Þ, PE (1) k 1 x 12, =, PE (2) k 2 x 22, , 2, , =, , 3, k1 æk2 ö, k, ´ç ÷ = 2 =, k2 èk1 ø, k1 2, 1, 2, , 74. PE of spring = kx 2 Þ PE µ x 2, \, , PE = 15 ´, , 119, When x becomes 2x, the potential energy will, be, 1, 1, U ¢ = k ( 2x ) 2 = 4 ´ kx 2, 2, 2, = 4 ´ 10 = 40 J, \ Work done = U ¢ - U = 40 - 10 = 30 J, , 76. One of the greatest technical achievements of, human kind occurred when we discovered, how to ignite and control fire . We learnt to, rub two flint stones together (mechanical, energy), got them to heat up and to ignite a, heap of dry leaves (chemical energy), which, then provided sustained warmth., , 77. Parabolic plots of the potential energy U and, kinetic energy K of a block attached to a, spring obey in a Hooke’s law. The two plots, are complementary, one decreasing as the, other increases. The total mechanical energy, E = K + U remains constant., Energy, U, , –xm, , X, , KE depends only on initial and final point, and not on path covered, i.e. at B, KE = mgh ., , 79. We know that, PE + KE = Mechanical energy, U +K =E, Þ, U = E -K, Now, K can never be negative, so, U <E, K = E -U, Now, U can be negative, so K > E is possible., , 80. According to the law of conservation of, energy,, 1, 1 æ1, ö, mu 2 = ç mu 2 ÷ + mgh, ø, 2, 2è2, , 16 ~, ( 4), = 15 ´, - 27 J, 9, ( 3) 2, , through a distance x,, 1, U = kx 2 = 10 J, 2, , K, xm, , 78. In a conservative field loss of PE or gain of, , 2, , 75. Potential energy of the spring when stretched, , 0, , E=K+U, , Þ, , 490 = 245 + 5 ´ 9.8 ´ h, 245, h =, = 5m, 49
Page 131 :
120, , CBSE New Pattern ~ Physics 11th (Term-I), , 07, System of Particles, and Rotational Motion, Quick Revision, 1. Rigid Body A body is said to be a rigid body,, when it has a perfectly definite shape and size., e.g. A wheel can be considered as rigid body by, ignoring a little change in its shape., 2. Rotational Motion (Fixed Axis of, Rotation) In pure rotational motion, every, particle of the rigid body moves in circles of, different radii about a fixed line, which is, known as axis of rotation., e.g. A potter’s wheel, a merry-go-round, etc., 3. Centre of Mass A point at which the entire, mass of the body or system of bodies is, supposed to be concentrated is known as the, centre of mass., ● For a System of two Particles The centre, of mass of the system at a point which is at, distance x CM from origin is given by, , x CM is x-coordinates of centre of mass of, system is expressed as,, m x + m 2x 2 + m 3x 3 + × × × + m n x n, x CM = 1 1, m1 + m2 + m3 + × × × + mn, n, , Centre of mass, x CM =, ●, , x1, , m1, , d, , If particles are distributed in threedimensional space, then the centre of mass, has 3-coordinates, which are, 1 n, 1 n, S m i x i , y CM =, S mi yi, x CM =, =, 1, i, M, M i=1, 1 n, S m iz i, z CM =, M i=1, i = 1, , X, , x2, , Sm i, , n, , m2, , xCM, , i = 1, , where, M = m 1 + m 2 + m 3 + ... = S m i is the, , Y, C, , S mi x i, , ●, , total mass of the system. The index i runs, from 1 to n , m i is the mass of the i th, particle and the position of the ith particle is, given by ( x i , y i , z i )., Relation between position vectors of, particles and centre of mass,, n, , x CM =, ●, , m 1x 1 + m 2x 2, m1 + m2, , For a System of n-Particles Suppose a, system having masses m 1, m 2, m 3 , ..., m n, occupying x-coordinates x 1, x 2, x 3 , ..., x n ,, then, , R=, , S m i ri, , i = 1, , m, where, ri = ( x i i$ + y i $j + z i k$ ) is the position, vector of the ith particle and, R = ( x $i + y $j + z k$ ) is the position vector of, the centre of mass.
Page 132 :
CBSE New Pattern ~ Physics 11th (Term-I), , 4. Centre of Mass of Rigid Continuous, Bodies For a real body which is a continuous, distribution of matter, point masses are, differential mass elements dm and centre of, mass is given as, 1, 1, x CM =, x dm , y CM =, y dm, M ò, M ò, 1, and, z CM =, z dm, M ò, where, M is total mass of that real body., If we choose the origin of coordinates axes at, centre of mass, then, , ò x dm = ò ydm = ò zdm = 0, 5. Motion of Centre of Mass, ● Velocity about centre of mass,, n, , v CM =, , ●, , S mi v i, , i = 1, , M, dr, where, v =, , i.e. rate of change of position, dt, vector is velocity., Acceleration about centre of mass,, n, , a CM =, , S mi ai, , i = 1, , ,, M, But m i a i is the resultant force on the ith, particle, so, Ma CM = F1 + F2 + F3 + K + Fn, Ma CM = Fnet, 6. Linear Momentum of a System of a, Particle The total momentum of a system of, particles is equal to the product of the total, mass and velocity of its centre of mass., \ Total linear momentum, p = M v CM, 7. Moment of Force ( Torque) Torque is also, known as moment of force. We can define the, torque for a particle about a point as the vector, product of position vector of the point, where, the force acts and with the force itself. Let us, consider a particle P and force F acting on it., Torque,, t=r´F, The magnitude of torque | t | is, , 121, = Fr sin q, \, t = Fr^, Here, r^ is the perpendicular distance of the, line of action of F from the origin., 8. Angular Momentum of a Particle The, angular momentum of a particle of mass m, moving with velocity v (having a linear, momentum, p = m v ) about a point O is, defined by the following vector product,, L=r ´ p, or Angular momentum, L = m ( r ´ v ), Angular momentum will be zero ( L = 0 ) , if, p = 0 or r = 0 or q = 0° , 180 °, It is a vector quantity and its direction could be, found out with the help of cross-product., The SI unit of angular momentum is kg-m 2s –1., 9. Relation between Torque ( t ) and Angular, Momentum (L), dL, =t, dt, Above equation gives Newton’s second law of, motion in angular form, i.e. the rate of change, of angular momentum is equal to the torque, applied., 10. Couple A pair of equal and opposite forces, with parallel lines of action are known as a, couple. It produces rotation without, translation., 11. Principle of Moments When an object is in, rotational equilibrium, then algebraic sum of, all torques acting on it is zero. Clockwise, torques are taken as negative and, anti-clockwise torques are taken as positive., 12. Centre of Gravity If a body is supported on, a point such that total gravitational torque, about this point is zero, then this point is called, centre of gravity of the body., 13. Moment of Inertia For a rotating body, its, n, , moment of inertia is I = S m i ri2, i = 1, , or Moment of inertia, I = mR 2, The SI unit of moment of inertia is kg-m 2 and, its dimensional formula is [ML2 ] .
Page 133 :
122, , CBSE New Pattern ~ Physics 11th (Term-I), , moment of inertia is equal to the moment of, inertia of the body about the axis. It is given, I, as, K =, M, , 14. Relation between Angular Momentum, and Moment of Inertia, For a rigid body (about an fixed axis),, L = sum of angular momenta of all particles, = (m 1r12 + m 2r22 + m 3r32 + ¼ ¼ ) w, L =Iw, where, I = moment of inertia and w = angular, velocity of rigid body., 15. Radius of Gyration The radius of gyration of a, body about an axis may be defined as the, distance from the axis of a mass point whose, mass is equal to the mass of whole body and, , where, K is radius of gyration of the body., For rotating body, K =, , r12 + r22 + × × × + rn2, n, , Hence, radius of gyration of a rotating body, about a given axis is equal to root mean, square distance of constituent particles from, the given axis., , 16. Moment of Inertia in Some Standard Cases, Body, , Axis of Rotation, , Thin circular, , About an axis passing, through CG and, perpendicular to its, plane, , ring, radius, R, , Figure, , Moment of Inertia, , K 2 /R 2, , K, , MR 2, , R, , 1, , 1, 2, , c, , Thin circular, ring, radius R, , About its diameter, , 1, MR 2, 2, , R, 2, , Thin rod,, length L, , Perpendicular to rod, at mid-point, , 1, ML2, 12, , L, 12, , Circular disc,, radius R, , Perpendicular to, plane of disc at centre, , 1, MR 2, 2, , R, 2, , 1, 2, , Circular disc,, radius R, , About its diameter, , 1, MR 2, 4, , R, 2, , 1, 4, , Hollow, cylinder,, radius R, , About its own axis, , MR 2, , R, , 1, , R, L
Page 134 :
CBSE New Pattern ~ Physics 11th (Term-I), , 123, Figure, , Axis of Rotation, , Solid, cylinder,, radius R, , About its own axis, , MR 2, 2, , R, 2, , 1, 2, , Solid sphere,, radius R, , About its diametric, axis, , 2, MR 2, 5, , 2, R, 5, , 2, 5, , 17. From the given table below, we can compare, translational motion and rotational motion about a, fixed axis, i.e. Z-axis., Pure Translational, , Pure Rotational, , Linear position, x, , Angular position, q, , dx, Linear velocity, v =, dt, , Angular velocity, w =, , Linear acceleration, a =, , dv, dt, , Angular acceleration,, dw, a=, dt, , Mass, m, , Rotational inertia, I, , Newton’s second law,, F = ma, , Newton’s second law,, t = Ia, , Work done,W =, , ò F dx, , Kinetic energy, K =, , 1, mv 2, 2, , dq, dt, , Work done,W =, , ò t dq, , Kinetic energy, K =, , 1 2, Iw, 2, , Power, P = Fv, , Power, P = tw, , Linear momentum, p = mv, , Angular momentum, L = Iw, , Moment of Inertia, , K 2/, R2, , Body, , K, , 18. Rolling Motion The rolling motion, can be regarded as the combination of, pure rotation and pure translation. It is, also one of the most common motions, observed in daily life., 19. Kinetic Energy of a Rolling, Body The kinetic energy of a body, rolling without slipping is the sum of, kinetic energies of translational and, rotational motions., \ (KE )rolling = (KE)rotation + (KE )translation, 1, 1 2, = Iw2 + mv CM, 2, 2, K 2ù, 1 2 é, = mv CM ê1 + 2 ú, R û, 2, ë, (Qv CM = Rw and I = mK 2)
Page 135 :
124, , CBSE New Pattern ~ Physics 11th (Term-I), , Objective Questions, Multiple Choice Questions, , Hollow sphere, Air, , 1. A system of particles is called a rigid, body, when, , R/2, , (a) any two particles of system may have, displacements in opposite directions under, action of a force, (b) any two particles of system may have, velocities on opposite directions under, action of a force, (c) any two particles of system may have a zero, relative velocity, (d) any two particles of system may have, displacements in same direction under, action of a force, , 2. The centre of mass of a system of, , A, B, C, , R/2, D, Sand, , (a) A, , (b) B, , (c) C, , (d) D, , 6. Two bodies of masses 1 kg and 2 kg are, lying on x-y plane at (1, 2) and (- 1, 3), respectively. What are the coordinates, of centre of mass?, , particles does not depend on, , (a) (2, - 1), , (a) masses of the particles, (b) internal forces of the particles, (c) position of the particles, (d) relative distance between two particles, , æ8 1 ö, (b) ç , - ÷, è 3 3ø, , æ 1 8ö, (c) ç - , ÷, è 3 3ø, , (d) None of these, , 3. In pure rotation, all particles of the, body, (a), (b), (c), (d), , move in a straight line, move in concentric circles, move in non-concentric circles, have same speed, , 4. For n particles in a space, the suitable, expression for the x-coordinate of the, centre of mass of a system is, Smi xi, (a), mi, Smi y i, (c), M, , Smi xi, (b), M, Smi zi, (d), M, , 7. Three identical spheres of mass M each, are placed at the corners of an, equilateral triangle of side 2m. Taking, one of the corner as the origin, the, position vector of the centre of mass is, (a), (c), , $i, + $j, 3, $j, (d) $i +, 3, , 3 ($i - $j ), , (b), , $i + $j, 3, , 8. Centre of mass of the given system of, particles will be at, A, , m, , 2m, B, O, , 5. Which of the following points is the, likely position of the centre of mass of, the system shown in figure?, , D, , m, , m, , C, , (NCERT Exemplar), , (a) OD, , (b) OC, , (c) OB, , (d) AO
Page 136 :
CBSE New Pattern ~ Physics 11th (Term-I), , 9. Two particles of equal masses have, , velocities v 1 = 4 $i ms -1 and, v 2 = 4 $j ms -1 . First particle has an, acceleration a 1 = ( 2$i + 2$j) ms -2 , while, the acceleration of the other particle is, zero. The centre of mass of the two, particles moves in a path of, (a), (b), (c), (d), , 10. The centre of mass of three particles of, masses 1 kg, 2 kg and 3 kg is at (3, 3, 3), with reference to a fixed coordinate, system. Where should a fourth particle, of mass 4 kg be placed, so that the, centre of mass of the system of all, particles shifts to a point (1, 1, 1)?, (a) (- 1, - 1, - 1), (c) (2, 2, 2), , (b) (- 2, - 2, - 2), (d) (1, 1, 1), , 11. A ball kept in a closed box moves in, the box making collisions with the, walls. The box is kept on a smooth, surface. The velocity of the centre of, mass, (a) of the box remains constant, (b) of the box and the ball system remains, constant, (c) of the ball remains constant, (d) of the ball relative to the box remains, constant, , 12. A force F is applied on a single particle, P as shown in the figure. Here, r is the, position vector of the particle., The value of torque t is, Z, τ, , F, r, , P, , θ, , O, , particle whose position vector is, r = $i - 2$j + k$ . What is the torque about, the origin ?, (a) 8$i + 10$j + 12 k$, (c) 8$i - 10$j - 8k$, , (b) 8$i + 10$j - 12 k$, (d) 10$i - 10$j - k$, , Y, , (c) r × F, , its centre. F1 , F2 and F3 represent three, forces acting along the sides AB , BC, and AC, respectively. If the total torque, about O is zero, then the magnitude of, F3 is, A, F3, O, B, , C, , F2, , F1, , (a) F1 + F2, F + F2, (c) 1, 2, , (b) F1 - F2, (d) 2 (F1 + F2 ), , 15. The angular momentum L of a single, particle can be represented as, (a) r ´ p, (c) rp^ n$, , (b) rp sin q n$, (d) Both (a) and (b), , ( n$ = unit vector perpendicular to plane, of r, so that r, p and n$ make right, handed system), , 16. Newton’s second law for rotational, motion of a system of particle can be, represented as (L for a system of, particles), dp, = t ext, dt, dL, (c), = t ext, dt, (a), , dL, = t int, dt, dL, (d), = t int + t ext, dt, (b), , 17. A particle of mass m moves in the, , X, , (b) r ´ F, , 13. A force F = 5$i + 2$j - 5k$ acts on a, , 14. ABC is an equilateral triangle with O as, , straight line, parabola, circle, ellipse, , (a) F ´ r, , 125, , (d) F× r, , xy-plane with a velocity v along the, straight line AB. If the angular, momentum of the particle with respect, to origin O is L A , when it is at A and L B, when it is at B, then
Page 137 :
126, , CBSE New Pattern ~ Physics 11th (Term-I), , (a), (b), (c), (d), , Y, B, A, X, , O, , (a) LA > LB, (b) LA = LB, (c) the relationship between LA and LB depends, upon the slope of the line AB, (d) LA < LB, , 18. A point mass m is attached to a massless, string whose other end is fixed at P as, shown in figure. The mass is, undergoing circular motion in xy -plane, with centre O and constant angular, speed w. If the angular momentum of, the system, calculated about O and P be, LO and LP respectively, then, , angular momentum, linear momentum, angular acceleration, centripetal acceleration, , 21. A particle of mass m is moving in, yz-plane with a uniform velocity v with, its trajectory running parallel to +ve, y-axis and intersecting z-axis at z = a in, figure. The change in its angular, momentum about the origin as it, bounces elastically from a wall at, y = constant is, (NCERT Exemplar), z, a, , v, , y, , z, P, , O, , (a) mva e$ x, (c) ymv e$ x, m, ω, , (a) L O and L P do not vary with time, (b) L O varies with time while L P remains, constant, (c) L O remains constant while L P varies with, time, (d) L O and L P both vary with time, , (b) 2 mva e$ x, (d) 2 ymv e$ x, , 22. The variation of angular position q of a, point on a rotating rigid body with time, t is shown in figure., θ, t1 t2, O, , t3, , t, , 19. A child stands at the centre of a, turntable with his two arms, outstretched. The turntable is set, rotating with an angular speed of, 40 rev min -1 . How much is the angular, speed of the child, if he folds his hands, back and thereby reduces his moment, of inertia to (2/5) times the initial value?, Assume that the turntable rotates, without friction., (NCERT Exemplar), (a) 40 rpm (b) 45 rpm (c) 55 rpm (d) 100 rpm, , 20. If the torque of the rotational motion, will be zero, then the constant quantity, will be, , In which direction, the body is rotating?, (NCERT Exemplar), , (a), (b), (c), (d), , Clockwise, Anti-clockwise, May be clockwise or anti-clockwise, None of the above, , 23. A rigid body is said to be in partial, equilibrium only, if, (a), (b), (c), (d), , it is in rotational equilibrium, it is in translational equilibrium, Either (a) or (b), None of the above
Page 138 :
CBSE New Pattern ~ Physics 11th (Term-I), , 24. In the game of see-saw, what should be, , 127, The radius of gyration for the rod is, , the displacement of boy B from right, edge to keep the see-saw in equilibrium?, (Given, M 1 = 40 kg and M 2 = 60 kg), A, , O, , M, , L, , B, M1, , M2, , (a) L/12, , (b) L/ 12, , (c) L/ 6, , (d) L/ 6, , 29. A wheel is rotating at 900 rpm about its, 2m, , 4, m, 3, 2, (c) m, 3, (a), , 2m, , (b) 1m, (d) Zero, , 25. The centre of gravity of a homogeneous, body is the point at which the whole, (a) volume of the body is assumed to be, concentrated, (b) area of the surface of the body is assumed, to be concentrated, (c) weight of the body is assumed to be, concentrated, (d) All of the above, , 26. One solid sphere A and another hollow, sphere B are of same mass and same, outer radius. Their moments of inertia, about their diameters are I A and I B, respectively, such that, (a) I A = I B, (c) I A < I B, , (b) I A > I B, (d) None of these, , 27. A disc of mass M and radius R is, rotating about one of its diameter. The, value of radius of gyration for the disc, is, , axis. When the power is cut-off, it, comes to rest in 1 min. The angular, retardation (in rad s -2 ) is, (a) -, , p, 2, , (b), , p, 4, , (c), , p, 6, , (d), , p, 2, , 30. If object starts from rest and covers, angle of 60 rad in 10 s in circular, motion, then magnitude of angular, acceleration will be, (a) 1.2 rad s -2, (c) 2 rad s -2, , (b) 1.5 rad s -2, (d) 2.5 rad s -2, , 31. When a ceiling fan is switched OFF, its, angular velocity fall to half while it, makes 36 rotations. How many more, rotations will it make before coming to, rest? (Assume uniform angular, retardation), (a) 36, , (b) 24, , (c) 18, , (d) 12, , 32. A disc is rotating with angular velocity, , w. A force F acts at a point whose, position vector with respect to the axis, of rotation is r. The power associated, with torque due to the force is given by, (a) (r ´ F) ×w, (c) r ´ (F× w), , (b) (r ´ F) ´ w, (d) r × (F ´ w), , 33. A flywheel of moment of inertia, (a) R /4, (c) R / 6, , (b) R /2, (d) None of these, , 28. A rod is rotating about an axis passing, through its centre and perpendicular to, its length., , 0.4 kg-m 2 and radius 0.2 m is free to, rotate about a central axis. If a string is, wrapped around it and it is pulled with, a force of 10N, then its angular velocity, after 4 s will be, (a) 10 rads -1, , (b) 5 rads -1, , (c) 20 rads -1, , (d) None of these
Page 139 :
128, , CBSE New Pattern ~ Physics 11th (Term-I), , 34. Two discs having mass ratio (1/2) and, diameter ratio (2/1), then find ratio of, moment of inertia., (a) 2 : 1, , (b) 1 : 1, , (c) 1 : 2, , 40. If frictional force is neglected and girl, bends her hand, then (initially girl is, rotating on chair), , (d) 2 : 3, , 35. A solid sphere is rotating freely about, its symmetry axis in free space. The, radius of the sphere is increased, keeping its mass same. Which of the, following physical quantities would, remain constant for the sphere?, (a), (b), (c), (d), , Rotational kinetic energy, Moment of inertia, Angular velocity, Angular momentum, , 36. A body having a moment of inertia, about its axis of rotation equal to, 3 kg-m 2 is rotating with angular, velocity of 3 rad s –1 . Kinetic energy of, this rotating body is same as that of a, body of mass 27 kg moving with a, velocity v. The value of v is, (a) 1 ms–1, , (b) 0.5 ms–1 (c) 2 ms–1 (d) 1 .5 ms–1, , 37. A disc of radius R is rotating with an, , angular speed w 0 about a horizontal, axis. It is placed on a horizontal table., The coefficient of kinetic friction is m k ., What was the velocity of its centre of, mass before being brought in contact, with the table?, (NCERT Exemplar), (a) w0 R, , (b) Zero, , (c), , w0 R, 2, , (d) 2 w0 R, , 38. Two bodies have their moments of, inertia I and 2I respectively about their, axis of rotation. If their kinetic energies, of rotation are equal, their angular, momenta will be in the ratio, (a) 1 : 2, , (b) 2 :1, , (c) 2 :1, , (d) 1 : 2, , 39. By keeping moment of inertia of a body, constant, if we double the time period,, then angular momentum of body, (a) remains constant, (c) doubles, , (b) becomes half, (d) quadruples, , (a), (b), (c), (d), , I girl will reduce, I girl will increase, wgirl will reduce, None of the above, , 41. A merry-go-round, made of a ring-like, platform of radius R and mass M, is, revolving with angular speed w. A, person of mass M is standing on it. At, one instant, the person jumps off the, round, radially away from the centre of, the round (as seen from the round). The, speed of the round of afterwards is, (NCERT Exemplar), , (a) 2 w, w, (c), 2, , (b) w, (d) zero, , 42. A wheel of radius R rolls on the ground, with a uniform velocity v. The velocity, of topmost point relative to the, bottommost point is, (a) v, (c) v /2, , (b) 2v, (d) zero, , 43. A hoop of radius 2 m weighs, 100 kg. It rolls along a horizontal floor,, so that its centre of mass has a speed of, 20 cms -1 . How much work has to be, done to stop it?, (NCERT Exemplar), (a) 10 J, (c) 4 J, , (b) 12 J, (d) 3 J
Page 140 :
CBSE New Pattern ~ Physics 11th (Term-I), , 44. A drum of radius R and mass M rolls, down without slipping along an, inclined plane of angle q. The frictional, force, (a) converts translational energy into rotational, energy, (b) dissipates energy as heat, (c) decreases the rotational motion, (d) decreases the rotational and translational, motion, , 45. The centre of mass lie outside the body, of a ……… ., , (NCERT Exemplar), , (a) pencil, (c) dice, , (b) shotput, (d) bangle, , 129, (a) The sense of rotation remains same., (b) The orientation of the axis of rotation, remains same., (c) The speed of rotation is non-zero and, remains same., (d) The angular acceleration is non-zero and, remains same., , 50. A bicycle wheel rolls without slipping, on a horizontal floor. Which one of the, following statements is true about the, motion of points on the rim of the, wheel, relative to the axis at the wheel’s, centre?, , 46. Figure shows a composite system of two, uniform rods of lengths as indicated., Then the coordinates of the centre of, mass of the system of rods are……… ., y, 2L, O, , L, , æ L 2L ö, (a) ç , ÷, è2 3 ø, æ L 2L ö, (c) ç , ÷, è6 3 ø, , x, , æ L 2L ö, (b) ç , ÷, è4 3 ø, æ L Lö, (d) ç , ÷, è 6 3ø, , 47. Analogue of mass in rotational motion, is ……… ., (a), (b), (c), (d), , 51. If radius of earth is reduced to half, without changing its mass, then match, the following columns and choose the, correct option from the codes given, below., Column I, , moment of inertia, angular momentum, gyration, None of the above, , 48. The angular acceleration of a flywheel, of mass 5 kg and radius of gyration, 0.5 m is ………, if a torque of 10N-m is, applied on it., (a) 2 rad s- 2, (c) 8 rad s- 2, , (a) Points near the top move faster than points, near the bottom., (b) Points near the bottom move faster than, points near the top., (c) All points on the rim move with the same, speed., (d) All points have the velocity vectors that are, pointing in the radial direction towards the, centre of the wheel., , (b) 4 rad s- 2, (d) zero, , 49. When a disc rotates with uniform, angular velocity, which of the following, statemnts is incorrect. (NCERT Exemplar), , Column II, , A., , Angular, momentum of, earth, , p., , Will, become one, fourth, , B., , Time period of, rotation of, earth, , q., , Will, become, four times, , C., , Rotational, kinetic energy, of earth, , r., , No change, , Codes, A, , B, , C, , A, , B, , C, , (a) p, , q, , r, , (b) p, , q, , p, , (c) r, , p, , q, , (d) p, , r, , p
Page 141 :
130, , CBSE New Pattern ~ Physics 11th (Term-I), , 52. A rigid body is rolling without slipping, on the horizontal surface, then match, the Column I with Column II and, choose the correct option from the, codes given below., C, , 53. Assertion The motion of the centre of, mass describes the translational part of, the motion., Reason Translational motion always, means straight line motion., , 54. Assertion The centre of mass of a, body must lie on the body., B, , v, , 60°, , Reason The centre of mass of a body, does not lie at the geometric centre of, body., , ω, D, , A, , 55. Assertion Two identical spherical, Column I, , Column II, , A., , Velocity at, point A, i.e. v A, , p., , v 2, , B., , Velocity at, point B, i.e. v B, , q., , zero, , C., , Velocity at, point C , i.e. vC, , r., , v, , D., , Velocity at, point D, i.e. vD, , s., , 2v, , (a), , Codes, A, , B, , C, , D, , (a) q, , p, , s, , r, , (b) p, , r, , s, , q, , (c) s, , r, , q, , p, , (d) q, , r, , s, , p, , spheres are half filled with two liquids, of densities r1 and r 2 ( > r1 ). The centre, of mass of both the spheres lie at same, level., , Assertion-Reasoning MCQs, For question numbers 53 to 64, two, statements are given-one labelled, Assertion (A) and the other labelled, Reason (R). Select the correct answer to, these questions from the codes (a), (b), (c), and (d) are as given below, (a) Both A and R are true and R is the, correct explanation of A., (b) Both A and R are true but R is not the, correct explanation of A., (c) A is true but R is false., (d) A is false and R is also false., , (b), , Reason The centre of mass does not lie, at centre of the sphere., , 56. Assertion If a particle moves with a, constant velocity, then angular, momentum of this particle about any, point remains constant., Reason Angular momentum does not, have the units of Planck’s constant., , 57. Assertion When a particle is moving, in a straight line with a uniform, velocity, its angular momentum is, constant., Reason The angular momentum is, non-zero, when particle moves with a, uniform velocity., , 58. Assertion For a system of particles, under central force field, the total, angular momentum is conserved., Reason The torque acting on such a, system is zero.
Page 142 :
CBSE New Pattern ~ Physics 11th (Term-I), , 59. Assertion Inertia and moment of, inertia are not same quantities., Reason Inertia represents the capacity, of a body that does not oppose its state, of motion or rest., , 60. Assertion Moment of inertia of a, particle is different whatever be the axis, of rotation., Reason Moment of inertia does not, depends on mass and distance of the, particle from the axis of rotation., , 61. Assertion The angular velocity of a, rigid body in motion is defined for the, whole body., Reason All points on a rigid body, performing pure rotational motion are, having same angular velocity., , 62. Assertion If bodies slide down an, inclined plane without rolling, then all, bodies reach the bottom simultaneously, is not necessary., Reason Acceleration of all bodies are, equal and independent of the shape., , 63. Assertion A solid sphere cannot roll, without slipping on smooth horizontal, surface., Reason If the sphere is left free on, smooth inclined surface, it can roll, without slipping., , 64. Assertion The work done against force, of friction in the case of a disc rolling, without slipping down an inclined, plane is zero., Reason When the disc rolls without, slipping, friction is required because for, rolling condition velocity of point of, contact is zero., , 131, , Case Based MCQs, Direction Answer the questions from, 65-69 on the following case., Centre of Mass, The centre of mass of a body or a system of, bodies is the point which moves as though all, of the mass were concentrated there and all, external forces were applied to it. Hence, a, point at which the entire mass of the body or, system of bodies is supposed to be, concentrated is known as the centre of mass., If a system consists of more than one particles, (or bodies) and net external force on the, system in a particular direction is zero with, centre of mass at rest. Then, the centre of, mass will not move along that direction. Even, though some particles of the system may, move along that direction., , 65. The centre of mass of a system of two, particles divides, the distance between, them, (a) in inverse ratio of square of masses of, particles, (b) in direct ratio of square of masses of, particles, (c) in inverse ratio of masses of particles, (d) in direct ratio of masses of particles, , 66. Two bodies of masses 1 kg and 2 kg are, lying in xy-plane at ( -1, 2) and ( 2, 4 ),, respectively. What are the coordinates, of the centre of mass?, æ 10 ö, (a) ç1, ÷, è 3ø, , (b) (1,0), , (c) (0, 1), , (d) None of these, , 67. Two balls of same masses start moving, towards each other due to gravitational, attraction, if the initial distance between, them is l. Then, they meet at
Page 143 :
132, , CBSE New Pattern ~ Physics 11th (Term-I), , M, , 70. If the F net, ext is zero on the cardboard, it, , M, F, , F, , means, , l, , l, (a), 2, , l, (c), 3, , (b) l, , l, (d), 4, , 68. All the particles of a body are situated, at a distance R from the origin. The, distance of centre of mass of the body, from the origin is, (a) = R, , (b) £ R, , (c) > R, , (d) ³ R, , 69. Two particles A and B initially at rest, move towards each other under a, mutual force of attraction. At the, instant, when the speed of A is v and the, speed of B is 2v, the speed of centre of, mass of the system is, (a) zero, (c) 15, .v, , (b) v, (d) 3v, , Direction Answer the questions from, 70-74 on the following case., Torque and Centre of Gravity, Torque is also known as moment of force or, couple. When a force acts on a particle, the, particle does not merely move in the, direction of the force but it also turns about, some point. So, we can define the torque for a, particle about a point as the vector product of, position vector of the point where the force, acts and with the force itself. In the given, figure, balancing of a cardboard on the tip of, a pencil is done. The point of support, G is, the centre of gravity., R, G, m1g, m2g, Mg, , (a) R = Mg, (c) m2 g = Mg, , (b) m1 g = Mg, (d) R = m1 / g, , 71. Choose the correct option., (a), (b), (c), (d), , t Mg about CG = 0, t R about CG = 0, Net t due to m1 g, m2 g ,...., mn g about CG = 0, All of the above, , 72. The centre of gravity and the centre of, mass of a body coincide, when, (a), (b), (c), (d), , g is negligible, g is variable, g is constant, g is zero, , 73. If value of g varies, the centre of gravity, and the centre of mass will, (a), (b), (c), (d), , coincide, not coincide, become same physical quantities, None of the above, , 74. A body lying in a gravitational field is, in stable equilibrium, if, (a), (b), (c), (d), , vertical line through CG passes from top, horizontal line through CG passes from top, vertical line through CG passes from base, horizontal line through CG passes from, base, , Direction Answer the questions from, 75-79 on the following case., Moment of Inertia, A heavy wheel called flywheel is attached to, the shaft of steam engine, automobile engine, etc., because of its large moment of inertia,, the flywheel opposes the sudden increase or, decrease of the speed of the vehicle. It allows, a gradual change in the speed and prevents, jerky motion and hence ensure smooth ride of, passengers.
Page 144 :
CBSE New Pattern ~ Physics 11th (Term-I), , 75. Moment of inertia of a body depends, upon, (a) axis of rotation, , (b) torque, , (c) angular momentum (d) angular velocity, , 76. A particle of mass 1 kg is kept at (1m,, 1m, 1m). The moment of inertia of this, particle about Z -axis would be, (a), (b), (c), (d), , 133, Direction Answer the questions from, 80-84 on the following case., Rolling Motion, The rolling motion can be regarded as the, combination of pure rotation and pure, translation. It is also one of the most common, motions observed in daily life., P1, , 1 kg-m2, 2 kg-m2, 3 kg-m2, None of the above, , vr, P2, , 77. Moment of inertia of a rod of mass m, , 3, I, 4, 27, (c), I, 64, , 9, I, 16, I, (d), 16, , (b), , 78. A circular disc is to be made by using, iron and aluminium, so that it acquires, maximum moment of inertia about its, geometrical axis. It is possible with, (a) iron and aluminium layers in alternate order, (b) aluminium at interior and iron surrounding, it, (c) iron at interior and aluminium surrounding, it, (d) Either (a) or (c), , 79. Three thin rods each of length L and, mass M are placed along X , Y and, Z -axes such that one end of each rod is, at origin. The moment of inertia of this, system about Z -axis is, 2 2, ML, 3, 4ML2, (b), 3, 5ML2, (c), 3, ML2, (d), 3, , vCM, vCM, , C, , and length l about its one end is I. If, one-fourth of its length is cut away, then, moment of inertia of the remaining rod, about its one end will be, (a), , v1, , v2, , R, , ω, , P0, , Suppose the rolling motion (without slipping), of a circular disc on a level surface. At any, instant, the point of contact P 0 of the disc with, the surface is at rest (as there is no slipping). If, v CM is the velocity of centre of mass which is, the geometric centre C of the disc, then the, translational velocity of disc is v CM , which is, parallel to the level surface., Velocity of centre of mass, v CM = Rw, , 80. A solid cylinder is sliding on a smooth, horizontal surface with velocity v 0, without rotation. It enters on the rough, surface. After that it has travelled some, distance, the friction force increases its, (a), (b), (c), (d), , (a), , translational kinetic energy, rotational kinetic energy, total mechanical energy, angular momentum about an axis passing, through point of contact of the cylinder and, the surface, , 81. A cylinder rolls down an inclined plane, of inclination 30°, the acceleration of, cylinder is, (a), , g, 3, , (b) g, , (c), , g, 2, , (d), , 2g, 3
Page 145 :
134, , CBSE New Pattern ~ Physics 11th (Term-I), , 82. Sphere is in pure accelerated rolling, , 83. Kinetic energy of a rolling body will be, , motion in the figure shown,, , 1, 2, mv CM, (1 + k 2 / R 2 ), 2, 1, (b) I w2, 2, 1, 2, (c) mv CM, 2, (d) None of the above, (a), , v, , ω, , s, mg, , in, , θ, , θ, , 84. A body is rolling down an inclined, , Choose the correct option., , plane. Its translational and rotational, kinetic energies are equal. The body, is a, , (a) The direction of fs is upwards, (b) The direction of fs is downwards, (c) The direction of gravitational force is, upwards, (d) The direction of normal reaction is, downwards, , (a), (b), (c), (d), , solid sphere, hollow sphere, solid cylinder, hollow cylinder, , ANSWERS, Multiple Choice Questions, 1. (c), 11. (b), 21. (b), , 2. (b), 12. (b), 22. (b), , 3. (b), 13. (a), 23. (c), , 4. (b), 14. (a), 24. (c), , 5. (c), 15. (d), 25. (c), , 6. (c), 16. (c), 26. (c), , 7. (d), 17. (b), 27. (b), , 8. (c), 18. (c), 28. (b), , 9. (a), 19. (d), 29. (a), , 10. (b), 20. (a), 30. (a), , 31. (d), 41. (a), 51. (c), , 32. (a), 42. (b), 52. (a), , 33. (c), 43. (c), , 34. (a), 44. (a), , 35. (d), 45. (d), , 36. (a), 46. (c), , 37. (b), 47. (a), , 38. (d), 48. (c), , 39. (b), 49. (d), , 40. (a), 50. (a), , 55. (c), , 56. (c), , 57. (b), , 58. (a), , 59. (c), , 60. (c), , 61. (b), , 62. (c), , 67. (a), 77. (c), , 68. (b), 78. (b), , 69. (a), 79. (a), , 70. (a), 80. (b), , 71. (d), 81. (a), , 72. (c), 82. (a), , 73. (b), 83. (a), , 74. (c), 84. (d), , Assertion-Reasoning MCQs, 53. (c), 63. (d), , 54. (d), 64. (a), , Case Based MCQs, 65. (c), 75. (a), , 66. (a), 76. (b)
Page 146 :
CBSE New Pattern ~ Physics 11th (Term-I), , 135, , SOLUTIONS, 1. A rigid body does not deform under action of, applied force and there is no relative motion, of any two particles constituting that rigid, body. So, it means that a system of particles, is called a rigid body, when any two particles, of system has a zero relative velocity., 2. A point at which the entire mass of the body, or system of bodies. This is supposed to be, concentrated is known as centre of mass., It does not depend on the internal forces, acting on the particle., , 3. In pure rotational motion, all the particles of, body moves in concentric circles without, doing any translational motion., 4. For system of n-particles in space, the centre, of mass of such a system is at ( x , y, z ), where, Sm i x i, Sm i y i, ,Y =, X =, M, M, Sm i z i, and Z =, M, Here, M = Sm i is the total mass of the, system. The index i runs from 1 to n. m i is, the mass of ith particle and position of ith, particle is ( x i , y i , z i )., , 5. Centre of mass of a system lies towards the, part of the system, having bigger mass. In the, above diagram, lower part is heavier, hence, CM of the system lies below the horizontal, diameter., Hence, option (c) is correct., , 6. Let the coordinates of the centre of mass be, ( x , y ) which are calculated as,, m x + m 2 x 2 1 ´ 1 + 2 ´ ( - 1), x= 1 1, =, 3, m1 + m2, 1-2, 1, =3, 3, m 1y 1 + m 2y 2 1 ´ 2 + 2 ´ 3, y=, =, 3, m1 + m2, =, , 2+6 8, =, 3, 3, Therefore, the coordinates of centre of mass, æ 1 8ö, be ç - , ÷ ., è 3 3ø, =, , 7. The given system of spheres is as shown, below, M (1m, 3m), , M, (2m, 0), , M, (0, 0), , The x and y -coordinates of centre of mass is, Sm i xi M ´ 0 + M ´ 1 + M ´ 2, x=, =, =1, Sm i, M+M +M, S m i yi M ´ 0 + M ( 3) + M ´ 0, =, M +M +M, Sm i, 3M, 1, y=, =, 3M, 3, , y=, Þ, , So, position vector of the centre of mass is, $j ö, æ$, çi +, ÷., 3ø, è, , 8. If all the masses were same, the centre of, mass was at O. But as the mass at B is 2m, so, the centre of mass of the system will shift, towards B. So, centre of mass will be on the, line OB., 9. Given, v1 = 4 $i ms -1, v2 = 4 $j ms -1, a = ( 2i$ + 2$j) ms -2, a = 0 ms -2, 1, , 2, , \Velocity of centre of mass,, m v + m 2 v2 ( v1 + v2 )m, vCM = 1 1, =, 2m, m1 + m2, [Qm 1 = m 2 = m ], $, $, 4i + 4 j, = 2( i$ + $j) ms -1, =, 2, Similarly, acceleration of centre of mass,, a + a2, a CM = 1, 2, $, 2i + 2$j + 0, =, = ( i$ + $j) ms -2, 2, Since, from above values, it can be seen that, v CM is parallel to a CM , so the path will be a, straight line.
Page 147 :
136, , CBSE New Pattern ~ Physics 11th (Term-I), , 10. Centre of mass of a system of particles is, given by, 1 ´ x1 + 2 ´ x2 + 3 ´ x3, x CM =, =3, 1+ 2+ 3, [Q x CM = y CM = z CM = 3], …(i), Þ x 1 + 2x 2 + 3x 3 = (1 + 2 + 3) 3 = 18, When fourth particle is placed, then, (given), x CM = y CM = z CM = 1, 1 ´ x1 + 2 ´ x2 + 3 ´ x3 + 4 ´ x4, Þ x CM =, (1 + 2 + 3 + 4 ), Þ, x 1 + 2 x 2 + 3 x 3 + 4 x 4 = 1 (1 + 2 + 3 + 4 ) = 10, …(ii), On solving Eqs. (i) and (ii), we get, 4 x 4 = 10 - 18 Þ x 4 = - 2, Similarly,, y 4 = - 2, z 4 = - 2, \ The fourth particle must be placed at the, point ( -2 , - 2 , - 2) ., 11. Net external force on the system is zero., Hence, velocity of centre of mass of the box, and ball system will remain constant., , 12. If a force acts on a single particle at a point P, whose position with respect to origin O is, given by the position vector r as shown in, given figure, the moment of the force acting, on the particle with respect to the origin O is, defined as the vector product., t=r´F, Þ, , | t | = r F sin q, , 13. Given, F = 5i$ + 2$j - 5k$ and r = $i - 2$j + k$, We know that, t = r ´ F, So, torque about the origin will be given by, $j, i$, k$, , 15. Angular momentum (L) can be defined as, moment of linear momentum about a point., It is given by,, L=r´p, $, L can also be represented as, L = rp sin qn., , 16. According to Newton’s second law of, rotational motion, the rate of the total, angular momentum of a system of particles, about a point is equal to the sum of the, external torques acting on the system taken, about the same point., dL, i.e. t ext =, dt, , 17. From the definition of angular momentum,, L = r ´ p = rmv sin f ( - k$ ), Y, φ, , B, , A, P, d, , r, X, , O, , Therefore, the magnitude of L is, L = mvr , sin f = mvd , where d = r sin f is the, distance of closest approach of the particle to, the origin. As d is same for both the particles,, hence L A = L B ., , 18. Angular momentum of a particle about a, point is given by, L = r ´ p = m ( r ´ v), For LO ,, LO, , = 1 -2 1, 5 +2 -5, , v, , = $i (10 - 2) - $j ( - 5 - 5) + k$ ( 2 + 10 ), = 8 $i + 10 $j + 12k$, , 14. If we take clockwise torque, then magnitude, of total torque is, t net = t F 1 + t F 2 + t F 3, 0 = - F 1r - F 2r + F 3r, Þ, F3 = F1 + F2, , O, ω, , 90°, R, , r, , | L | = (mvr sin q) = m ( Rw)( R ) sin 90° = mR 2 w, = constant, Direction of LO is always upwards, therefore, LO is constant, both in magnitude as well as, direction.
Page 148 :
CBSE New Pattern ~ Physics 11th (Term-I), , For LP , | LP | = (mvr sin q), = (m ) ( Rw) ( l ) sin 90° = mRlw, P, , LP, , is the point at which the whole weight of the, body is assumed to be concentrated., v, , R, , 26. Let mass and outer radii of solid sphere and, r, , Magnitude of LP will remains constant but, direction of LP keeps on changing, i.e. it, varies with time., , 19. From law of conservation of angular, momentum,, L1 = L2, I 1 w1 = I 2 w2 Þ w2 =, , I 1 w1, I2, , I 1 ´ 40 200, =, = 100 rpm, 2, 2, I1, 5, dL, 20. As, torque, t =, dt, If t = 0, then L = constant., Þ, , So, 60 kg boy has to be displaced to, 4 2, =2- = m, 3 3, , 25. The centre of gravity of a homogeneous body, I, , Þ, , 137, , hollow sphere be M and R, respectively. The, moment of inertia of solid sphere A about its, diameter,, 2, …(i), I A = MR 2, 5, The moment of inertia of hollow sphere, (spherical shell) B about its diameter,, 2, …(ii), I B = MR 2, 3, It is clear from Eqs. (i) and (ii), that, , w2 =, , Hence, option (a) is correct., 21. The initial velocity is v i = v e$ y and after, reflection from the wall, the final velocity is, v f = - v e$ y . The trajectory is described as, position vector r = ye$ y + ae$ z ., Hence, the change in angular momentum is, r ´ m ( v f - v j ) = 2mvae$ x ., , 22. As the slope of q-t graph is positive and, positive slope indicates anti-clockwise, rotation., 23. A body may remain in partial equilibrium, means that body may remain only in, translational equilibrium or only in rotational, equilibrium., , 24. Let x be the distance from centre, then, for rotational equilibrium,, M 1 g ´ rA = M 2 g ´ x, M1g 2m, , x, , ( 40 ´ 10 ) ´ 2 = ( 60 ´ 10 ) x, 8 4, Þ, x= = m, 6 3, , M2g, , IA < IB, , 27. I disc about the axis along its diameter, MR 2, 4, Using radius of gyration, I = Mk 2, =, , …(i), , …(ii), R, Comparing Eqs. (i) and (ii), we get k = ., 2, , 28. As, moment of inertia of rod,, ML2, 12, Using radius of gyration, I = Mk 2, Comparing Eqs. (i) and (ii), we get, I rod =, , Radius of gyration, k = L / 12, , 29. Angular retardation,, a=, , w f - wi, , 0 - 900 ´, , 2p, 60 rad s- 2, , =, Dt, 60, 900 ´ 2 ´ p, p, == - rad s-2, 3600, 2, , 30. Given, initial angular velocity of object,, w0 = 0, Angular displacement, q = 60 rad, and Dt = 10 s, From equation of rotational motion,, 1, q = w0 t + at 2, 2, , …(i), …(ii)
Page 149 :
138, , CBSE New Pattern ~ Physics 11th (Term-I), , 60 = 0 ´ t +, , 1, ´ a ´ 10 2, 2, , 35. As, we know that external torque, t ext =, , 60, 50, , Þ, , a=, , Þ, , a = 1.2 rads -2, , 31. Total angular displacement in 36 rotation,, q = 36 ´ 2p, Using w22 - w21 = 2aq, we get, ( w/ 2) 2 - w2 = 2a(36 ´ 2p), Similarly, 0 2 - ( w/ 2) 2 = 2a (n ´ 2p), Dividing Eq. (i) by Eq. (ii), we get, 3, - w2, 36, 4, =, Þn = 12, - w2 / 4 n, , …(i), …(ii), , Hence, ceiling fan will make 12 more, rotations before coming to rest., , 32. Power, P = tw, P = (r ´ F )× w, , 33. Given, moment of inertia of flywheel,, I = 0.4 kg-m 2, Radius, r = 0.2 m, Force, F = 10 N, ( w2 - w1 ), Q F ´ r = Ia = I, t, F ´r ´t, Þ, w2 - w1 =, I, (from t = F ´ r and t = Ia), 10 ´ 0.2 ´ 4, =, 0.4, = 20 rads -1, , 34. Given, mass ratio of two discs,, m 1 : m 2 = 1 : 2 , i.e., , m1 1, =, m2 2, , and diameter ratio,, Þ, , d1 2, =, d2 1, , r1 d 1 / 2 d 1 2, =, =, =, r2 d 2 / 2 d 2 1, , \ Ratio of their moment of inertia,, m 1r12, 2, 2, I1, 1 æ 2ö, 2, m ær ö, = 2 2 = 1 ×ç 1÷ = ç ÷ =, I 2 m 2r2 m 2 è r2 ø, 2 è1ø, 1, 2, \, I 1 : I 2 = 2 :1, , dL, dt, , where, L is the angular momentum., Since, in the given condition,, dL, t ext = 0 Þ, =0, dt, or, L = constant, Hence, when the radius of the sphere is, increased keeping its mass same, only the, angular momentum remains constant. But, other quantities like moment of inertia,, rotational kinetic energy and angular velocity, changes., , 36. We know that, kinetic energy,, 1, 1, K = mv 2 = Iw2, 2, 2, Given,m = 27 kg (mass of the body),, w = 3 rads -1 (angular velocity), and, I = 3 kg-m 2 (moment of inertia), Iw2, Þ mv 2 = Iw2, Þ v2 =, m, 3 ´ 32, 27, 2, 2, Þ v =, =1, v =, 27, 27, Þ, , v = 1 = 1 ms -1, , 37. Before being brought in contact with the, table, the disc was in pure rotational motion,, hence v CM = 0., , 38. Rotational kinetic energy remains same., 1, 1, I 1 w12 = I 2 w22, 2, 2, 1, 1, 2, or, ( I 1 w1 ) =, ( I 2 w2 ) 2, 2I 1, 2I 2, , i.e., , L21 L22, or, =, I1 I2, , Þ, , L1, =, L2, , I1, I2, , I 1 = I , I 2 = 2I, 1, L1, I, =, =, Þ L1 : L2 = 1 : 2, 2I, L2, 2, , But, Þ, , 39. We know that, angular momentum of the, body is given by, L = Iw or L = I ´, Þ, , L, 2T, =, L2 T, , L, T, 2p, 1, or L µ, Þ 1 = 2, L2 T 1, T, T, (as, T 2 = 2T and L 1 = L )
Page 150 :
CBSE New Pattern ~ Physics 11th (Term-I), , L, . Thus, on doubling the time, 2, period, angular momentum of body becomes, half., 40. As there is no external torque, so if the girl, bends her hands, her moment of inertia, about the rotational axis will decrease. By, conservation of angular momentum,, L = Iw = constant. So, in order to keep L, constant, if I is decreasing, then w will, increase., So,, , L2 =, , 41. As no external torque acts on the system,, angular momentum should be conserved., Hence, I w = constant., ...(i), where, I is moment of inertia of the system, and w is angular velocity of the system., From Eq. (i) I1w1 = I 2 w2, where, w1 and w2 are angular velocities, before and after jumping), I, Iw = ´ w2, Þ, 2, (as mass reduced to half, hence moment of, inertia also reduced to half), Þ w2 = 2w, , 42. Velocity of the particle,, v P = r w = ( 2R ), w = 2 v, P, v, , ⇒, , 2v, , 45. A bangle is in the form of a ring as shown in, the adjacent diagram. The centre of mass lies, at the centre, which is outside the body, (boundary)., , C, Centre, , 46. As rods are uniform, therefore centre of mass, of both rods will be at their geometrical, centres. The coordinates of CM of first rod C 1, æL ö, are ç , 0 ÷ and second rod C 2 are (0, L)., è2 ø, y, C2, (0, L), , 2M, CM, M, x, C1 L , 0, 2, , O, , \ x CM =, y CM, , æL ö, M ç ÷ + 2M ( 0 ), è 2ø, M + 2M, , =, , L, 6, , M ( 0 ) + 2M ( L ) 2L, =, =, M + 2M, 3, , æ L 2L ö, Hence, coordinates of CM are ç ,, ÷., è6 3 ø, , r, , ω, v0 = Rω, , 139, , ω, , 43. Work done = DK = Change in rotational, kinetic energy + Change in linear kinetic, energy, 1, 1, 2, = mv CM, + Iw2, 2, 2, (Q I = mr 2 and v CM = rw), 2, = mv CM, = 100 ´ ( 20 ´ 10 -2 ) 2 = 4 J, 44. When a body rolls down without slipping along, an inclined plane of inclination q, it rotates, about a horizontal axis through its centre of, mass and its centre of mass also moves., As it rolls down, it suffers loss in gravitational, potential energy which provides translational, energy and due to frictional force, it gets, converted into rotational energy., , 47. The role of moment of inertia in the study of, rotational motion is analogous to that of mass, in study of linear motion., , 48. As, t = Ia = Mk 2a, Þ a=, , t, Mk 2, , Þ a=, , 10, = 8 rad s - 2, 5 ´ 0.5 ´ 0.5, , 49. We know that, angular acceleration,, dw, , given w = constant, dt, where, w is angular velocity of the disc., dw, 0, Þa=, =, =0, dt, dt, Hence, angular acceleration is zero., , a=
Page 151 :
140, , CBSE New Pattern ~ Physics 11th (Term-I), , But it is not necessary that, translational, motion of body is always in straight line. A, parabolic motion of an object without, rotation is also translational motion., Therefore, A is true but R is false., , 50. Velocity of the particle at Q ,, v Q = r w = Rw, Velocity of the particle at P,, v P = r w = ( 2R ) w = 2 vQ, P, , 2v, , 54. The centre of mass of a body may lie on or, outside the body., , v, ω, , ⇒, , r, , Q, , vQ = Rω, , Q, , ω, , Hence, points near the top move faster than, points near the bottom., 2, 51. L 1 = Iw = MR 2w, 5, 2, , L2 =, , 2 æRö, M ç ÷ w¢, 5 è 2ø, , L 1 = L 2 Þ w¢ = 4 w, 2p, æ 2p ö, =4ç ÷, \, èT ø, T¢, T, Þ, T¢=, 4, , As, , æ1ö, Time period will become ç ÷ th., è 4ø, 2, , L, 2I, Since, angular momentum is constant and I, has become (1/4)th., Therefore, kinetic energy will become 4, times., Hence, A ® r, B ® p and C ® q., , Further, K =, , 52. If v is the velocity of centre of mass of the, body of radius r, then, velocity at point A, v A = 0, velocity at point B, v B = v 2, velocity at point C, vC = v + rw = 2v, velocity of point D , vD = rw = v, Hence, A ® q, B ® p, C ® s and D ® r., , 53. The motion of centre of mass describes the, translational part of the motion., In translational motion, all points of a, moving body move along a straight line, i.e., the relative velocities between any two, particles, must be zero., , CM, , CM, , (a), , (b), , Hence, in Fig. (a), centre of mass is on the, body and in Fig. (b), centre of mass does not, lie on the body., The centre of mass of an object is the, average position of all the parts of the, system, weighted according to their masses., Therefore, centre of mass of a body lie at the, geometric centre of body., Therefore, A is false and R is also false., , 55. We know that, centre of mass of half disc, depends only on radius and not only the, density of the material of disc similarly in this, case centre of mass of half filled sphere will, depends only on radius and not on density of, liquid inside. Since, both sphere are of same, radius so both have CM at the same level., Therefore, A is true and R is false., , 56. L = mvr sin q or mvr^, In case of constant velocity m , v and r^ all are, constant., Therefore, angular momentum is constant., h, Further, L = n, (in Bohr’s theory), 2p, Hence, L and h have same units., Therefore, A is true but R is false., , 57. Angular momentum remains constant as, particle is moving in a straight line. The, angular momentum is constant, when particle, moves with a uniform velocity., Therefore, both A and R are true but R is, not the correct explanation of A.
Page 152 :
CBSE New Pattern ~ Physics 11th (Term-I), , 141, , 58. When t ext = 0, then L = constant., , ω, , So, for a system of particles under central, force field, the total angular momentum on, the system is conserved because torque acting, on such a system is zero., Therefore, both A and R are true and R is, the correct explanation of A., , 59. There is a difference between inertia and, , v, , Therefore, A is false and R is also false., , 64. The work done on a body is given by, W = ò F × vdt , where F is force of friction., , moment of inertia of a body. The inertia of a, body depends only upon the mass of the, body but the moment of inertia of a body, about an axis not only depend upon the mass, of the body but also upon the distribution of, mass about the axis of rotation., Inertia represents the capacity (ability) of a, body to oppose its state of motion or rest., , For the rolling disc without slipping down an, inclined plane, the velocity of the particle on, which the friction force is acting, is zero., Hence, work done is zero, i.e. when the disc, rolls without slipping, the friction force is, required because for rolling condition,, velocity of point of contact is zero., Therefore, both A and R are true and R is, the correct explanation of A., , Therefore, A is true but R is false., , 60. Moment of inertia changes with axis chosen., It is because moment of inertia of a particle, depends on its mass and its distance from, axis of rotation., , 65. Centre of mass of a system of two particles, is, Then, rCM =, , Therefore, A is true but R is false., , 61. Angular velocity for a rigid body can be, , If m 1 + m 2 = M = total mass of the particles,, m r + m 2r2, then, rCM = 1 1, M, \, rCM µ1/ M, So, the above relation clearly shows that the, centre of mass of a system of two particles, divide the distance between them in inverse, ratio of masses of particles., , described as the rate of change at which the, object rotates about an axis. It is defined for, the whole body., Angular velocity of particle of rigid body is, same in rotational motion., Therefore, both A and R are true but R is, not the correct explanation of A., , 62. Friction force between sliding body and, inclined plane depends upon the nature of, surfaces of both the body and inclined plane,, hence if bodies slide down an inclined plane, without rolling, then it is not necessary that, all bodies reach the bottom simultaneously., Acceleration of all bodies are also not equal, due to different values of friction between the, surfaces of body and inclined plane., Therefore, A is true but R is false., , 63. Sphere can roll without slipping on surface, if, v = r w on an inclined plane, it is friction, which creates rotation on sphere. So, smooth, surface cannot create rotation., , m 1r1 + m 2r2, m1 + m2, , 66. Let the coordinates of the centre of mass be, ( x , y )., \, , x=, , m 1x 1 + m 2x 2, m1 + m2, , 1 ´ (-1) + 2 ´ 2 -1 + 4, =, =1, 3, 3, m y + m 2y 2, y= 1 1, m1 + m2, =, , 1 ´ 2 + 2 ´ 4 2 + 8 10, =, =, 3, 3, 3, Therefore, the coordinates of centre of mass, æ 10 ö, be ç1, ÷ ., è 3ø, =
Page 153 :
142, , CBSE New Pattern ~ Physics 11th (Term-I), , 67. As the balls were initially at rest and the, forces of attraction are internal, then their, centre of mass (CM) will always remain at, rest., So, v CM = 0, As CM is at rest, they will meet at CM., Hence, they will meet at l/2 from any initial, positions., , 68. For a single particle, distance of centre of, mass from origin is R. For more than one, particles, distance £ R., , 69. As per the question, two particles A and B, are initially at rest, move towards each other, under a mutual force of attraction. It means, that, no external force is applied on the, system. Therefore, F ext = 0., So, there is no acceleration of CM. This, means velocity of the CM remain constant., As, initial velocity of CM, v i = 0 and final, velocity of CM, v f = 0., So, the speed of centre of mass of the system, will be zero., , 70. The tip of the pencil provides a vertically, upward force due to which the cardboard is, in equilibrium. As shown in given figure, the, reaction of the tip is equal and opposite to, Mg, the total weight of the cardboard, i.e., R = Mg ., , 71. Net t due to all the forces of gravity, m 1 g , m 2 g , ×××, m n g about CG is zero., t of reaction R about CG is also zero as it is, at CG., Point G is the centre of gravity of the, cardboard and it is so located that the total, torque on it due to forces m 1 g, m 2 g, .., m n g is, zero., It means, t g = S t i, = S ri ´ m i g = 0., , 72. As, t g = Sri ´ m i g, (t g = total gravitational torque), S ri ´ m i g = 0, If g is constant,, ( Sm i ri ) ´ g = g S m i ri, As g ¹ 0, so S m i ri = 0, , It is the condition where the centre of mass, (CM) of the body lies at origin and here origin, is considered at centre of gravity (CG), when g, is constant., , 73. If the value of g varies, then CM and CG, will not coincide. Keep in mind that, CG and, CM both are two different concepts. CM has, nothing to do with CG., , 74. A body in a gravitational field will be in, stable equilibrium, if the vertical line through, CG passes from the base of the body., , 75. Moment of inertia of a body depends on, position and orientation of the axis of, rotation with respect to the body., , 76. Perpendicular distance from Z -axis would be, (1) 2 + (1) 2 = 2 m, \ I = Mr 2 = (1) ( 2 ) 2 = 2 kg -m2, , 77. Initial moment of inertia, I =, , ml 2, 3, , New moment of inertia,, I¢=, , ( 3m / 4 ) ( 3l / 4 ) 2 27 æml 2 ö 27, I, =, ç, ÷=, 64 è 3 ø 64, 3, , 78. A circular disc is made up of larger number, of circular rings., Moment of inertia of a circular ring in given, by, I = MR 2, Þ, I µM, Since, mass is proportional to the density of, material. The density of iron is more than, that of aluminium. Hence to get maximum, value of I , the less dense material should be, used at interior and denser at the, surrounding., Therefore, using aluminium at the interior, and iron at its surrounding will maximise the, moment of inertia., , 79. Moment of inertia of the rod lying along, Z -axis will be zero. Moment of inertia of the, ML 2, rods along X and Y -axes will be, each., 3, 2, Hence, total moment of inertia is ML 2 ., 3
Page 154 :
CBSE New Pattern ~ Physics 11th (Term-I), , 80. The frictional force will reduce v 0 , hence, translational KE will also decrease., It will increases w, which increases its, rotational kinetic energy., There is no torque about the line of contact,, angular momentum will remain constant. The, frictional force will decrease the mechanical, energy., g sin q g sin 30°, g /2, 81. a =, =, Þ a =, = g /3, 1, k2, 3/ 2, 1+, 1+ 2, 2, R, , 82. As we know that,, ω, v, , m, , gs, , in, , fs, , θ, , The direction of f s will be upwards to, provide torque for rolling of sphere., , 83. KE of a rolling body = Rotational, KE + Translational KE, =, =, , 1 2 1 2, Iw + mv CM, 2, 2, 2, 1 m k 2v CM, 1, 2, + mv CM, 2, 2 R, 2, , ö, æQ I = mk 2, ÷, ç, è and v CM = Rwø, , 143, where, k is the corresponding radius of, gyration of the body., k2 ö, 1 2 æ, = mv CM, ç1 + 2 ÷, è, R ø, 2, It applies for any rolling body., , 84. When a body rolls down on inclined plane, it, is accompanied by rotational and, translational kinetic energies., 1, Rotational kinetic energy = Iw2 = K R, 2, where, I is the moment of inertia and w is, the angular velocity., Translational kinetic energy for pure rolling,, v CM = rw, 1 2, 1, = mv CM, = K T = m ( rw) 2, 2, 2, where, m is mass of the body, v CM is the, velocity and w is the angular velocity., Given,, Translational KE = Rotational KE, 1, 1, \, m ( r 2 w2 ) = Iw2, 2, 2, Þ, I = mr 2, We know that, mr 2 is the moment of inertia of, hollow cylinder about its axis, where m is the, mass of hollow cylindrical body and r is the, radius of the cylinder.
Page 155 :
144, , CBSE New Pattern ~ Physics 11th (Term-I), , 08, Gravitation, Quick Revision, 1. Universal Law of Gravitation It states that,, every body in this universe attracts every other, body with a force whose magnitude is directly, proportional to the product of their masses and, inversely proportional to the square of the, distance between their centres., mm, Gravitational force, F = G 1 2 2, r, where, G is a constant of proportionality and is, known as universal gravitational constant., In CGS system, the value of G is, 6.67 ´ 10 -8 dyne cm 2g -2 and its SI value is, 6.67 ´ 10 -11 N-m 2kg -2 ., Dimensional formula for G is [M -1L3T - 2 ]., 2. Vector Form of Newton’s Law of, Gravitation In vector notation, Newton’s law, of gravitation is written as follows, mm, …(i), F12 = - G 12 2 r$21, r21, where, F12 = gravitational force exerted on A by, B and r$21 is a unit vector pointing towards A., Negative sign shows that the gravitational force, is attractive in nature., mm, Similarly, F21 = - G 12 2 r$12, …(ii), r12, where, r$12 is a unit vector pointing towards B., Equating Eqs. (i) and (ii), we have, F12 = - F21, , As, F12 and F21 are directed towards the, centres of the two particles, so gravitational, force is a central force., 3. Principle of Superposition According to, this principle, the resultant gravitational force, F can be expressed in vector addition of all, forces, at a point (as shown below)., i.e., F = F12 + F13 + F14 + ... + F1n, m4, ^, r41, , ^, r31, F14 F13, m1, ^, F1n, , ^, r21, , m3, , m2, , F12, , ^, rn 1, mn, , Resultant force,, æm, ö, m, m, F = - Gm 1 ç 22 r$21 + 23 r$31 +... + 2n r$n 1 ÷, r31, rn 1, è r21, ø, 4. Acceleration due to gravity The, acceleration produced in the motion of a body, under the effect of gravity is called, acceleration due to gravity ( g )., GM, At the surface of the earth, g = 2, R
Page 156 :
CBSE New Pattern ~ Physics 11th (Term-I), , 5. Weight of a body It is the gravitational force, with which a body is attracted towards the, centre of the earth w = mg, It is a vector quantity and its SI unit is, newton (N)., 6. Factors Affecting Acceleration Due to, Gravity, ● Shape of Earth Acceleration due to gravity,, 1, g µ 2, R, Therefore, g is minimum at equator and, maximum at poles., ●, , Rotation of Earth about Its Own Axis If, w is the angular velocity of rotation of earth, about its own axis, then acceleration due to, gravity at a place having latitude l is given, by, g ¢ = g - Rw2 cos 2 l, At poles, l = 90° and g ¢ = g ., Therefore, there is no effect of rotation of, earth about its own axis at poles., At equator, l = 0° and g ¢ = g - Rw2, The value of g is minimum at equator., , ●, , ●, , If earth stops its rotation about its own axis,, then g will remain unchanged at poles but, increases by Rw2 at equator., Effect of Altitude The value of g at height, h from earth’s surface,, g, g¢=, 2, hö, æ, ç1 + ÷, è, Rø, Therefore, g decreases with altitude., Effect of Depth The value of g at depth h, from earth’s surface,, hö, æ, g ¢ = g ç1 - ÷, è, Rø, Therefore, g decreases with depth from, earth’s surface., The value of g becomes zero at earth’s, centre., , 145, 7. Intensity of Gravitational Field at a, Point The gravitational force acting per unit, mass at any point in gravitational field is called, intensity of gravitational field at that point., Intensity of gravitational field at a distance r ,, from a body of mass M is given as, F GM, E =, = 2, m, r, It is a vector quantity and its direction is, towards the centre of gravity., 8. Gravitational Potential Gravitational, potential at a point in the gravitational field is, defined as the amount of work done per unit, mass in bringing a body of unit mass from, infinity to that point without acceleration., W, i.e., V =m, F ×d r, -GM, =-ò, = - ò E ×d r =, m, r, It is a scalar quantity. The unit of gravitational, potential in SI system is Jkg –1 and in CGS, system is erg-g –1 ., Dimensional formula for gravitational potential, is [M 0L2T -2 ]., Special Cases, ● When r = ¥, then V = 0, hence gravitational, potential is maximum (zero) at infinity., ● At surface of the earth r = R , then, -GM, V =, R, 9. Gravitational Potential Energy, Gravitational potential energy of a body at a, point is defined as the amount of work done in, bringing the given body from infinity to that, point against the gravitational force., Gravitational potential energy,, æ GM ö, U = ç÷ ´m, è r ø, 10. Escape Speed Escape speed on the earth, (or any other planet) is defined as the, minimum speed with which a body should be, projected vertically upwards from the surface
Page 157 :
146, , CBSE New Pattern ~ Physics 11th (Term-I), , of the earth, so that it just escapes out from, gravitational field of the earth and never, returns on its own., \ Escape velocity, v e = 2 gR, where, R is the radius of the earth., 8, Also, escape velocity, v e = R, p Gr, 3, where, r is the mean density of the earth., 11. Earth’s Satellites A satellite is a body which, is constantly revolving in an orbit around a, comparatively much larger body. e.g. The, moon is a natural satellite while INSAT-1B is, an artificial satellite of the earth. Condition for, establishment of satellite is that the centre of, orbit of satellite must coincide with centre of, the earth or satellite must move around in, greater circle of the earth., 12. Orbital Velocity of a Satellite Orbital, velocity is the velocity required to put the, satellite into its orbit around the earth or a, planet., Satellite, m, , vo, , R+h, R, O, Earth, , h, , Mathematically, it is given by v o =, , GM, R +h, , 13. Energy of an Orbiting Satellite When a, satellite revolves around a planet in its orbit, it, possesses both potential energy (due to its, position against gravitational pull of the earth), and kinetic energy (due to orbital motion). If m, is the mass of the satellite and v is its orbital, velocity, then KE of the satellite,, 1, 1 GM, (Qv = GM /r ), K = mv 2 = m, 2, 2, r, GMm, (Qr = R + h ), K =, 2 (R + h ), , GMm, R +h, Total mechanical energy of satellite,, E = K +U, GMm, E =2(R + h ), , PE of the satellite, U = - mv 2 = -, , Satellites are always at finite distance from the, earth and hence their energies cannot be, positive or zero., 14. Geo-stationary Satellite These satellites, revolves in a circular orbits around the earth in, the equatorial plane with period of revolution, same as that of earth, i.e. T = 24 h and also, known as geo-synchronous satellites., ● It should revolve in an orbit concentric and, coplanar with the equatorial plane of earth., ● These satellites appears stationary due to its, law relative velocity w.r.t. that place on, earth., ● It should be at a height around 36000 km., ● These satellites are used for communication, purpose like radio broadcast, TV broadcast,, etc., 15. Polar Satellite They are low-altitude satellites, (h » 500 to 800 km) which circle in a, North-South orbit passing over the North and, South poles. It is also known as sun, synchronous satellite., ● The time period is about 100 min., ● These satellites are used for military purpose., 16. Weightlessness A body is said to be in a state, of weightlessness when the relation of the, supporting surface is zero or its apparent, weight is zero. At one particular position, the, two gravitational pulls may be equal &, opposite and the net pull on the body becomes, zero. This is zero gravity region or the null, point where the body is said to be weightless., The state of weightlessness can be observed in, the following situations, ● When objects fall freely under gravity., ● When a satellite revolves in its orbit around, the earth., ● When bodies are at null points in outer space.
Page 158 :
CBSE New Pattern ~ Physics 11th (Term-I), , 147, , Objective Questions, Multiple Choice Questions, , 6. If the distance between the sun and the, , 1. The force of gravitation is, , earth is increased by three times, then, attraction between two will, , (a) repulsive, (c) conservative, , (b) electrostatic, (d) non-conservative, , 2. Newton’s law of gravitation is universal, because, (a) it acts on all bodies in the universe, (b) it acts on all the masses at all distances and, not affected by the medium, (c) it is a attractive force, (d) it acts only when bodies are in contact, , 3. Both the earth and the moon are, subject to the gravitational force of the, sun. As observed from the sun, the, orbit of the moon, (NCERT Exemplar), (a) will be elliptical, (b) will not be strictly elliptical because the, total gravitational force on it is not central, (c) is not elliptical but will necessarily be a, closed curve, (d) deviates considerably from being elliptical, due to influence of planets other than the, earth, , 4. Two sphere of masses m and M are, situated in air and the gravitational, force between them is F . The space, around the masses is now filled with a, liquid of specific gravity. The, gravitational force will now be, (a) F, (c), , F, 9, , (b), , F, 3, , (d) 3 F, , 5. A mass of 1g is separated from another, mass of 1g by a distance of 1 cm. How, many force (in g-wt) exists between, them?, (a) 7 ´ 10- 11 g-wt, (c) 9 ´ 10- 11 g-wt, , (b) 7 ´ 1011 g-wt, (d) 9 ´ 1011 g-wt, , (a) remain constant, (c) increase by 63%, , (b) decrease by 63%, (d) decrease by 89%, , 7. If the gravitation force on body 1 due, to 2 is given by F12 and on body 2 due, to 1 is given as F 21 , then, (a) F12 = F21, (b) F12 = - F21, F21, (c) F12 =, 4, (d) None of the above, , 8. Two equal point masses are separated, by a distance d 1 . The force of, gravitation acting between them is F 1 ., If the separation is decreased to d 2 ,, then the new force of gravitation F 2 is, given by, æd ö, (b) F2 = F1 ç 1 ÷, è d2 ø, , (a) F2 = F1, æd ö, (c) F2 = F1 ç 2 ÷, è d1 ø, , 2, , 2, , æd ö, (d) F2 = F1 ç 1 ÷, è d2 ø, , 9. Particles of masses 2M , m and M are, respectively at points A, B and C with, 1, AB = (BC ), m is much-much smaller, 2, than M and at time t = 0, they are all at, rest as given in figure. At subsequent, times before any collision takes place., (NCERT Exemplar), A, , B, , C, , 2M, , m, , M, , (a) m will remain at rest, (b) m will move towards M, (c) m will move towards 2M, (d) m will have oscillatory motion
Page 159 :
148, , CBSE New Pattern ~ Physics 11th (Term-I), , 10. Two particles of equal masses go round, a circle of radius R under the action of, their mutual gravitational attraction., The speed v of each particle is, æ GM ö, (a) ç, ÷, è 2R ø, (c), , (b), , 1 æ GM ö, ç, ÷, 2 è R ø, , 1 æ 1 ö, ç, ÷, 2 R è GM ø, , æ 4GM ö, (d) ç, ÷, è R ø, , 11. A point mass m is placed outside a, hollow spherical shell of mass M and, uniform density at a distance d from, centre of the sphere as shown in figure., Gravitational force on point mass m at, P is, d, , m, P, , GmM, d2, 2 GmM, (c), d2, , (b) zero, (d) Data insufficient, , placed at the vertices of an equilateral, triangle and a mass of 4 kg is placed at, the centroid of the triangle which is at a, distance of 2 m from each of the, vertices of the triangle. The force, (in newton) acting on the mass of 4 kg, is, (b) 2, , (c) 1, , (d) zero, , 13. During the free fall of an object,, (a), (b), (c), (d), , 15. If G is universal gravitational constant, and g is acceleration due to gravity, then, G, the unit of the quantity, is, g, (a) kg-m2, (c) kgm-2, , (b) kgm -1, (d) m2 kg -1, , 16. The earth is an approximate sphere. If, the interior contained matter which is, not of the same density everywhere,, then on the surface of the earth, the, acceleration due to gravity, (NCERT Exemplar), , 12. Three equal masses of 2 kg each are, , (a) 2, , No change, Increases, Decreases but not become zero, Reduces to zero, , (a) will be directed towards the centre but not, the same everywhere, (b) will have the same value everywhere but, not directed towards the centre, (c) will be same everywhere in magnitude, directed towards the centre, (d) cannot be zero at any point, , M, , (a), , (a), (b), (c), (d), , acceleration due to gravity is zero, force on object is zero, force on object decreases with height, acceleration due to gravity is 9.8 m/s 2, , 14. What will happen to the weight of the, body at the south-pole, if the earth, stops rotating about its polar axis?, , 17. The height at which the weight of a, body becomes 1/16th of its weight, on, the surface of the earth (radius R), is, (a) 5R, (c) 3 R, , (b) 15 R, (d) 4 R, , 18. The radius of earth is R. Height of a, point vertically above the earth’s, surface at which acceleration due to, gravity becomes 1% of its value at the, surface is, (a) 8 R, (c) 10 R, , (b) 9 R, (d) 20 R, , 19. What will be the value of g at the, bottom of sea 7 km deep? Diameter of, the earth is 12800 km and g on the, surface of the earth is 9.8 ms -2 ., (a) 9.789 m/s 2, , (b) 9.259 m/s 2, , (c) 97.89 m/s 2, , (d) 0987, m/s 2, .
Page 160 :
CBSE New Pattern ~ Physics 11th (Term-I), , 149, , 20. Starting from the centre of the earth, , 24. The gravitational potential energy of a, , having radius R, the variation of g, (acceleration due to gravity) is shown, by which of the following option ?, (a), , g, , O, , (b), r, , R, , (a) directly proportional to product of the, masses of particles, (b) inversely proportional to the separation, between them, (c) independent of distance r, (d) Both (a) and (b), , g, , O, , g, , system consisting two particles, separated by a distance r is, , r, , R, , 25. The mass of the earth is 6.00 ´ 10 24 kg, , g, , (c), , (d), O, , R, , O, , r, , r, , R, , 21. Dependence of intensity of gravitational, field ( E ) of earth with distance (r ) from, centre of earth is correctly represented, by, E, , (a) O, , E, , R, , (b) O, , r, , E, , (c) O, , r, , (d) O, , r, , R, , (a) 3.80 ´ 108 m, (c) 7.60 ´ 104m, , r, , separated by a distance r. The, gravitational potential energy of the, system is G1 . When the separation, between the particles is doubled, the, gravitational potential energy is G 2 ., G, Then, the ratio of 1 is, G2, (a) 1, , 22. A thin rod of length L is bent to form a, semicircle. The mass of rod is M . What, will be the gravitational potential at the, centre of the circle?, GM, L, pGM, (c) 2L, , (a) -, , (b) 2, , (b) 3.33 ´ 10 -10 J, (d) 6.67 ´ 10 - 8 J, , (d) 4, , system of particles as shown in the, figure is, m1, , GM, 2pL, pGM, (d) L, , uniform sphere of mass 100 kg and, radius 10 cm. Find the work to be done, per unit mass against the gravitational, force between them, to take the particle, far away from the sphere (you may take, h = 6.67 ´ 10 -11 Nm 2 kg -2 ) ., , (c) 3, , 27. Gravitational potential energy of a, , (b) -, , 23. A particle is kept on the surface of a, , (a) 13.34 ´ 10 -10 J, (c) 6.67 ´ 10 - 9 J, , (b) 3.37 ´ 106 m, (d) 1.90 ´ 102 m, , 26. Two point masses m 1 and m 2 are, , R, , E, R, , and that of the moon is 7.40 ´ 10 22 kg ., The constant of gravitation, G = 6.67 ´ 10 -11 N-m 2 kg - 2 . The, potential energy of the system is, - 7.79 ´ 10 28 J. The mean distance, between the earth and the moon is, , r3, , r1, , m2, , (a), (b), (c), (d), , r2, , m3, , Gm1 m2 Gm2 m3 Gm1 m3, +, +, r1, r3, r3, æ - Gm1 m2 ö æ - Gm2 m3 ö æ - Gm1 m3 ö, ÷+ç, ÷, ç, ÷+ç, è, ø è, r1, r2, r3, ø, ø è, - Gm1 m2 Gm2 m3 Gm1 m3, +, r1, r2, r3, Gm1 m2 Gm2 m3 Gm1 m3, +, r1, r2, r3
Page 161 :
150, , CBSE New Pattern ~ Physics 11th (Term-I), , 28. Escape velocity of a body on the, surface of earth is independent of, (a), (b), (c), (d), , mass, radius of earth, direction of projection of body, Both (a) and (c), , 29. An object is thrown from the surface of, the moon. The escape speed for the, object is, (a) 2g ¢ Rm , where g¢= acceleration due to, gravity on the moon and Rm = radius of the, moon, (b) 2g ¢ Re , where g¢ = acceleration due to, gravity on the moon and Re = radius of the, earth, (c) 2gRm , where g = acceleration due to, gravity on the earth and Rm = radius of the, moon, (d) None of the above, , 30. A body is projected vertically upwards, from the surface of a planet of radius R, with a velocity equal to 1/3rd of the, escape velocity for the planet. The, maximum height attained by the body, is, (a) R/2, (c) R/5, , (b) R/3, (d) R/9, , 31. Two planets A and B have the same, material density. If the radius of A is, twice that of B, then the ratio of escape, v, velocity A is, vB, (a) 2, 1, (c), 2, , (b) 2, 1, (d), 2, , 32. Escape velocity on earth is 11.2 kms -1 ,, what would be the escape velocity on a, planet whose mass is 1000 times and, radius is 10 times that of earth?, (a) 112 kms -1, (c) 1.12 kms -1, , (b) 11.2 kms -1, (d) 3.7 kms -1, , 33. The time period of a satellite in a, circular orbit around a planet is, independent of, (a), (b), (c), (d), , the mass of the planet, the radius of the planet, the mass of the satellite, All the three parameters (a), (b) and (c), , 34. Satellites orbiting the earth have finite, life and sometimes debris of satellites, fall to the earth. This is because, (NCERT Exemplar), , (a) the solar cells and batteries in satellites run, out, (b) the laws of gravitation predict a trajectory, spiralling inwards, (c) of viscous forces causing the speed of, satellite and hence height to gradually, decrease, (d) of collisions with other satellites, , 35. An artificial satellite is revolving around, the earth, close to its surface. Find the, orbital velocity of artificial satellite?, (Take, radius of earth = 6400 km), (a) 7.2 km/s, (c) 11.2 km/s, , (b) 7.9 km/s, (d) 9.5 km/s, , 36. Two satellites A and B go around a, planet P in circular orbits having radius, 4R and R, respectively. If the speed of, satellite A is 3v, then the speed of satellite, B will be, (a) 6v, (b) 9v, (c) 3v, (d) None of the above, , 37. An artificial satellite moving in a, circular orbit around the earth has a, total (kinetic + potential) energy E 0 . Its, potential energy and kinetic energy, respectively are, (a) 2 E0 and – 2 E0, (c) 2 E0 and - E0, , (b) – 2 E0 and E0, (d) – 2 E0 and – E0
Page 162 :
CBSE New Pattern ~ Physics 11th (Term-I), , 38. The kinetic energy of the satellite in a, circular orbit with speed v is given as, (a) KE =, , -GmM e, 2(Re + h), , (b) KE =, , (c) KE =, , GmM e, 2 (Re + h), , (d) KE = -, , GmM e, (Re + h), 1, mv 2, 2, , 39. The time period of geo-stationary, satellite is, (a) 6 h, , (b) 12 h, , (c) 24 h, , (d) 48 h, , 40. Geo-stationary satellites are placed in, equatorial orbits at the height, approximately, (a) 1000 km, (c) 25000 km, , (b) 15000 km, (d) 36000 km, , 41. An astronaut experiences, weightlessness in a space satellite. It is, because, (a) the gravitational force is small at that, location in space., (b) the gravitational force is large at that, location in space., (c) the astronaut experiences no gravity, (d) the gravitational force is infinitely large at, that location in space., , 42. A pendulum beats sounds on the earth., Its time period on a stationary satellite, of the earth will be, (a) zero, (c) 2s, , (b) 1s, (d) infinity, , 43. …… is defined to be numerically equal, to the force of attraction between two, bodies each of mass 1 kg and separated, by a distance of 1 m., (a), (b), (c), (d), , Universal gravitational constant (G), Gravity (g), Force (F), Magnetic field (B), , 44. Weight of a body is maximum at, ……… ., (a) poles, (c) centre of earth, , (b) equator, (d) at latitude 45°, , 151, , 45. The ratio of the magnitude of potential, energy and kinetic energy of a satellite, is ……… ., (a) 1 :2, (c) 3 :1, , (b) 2 :1, (d) 1 : 3, , 46. Weightlessness experienced while, orbiting the earth in spaceship, is the, result of ……… ., (a), (b), (c), (d), , inertia, acceleration, zero gravity, centre of gravity, , 47. Which of the following statement is, incorrect?, , (NCERT Exemplar), , (a) Acceleration due to gravity decreases with, increasing altitude., (b) Acceleration due to gravity increases with, increasing depth (assume the earth to be a, sphere of uniform density) ., (c) Acceleration due to gravity increases with, increasing altitude., (d) None of the above, , 48. If the gravitational attraction of earth, suddenly disappears, then which of the, following statement is correct?, (a) Both masses as well as the weight will be zero., (b) Weight of the body will become zero but the, mass will remain unchanged., (c) Weight of the body will remain unchanged, but the mass will become zero., (d) Neither mass nor weight will be zero., , 49. Study the following statements and, choose the incorrect option., I. G is not equal to, 6.67 ´ 10 - 11 Nm - 2 kg - 2 on the, surface of earth., II. The escape velocity on the surface, of earth is lesser than the escape, velocity from moon’s surface., III. The angular momentum of a, satellite going around the earth, remains conserved.
Page 163 :
152, , CBSE New Pattern ~ Physics 11th (Term-I), , GM, holds good for, r2, all the celestial bodies., , IV. The relation, g =, (a) Only I, (c) Both I and II, , (b) Only III, (d) Both II and IV, , 50. Which of the following statement is, correct?, , (NCERT Exemplar), , (a) A polar satellite goes around the earth’s, pole in north-south direction., (b) A geo-stationary satellite goes around the, earth in east-west direction., (c) A geo-stationary satellite goes around the, earth in north-south direction., (d) A polar satellite goes around the earth in, east-west direction., , 51. Match the Column I (quantities) with, Column II (approximate values) and, select the correct answer from the codes, given below., Column I, , Column II, , A. Escape velocity, of earth, , p. 1.6 m/s 2, , B. Gravitational, acceleration at, moon’s surface, , q., , 6400 km, , C. Radius of earth, , r., , 11.2 km/s, , Codes, A B, (a) p q, (c) q p, , C, r, r, , A, (b) r, (d) r, , B, q, p, , C, p, q, , 52. A satellite of mass m revolving with a, velocityv around the earth. With reference, to the above situation, match the Column I, (types of energy) with Column II, (expression) and select the correct answer, from the codes given below., Column I, , Column II, , p. - 1 mv 2, 2, B. Potential energy of q. 1 mv 2, the satellite, 2, A. Kinetic energy of, the satellite, , C. Total energy of the r., satellite, , - mv 2, , Codes, A, (a) p, (b) q, (c) r, (d) r, , B, q, r, q, p, , C, r, p, p, q, , Assertion-Reasoning MCQs, For question numbers 53 to 66, two, statements are given-one labelled, Assertion (A) and the other labelled, Reason (R). Select the correct answer to, these questions from the codes (a), (b), (c), and (d) are as given below, (a) Both A and R are true and R is the, correct explanation of A., (b) Both A and R are true but R is not the, correct explanation of A., (c) A is true but R is false., (d) A is false and R is also false., , 53. Assertion Newton’s law of universal, gravitation states that a particle attracts, every other particle in the universe, using a force of attraction that is, directly proportional to the product of, their masses and inversely proportional, to the square of distance between them., Reason Law of gravitation is analogous, to magnetic force between the moving, charges., , 54. Assertion The value of acceleration, due to gravity does not depend upon, mass of the body on which force is, applied., Reason Acceleration due to gravity is a, variable quantity., , 55. Assertion As we go up the surface of, the earth, we feel light weighed than on, the surface of the earth., Reason The acceleration due to, gravity decreases on going up above, the surface of the earth.
Page 164 :
CBSE New Pattern ~ Physics 11th (Term-I), , 56. Assertion Work done by or against, gravitational force in moving a body, from one point to another is, independent of the actual path, followed between the two points., Reason This is because gravitational, forces are conservative in nature., , 57. Assertion If gravitational potential at, some point is positive, then the, gravitational field strength at that, point will also be zero., Reason Except at infinity, gravitational potential due to a, system of point masses at some finite, distance cannot be negative., , 58. Assertion The force of attraction, between a hollow spherical shell of, uniform density and a point mass, situated inside it, is zero., Reason The value of G does not, depend on the nature and size of the, masses., , 59. Assertion Moon has no atmosphere., Reason The escape speed for the, moon is much smaller., , 60. Assertion The escape velocity for a, , 153, , 61. Assertion The velocity of the satellite, increases as its height above earth’s, surface increases and is minimum near, the surface of the earth., Reason The velocity of the satellite is, directly proportional to square root of its, height above earth’s surface., , 62. Assertion A satellite moves around the, earth in a circular orbit under the action, of gravity. A person in the satellite, experience zero gravity field in the, satellite., Reason The contact force by the surface, on the person is not zero., , 63. Assertion The total energy of the satellite, is always negative irrespective of the nature, of its orbit, i.e. elliptical or circular and it, cannot be positive or zero., Reason If the total energy is negative the, satellite would leave its orbit., , 64. Assertion The geo-stationary satellite, goes around the earth in west-east, direction., Reason Geo-stationary satellites orbits, around the earth in the equatorial plane, with T = 24 h same as that of the rotation, of the earth around its axis., , planet is v e = 2gR . If the radius of, the planet is four times, the escape, v, velocity becomes half (i.e. v e ¢ = e )., 2, , 65. Assertion In the satellite, everything, , Reason In the relation for escape, velocity, v e = 2gR , the acceleration, due to gravity g is inversely, proportional to radius of the planet., 1, Thus, v e µ, ., R, , 66. Assertion An object is weightless when, , inside it is in a state of free fall., Reason Every part and parcel of the, satellite has zero acceleration., it is in free fall and this phenomenon is, called weightlessness., Reason In free fall, there is upward force, acting on the object.
Page 165 :
154, , CBSE New Pattern ~ Physics 11th (Term-I), , Case Based MCQs, Direction Answer the questions from, 67-71 on the following case., Cavendish’s Experiment, The figure shows the schematic drawing of, Cavendish’s experiment to determine the, value of the gravitational constant. The bar, AB has two small lead spheres attached at its, ends. The bar is suspended from a rigid, support by a fine wire., Two large lead spheres are brought close to, the small ones but on opposite sides as, shown. The value of G from this experiment, came to be 6.67´10 -11 N-m 2 /kg 2 ., S1′, , S2, , A, , B, S2′, , S1, , 67. The big spheres attract the nearby small, ones by a force which is, (a), (b), (c), (d), , equal and opposite, equal but in same direction, unequal and opposite, None of the above, , 68. The net force on the bar is, (a) non-zero, (c) Data insufficient, , (b) zero, (d) None of these, , 69. The net torque on the bar is, (a) zero, (b) non-zero, (c) F times the length of the bar, where F is the, force of attraction between a big sphere and, its neighbouring, (d) Both (b) and (c), , 70. The torque produces twist in the, suspended wire. The twisting stops, when, , (a) restoring torque of the wire equals the, gravitational torque, (b) restoring torque of the wire exceeds the, gravitational torque, (c) the gravitational torque exceeds the, restoring torque of the wire, (d) None of the above, , 71. After Cavendish’s experiment, there, have been given suggestions that the, value of the gravitational constant G, becomes smaller when considered over, very large time period (in billions of, years) in the future. If that happens, for, our earth,, (a) nothing will change, (b) we will become hotter after billions of years, (c) we will be going around but not strictly in, closed orbits, (d) None of the above, , Direction Answer the questions from, 72-76 on the following case., Acceleration due to gravity, The acceleration for any object moving under, the sole influence of gravity is known as, acceleration due to gravity. So, for an object, of mass m, the acceleration experienced by it, is usually denoted by the symbol g which is, related to F by Newton’s second law by, relation F = mg . Thus,, F GM e, g =, =, m, Re2, Acceleration g is readily measurable as Re is a, known quantity. The measurement of G by, Cavendish’s experiment (or otherwise),, combined with knowledge of g and Re, enables one to estimate M e from the above, equation. This is the reason why there is a, popular statement regarding Cavendish, “Cavendish weighed the earth”. The value of, g decrease as we go upwards from the earth’s, surface or downwards, but it is maximum at, its surface.
Page 166 :
CBSE New Pattern ~ Physics 11th (Term-I), , 72. If g is the acceleration due to gravity at, the surface of the earth, the force acting, on the particle of mass m placed at the, surface is, (a) mg, , GmM e, (b), Re2, , (c) Data insufficient, , (d) Both (a) and (b), , 73. The weight of a body at the centre of, earth is, (a), (b), (c), (d), , same as on the surface of earth, same as on the poles, same as on the equator, None of the above, , 74. If the mass of the sun is ten times, smaller and gravitational constant G is, ten times larger in magnitude, then for, earth,, (a) walking on ground would become more, easy, (b) acceleration due to gravity on the earth will, not change, (c) raindrops will fall much slower, (d) airplanes will have to travel much faster, , 75. Suppose, the acceleration due to gravity, at the earth’s surface is 10 ms -2 and at, the surface of mars, it is 4.0 ms -2 . A, 60 kg passenger goes from the earth to, the mars in a spaceship moving with a, constant velocity. Neglect all other, objects in the sky., , Which curve best represents the weight, (net gravitational force) of the, passenger as a function of time?, Weight, , 76. If the mass of the earth is doubled and, its radius halved, then new acceleration, due to the gravity g ¢ is, (a) g ¢ = 4 g, (c) g ¢ = g, , (b) g ¢ = 8 g, (d) g ¢ = 16 g, , Direction Answer the questions from, 77-81 on the following case., Earth’s Satellite, Earth satellites are objects which revolve, around the earth. Their motion is very similar, to the motion of planets around the Sun. In, particular, their orbits around the earth are, circular or elliptic. Moon is the only natural, satellite of the earth with a near circular orbit, with a time period of approximately 27.3 days, which is also roughly equal to the rotational, period of the moon about its own axis. Also,, the speed that a satellite needs to be travelling, to break free of a planet or moon’s gravity, well and leave it without further propulsion is, known as escape velocity. For example, a, spacecraft leaving the surface of earth needs, to be going 7 miles per second or nearly, 25000 miles per hour to leave without falling, back to the surface or falling into orbit., , 77. Gas escapes from the surface of a planet, because it acquires an escape velocity., The escape velocity will depend on, which of the following factors?, (a), (b), (c), (d), , Mass of the planet, Mass of the particle escaping, Temperature of the planet, None of the above, , 78. The escape velocity of a satellite from, the earth is v e . If the radius of earth, contracts to (1 /4 )th of its value, keeping, the mass of the earth constant, escape, velocity will be, , 600 N, A, 240 N, , B, C, D, , (a) A, , 155, , (b) B, , t0, , (c) C, , Time, , (d) D, , (a), (b), (c), (d), , doubled, halved, tripled, unaltered
Page 167 :
156, , CBSE New Pattern ~ Physics 11th (Term-I), , 79. The ratio of escape velocity at earth (v e ), to the escape velocity at a planet (v p ),, whose radius and mean density are, twice as that of earth is, (b) 1: 4, (d) 1: 2, , (a) 1 :2 2, (c) 1 : 2, , (b) the total mechanical energy of S varies, periodically with time, (c) the linear momentum of S remains, constant in magnitude, (d) the acceleration of S is always directed, towards the centre of the earth, , 81. The orbital velocity of an artificial, , 80. A satellite S is moving in an elliptical, orbit around the earth. The mass of the, satellite is very small as compared to the, mass of the earth, then, (a) the angular momentum of S about the, centre of the earth changes in direction,, but its magnitude remains constant, , satellite in a circular orbit just above the, earth’s surface is v o . The orbital velocity, of a satellite orbiting at an altitude of, half of the radius, is, (a), , 3, vo, 2, , (b), , 2, vo, 3, , (c), , 3, 2, v o (d) v o, 2, 3, , ANSWERS, Multiple Choice Questions, 1. (c), 11. (a), 21. (a), , 2. (b), 12. (d), 22. (d), , 3. (b), 13. (d), 23. (d), , 4. (a), 14. (a), 24. (d), , 5. (a), 15. (d), 25. (a), , 6. (d), 16. (d), 26. (b), , 7. (b), 17. (c), 27. (b), , 8. (b), 18. (b), 28. (d), , 9. (c), 19. (a), 29. (a), , 10. (c), 20. (b), 30. (d), , 31. (a), 41. (c), 51. (d), , 32. (a), 42. (d), 52. (b), , 33. (c), 43. (a), , 34. (c), 44. (a), , 35. (b), 45. (b), , 36. (a), 46. (c), , 37. (c), 47. (b), , 38. (c), 48. (b), , 39. (c), 49. (c), , 40. (d), 50. (a), , 55. (a), 65. (c), , 56. (a), 66. (c), , 57. (d), , 58. (b), , 59. (a), , 60. (a), , 61. (d), , 62. (c), , 69. (d), 79. (a), , 70. (a), 80 (d), , 71. (c), 81. (d), , 72. (d), , 73. (d), , 74. (d), , 75. (c), , 76. (b), , Assertion-Reasoning MCQs, 53. (c), 63. (c), , 54. (a), 64. (a), , Case Based MCQs, 67. (a), 77. (a), , 68. (b), 78. (a), , SOLUTIONS, 1. As the work done by the gravitational force, F in closed path is zero. So, it is conservative, in nature, i.e. work done by the body is, independent of path followed., , 2. According to universal law of gravitation,, gravitational force is given by, Gm 1m 2, r2, It depends on all the masses at all distances, but does not depend on medium between, them., F =, , 3. As observed from the sun, two types of forces, are acting on the moon one is due to, gravitational attraction between the sun and, the moon and the other is due to gravitational attraction between the earth and the, moon. Therefore, total force on the moon is, not central., Hence, the orbit of the moon will not be, strictly elliptical., , 4. Gravitational force does not depend on the, medium between masses., So, it will remains same, i.e. F .
Page 169 :
158, , CBSE New Pattern ~ Physics 11th (Term-I), , The gravitational force on mass 4 kg due to, mass 2 kg at point P is, 4´2, FOP = G, = 4 G , along OP, ( 2 )2, Similarly, FOQ = G, and, , FOR, , For height h above the surface of the earth,, 2, , æ R ö, g¢ æ R ö, =ç, g¢ = gç, ÷, ÷ Þ, èR + h ø, g èR + h ø, , 4´2, = 4 G , along OQ, ( 2 )2, , 4´2, =G, = 4 G , along OR, ( 2 )2, , FOQ cos 30° and FOR cos 30° are equal and, acting in opposite directions, hence cancel, out each other. Then, the resultant force on, the mass 4 kg at point O, F = 0 (zero)., Gm 1m 2, 13. Force on the object is given by F =, r2, It is not zero under free fall. As, the height, decreases, force will increases on object. The, acceleration due to gravity is constant and, equal to 9.8 m/s 2 during the free fall., , 14. As, weight of the body at pole is mg and g is, not affected by the rotation of earth at poles., So, there is no change in weight of body., GM, G R2, 15. As, we know, g = 2 or =, g, M, R, Hence, the unit of the quantity, , G m2, =, g, kg, , 16. If we assume the earth as a sphere of uniform, density, then it can be treated as point mass, placed at its centre. In this case, acceleration, due to gravity g = 0, at the centre., It is not so, if the earth is considered as a, sphere of non-uniform density, in that case, value of g will be different at different points, and cannot be zero at any point., , 17. According to the question,, GMm, 1 GMm, =, 2, (R + h ), 16 R 2, where, m = mass of the body, GM, and, = gravitational acceleration., R2, 1, 1, So,, =, 2, (R + h ), 16 R 2, or, , 1 ö, 1, ÷ g or g ¢ / g =, è100 ø, 100, , 18. Given, g ¢ = æç, , R, R+h, 1, or, =4, =, R+h 4, R, h = 3R, , Þ, \, , æ 1 ö æ R ö, ç, ÷=ç, ÷, è100 ø è R + h ø, , 2, , Þ, , 2, , R, 1, =, R + h 10, , h = 10 R - R = 9 R, , 19. Given, depth of sea, d = 7 km and g = 9.8 ms -2, Radius of the earth,, D 12800, R=, =, km = 6400 km, 2, 2, dö, æ, Value of g at bottom of sea, g d = g ç1 - ÷, è, Rø, 7 ö 9.8 ´ 6393, æ, = 9.8 ç1 ÷=, è 6400 ø, 6400, = 9.789 m/s 2, , 20. Acceleration due to gravity at a depth d, below the surface of the earth is given by, æ dö, g depth = g surface ç1 - ÷, è Rø, æR -dö, ær ö, = g surface ç, ÷ = g surface ç ÷, è R ø, èRø, Also, for a point at height h above surface,, é R2 ù, g height = g surface ê, 2ú, ë(R + h ) û, Therefore, we can say that value of g increases, from centre to maximum at the surface and, then decreases as depicted in graph (b)., , 21. Dependence of gravitational field ( E ) with, distance is depicted properly in option (a), because at centre r = 0,, \, E =0, For a point outside the earth (r > R),, GM, 1, E =- 2 ÞE µ 2, r, r, and at the surface of earth (r = R),, GM, E =- 2, R, Inside the earth (r < R),, GMr, E = - 3 Þ E µr, R
Page 170 :
CBSE New Pattern ~ Physics 11th (Term-I), , 22. Since, length if rod is equal to the, circumference of semicircle, L, p, Therefore, the gravitational at the centre of, circle will be, GM, pGM, V ==R, L, GM, 23. Gravitational potential, V i = r, 6.67 ´ 10 -11 ´ 100, Vi = 0.1, 6.67 ´ 10 -9, Vi = = - 6.67 ´ 10 -8 J, 0.1, Q Vf = 0, \ Work done per unit mass,, , 159, , 26. As we know, G 1 =, , pR = L Þ R =, , m1, , r, , 25. Given, U = -7.79 ´ 10 28 J, G = 6.67 ´ 10 -11 N-m2 kg -2, m = 6 ´ 10 24 kg, and, M = 7.40 ´ 10 22 kg, Potential energy of the system,, - GMm, U =, R, Þ - 7.79 ´ 10 28, - 6.67 ´10 -11 ´ 7.4 ´ 10 22 ´ 6 ´ 10 24, =, R, - 6.67 ´ 10 -11 ´ 7.4 ´ 10 22 ´ 6 ´ 10 24, Þ R=, - 7.79 ´ 10 28, R = 3.8 ´ 10 8 m, , …(ii), , G1, = 2 [dividing Eq. (i) by Eq. (ii)], G2, , = U 12 + U 23 + U 31, - Gm 1m 2 Gm 2m 3 Gm 1m 3, =, r1, r2, r3, æ - Gm 1m 2 ö æ - Gm 2m 3 ö æ - Gm 1m 3 ö, =ç, ÷+ç, ÷+ç, ÷, è, ø è, ø è, ø, r1, r2, r3, , 28. Escape velocity on the surface of earth is, m2, , Gravitational potential energy (U ) of the, above system is given as, Gm 1m 2, U =r, 1, i.e. U µm 1m 2 and U µ or gravitational, r, potential energy is directly proportional to, the product of the masses of particles and, inversely proportional to the separation, between them., , Þ, , m2, , are taken and total gravitational potential, energy is the algebraic sum of the potential, energies due to each pair, applying the, principle of superposition., Total gravitational potential energy, , 24. Two point masses m 1 and m 2 are separated by, , m1, , …(i), , 27. For a system of particles, all possible pairs, , W = DV = (V f - V i ) = 6.67 ´ 10 -8 J, a distance r is shown as, , r, , Gm 1m 2, G2 = 2r, , and, \, , - Gm 1m 2, r, , given by, v = 2g Re, i.e., , v µ Re, , Hence, escape velocity does not depend on, the mass and direction of projection of body,, it depends on the radius of earth., , 29. Escape speed from the moon = 2g ¢Rm, where, g ¢ = acceleration due to gravity on the, surface of moon, and Rm = radius of the moon., , 30. Let h be the maximum height attained. Then, from equation of the motion v 2 = u 2 + 2gh, When u = 0, v = 2 gh, , ve, , where v e = 2gR, 3, 1, Þ, 2 gh =, 2 gR, 3, On squaring both sides, we get, R, h =, 9, Given,, , v =, , 31. Escape velocity is given by, ve =, , 2GM, =, R, , 2G 4, ´ p R 3r, R, 3
Page 171 :
160, , CBSE New Pattern ~ Physics 11th (Term-I), , 8, p Gr, 3, , Þ, , ve = R, , Þ, , v µR, v A RA, =, =2, v B RB, , \, , 1, 2, , [Q r is same for A and B], , =, , [Q RA = 2RB , given ], , GM, M, 32. v e = 2gR = 2 2 × R or v e µ, R, R, Mass is 1000 times and radius is 10 times., (1000 M )G, 10 R, , \, , v e¢ =, , Þ, , v e¢ = 10, , Þ, , v e¢ = 10 ´ 11.2 Þ v e¢ = 112 kms -1, , GM, Þ v e¢ = 10v e, R, , 33. The time period of satellite in a circular orbit, around a planet is independent of the mass of, satellite., , 34. As the total energy of the earth satellite, , - GM ö, æ, bounded system is negative ç i.e., ÷,, è, 2a ø, , where a is radius of the satellite and M is, mass of the earth., Due to the viscous force acting on satellite,, energy decreases continuously and radius of, the orbit or height decreases gradually., , 35. Here, Re = 6400 km = 6.4 ´ 10 6 m, g = 9.8 m/s 2, Hence, orbital velocity of artificial satellite, near the earth’s surface,, v o = gRe = 9.8 ´ 6.4 ´ 10 6, = 7. 9 ´ 10 3 m/s = 7.9 km/s, , 36. Velocity of satellite varies inversely as the, square root of the orbit of radius R,, 1, v µ, R, RB, =, RA, , 1, 2, , æ, , GM e ö, ÷, è ( Re + h ) ø, , 2, , 38. KE of satellite = mv 2 = m ç, , \, , vA, =, vB, , R, 4R, , Þ, , 3v 1, =, Þ v B = 6v, vB 2, , GMm, 2r, GMm, Potential energy, U = = 2E 0, r, GMm, Kinetic energy, K = +, = - E0, 2r, , 37. Q Total energy, E 0 = -, , 1 GmM e, 2 ( Re + h ), , 39. Geo-stationary satellite has an orbital period, equal to earth’s rotational period of 23 h and, 56 min, i.e. approx. 24 h., 40. A geo-stationary satellite is an earth’s orbiting, satellite. It is placed at an altitude of, approximately 36000 km directly over the, equator and it revolves in the same direction, the earth rotates., 41. An astronaut experiences weightlessness in a, space satellite. It is because the astronaut, experiences no gravity., , 42. Inside a satellite, every object experiences, weightlessness. Therefore, time period of a, L, pendulum inside a satellite is T = 2 p, g, As,, g =0, \, T = ¥ (infinity), , 43. According to universal law of gravitation,, F =, , Gm 1m 2, Fr 2, Þ G =, 2, r, m 1m 2, , Here, m 1 = m 2 = 1 kg and r = 1 m, \, G =F, 44. At poles, value of g is maximum. So, there is, no effect of rotation of earth., , 45. The ratio of magnitude of PE and KE of a, satellite is 2 : 1., PE, - mv 2, 2, =, =, = 2 :1, 1, KE, 1, mv 2, 2, 46. Weightlessness experienced while orbiting the, earth in spaceship is the result of zero gravity, because the surface does not exert any force, on the body., GMm, By Newton’s law,, - R = ma, r2, GMm, æGM ö, Þ, - R =m ç 2 ÷ Þ R = 0, è r ø, r2, Q, , Since, reaction force by the surface of, spaceship is zero, so body will experience, weightlessness. This happens in zero gravity, region and also from, w = mg , when g = 0,, then w = 0.
Page 172 :
CBSE New Pattern ~ Physics 11th (Term-I), , 47. Acceleration due to gravity at altitude h ,, gh =, , g, »g, (1 + h / R ) 2, , 2h ö, æ, ç1 ÷, è, Rø, , dö, æ, At depth d , g d = g ç1 - ÷, è, Rø, In both cases, with increase in h and d , g, decreases., At latitude f, g f = g - w2 R cos 2 f, As f increases g f increases., So, option (b) is incorrect., , 48. As, w = mg and g = 0., So, weight of the body will become zero but, the mass will remain unchanged., , 49. The statements I and II are incorrect and, these can be corrected as,, As, G = 6.67 ´ 10 - 11 N-m2 kg - 2 on the surface, of earth., The escape velocity on the surface of earth is, greater than the escape velocity from moon’s, surface because the moon has no atmosphere, while earth has a very draws one., , 50. A geo-stationary satellite is having same, sense of rotation as that of earth, i.e. west-east, direction., A polar satellite goes around the earth’s pole, in north-south direction., Earth, , Geo-stationary, satellite, , 161, C. Radius of moon is 1740 km while radius of, earth is 6400 km., Hence, A ® r, B ® p and C ® q., A., If the velocity of satellite is v and mass m ,, 52., then, 1, KE = mv 2, 2, B. Since, potential energy of the satellite, = - 2 kinetic energy of satellite, Þ PE = - mv 2 ), C. Also, total energy = KE + PE, 1, 1, = mv 2 - mv 2 = - mv 2, 2, 2, Hence, A ® q, B ® r and C ® p., , 53. According to universal law of gravitation,, Gm 1m 2, r2, This force of attraction between two bodies is, directly proportional to products of their, masses and inversely proportional to the, square of distance between them., Law of gravitation is not analogous to, magnetic force between the moving charges., Therefore, A is true but R is false., F =, , 54. Acceleration due to gravity is given by, GM, R2, Thus, it doesn’t depend on mass of the body, on which it is acting. Also, it is a variable, quantity, it changes with change in value of, both M and R., Therefore, both A and R are true and R is, the correct explanation of A., g =, , 55. Variation of acceleration due to gravity at, height is given by, , Earth, , Polar satellite, , 51. A. Escape velocity of earth is 11.2 km/s while, for moon is 2.4 km/s., B. Gravitational acceleration of earth is, 9.8 ms 2 while for moon’s surface is, 1.6 m/s 2 ., , 2h ö, æ, g h = ç1 ÷, è, Rø, Since, acceleration due to gravity decreases, above the surface of the earth and weight is, directly proportional to the acceleration due, to gravity, so as we go up, we feel light, weighted than on the surface of the earth., Therefore, both A and R are true and R is, the correct explanation of A.
Page 173 :
162, , CBSE New Pattern ~ Physics 11th (Term-I), , 56. As, work done by or against gravitational, force in moving a body from one point to, another is independent of the actual path, followed because it is conservative force of, nature which only depends on the initial and, final positions., Therefore, both A and R are true and R is, the correct explanation of A., , 57. Gravitational potential due to a point mass at, some finite distance is always negative., Gravitational potential at infinity is zero., Therefore, A is false and R is also false., , 58. The force of attraction due to a hollow, spherical shell of uniform density on a point, mass situated inside it, is zero because, gravitational force possesses spherical, symmetry., Gravitational force is conservative in nature, and the value of G does not depend on the, nature and size of the masses., Therefore, both A and R are true but R is, not the correct explanation of A., , 59. The escape speed for the moon is much, smaller and hence any gas molecule formed, having thermal velocity larger than escape, speed will escape from the gravitational pull, of the moon., So, moon has no atmosphere., Therefore, both A and R are true and R is, the correct explanation of A., , 60. Escape velocity, v e = 2gR, GM, 2 GM, Þ ve =, 2, R, R, 1, i.e., ve µ, R, So, if radius is four times, i.e. R ¢ = 4 R, where, g =, , v ¢e =, , 2 GM 1, =, ( 4R ), 2, , 2 GM v e, =, R, 2, , Therefore, both A and R are true and R is, the correct explanation of A., GM e, 61. Orbital velocity of satellite, v o =, ( Re + h ), Þ, , vo µ, , 1, Re + h, , Thus, v o is maximum near the surface of the, earth for h = 0., GM e, ( v o ) max =, Re, Therefore, A is false and R is also false., , 62. The person experiences zero net force as the, force of gravity is balanced by the centrifugal, force inside the satellite. So, person, experience no gravity., The contact force by the surface on the, person is zero., Therefore, A is true but R is false., , 63. Total energy of a satellite is always negative, irrespective of the nature of its orbit. It, indicates that the satellite is bound to the, earth. At infinity, the potential energy and, kinetic energy of satellite is zero., Hence, total energy at infinity is zero,, therefore only negative energy of satellite is, possible when it is revolved around the earth., If it is positive or zero, the satellite would, leave its definite orbit and escape to infinity., Therefore, A is true but R is false., , 64. The geo-stationary satellite goes around the, earth in west-east direction., It is because it orbits around earth in the, equatorial plane with a time period of 24 h, same as that of rotation of the earth around, its axis., Therefore, both A and R are true and R is, the correct explanation of A., , 65. In a satellite around the earth, every part and, parcel of the satellite has an acceleration, towards the centre of the earth which is, exactly the value of earth’s acceleration due, to gravity at that position., Thus, in the satellite, everything inside it is in, a state of free fall., Therefore, A is true but R is false., , 66. An object is weightless when it is in free fall, as during free fall, there is no upward force, acting on the body and this phenomenon is, called weightlessness., Therefore, A is true but R is false., 67. The force of attraction on small spheres due, to big sphere are equal and opposite in
Page 174 :
CBSE New Pattern ~ Physics 11th (Term-I), , direction. Hence, equal and opposite force, separated by a fixed distance forms a couple., , 163, , Þ, , F = mg =, , GmM e, Re2, , Hence, options (a) and (b) are correct., F, A, , m, m, , 73. Gravitational acceleration (g ) at the centre of, B, , F, , 68., , earth is zero, hence weight of body ( w = mg ), at the centre of earth becomes zero., , 74. Consider the given diagram, , | Fnet | = zero, F, A, Bar, , B, , F, , Since, the force are equal and opposite, net, force on the bar is zero., , 69. Magnitude of torque due to a couple, = (Either Force) ´ (Distance between of forces), =F ´l, where, l = length of the bar, and F = force of attraction between a big, sphere and its neighbouring small sphere., , 70. The torque produces a twist in the suspended, wire. The twisting stops when the restoring, torque of the wire equal the gravitational, torque., , 71. We know that, gravitational force between, the earth and the sun., GMm, , where M is mass of the sun and, FG =, r2, m is mass of the earth., When G decreases with time, the, gravitational force FG will become weaker, with time. As FG is changing with time. Due, to it, the earth will be going around the sun, not strictly in closed orbit and radius also, increases, since the attraction force is getting, weaker. Hence, after long time the earth will, leave the solar system., , 72. The force acting on the particle of mass m at, surface of the earth,, …(i), F = mg, where, g = acceleration due to gravity at the, earth’s surface., GM, Also,, …(ii), g = 2e, Re, Then, from Eqs. (i) and (ii), we get, , Sun, , m, r, Earth, , Object, , Force on the object due to the earth,, G ¢ M em 10 GM em, (Q G ¢ = 10 G ), F =, =, R2, R2, æGM em ö, ...(i), = 10 ç, ÷ = (10 g ) m = 10 mg, è R2 ø, GM, ö, æ, çQ g = 2 e ÷, è, R ø, Now, force on the object due to the sun ,, GM s¢m, F¢=, r2, G ( M s )m, M ö, æ, =, çQ M s¢ = s ÷, è, 10 r 2, 10 ø, As, r >> R (radius of the earth), Þ F ¢ will be very small, so the effect of the, sun will be neglected., Now, as g ¢ = 10 g, Hence, weight of person = mg ¢ = 10 mg, [from Eq. (i)], i.e. Gravity pull on the person will increase., Due to it, walking on ground would become, more difficult., Escape velocity v e is proportional to g , i.e., ve µ g., As, g ¢ > g Þ v e ¢ > v e, Hence, rain drops will fall much faster., To overcome the increased gravitational force, of the earth, the airplanes will have to travel, much faster.
Page 175 :
164, , CBSE New Pattern ~ Physics 11th (Term-I), , 75. Initially, the weight of the passenger at the, earth’s surface, w = mg = 60 ´ 10 = 600 N., Finally, the weight of the passenger at the, surface of the mars = 60 ´ 4 = 240 N and, during the flight in between somewhere its, weight will be zero because at that point,, gravitational pull of earth and mars will be, equal., Only the curve (c) represents the weight = 0., So, (c) is correct option., , 76. As we know that, acceleration due to gravity,, g =, , GM, R2, , Given, M ¢ = 2M, Þ, , R ¢ = ( R / 2), , (Q mass gets doubled), (Q radius gets halved), , Then, acceleration becomes, GM ¢ G ( 2M ) 8 GM, Þ, g¢ =, =, =, ( R / 2) 2, R ¢2, R2, , 77. As we know that, escape velocity,, 2GM, R, , …(i), , where, M is mass of planet., So, on the basis of Eq. (i), it can be said that, escape velocity will depend upon the mass of, the planet (M )., , 78. Given, escape velocity on the surface of, earth,, ve =, , 2GM e, Re, , where, M e = mass of the earth, and, Re = radius of the earth., Now, according to the question, radius of, earth,, R¢ = R e /4, , æ 2 GM e ö, 2GM e, = 4ç, ÷, è Re ø, R¢, , =2, , 2GM e, Re, , or, v e¢ = 2 v e, Hence, the escape velocity will be doubled., 79. Since, the escape velocity of earth can be, given as, v e = 2gR, Þ ve = R, , 8, pGr (r = density of earth)…(i), 3, , As it is given that, the radius and mean, density of planet are twice as that of earth., So, escape velocity at planet will be, v p = 2R, , \, g¢ =8g, Thus, the new acceleration due to gravity g ¢, is 8 times that of g ., ve =, , v e¢ =, , Þ, , 8, pG 2r, 3, , …(ii), , Dividing Eq. (i) by Eq. (ii), we get, 8, R, pGr, ve, 3, =, vp, 8, 2R, pG 2r, 3, ve, 1, Þ, =, =1: 2 2, vp 2 2, , 80. As, we know that, force on satellite is only, gravitational force which will always be, towards the centre of earth. Thus, the, acceleration of S is always directed towards, the centre of the earth., GM, 81. Orbital velocity is given by v o =, r, where,, r = R + h., R, R 3, If h = , then r = R +, = R, 2, 2 2, Then orbital velocity of satellite orbiting at, half altitude becomes,, GM ´ 2, 2, \, v =, =, vo, 3R, 3
Page 176 :
165, , Practice Paper 1, , PRACTICE PAPER 1, Physics Class 11th (Term I), Instructions, 1. This paper has 35 questions., 2. All questions are compulsory., 3. Each question carry 1 mark., 4. Answer the questions as per given instructions., Time : 90 Minutes, , Max. Marks : 35, , Multiple Choice Questions, , 4. What is the maximum height attained, , 1. A cyclist is travelling with velocity v on a, banked curved road of radius R. The, angle q through which the cyclist lean, inwards is given by, Rg, v2, v2R, (c) tanq =, g, , (a) tanq =, , (a) R /2, , (b) R / 3, , (c) R /5, , (d) R /8, , (b) tanq = v 2 Rg, (d) tanq =, , v2, Rg, , 2. Three particles each of mass m are kept, at vertices of an equilateral triangle of, side L. The gravitational potential at the, centre due to these particles is, 3Gm, L, - 9 Gm, (b), 3L, , 5. A body of mass 2 kg is thrown up, vertically with kinetic energy of 490 J., The height at which the kinetic energy, of the body becomes half of its original, value is, (a) 50 m, (c) 25 m, , (b) 12.25 m, (d) 10 m, , 6. A rocket is fired vertically from the, , (a) -, , 3 3 Gm, L, (d) Both (b) and (c), (c) -, , 3. Two bodies having masses m 1 & m 2 and, velocities v 1 & v 2 collide and form a, composite system. If, m 1v 1 + m 2v 2 = 0 (m 1 ¹ m 2 ), then the, velocity of composite system will be, (a) v 1 - v 2, v + v2, (c) 1, 2, , by a body projected with a velocity, equal to one-third of the escape velocity, from the surface of the earth? (Take,, radius of the earth = R), , (b) v 1 + v 2, (d) zero, , surface of mars with a speed of 2 kms -1 ., If 20% of its initial energy is lost due to, martian atmospheric resistance, how far, will the rocket go from the surface of, mars before returning to it? (Take, mass, of mars = 6.4 ´ 10 23 kg , radius of mars, = 3395 km and, G = 6.67 ´ 10 -11 N-m 2 kg -2 ), (a) 685 km, (c) 495 km, , (b) 785 km, (d) 500 km, , 7. Force F on a particle moving in a, straight line varies with distance d as, shown in figure.
Page 177 :
166, , CBSE New Pattern ~ Physics 11th (Term-I), , The work done on the particle during, its displacement of 12 m is, , (a) 25 ms -2, (c) 25 rad s -2, , (b) 0.25 rad s -2, (d) 5 ms -2, , 12. A body of mass 2kg travels according to, , F(N), , the law x (t ) = pt + qt 2 + rt 3 , where, q = 4ms -2 , p = 3ms -1 and r = 5ms -3 ., The force acting on the body at t = 2s is, , 2, , (a) 136 N, (c) 158 N, 0, , (a) 18 J, , 3, , 12, , 7, , (b) 21 J, , d(m), , (c) 26 J, , 13. The orbital velocity of a body close to, (d) 13 J, , 8. Weak nuclear forces operates among, (a) all charged particles, (b) electrons and neutrino, (c) all objects in universe, (d) Both (a) and (b), , the earth’s surface is, (a) 8 kms -1, (c) 3 ´ 108 ms -1, , are connected by strings, as shown in, the figure.After an upward force F is, applied on block m, the masses move, upward at constant speed v. What is the, net force on the block of mass 2m?, v, , m, , mass 5 kg resting on a horizontal plane., The body gains a kinetic energy of 10 J, after it moves a distance 2 m. The, frictional force is, (a) 10 N, (c) 20 N, , (b) 15 N, (d) 30 N, , 15. If E energy is required to project a, body with speed v, what is the energy, required to give an initial speed of 2v to, the body?, (a) E, (c) 4E, , 2m, , (b) 2E, (d) E /2, , 16. A block of mass 10 kg is suspended by, three strings as shown in the figure. The, tension T 2 is, , 3m, , (a) Zero, (b) 2 mg, (c) 3 mg, (d) 6 mg, Here, g is the acceleration due to gravity., , 60°, , length 1 km and the unit of time is 100s,, what will be the unit of mass?, (c) 102 kg, , (d) 104 kg, , 11. A rope is wound around a hollow, cylinder of mass 3 kg and radius 40 cm., What is the angular acceleration of the, cylinder, if the rope is pulled with a, force of 30 N?, , 30°, , T2, , T3, T1, , 10. If the unit of force is 1 kN, unit of, (a) 10- 4 kg (b) 103 kg, , (b) 11.2 kms -1, (d) 2.2 ´ 103 kms -1, , 14. A force of 20 N is applied on a body of, , 9. Three blocks with masses m , 2m and 3m, , F, , (b) 134 N, (d) 68 N, , 10 kg, s, , (a) 100 N, (c), , 3 ´ 100 N, , 100, (b), N, 3, (d) 50 3 N, , 17. A smooth sphere of mass M moving, with velocity u directly collides, elastically with another sphere of mass, m at rest. After collision, their final
Page 178 :
167, , Practice Paper 1, , velocities are v ¢ and v, respectively. The, value of v is, 2uM, m, 2u, (c), m, 1+, M, , 2um, M, 2u, (d), M, 1+, m, , (b), , (a), , 18. A solid cylinder rolls down an inclined, plane of height 3 m and reaches the, bottom of plane with angular velocity,, of 2 2 rad s -1 . The radius of cylinder, must be (take, g = 10 ms -2 ), (a) 5 cm, (c) 10 cm, , (b) 0.5 cm, (d) 5 m, , 19. The displacement of a particle is given, , by x = (t - 2) 2 m, where x is in metre, and t is in second. The distance covered, by the particle in first 4 s is, (a) 4 m, , (b) 8 m, , (c) 12 m, , (d) 16 m, , 20. A projectile can have same range for, two angles of projection with same, initial speed. If h1 and h 2 be the, maximum heights attained by them,, then, (a) R = h1h2, (b) R = 2h1h2, (c) R = 4 h1h2, (d) R = 2 h1h2, , about any diameter is ……… ., R, 2, (c) 2R, , R, 2, (d) R, (b), , 22. The SI unit of intensity of gravitational, field is ……… ., (a), (b), (c), (d), , N kg - 1, N kg, N-m kg - 1, N m- 1kg - 1, , For question numbers 23 to 27, two, statements are given-one labelled, Assertion (A) and the other labelled, Reason (R). Select the correct answer to, these questions from the codes (a), (b), (c), and (d) are as given below, (a) Both A and R are true and R is the, correct explanation of A., (b) Both A and R are true but R is not the, correct explanation of A., (c) A is true but R is false., (d) A is false and R is also false., , 23. Assertion For a system of particles, under central force field, the total, angular momentum is conserved., Reason The torque acting on such a, system is zero., , 24. Assertion Mass and energy are not, conserved separately, but are conserved, as a single entity called mass-energy., Reason Mass and energy are, inter-convertible in accordance with, Einstein’s relation, E = mc 2 ., , 25. Assertion In the system of two blocks, , 21. The radius of gyration of a thin ring, (a), , Assertion-Reasoning MCQs, , of equal masses as shown, the, coefficient of friction between the, blocks (m 2 ) is less than coefficient of, friction (m 1 ) between lower block and, ground., µ2, , m, m, , F, µ1, , For all values of force F applied on, upper block, lower block remains at, rest.
Page 179 :
168, , CBSE New Pattern ~ Physics 11th (Term-I), , Reason Frictional force on lower block, due to upper block is not sufficient to, overcome the frictional force on lower, block due to ground., , 26. Assertion Spring force, friction force,, normal force, tension in rope, etc., are, similar forces., Reason They arise out of the, gravitational force between the, particles., , 27. Assertion The light year and, wavelength consist of dimensions of, length., Reason Both light year and wavelength, represent time., , A = $i + 2$j + 2k$ and B = 3$i + 6 $j + 2k$ ., Another vector C has the same, magnitude as that of B but has the same, direction as that of A. Then, which of, the following vectors represents C?, 7 $, ( i + 2 $j + 2 k$ ), 3, 7, (c) ( $i - 2 $j + 2 k$ ), 9, (a), , 3 $ $, ( i - 2 j + 2 k$ ), 7, 9, (d) ($i + 2 $j + 2 k$ ), 7, , (b), , 29. The X and Y -components of a force F, acting at 30º to X-axis are respectively, F, ,F, 2, 3 1, (c), F, F, 2, 2, , (a), , F 3, ,, F, 2 2, F, (d) F,, 2, , (b), , 30. A person walks in the following pattern, , Case Based MCQs, Direction Answer the questions from, 28-31 on the following case., Resolution of Vectors, The process of splitting a single vector into, two or more vectors are known as resolution, of vectors. These vectors are in different, directions which collectively has same effect, as that of by the single vector alone., With the help of graph shown below, P(x, y), j, , 3.1 km north, then 2.4 km west and, finally 5.2 km south. How far the, person will be from its initial point?, (a) 4.2 km, (c) 3.0 km, , (b) 3.2 km, (d) 8.0 km, , 31. A unit radial vector $r makes angles of, a = 30º relative to the X-axis, b = 60º, relative to the Y-axis and g = 90º, relative to the Z-axis. The vector $r can, be written as, 1$, 3$, i+, j, 2, 2, 2$, 1 $, (c), i+, j, 3, 3, , (a), , (b), , 3$ 1$, i+ j, 2, 2, , (d) None of these, , Direction Answer the questions from, 32-35 on the following case., , r, θ, O, , 28. Two vectors are given by, , i, , Any vector r can be expressed as a linear, combination of two unit vectors $i and $j at, right angle, i.e. r = x$i + y$j in two-dimension, and r = x$i + y$j + z k$ in three-dimension., , Acceleration, Acceleration is defined as the rate of change, of velocity with time. Since, velocity is a, quantity having both magnitude and, direction, a change in velocity may involve, either or both of these factors.
Page 180 :
169, , Practice Paper 1, , Acceleration, therefore may result from a, change in speed (magnitude), a change in, direction or changes in both., For motion with constant acceleration, the, average acceleration equals the constant value, of acceleration during the interval., 32. The acceleration of a moving car is, , found from, (a) area under velocity-time graph, (b) area under displacement-time graph, (c) slope of distance-time graph, (d) slope of velocity-time graph, , 33. The motion of a car along a straight, line is described by equation, x = 8 + 12t - t 3 , where x is in metre and, t is in second. The retardation of the, particle, when its velocity becomes, zero, is, , (a) 24 ms- 2, (c) 6 ms- 2, , (b) zero, (d) 12 ms - 2, , 34. The graph between displacement and, time for a particle moving with uniform, acceleration is, (a), (b), (c), (d), , straight line with a positive slope, parabola, ellipse, straight line parallel to time axis, , 35. At a certain time, a car has a speed of, 18 m/s in positive x-direction and 2.4 s, later its speed was 30 m/s in the, opposite direction., What is the magnitude of the average, acceleration of the particle during the, 2.4 s interval?, (a) 20 m/s 2, (c) 5 m/s 2, , (b) 10 m/s 2, (d) 2.5 m/s 2
Page 181 :
170, , CBSE New Pattern ~ Physics 11th (Term-I), , PRACTICE PAPER 1, OMRSHEET, Instructions, Use black or blue ball point pens and avoid gel pens and fountain, pens for filling the sheets, Darken the bubbles completely. Don't put a tick mark or a cross, mark half-filled or over-filled bubbles will not be read by the software., , ü, , û, , Incorrect, , Incorrect, , Incorrect, , Correct, , Do not write anything on the OMR Sheet, Multiple markings are invalid, 1, , a, , 19, , a, , d, , c, , b, , a, , 6, , b, , c, , b, , c, , 2, , a, , b, , c, , d, , 20, , a, , b, , c, , d, , 3, , a, , b, , d, , 21, , a, , b, , c, , d, , 4, , a, , c, , d, , 22, , a, , b, , c, , d, , 5, , b, , c, , d, , 23, , a, , b, , c, , d, , a, , b, , c, , d, , 24, , a, , b, , c, , d, , 7, , a, , b, , c, , d, , 25, , a, , b, , c, , d, , 8, , a, , b, , c, , d, , 26, , a, , b, , c, , d, , 9, , a, , b, , c, , d, , 27, , a, , b, , c, , d, , 10, , a, , b, , c, , d, , 28, , a, , b, , c, , d, , 11, , a, , b, , c, , d, , 29, , a, , b, , c, , d, , 12, , a, , b, , c, , d, , 30, , a, , b, , c, , d, , 13, , a, , b, , c, , d, , 31, , a, , b, , c, , d, , 14, , a, , b, , c, , d, , 32, , a, , b, , c, , d, , 15, , a, , b, , c, , d, , 33, , a, , b, , c, , d, , 16, , a, , b, , c, , d, , 34, , a, , b, , c, , d, , 17, , a, , b, , c, , d, , 35, , a, , b, , c, , d, , 18, , a, , b, , c, , d, , d, , Students should not write anything below this line, , SIGNATURE OF EXAMINER WITH DATE, , MARKS SCORED
Page 182 :
171, , Practice Paper 2, , PRACTICE PAPER 2, Physics Class 11th (Term I), Instructions, 1. This paper has 35 questions., 2. All questions are compulsory., 3. Each question carry 1 mark., 4. Answer the questions as per given instructions., Time : 90 Minutes, , Max. Marks : 35, , Multiple Choice Questions, 1. If the length and time period of an, oscillating pendulum have errors 1% and, 3% respectively, then the error in, measurement of acceleration due to, gravity is, (a) 4%, , (b) 5%, , (c) 6%, , (d) 7%, , 2. A ball is travelling with uniform, translatory motion. This means that, (a) it is at rest, (b) the path can be a straight line or circular, and the ball travels with uniform speed, (c) all parts of the ball have the same velocity, (magnitude and direction) and the velocity, is constant, (d) the centre of the ball moves with constant, velocity and the ball spins about its centre, uniformly, , 3. The equation of state for a real gas is, a, given by æç P + 2 ö÷ (v - b ) = RT, è, v ø, , The dimensions of the constant a is, 5, , -2, , (a) [ML T ], (c) [ML- 5 T- 1 ], , -1 5, , 2, , (b) [M L T ], (d) [ML5 T- 1 ], , 4. The acceleration due to gravity g and, mean density of earth r are related by, which of the following relations?, , (Take, G = gravitational constant and, R = radius of earth), 4 pgR 2, 3G, 3g, (c) r =, 4 pGR, , (a) r =, , 4 pgR 3, 3G, 3g, (d) r =, 4 pGR 3, , (b) r =, , 5. A body is rolling down an inclined, plane. Its translational and rotational, kinetic energies are equal. The body, is a, (a) solid sphere, (c) solid cylinder, , (b) hollow sphere, (d) hollow cylinder, , 6. A person is moving with a velocity of, , 10 m s -1 towards north. A car moving, with a velocity of 20 ms -1 towards, south crosses the person., The velocity of car relative to the, person is, (a) - 30 ms-1, (c) 10 ms-1, , (b) + 20ms-1, (d) - 10 ms-1, , 7. Which of the following does not specify, the correct link between technology, and physics?, (a) Optical fibres « total internal reflection, (b) Nuclear reactor « nuclear fusion, (c) Electron microscope « wave nature of, electrons, (d) Electric generator « laws of, electromagnetic induction
Page 183 :
172, , CBSE New Pattern ~ Physics 11th (Term-I), , 8. Amongst the following graphs, which, graph represents the correct relation, between the height of projectile h and, time t , when a particle (projectile) is, thrown from the ground obliquely?, h, , h, , (a), , (b), t, , O, , t, , O, , h, , (c) g, h, , h, , 12. A uniform square plate has a small, piece Q of an irregular shape removed, and glued to the centre of the plate, leaving a hole behind in figure. The, moment of inertia about the Z-axis is, , h, , (c), , (d) g, , y, , y, , (d), , Hole, , Q, , t, , O, , x, , t, , O, , x, , 9. A stone of mass 0.05 kg is thrown, vertically upwards. What is the, magnitude and direction of net force on, the stone during its upward motion?, (a), (b), (c), (d), , 0.98 N, vertically downwards, 0.49 N, vertically downwards, 9.8 N, vertically downwards, 0.49 N, vertically upwards, , from the same point with the same, initial speeds making angle 30°, 45° and, 60°, respectively with the horizontally., Which of the following statement is, correct?, (a) A,B and C have unequal ranges., (b) Ranges of A and C are less than that of B ., (c) Ranges of A and C are equal and greater, than that of B., (d) A, B and C have equal ranges., , 11. Which of the following graph shows the, variation of acceleration due to gravity, g with depth h from the surface of the, earth?, (b) g, h, , increased, decreased, the same, changed in unpredicted manner, , 13. The object is released from rest under, , 10. Three particles A, B and C projected, , (a) g, , (a), (b), (c), (d), , h, , gravity at y = 0. The equation of motion, which correctly expresses the above, situation is, , (a), (b), (c), (d), , v = -9.8 t ms-1, v = (9.8 - 9.8 t) m/s, v 2 = - 19.6 y 2 m2s-2, v 2 = (v 02 + 29.6 y) m 2 s -2, , 14. The unification of electromagnetism, and optics leads to the, (a), (b), (c), (d), , celestial and terrestrial mechanics, discovery of uncertainty principle, discovery of optical fibres, discovery of light as an electromagnetic, wave, , 15. A body falling from the rest has a, velocity v after it falls through a height, h. The distance it has to fall down, further for its velocity to become, double, will be, (a) 8h, (c) 4h, , (b) 6h, (d) 5h
Page 184 :
173, , Practice Paper 2, , 16. An aircraft executes a horizontal loop, of radius 1 km with a speed of, 900 kmh - 1 . What is the ratio of its, centripetal acceleration with the, acceleration due to gravity?, (a) 6.38, (c) 12.76, , (b) 3.19, (d) 5.38, , 17. A body possessing kinetic energy T, moving on a rough horizontal surface is, stopped in a distance y. The frictional, force exerted on the body is, T, y, T, (d), y, , (a)Ty, (c), , (b), , T, y, , 18. A particle moves from a point, , ( - 2$i + 5$j ) to ( 4 $j + 3k$ ) when a force of, ( 4 $i + 3$j ) N is applied. How much work, has been done by the force?, (a) 8 J, (c) 5 J, , (b) 11 J, (d) 2 J, , 19. The displacement-time graph of two, moving particles make angles of 30°, and 60° with the X -axis. The ratio of, their velocities is, , (a) 1 : 3, (c) 1 :1, , (a), (b), (c), (d), , the sign of displacement, the initial position of the object, the final position of the object, None of the above, , 22. In projectile motion, both the, magnitude and direction of acceleration, ……… ., (a) decreases with the height, (b) increases with the height, (c) remains constant, (d) increases with the range, , Assertion-Reasoning MCQs, For question numbers 23 to 27, two, statements are given-one labelled, Assertion (A) and the other labelled, Reason (R). Select the correct answer to, these questions from the codes (a), (b), (c), and (d) are as given below, (a) Both A and R are true and R is the, correct explanation of A., (b) Both A and R are true but R is not the, correct explanation of A., (c) A is true but R is false., (d) A is false and R is also false., , pendulum on a satellite orbiting the, earth is infinity., 30°, , Reason Time-period of a pendulum is, inversely proportional to g ., , 60°, Time (t), , (b) 1 :2, (d) 3 :2, , 20. The ceiling of a long hall is 25 m high., What is the maximum horizontal, distance that a ball thrown with a speed, of 40 ms - 1 can go without hitting the, ceiling of the hall?, (a) 150.5 m, (c) 130.2 m, , velocity depends only upon ……… ., , 23. Assertion The time-period of, , Displacement (x), , 0, , 21. The sign (+ ve or - ve) of the average, , (b) 250.5 m, (d) 100.5 m, , 24. Assertion In case of pure rolling, the, force of friction becomes zero., Reason The speed at the point of, contact is non-zero., , 25. Assertion A lift is ascending with, decreasing speed means acceleration of, lift is downwards., Reason A body always moves in the, direction of its acceleration.
Page 185 :
174, , CBSE New Pattern ~ Physics 11th (Term-I), , 26. Assertion The centripetal and, , F1, , F2, , centrifugal forces never cancel out., Reason They do not act at the same, time., , Body, , 27. Assertion In universe, gravitational, force dominates in long distance and, electric force dominates in short distance., Reason For gravitational force µ, charge 2, mass 2, and, electric, force, µ, distance 2, distance 2, , Case Based MCQs, Direction Answer the questions from, 28-31 on the following case., Equilibrium of a Particle, Equilibrium of a particle in mechanics refers, to the situation when the net external force on, the particle is zero. According to the first law,, the particle is either at rest or in uniform, motion., If two forces F1 and F 2 act on a particle,, equilibrium requires, F1 = - F 2, i.e. The two forces on the particle must be, equal and opposite. Equilibrium under three, concurrent forces F1 , F 2 and F 3 requires that, the vector sum of three forces is zero., F1 + F 2 + F 3 = 0, In other words, the resultant of any two forces, say F1 and F 2 , obtained by the parallelogram, law of forces must be equal and opposite to, the third force F 3 ., , 28. Three forces F 1 , F 2 and F 3 together, keep a body in equilibrium. If F 1 = 3 N, along the positive X-axis, F 2 = 4N along, the positive Y-axis, then the third force, F 3 is, , F3, , æ 3ö, (a) 5 N making an angle q = tan- 1 ç ÷ with, è4ø, negative Y-axis, æ4ö, (b) 5N making an angle q = tan- 1 ç ÷ with, è 3ø, negative Y-axis, æ 3ö, (c) 7N making an angle q = tan- 1 ç ÷ with, è4ø, negative Y-axis, æ4ö, (d) 7N making an angle q = tan- 1 ç ÷ with, è 3ø, negative Y-axis, , 29. Three concurrent coplanar forces, 1N, 2N and 3N acting along different, directions on a body, (a) can keep the body in equilibrium, if 2N and, 3N act at right angle, (b) can keep the body in equilibrium, if 1N and, 2N act at right angle, (c) cannot keep the body in equilibrium, (d) can keep the body in equilibrium, if 1N and, 3N act at an acute angle, , 30. P , Q and R are three coplanar forces, acting at a point and are in equilibrium., Given, P = 19318, kg-wt and, ., sin q 1 = 0.9659, find the value of R (in, kg-wt)., P, , Q, 150°, θ2, , θ1, R, , (a) 0.9659 (b) 2, , (c) 1, , (d), , 1, 2
Page 186 :
175, , Practice Paper 2, , 31. A mass of 10 kg is suspended from a, spring balance. It is pulled aside by a, horizontal string, so that it makes an, angle of 60º with the vertical. The new, reading of the balance is, (a) 10 3 kg-wt, (c) 20 kg-wt, , (b) 20 3 kg-wt, (d) 10 kg-wt, , Direction Answer the questions from, 32-35 on the following case., Collision, A collision is an isolated event in which two, or more colliding bodies exert strong forces, on each other for a relatively short time. For a, collision to take place, the actual physical, contact is not necessary., At the time of collision, the two colliding, objects are deformed and may be, momentarily at rest with respect to each, other. If the initial and final velocities of both, the bodies are along the same straight line,, then it is called a one-dimensional collision or, head-on collision., m1, , m1, , m2, u1, , m2, , u2, B, , A, , B, A, During collision, , Before collision, u1> u2, m1, v1, , m2, , B, A, , After collision, v1 < v2, , v2, , 32. In elastic collision,, I. initial kinetic energy is equal to the, final kinetic energy., II. kinetic energy during the collision, time Dt is constant., Which of the following option is correct?, (a) Only I, (c) Both I and II, , (b) Only II, (d) None of these, , 33. A particle of mass m 1 moves with, velocity v 1 collides with another particle, at rest of equal mass. The velocity of, second particle after the elastic collision, is, (a) 2v 1, , (b) v 1, , (c) - v 1, , (d) zero, , 34. A molecule in a gas container hits a, , horizontal wall with speed 200 ms -1 at, an angle 30° with the normal and, rebounds with the same speed. Which, statement is correct?, (a), (b), (c), (d), , Momentum is conserved, Elastic collision, Inelastic collision, Both (a) and (b), , 35. A particle of mass 1g moving with a, , velocity v 1 = 3$i - 2$j ms -1 experiences, , a perfectly elastic collision with another, particle of mass 2 g and velocity, v 2 = 4 $j - 6 k$ ms -1 . The velocity of the, particle is, (a) 2.3 ms-1, (c) 9.2 ms-1, , (b) 4.6 ms-1, (d) 6 ms-1
Page 187 :
176, , CBSE New Pattern ~ Physics 11th (Term-I), , PRACTICE PAPER 2, OMRSHEET, Instructions, Use black or blue ball point pens and avoid gel pens and fountain, pens for filling the sheets, Darken the bubbles completely. Don't put a tick mark or a cross, mark half-filled or over-filled bubbles will not be read by the software., , ü, , û, , Incorrect, , Incorrect, , Incorrect, , Correct, , Do not write anything on the OMR Sheet, Multiple markings are invalid, 1, , a, , 19, , a, , d, , c, , b, , a, , 6, , b, , c, , b, , c, , 2, , a, , b, , c, , d, , 20, , a, , b, , c, , d, , 3, , a, , b, , d, , 21, , a, , b, , c, , d, , 4, , a, , c, , d, , 22, , a, , b, , c, , d, , 5, , b, , c, , d, , 23, , a, , b, , c, , d, , a, , b, , c, , d, , 24, , a, , b, , c, , d, , 7, , a, , b, , c, , d, , 25, , a, , b, , c, , d, , 8, , a, , b, , c, , d, , 26, , a, , b, , c, , d, , 9, , a, , b, , c, , d, , 27, , a, , b, , c, , d, , 10, , a, , b, , c, , d, , 28, , a, , b, , c, , d, , 11, , a, , b, , c, , d, , 29, , a, , b, , c, , d, , 12, , a, , b, , c, , d, , 30, , a, , b, , c, , d, , 13, , a, , b, , c, , d, , 31, , a, , b, , c, , d, , 14, , a, , b, , c, , d, , 32, , a, , b, , c, , d, , 15, , a, , b, , c, , d, , 33, , a, , b, , c, , d, , 16, , a, , b, , c, , d, , 34, , a, , b, , c, , d, , 17, , a, , b, , c, , d, , 35, , a, , b, , c, , d, , 18, , a, , b, , c, , d, , d, , Students should not write anything below this line, , SIGNATURE OF EXAMINER WITH DATE, , MARKS SCORED
Page 188 :
177, , Practice Paper 3, , PRACTICE PAPER 3, Physics Class 11th (Term I), Instructions, 1. This paper has 35 questions., 2. All questions are compulsory., 3. Each question carry 1 mark., 4. Answer the questions as per given instructions., Time : 90 Minutes, , Max. Marks : 35, , Multiple Choice Questions, , 5. Two solid spheres A and B are made, , 1. At a metro station, a girl walks up a, stationary escalator in time t 1 . If she, remains stationary on the escalator,, then the escalator take her up in time, t 2 . The time taken by her to walk up on, the moving escalator will be, (a) (t1 + t2 ) / 2, (c) t1 t2 / (t2 + t1 ), , (b) t1 t2 / (t2 - t1 ), (d) t1 - t2, , 2. Force of 4N is applied on a body of, mass 20 kg. The work done in 3 s is, (a) 3 J, , (b) 2 J, , (c) 4 J, , (d) 1 J, , 3. Weight of a body on surface of earth is, 63 N. When this object is taken below, earth surface at a height equals to half, the radius, its weight becomes, (a) 28 N, (c) 0, , (b) 10 N, (d) 63 N, , 4. The three initial and final positions of a, man on the X -axis are given as, (i) ( -8 m, 7 m), (ii) (7 m, - 3 m), (iii) ( -7 m, 3 m), Which pair gives the negative, displacement?, (a) (i), (c) (iii), , (b) (ii), (d) (i) and (iii), , of metals of different densities r A and, r B , respectively. If their masses are, equal,then the ratio of their moments, of inertia ( I B / I A ) about their, respective diameters is, ær ö, (a) ç B ÷, è rA ø, , 2 /3, , ær ö, (b) ç A ÷, è rB ø, , 2 /3, , (c), , rA, rB, , (d), , rB, rA, , 6. A body of mass 1 kg is moving in a, vertical circular path of radius 1 m. The, difference between the kinetic energies, at the highest and the lowest positions is, (a) 20 J, (c) 4 5 J, , (b) 10 J, (d) 10( 5 - 1) J, , 7. A particle moves along a circle of radius, r with constant tangential acceleration. If, the velocity of the particle isv at the end, of second revolution, after the revolution, has started, then the tangential, acceleration is, (a), , v2, 8pr, , (b), , v2, v2, (c), 6pr, 4pr, , (d), , v2, 2pr, , 8. If K i and K f are the initial and final, , values of kinetic energy of a body, respectively, then the work done by the, net force on the body is equal to, (a), , K fK i, Kf -Ki, , (b) K f + K i (c) K f - K i (d), , Kf + Ki, 2
Page 189 :
178, , CBSE New Pattern ~ Physics 11th (Term-I), , (a) A shows the kinetic energy, B shows the, total energy and C shows the potential, energy of the satellite., (b) A and B are the kinetic energy and potential, energy respectively and C is the total energy, of the satellite., (c) A and B are the potential energy and kinetic, energy respectively and C is the total energy, of the satellite., (d) C and A are the kinetic energy and potential, energy respectively and B is the total energy, of the satellite., , 9. Conservation of momentum in a, collision between particles can be, understood from, (a), (b), (c), (d), , law of conservation of energy, Newton’s first law only, Newton’s second law only, Both Newton’s second and third laws, , 10. Electromagnetic force is, (a) the force between charged particles, (b) charges in motion, (c) 1036 times the gravitational force between, two protons for any fixed distance, (d) All of the above, , 15. A projectile, thrown with velocity v 0 at, , 11. The respective number of significant, figures for numbers 23.023, 0.0003 and, . ´ 10 - 3 are, 21, (a) 4, 4, 2, (c) 5, 1, 2, , (b) 5, 1, 5, (d) 5, 5, 2, , 12. If the mass of the earth is doubled and, its radius halved, then new acceleration, due to the gravity g ¢ is, (a) g ¢ = 4 g, (c) g ¢ = g, , (b) g ¢ = 8 g, (d) g ¢ = 16 g, , inextensible string of length l is, suspended from a vertical support. The, bob rotates in a horizontal circle with, an angular speed w rads -1 about the, vertical support. About the point of, suspension ,, (a) angular momentum is conserved, (b) angular momentum changes in magnitude, but not in direction, (c) angular momentum changes in direction but, not in magnitude, (d) angular momentum changes both in, direction and magnitude, , variation of energy E, with the orbital radius r, of a satellite in a circular, motion. Select the, correct statement., , (a) v 0, , (b) v 0 sina, , (c) v 0 cos a, , (d), , 10 cm rolls down an inclined plane of, height 7 m. The velocity of its centre as, it reaches the ground level is, , 17. In a two dimensional motion,, instantaneous speed v 0 is a positive, constant. Then, which of the following, are necessarily true?, (a) The average velocity is not zero at any time., (b) Average acceleration must always vanish., (c) Displacements in equal time intervals are, equal., (d) Equal path lengths are traversed in equal, intervals., , radius 40 m completes half a revolution, in 40 s. Its average velocity is, (a) zero, (c) 4 p ms-1, , A, r, B, , (b) 10 ms -1, (d) 20 ms -1, , 18. A cyclist moving on a circular track of, , E, , C, , gR, 2, , 16. A solid sphere of mass 1 kg and radius, , (a) 7 ms -1, (c) 15 ms -1, , 13. A bob of mass m attached to an, , 14. Figure shows the, , an angle a to the horizontal, has a range, R. It will strike a vertical wall at a, distance R/2 from the point of, projection with a speed of, , (b) 2 ms -1, (d) 8 p ms-1, , 19. An explosion breaks a rock into three, parts in a horizontal plane. Two of them, go off at right angles to each other. The
Page 190 :
179, , Practice Paper 3, , first part of mass 1 kg moves with a, speed of 12 ms -1 and the second, part of mass 2 kg moves with, 8 ms -1 speed. If the third part flies off, with 4 ms -1 speed, then its mass is, (a) 3 kg, , (b) 5 kg, , (c) 7 kg, , (c) A is true but R is false., (d) A is false and R is also false., , 23. Assertion When the objects A and B, , (d) 17 kg, , 20. A and B are two inclined vectors and R, is their sum., Choose the correct figure for the given, description., P, , A, , (a) O, , B, , R=, , A+, A, , (c) O, , Q, P, B, , R=, , A+, , B, , A, , Q, P, , Reason The total energy of an isolated, system is not conserved., , B, , A+, , Q, , B, , divisions is equal to ……… main scale, divisions., (b) 9, (d) 0, , 22. In a rotating frame of reference, the, pseudo force is called ……… , when, applied for centripetal acceleration., (a), (b), (c), (d), , 25. Assertion An astronaut experiences, weightlessness in a space satellite., , Q, , 21. In vernier callipers, 10 vernier scale, (a) 10, (c) 11, , 24. Assertion In an elastic collision, between two bodies, the energy of each, body is conserved., , (d) O, R=, , B, , A+, , Reason When the objects A and B, move in opposite direction, then, relative velocity of object B w.r.t. object, A is v BA = v B - v A ., , B, , (b) O, R=, , B, , P, , A, , move in the same direction, then, relative velocity of object A w.r.t. object, B is v AB = v A - v B ., , gravitational force, centrifugal force, centripetal force, dynamic force, , Assertion-Reasoning MCQs, For question numbers 23 to 27, two, statements are given-one labelled, Assertion (A) and the other labelled, Reason (R). Select the correct answer to, these questions from the codes (a), (b), (c), and (d) are as given below, (a) Both A and R are true and R is the, correct explanation of A., (b) Both A and R are true but R is not the, correct explanation of A., , Reason When a body falls freely, it, does not experience gravity., , 26. Assertion Static friction acting on a, body is always greater than the kinetic, friction acting on this body., Reason Coefficient of static friction is, more than the coefficient of kinetic, friction., , 27. Assertion In projectile motion, if time, of flight is made two times, then, maximum height will become four, times., Reason T µ sin q and H µ sin 2 q,, where q is angle of projection., , Case Based MCQs, Direction Answer the questions from, 28-31 on the following case., Universal Law of Gravitation, In a school, teacher is explaining an, experiment to the students. He tells them, about the law of gravitation by taking two
Page 191 :
180, , CBSE New Pattern ~ Physics 11th (Term-I), , bodies having masses m 1 and m 2 , respectively., Both the bodies are mutually separated by a, distance r. The teacher explains that there is a, force of gravitational attraction between two, bodies. This force depends on both the, masses and also on the separation distance, between them., , 28. Two particles of masses m 1 and m 2 ,, , approach each other due to their, mutual gravitational attraction only., Then,, , about the dynamics of the tyre of a car. He, was curious to know the reason behind the, rotation of a tyre. He went to his teacher and, asked the same. Teacher explains him,, suppose there is a car moving with velocity v, in a straight line. The tyre of a car rotates with, an angular velocity w. Linear velocity depends, on the angular velocity of the tyre and on the, radius r of the tyre as shown in the figure, below, ω, , (a) acceleration of both the particles are equal, (b) acceleration of the particle of mass m1 is, directly proportional to m1, (c) acceleration of the particle of mass m1 is, directly proportional to m2, (d) acceleration of the particle of mass m1 is, inversely proportional to m1, , v, , r, , Tyre of a car, , 29. A body of mass M is divided into two, , parts m and M - m . The gravitational, m, is, force between them is maximum, if, M, (a) 1 : 1, , (b) 1 : 2, , (c) 1 : 3, , (d) 1 : 4, , 30. Apple falls towards the earth but the, earth does not move towards the apple, because, (a) acceleration is inversely proportional to, mass, so acceleration of earth is negligible, (b) only earth exerts force on apple, apple does, not exert force on earth, (c) apple experience greater force than the, earth, (d) only apple exerts force on earth, earth does, not exert force on apple, , 31. What is the mass of a body whose, , weight is 59 N? (Take, g = 9 .8 m/ s 2 ), (a) 5 kg, , (b) 9 kg, , (c) 6 kg, , (d) 50 kg, , Direction Answer the questions from, 32-35 on the following case., Angular Velocity and its Relation with, Linear Velocity, Shyam is playing football in his society and, suddenly a car passes near him. He thought, , 32. Angular speed of hour hand of a clock, (in degree per second) is, 1, 30, 1, (c), 120, (a), , 1, 60, 1, (d), 720, , (b), , 33. Given, w = 2k$ and r = 2$i + 2$j. Find the, linear velocity., (a) 4 $i + 4 $j, (c) - 4 $i + 4 $j, , (b) 4 $i - 4 $j, (d) - 4 $i - 4 $j, , 34. What is the value of linear velocity, if, , $, r = 3$i + 4 $j + 6 k$ and w = - 5$i + 3$j + 5k?, (a) -2 $i + 45 $j - 29 k$, (b) 2 $i - 45 $j + 29 k$, (c) 3$i - 29 $j + 45 k$, (d) 5 $i - 6 $j + 4 k$, , 35. The correct vector relation between, , linear velocity v and angular velocity w, in rigid body dynamics is, (a) w = v ´ r, (b) v = r ´ w, (c) v = w ´ r, (d) r = v ´ w, Here, r is the position vector.
Page 192 :
181, , Practice Paper 3, , PRACTICE PAPER 3, OMRSHEET, Instructions, Use black or blue ball point pens and avoid gel pens and fountain, pens for filling the sheets, Darken the bubbles completely. Don't put a tick mark or a cross, mark half-filled or over-filled bubbles will not be read by the software., , ü, , û, , Incorrect, , Incorrect, , Incorrect, , Correct, , Do not write anything on the OMR Sheet, Multiple markings are invalid, 1, , a, , 19, , a, , d, , c, , b, , a, , 6, , b, , c, , b, , c, , 2, , a, , b, , c, , d, , 20, , a, , b, , c, , d, , 3, , a, , b, , d, , 21, , a, , b, , c, , d, , 4, , a, , c, , d, , 22, , a, , b, , c, , d, , 5, , b, , c, , d, , 23, , a, , b, , c, , d, , a, , b, , c, , d, , 24, , a, , b, , c, , d, , 7, , a, , b, , c, , d, , 25, , a, , b, , c, , d, , 8, , a, , b, , c, , d, , 26, , a, , b, , c, , d, , 9, , a, , b, , c, , d, , 27, , a, , b, , c, , d, , 10, , a, , b, , c, , d, , 28, , a, , b, , c, , d, , 11, , a, , b, , c, , d, , 29, , a, , b, , c, , d, , 12, , a, , b, , c, , d, , 30, , a, , b, , c, , d, , 13, , a, , b, , c, , d, , 31, , a, , b, , c, , d, , 14, , a, , b, , c, , d, , 32, , a, , b, , c, , d, , 15, , a, , b, , c, , d, , 33, , a, , b, , c, , d, , 16, , a, , b, , c, , d, , 34, , a, , b, , c, , d, , 17, , a, , b, , c, , d, , 35, , a, , b, , c, , d, , 18, , a, , b, , c, , d, , d, , Students should not write anything below this line, , SIGNATURE OF EXAMINER WITH DATE, , MARKS SCORED
Page 193 :
182, , CBSE New Pattern ~ Physics 11th (Term-I), , ANSWERS, Practice Paper 1, 1. (d), 11. (c), 21. (a), , 2. (c), 12. (a), 22. (a), , 3. (d), 13. (a), 23. (a), , 4. (d), 14. (b), 24. (a), , 5. (b), 15. (c), 25. (a), , 31. (b), , 32. (d), , 33. (d), , 34. (b), , 35. (a), , 6. (c), 16. (d), 26. (c), , 7. (d), 17. (c), 27. (c), , 8. (b), 18. (d), 28. (a), , 9. (a), 19. (b), 29. (c), , 10. (d), 20. (c), 30. (b), , 6. (a), 16. (a), 26. (c), , 7. (b), 17. (c), 27. (a), , 8. (c), 18. (c), 28. (a), , 9. (b), 19. (a), 29. (c), , 10. (b), 20. (a), 30. (c), , 6. (a), 16. (b), 26. (a), , 7. (a), 17. (d), 27. (b), , 8. (c), 18. (b), 28. (c), , 9. (d), 19. (b), 29. (b), , 10. (a), 20. (d), 30. (a), , Practice Paper 2, 1. (d), 11. (c), 21. (a), , 2. (c), 12. (b), 22. (c), , 3. (a), 13. (a), 23. (a), , 4. (c), 14. (d), 24. (d), , 5. (d), 15. (c), 25. (c), , 31. (c), , 32. (a), , 33. (b), , 34. (d), , 35. (b), , Practice Paper 3, 1. (c), 11. (c), 21. (b), , 2. (b), 12. (b), 22. (b), , 3. (a), 13. (c), 23. (b), , 4. (b), 14. (b), 24. (d), , 5. (b), 15. (c), 25. (b), , 31. (c), , 32. (c), , 33. (c), , 34. (a), , 35. (c)