Page 1 :
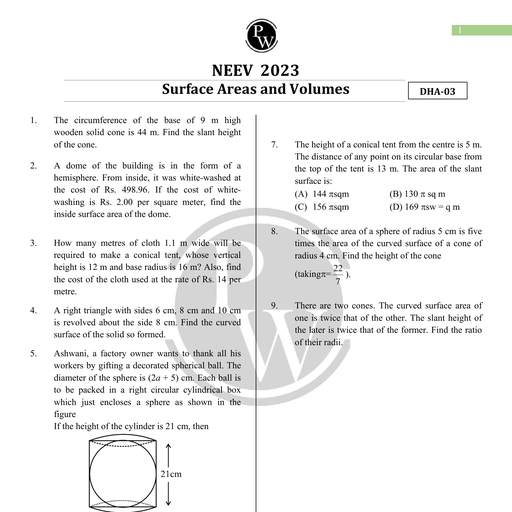
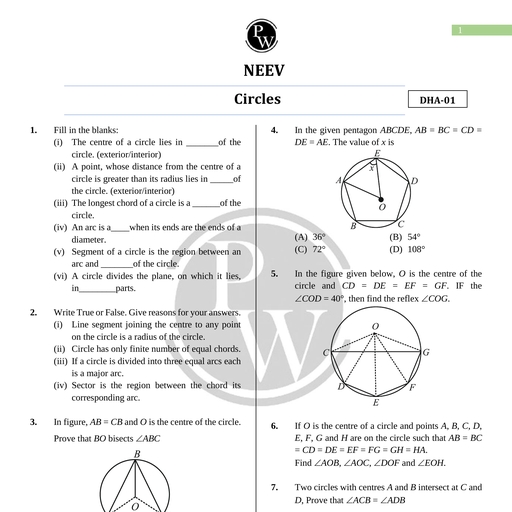
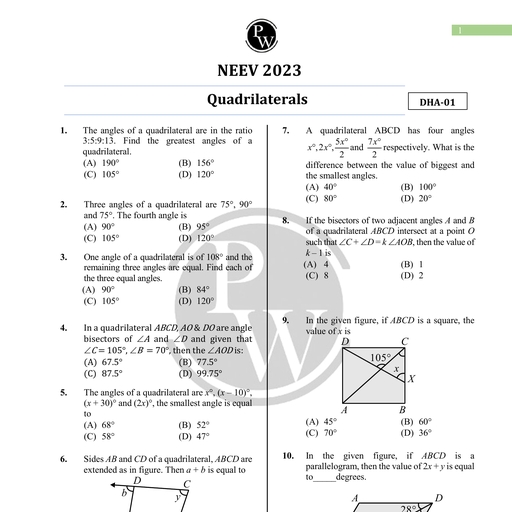
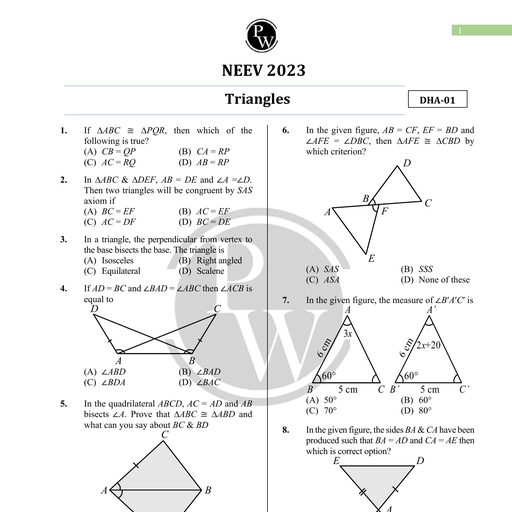
Chapter 8, , Gravitation, , e Introduction, e Kepler's Laws, , e Universal Law of, Gravitation, , e The Gravitational Constant, , © Acceleration Due to Gravity, of the Earth, , e Acceleration Due to Gravity, above the Surface of Earth, , e Acceleration Due to Gravity, below the Surface of Earth, , e Gravitational Potential, Energy, , © Escape Speed, © Earth Satellite, , © Energy of an Orbiting, Satellite, , e Geostationary and Polar, Satellites, , © Weightlessness, , e Some Important Definitions, © Formulae Chart, , e Quick Recap, , Introduction, , Have you ever wondered, why does anything thrown up falls down?, Why doesn’t the moon fall to the earth? Why doesn’t the earth fly off, into space rather than remaining in orbit around the sun? Why do, all the planets revolve around the sun? Why is the life of astronauts, inside an orbiting satellite very different? Why is going uphill lot more, tiring than going downhill? In all these cases, there must be some, force acting on the moon, the planets and the falling bodies. The, force acting on them is called Gravitational force. One of the long, standing goals of physics is to understand the gravitational force-the, force that holds you to the earth, holds all the objects surrounding, you to earth, holds the moon in orbit around earth, holds earth and, other planets in orbit around the sun. This force also reaches out, through our milky way galaxy, holding together billions of stars in, the galaxy and the countless molecules and particles between stars., , In this chapter we shall learn about the basic laws governing, gravitation and the motion of objects under the influence of, gravitation. We shall apply the law of gravitation to phenomena such, as the variation of weight with altitude. the orbits of satellites, around the earth and the orbits of planets around the sun., , Historical Introduction, , People have observed the movements of the moon, planets, stars, and other celestial bodies for thousands of years. The earliest, recorded model for planetary motion was ‘geocentric model’ which was, proposed and elaborated by the Greek astronomer Claudius Ptolemy, about 2000 years ago. He formalized that all celestial bodies, stars,, the sun and the planets all revolve around the earth which was, regarded as the centre of the universe. Ptolemy put a very, complicated scheme for describing the motion of celestial bodies. The, planets were described as moving in circles with the centre of the, circles themselves moving in a larger circle., , Aakash Educational Services Pvt. Ltd.-Regd. Office: Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph.011-47623456
Page 2 :
2 = ~Gravitation Board & Competitive Exams., , , , , , , , , , , , Fig.: Ptolemaic model of a planet revolving around the earth, , For about 1400 years, Ptolemaic Geocentric model was almost universally accepted until a Polish monk, astronomer Nicolas Copernicus (1473-1543) suggested and proposed that the earth and other planets, revolved in circular orbits around the sun which is popularly called the heliocentric model. His theory, was discredited by many people and by the church. Even Galileo who was one of the supporters of, Copernicus model, had to face prosecution from the state for his beliefs., , The Danish astronomer Tycho Brahe (1546-1601) wanted to determine how the heavens were, constructed. It is interesting to note that he spent his entire lifetime to note the observations of planets, and stars visible to the naked eyes only with a large sextant and a compass as the telescope had not, yet been invented. His compiled data were analysed later by his assistant Johannes Kepler (1571-1640), a German astronomer, whereupon he acquired his mentor’s astronomical data and spent around 16, years trying to deduce a mathematical model for the motion of planets. He could extract from the data, three elegant laws that now go by the name of Kepler's laws of planetary motion. These laws were, known to Newton, who provided the key that unlocked the secrets of the heavens and made a great, achievement in physical science by proposing the universal law of gravitation., , KEPLER’S LAWS, The three basic laws of planetary motion given by Kepler can be stated as follows:, 1. Law of orbits : All planets move in elliptical orbits, with the sun at one of the foci of the ellipse., , , , , , , , , , Fig.: An ellipse traced out by a planet around the sun. The closest, pointis P(Perihelion)and the farthest point is A (Aphelion)., , Note : Kepler's first law was a deviation from Copemican model which was applicable for only, , circular orbits., Ellipse can be drawn by a very simple method using 2 pins, string and a pencil. In this method, push, the pins into the paper at two points F, and F,. Tie a string at each end to the two pins. Stretch the, string taut with the tip of a pencil and then draw a curve by moving the pencil. The closed curve that, we get is called an ellipse. It is clear that the sum of the distance from any point at ellipse to F, and, F, is constant. F, and F, are called the Focii of the ellipse. Now, join F, and F, and extend it to intersect, the ellipse both sides at P and A as shown in the figure. The midpoint of line PA is known as the centre, of the ellipse O. Half of the length of PA is called semi-major axis of the ellipse., , Note : Circles are special cases of ellipse which is formed when the two foci merge into one and, semi-major axis becomes the radius of the circle., , Aakash Educational Services Pvt. Ltd.-Regd. Office: Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph.011-47623456
Page 3 :
Board & Competitive Exams. Gravitation 3, , 2., , Law of areas : The line that joins any planet to the sun sweeps out equal areas in equal, intervals of time., , It means that the area covered by the planet around the sun in given time intervals is constant i.e., areal, velocity is constant. This law comes from the observations that planets appear to move slower when they, are farther from the sun than when they are nearer., , , , Fig.: The plant P moves around the sun in an elliptical orbit. The, shaded area is the area AA swept out in a small interval of time At., , This law can be considered as a consequence of conservation of angular momentum which is valid for, any force that is always directed towards or away from a fixed point i.e., the central force. This area, swept out by the planet of mass m in the time interval Af is AA., , AA is given by AA= S/F xvat|, , , , where L =f x 6 = angular momentum, 6 = momentum and 7 is the position vector where sun is taken, as the origin., , The torque acting on a planet due to the central force is clearly zero; i.e., because Fis antiparallel to, hig, , Since, || =|FxF|=+F sino, , , , This implies that £ is constant as the planet goes around., , Hence, a is a constant., , Aakash Educational Services Pvt. Ltd.-Regd. Office: Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph.011-47623456
Page 4 :
4 ~~ Gravitation Board & Competitive Exams., , 3. Law of periods : The square of the time period of revolution of a planet is proportional to the, cube of the semi-major axis of the ellipse traced out by the planet., , T? « a, , where,, , T = Time period of revolution of a planet., , a = Semi-major axis of the elliptical orbit traced out by the planet., , Note : Shorter the orbit of the planet around the sun, shorter is the time to take one complete, revolution., , The graph between 7? and a? may be drawn as:, , Example 1: The distance of two planets from the sun are 10’? m and 10'? m respectively. What is the ratio, of their time periods?, , Solution : As T? « RS ...(By Kepler's third law), , , , Try Yourself, , 1. If the distance between earth and the sun were half of its present value, then how many number, of days will be there in a year?, Hint : use 7? < R® i.e., Kepler's third law., , 2. The time period of earth is taken as T and its distance from sun as R. What will be the distance, of a certain planet from sun whose time period is 64 times that of earth?, , Hint : By Kepler's third law., , , , , , , , Aakash Educational Services Pvt. Ltd.-Regd. Office: Aakash Tower, 8, Pusa Road, New Deihi-110005 Ph.011-47623456
Page 5 :
Board & Competitive Exams. Gravitation 5, , , , =, Example 2: A geostationary satellite is orbiting the earth at a height 6R above the surface of earth, where R, is radius of earth. What will be the time period of another satellite at a height 2.5 R from the, surface of earth?, Solution : As T2 « R8 ...(By Kepler's third law), 7, TR, , Try Yourself, , 3., , , , L= se (CT, = 24 hours), , , , The period of revolution of a satellite in an orbit of radius 2R is T. What will be its period of, revolution in an orbit of radius 8R?, , Hint : By Kepler's third law., , The radius of the nearly circular orbit of mercury is 5.8 =< 10’ m and its orbital period is, 88 days. If a hypothetical planet has an orbital period of 55 days, what is the radius of its, circular orbit?, , Hint : By Kepler's third law., , , , , , UNIVERSAL LAW OF GRAVITATION, , Historically it was the Italian astronomer and physicist Galileo Galilei (1564-1642) who recognized the fact, that all bodies, irrespective of their masses, are accelerated towards earth with a constant acceleration. He, made several experiments and public demonstration of this fact. But in 1665, the 23 year old Isaac Newton, made a basic contribution to physics when he showed that the force that holds the moon in its orbit is the, same force that makes an object to fall on earth. This assumption was made by Newton by observing an, apple falling from a tree. Newton concluded that not only earth attracts both apples and moon but also that, every particle in the universe attracts each other. This tendency of particles (or bodies) to move towards each, other is called gravitation. In formulating his law of gravity, Newton used the following reason, supporting, the assumption that the force of gravitation is proportional to the inverse square of the separation between, the two interacting bodies. He compared the acceleration of the moon in its orbit with the acceleration of an, object falling near the Earth's surface, such as the legendary apple., , Aakash Educational Services Pvt. Ltd.-Regd. Office: Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph.011-47623456