Page 1 :
Chapter 13, , Kinetic Theory, , ¢ Introduction Introduction, , Molecular Theory of Matter Gases have no shape and size and can be contained in vessels of, any shape and size. They have negligible force of molecular, , ¢ Behaviour of Gases interaction Le., the molecules of gases are further apart. So, gases, , © Kinetic Theory of an Ideal expands indefinitely and uniformly to fill the available space by, Gas considering that gases are made up of atomic particles, many, scientists like Boyle and Newton tried to explain the behaviour of, , © Law of Equipartition of gases. But the real theory was developed in the nineteenth century, Energy by Maxwell and Boltzmann. This theory is Kinetic theory. which, , © Specific Heat Capacity explains the behaviour of gases. It is consistent with gas laws and, Avogadro Hypothesis. It gives the interpretation of pressure and, ¢ Mean Free Path temperature of gases. It also explains the specific heat capacities of, Some Important Definitions gases correctly, and also relates the measurable properties of gases., Such as viscosity, conduction and diffusion, which we have, discussed in the previous chapters, with molecular parameters. In, Quick Recap this chapter we shall study some of the features of kinetic theory., , © Formulae Chart, , MOLECULAR THEORY OF MATTER, , John Dalton, about 200 years ago, proposed the atomic theory., According to this theory, , (a) The smallest constituents of an element are atoms., , (b) Atoms of one element are identical but differ from those of other, elements., , , , (c) A small number of atoms of each element combine to form a, molecule of a compound., , He suggested this theory to explain the laws of definite and multiple, Proportions, which are obeyed by the elements when they combine to, form compounds., , Aakash Educational Services Pvt. Ltd. Regd. Office : Aakash Tower, 8, Pusa Road, New Deihi-110005 Ph.011-47623456
Page 2 :
2 ‘Kinetic Theory Board & Competitive Exams., , , , According to the law of definite proportions, a given compound always contains same element in the same, proportion, irrespective of the source. Let us try to understand this with the help of an example, suppose we, take sample of water from two different sources. The molecular formula of water is H,O, it means it consists, of two hydrogen atoms and one oxygen atom and this will be same for both the samples. According to the, laws of multiple proportions if two elements can combine to form more than one compound, the masses of, one element that combine with a fixed mass of other element, are in the same ratio. For example, hydrogen, combine with oxygen to form two compounds; namely, water (HO) and hydrogen peroxide (H,O,)., , Hydrogen + Oxygen ———> Water, 29 16g 189, , Hydrogen + Oxygen ———> Hydrogen peroxide, 29 329 349g, , Here, the masses of oxygen (i.e., 16 g and 32 g), which combines with a fixed mass of hydrogen (2 g) bear, a simple ratio i.e., 16 : 32 or 1: 2., , Avogadro's hypothesis says that equal volumes of all gases at equal temperature and pressure have the same, number of molecules. When this hypothesis is combined with Dalton’s theory this explains Gay-Lussac’s law,, according to which when gases are combined or produced in a chemical reaction, they do so in a simple, ratio by volume, provided all gases are at the same temperature and pressure. Since the elements are often, in the form of molecules, Dalton’s atomic theory can also be referred to as the molecular theory of matter., , From many observations, in recent times we now know that molecules (made up of more than one atoms), constitute matter. We are able to measure their dimensions with the help of electron microscope and scanning, tunnelling microscope. The size of an atom is about an angstrom (10-19 m)., , In solids the atom is tightly packed (about 2 A). In liquids the atoms are not as rigidly fixed as in solids and, can move around, this enables a liquid to flow. In gases the interatomic distances are very large therefore,, the mean free path (the average distance a molecule can travel without colliding) is very large i.e., of the, order of thousands of angstroms. Hence, if the gases are not enclosed, they disperse away., , In solids as the atoms are closer therefore, they have more strong interatomic forces than gases. The, interatomic forces have long range attraction and small range repulsion i.e., the atoms attract each other when, they are few angstroms apart, but repel when they come closer., , Richard Feynman, one of the great physicists of 20th century considers the discovery that “Matter is made, up of atoms” to be very significant one. According to him if due to environmental disasters or nuclear, catastrophe. Humanity suffers annihilation and all scientific knowledge is going to destroy. Then he would like, the “Atomic hypothesis” to be communicated to the next generation of creatures in the universe. According, to which, all things are made up of atoms that moves around, they attract each other, when they are a little, distance apart. But repel each other when being squeezed into one another., , , , Knowledge Cloud, , Atomic Hypothesis in Ancient India and Greece, , Though John Dalton is credited with the introduction of atomic viewpoint in modern science, scholars in, ancient India and Greece conjectured long before the existence of atoms and molecules. Atoms were thought, to be eternal, indivisible, infinitesimal and ultimate parts of matter. It was argued that if matter could be, subdivided without an end, there would be no difference between a mustard seed and the Meru mountain., The four kinds of atoms (Paramanu — Sanskrit word for the smallest particle) postulated were (Earth),, , , , , , , , , , Aakash Educational Services Pvt. Ltd. Regd. Office : Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph.011-47623456
Page 3 :
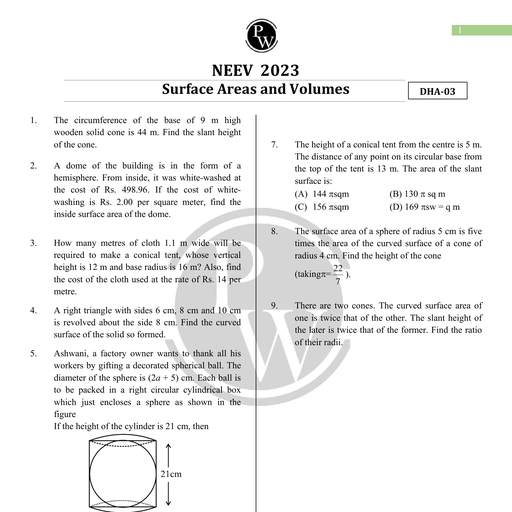
Board & Competitive Exams. Kinetic Theory 3, , , , Ap (water), Tejas (fire) and Vayu (air) that have characteristic mass and other attributes, were propounded., Akasa (space) was thought to have no atomic structure and was continuous and inert. Atoms combine to, form different molecules (e.g. two atoms combine to form a diatomic molecule dvyanuka, three atoms form, a tryanuka or a triatomic molecule), their properties depending upon the nature and ratio of the constituent, atoms, The size of the atoms was also estimated, by conjecture or by methods that are not known to us., , In ancient Greece, Democritus (Fourth century B.C.) is best known for his atomic hypothesis. The word ‘atom’, means ‘indivisible’ in Greek. According to him, atoms differ from each other physically. In shape, size and, other properties and this resulted in the different properties of the substances formed by their combination., The atoms of water were smooth and round and unable to ‘hook’ on to each other, which is why liquid/water, flows easily. The atoms of earth were rough and jagged, so they held together to form hard substances. The, atoms of fire were thomy which is why it caused painful burns. These fascinating ideas, despite their ingenuity,, could not evolve much further, perhaps because they were intuitive conjectures and speculations not tested, and modified by quantitative experiments - the hallmark of modem science., , , , , , , , =, , Example 1: State the law of multiple proportion with example., , Solution : According to the law of multiple proportion, if two elements can be combined to form more than, one compound the masses of one element that combine with a fixed mass of other element, are, in the same ratio. For e.g., hydrogen combines with oxygen to form two compounds; namely water, (H,O) and hydrogen peroxide (H,O,)., , Hydrogen + Oxygen ———> Water, 2g 16g 189, , Hydrogen + Oxygen ———» Hydrogen peroxide, 29g 32g 34g, , Here, the masses of oxygen (/.e., 16 g and 32 g), which combines with a fixed mass of hydrogen, (2g) bear a simple ratio i.e., 16 : 32 of 1: 2., , , , Try Yourself, , 1. State Avogadro's hypothesis., 2. State the law of definite proportion., , , , , , , , —, Example 2: Define mean free path., Solution : The average distance a molecule can travel without colliding., , , , Try Yourself, , 3., 4., , , , Write Dalton’s postulates of atomic theory., State Gay-Lussac’s law., , , , Aakash Educational Services Pvt. Ltd. Regd. Office : Aakash Tower, 8, Pusa Road, New Deihi-110005 Ph.011-47623456
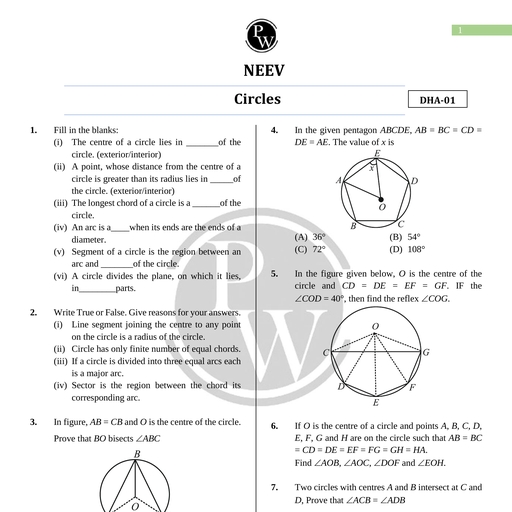
Page 4 :
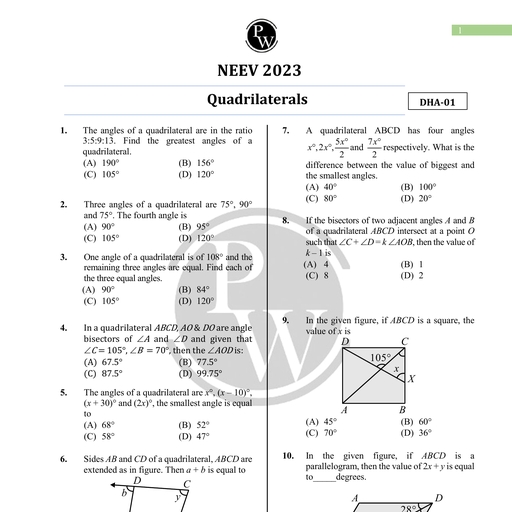
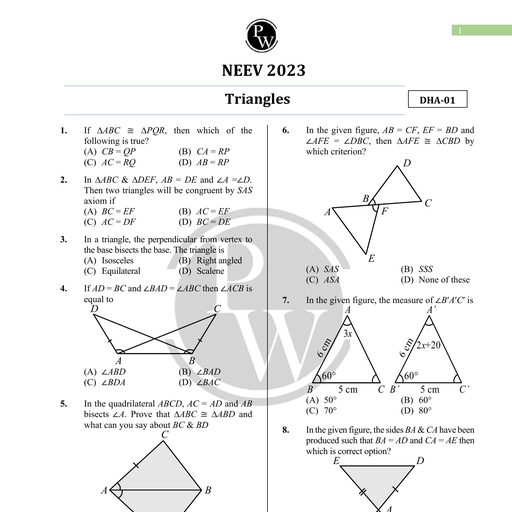
4, , Kinetic Theory Board & Competitive Exams., , Example 3: State Atomic hypothesis., Solution : According to atomic hypothesis all the things are made up of atoms, that move around, they, , attract each other, when they are at little distance apart but repel each other when being squeezed, one another., , , , , , 5. Explain the law of multiple proportion with the help of an example., 6. How does Dalton’s hypothesis explain the Gay-Lussac’s law?, , , , , , BEHAVIOUR OF GASES, , , , In gases molecules are far from each other and due to this the interatomic forces between the molecules is, negligible except, when two molecules collide. Hence, the properties of gases are easier to understand than, those of solids and liquids., , Gases satisfy a simple relation between pressure, temperature and volume at low pressure and high, temperature, this relation is given by equation, PV =KT, , for a given sample of the gas. K is constant, but it is proportional to the number of molecules, hence varies, with the volume of the gas if we take N molecules of a gas then K = Nk. Now k is independent of N hence, is same for all gases, and is called Boltzmann constant denoted by kg., , PM, _ PeVo, NT, Nee, , , , , , = constant = ky «-fi), , Avogadro’s Hypothesis, , If P, V and T are same for two gases then, N is also same i.e., at a fixed temperature and pressure the, number of molecules per unit volume is same for all gases., , The number of molecules in 22.4 litres of any gas at STP are 6.02 « 10°. This is known as Avogadro number, and is denoted by N,. The mass of 22.4 litres of any gas at S.T.P. (standard temperature 273 K and pressure, 1 atm) is equal to its molecular weight which is equal to one mole., , Note : Avogadro had guessed the equality of numbers in equal volume of gas at fixed temperature and, pressure from chemical reactions. Kinetic theory justifies this hypothesis., The perfect gas equation can be written as, PV =uRT -.(i), 1 is number of moles and R = N,k, which is universal gas constant. Temperature T is absolute temperature, R= 8314 J mort kK", , b=. = «+ (ii), , Aakash Educational Services Pvt. Ltd. Regd. Office : Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph.011-47623456
Page 5 :
Board & Competitive Exams. Kinetic Theory 5, , where M is the mass of the gas containing N molecules, M, is the molar mass and N, the Avogadro's number., Using equation (ii), equation (i) can be written as, , NxRxT, PS, R, , nil, N°, , PV = NkgT, , N, P = kpT = nkgT, vee 8, , where n is the number density of molecules i.e., number of molecules per unit volume, k, is Boltzmann's, constant. Its value in SI unit is 1.38 x 10-%9 JK-"., , We can also write equation (i) as, , , , -Hrr=-™ rr-2 rr [- M.o|, 7 MoV Mo Vv, where » is the mass density of the gas. If a gas satisfy equation (i) at all pressures at temperature then, it, is known as ideal gas. It is a theoretical model of a gas practically no gas is truly ideal. The figure given, below shows the departure of real gases from ideal behaviour. A straight line parallel to x-axis shows an ideal, gas. Curves of other gases approaches ideal gas behaviour at low pressure and high temperatures., , 1, Xe, z, =, , als, a} =, O}, , , , , , P (atm), , Fig.: Real gases approach ideal gas behaviour at low pressures and high temperatures., , Now, the question arises why real gases approaches ideal behaviour at low pressure and high temperatures., Let us try to seek for the answer to this question. At low pressures or high temperatures the molecules of, the gas are far apart and the interaction between the molecules becomes negligible, without interactions the, gas behaves like an ideal one., , Boyle’s law, , If » and T are fixed in equation (i) then, PV is constant. This gives Boyle's law. According to which keeping, temperature and number of moles of gas constant, the pressure of a given mass of a gas varies inversely, with volume., , 41, Pot, Vv, , PM, , , , V2, , Aakash Educational Services Pvt. Ltd. Regd. Office : Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph.011-47623456