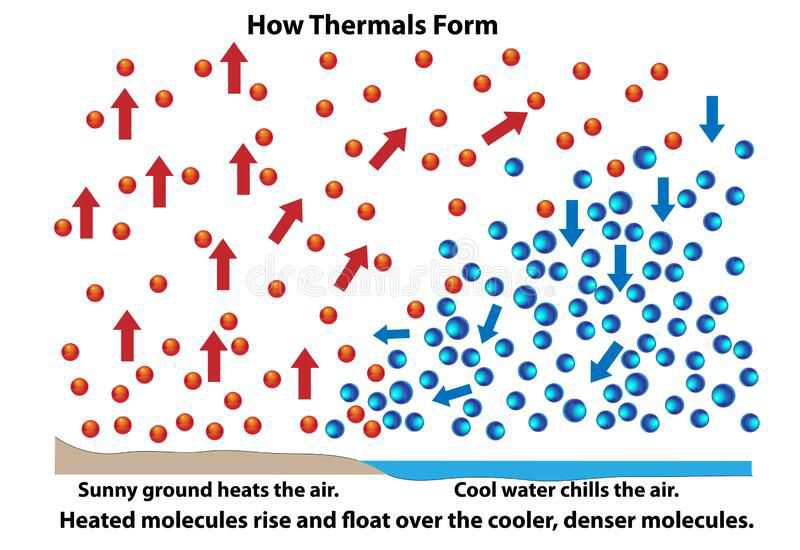
Page 5 :
MECHANICAL PROPERTIES OF FLUIDS, 10.41, Example 53. WVater flous through a horizontal pipe whose, internal diameter is 2.0 cm at a speed of 1.0 ms-', What, chuld be the diameter of the ozzle, if the water is to emerge, Total energy possessed by per kg of water, - 196 + 4.90+ 0.005 200.905 J., at a spend of 4.0 ms?, Here d, (Central Schools 04], Solution., r, =4 ms, d, = ?, Using equation of continuity,, =2 cm 002 m, , 1 ms-, Example 57, The reading of pressure meter attached with a, closed pipe is 3.5x 10 Nm2.On opening the vale of the pipe,, the reading of the pressure meter is reduced to 3.0 x 10° Nm., Calculate the speed of the water flowing in the pipe., Solution. Before opening the valve :, p, =3.5 x 10° Nm2, v, =0, x v, =, 4, or, After opening the valve:, 1, × (0.02)² = (0.01)?, 4, B = 3.0 x 10 Nm-2,, or, %3D, V2 = ?, In horizontal flow, P.E. remains unchanged. So, Bernoulli's theorem can be written as, or, d, = 0.01 m = 1.0 cm., Example 54. At what speed will the velocity head of stream, of water be 40 cm ?, Solution. Here h = 40 cm, g = 980 cms 2, 1, P2 +, 2, 1, pvý = P1 + pvi, +x 10° x v =35 x 10° +, 1, v, = 3.5 x 105 +, 2, 10 x (0)?, 3.0 x 105, Velocity head, h =, 28, 1, x 10° x v = (3.5 - 3.0) x 105 = 0.5 x 105, v =, 2 gh = 2 x 980 x 40 = 280 cms., :-, or, v, = 2 x 0.5 x 102 = 100, Example 55. At what speed will the velocity of a stream of or, water be equal to 20 cm of mercury column ? Take g = 10 ms2., Solution. Velocity head =20 cm of Hg, v2 = 10 ms-1., Example 58. A fully loaded Boeing aircraft 747 has a mass, 105, of 33 x, flight with a speed of 960 km/h. (a) Estimate the pressure, difference between the lower and upper surfaces of the wings., (b) Estimate the fractional increase in the speed of the air on, the upper surface of the wing relative to the lower surface., The density of air is p 1.2 kg m and g =9.81 ms2., kg. Its total wing area is 500 nt. It is in level, = 20 x 13.6 cm of water, But velocity head, 28, %3D, 20 x 136 =, 2 x 1000, [NCERT], Solution. (a) For the Boeing aircraft in level flight,, upward force due to the pressure difference =, of the aircraft, or, v = 20x 13.6x 2x 1000 =737.56 cms, 1, weight, = 7.3756 ms., Example 56. Calculate the total energy possessed by one kg or, of water at a point where the pressure is 20 gf I mm, velocity, is 0.1 ms and the height is 50 cm above the ground level., Apx A = mg, mg 3.3x 105 x 9.81, A =, A, or, %3D, %3D, 500, Solution. Here, = 6.47 x 10 Nm= 6.5 x 10' Nm-., 20, -2, P = 20 gf mm, x (10-)-2 kg f m', -2, (b) If v, and, the upper surfaces of the wings of the aircraft and p,, and p are the corresponding pressures, then from, Bernoulli's principle, we have, 1000, are the speeds of air on the lower and, = 20 x 10 x 9.8 Nm-2 = 19.6 x 10 Nm2, D = 0.1 ms, h = 50 cm =0.50 m,, p = 10' kg m, -3, 1, 1, 2, P 19.6 x 104, 103, Pressure energy per kg, = 196 J, %3D, or, %3D, Gravitational P.E. per kg = gh =9.8x 0.50= 4,90 J, V, + l'1, 1, 1, (0.1)?, 0.005 J, Ap =p, or, K.E. per kg =, v?, %3D, ('a – ?a) "a d= ('a - *a)