Page 1 :
Chapter 14, , Oscillations, , © Introduction Introduction, Periodic and Oscillatory When we look around us, we often find objects moving back and, ‘Motors forth repeatedly. Some oscillations are annoying, some are amusing, and some are dangerous. During an earthquake, buildings may be, © Period and Frequency set oscillating so strongly that they are shaken apart. When an, airplane is in flight. wings may oscillate due to turbulence of the, ° Displacement air, resulting in metal fatigue and even failure. When a tall building, * Simple Harmonic Motion sways slowly, we may not even notice it. But it becomes annoying, , if tt repeats more than 10 times/second. When you are standing, the, , e Simple Harmonic Motion and head tends to sway more than the feet, this sets off motion sensors, Uniform Circular Motion in the balancing region of the inner ear. Some mechanisms are used, . ey to decrease sway of tall buildings. e.g.. a large ball (5.4 x 10° kg), Velocity and Acceleration tn hangs on the 92™ floor of one of the worlds tallest buildings. How, , Simple Harmonic Motion the sway of the buildings is countered? (You will find the answer for, © Force Lain for siniple this in the section — Energy in simple harmonic motion)., Ferrero Motion Resonance appears to be one reason behind collapse of buildings. e.g.., , in 1985, buildings of intermediate height collapsed in Mexico city during, * Energy in Simple Harmonic an earthquake, while taller and shorter buildings remained standing., , Motion Aircraft designers ensure that none of the natural angular frequencies, at which a wing can oscillate matches the angular frequency of the, 2) Gane Sisters mein engine in flight (you will find reasons for these two in the section on, , Simple Harmonic Motion forced oscillations and resonance-chapter in daily life)., © Damped Simple Harmonic ‘The study and control of oscillations are two of the main goals of both, Motion Physics and Engineering. The back and forth motion of pistons in the, engines of a car is an example of motion that repeats itself, but it is, © Forced Oscillations and different from periodic motion of a planet around the sun. Here, the, Resonance object moves to and fro about a mean position. Such a motion is, , called ‘oscillatory’ or ‘vibratory motion’. Each atom/molecule of an, *) SChapieren Datyila/e object vibrates. We have sensation of sound due to vibration of air, Some Important Definitions molecules. We use the vibrations of electrons in the antennas of radio, and TV transmitters. There are oscillating quartz crystals in wrist, ° Formulae Chart watches, diaphragms in telephones, light, infrared rays, ultraviolet, Quick Recap rays, X-rays, y-rays etc. are also examples of oscillatory motion., In this chapter we shall study simple harmonic motion, periodic, motion of pendulum and spring mass system, damped and forced, oscillations and many more interesting topics., , Aakash Educational Services Pvt. Ltd. Regd. Office : Aakash Tower, 8, Pusa Road, New Deihi-110005 Ph.011-47623456

Page 2 :
52 Oscillations Board & Competitive Exams., pel, , , , PERIODIC AND OSCILLATORY MOTIONS, , , , Periodic Motion, , Consider motion of planets around the sun, rotation of earth about its polar axis, motion of moon around the, earth, motion of the hands of a clock, motion of Halley's comet around the sun, What is common to these, motions? Yes, you have guessed it correctly. The motion repeats itself after equal intervals of time. Periodic, motion is defined as that motion which repeats itself after equal intervals of time. The interval of, time is called the time period of periodic motion., , Let us consider another example — An insect climbing up a ramp, falling down, coming back to the initial, position and repeating the process identically. Let's plot the graph of its height about the ground versus time., It is shown in figure, , x), A, , VX, , —T— t, , It takes more time to climb up (shown by OA) and less time to fall down (shown by AB). After that, this, process is repeated, so time taken for this is time period of periodic motion indicated as T., , Let us consider another example of a ball bouncing off the ground between your palm and the ground. Let's, plot the graph of its height versus time. It is shown in figure. Both the curved path are sections of parabola, , given by equation of motion h=ut+3g¢? (downward motion) and h=ut-Lgt? (upward motion) with different, , values of u in each case., , af), , Ol, —T— t, , Oscillatory Motion, , Consider motion of the pendulum of a wall clock, motion of the bob of a simple pendulum displaced once, from its mean position and left to itself, the motion of a loaded spring, when the load attached to the spring, is pulled once a little from its mean position and left to itself, the motion of a liquid contained in U-tube when, it is compressed once in one limb and left to itself. Motion of a ball placed in a hemispherical bow! (it is in, equilibrium at the bottom), displaced a little from the point. What is common to these motions? Yes, in all, these cases, body moves to and fro or back and forth repeatedly about a fixed point (called mean position, or equilibrium position) in a definite interval of time. So, oscillatory or vibratory motion is defined as a, periodic and bounded motion of a body about a fixed point., , O is the equilibrium position for the ball placed in hemispherical bowl. When it is displaced to A, it moves, asO>A>5O>BO., , , , Aakash Educational Services Pvt. Ltd. Regd. Office : Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph.011-47623456

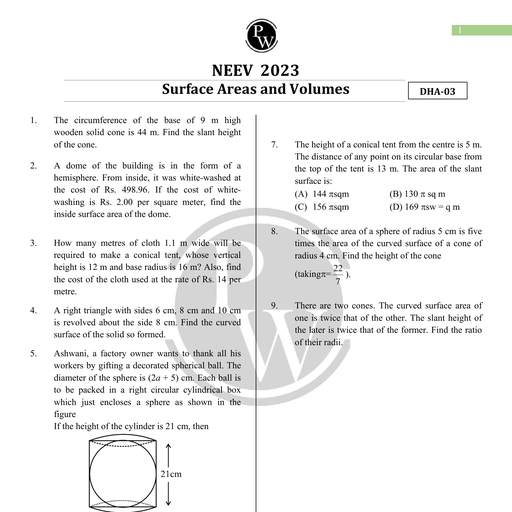
Page 3 :
Board & Competitive Exams. Oscillations 53, , In case of a simple pendulum, O is the equilibrium or mean position. As the bob is displaced a little to A,, it oscillates about O. Time taken by the bob from O — A — O -» B - Ois the time period of the oscillation., , , , Note : The body is confined within well defined limits (called extreme position) on either side of mean, position., , Difference between Periodic and Oscillatory Motion, , Every oscillatory motion is periodic, but every periodic motion need not be oscillatory. e.g., circular motion, (or the motion of planets around the sun) is a periodic motion, but it is not oscillatory because, the basic, concept of to and fro motion about the mean position for oscillatory motion is not present here., , Note : There is no significant difference between oscillations and vibrations. When the frequency is small,, we call it oscillation (like the oscillation of a branch of a tree), when the frequency is high (like the, vibration of a string of a musical instrument), we cail it vibration., , , , Try Yourself, , , , 1. Categorize the motion as periodic or oscillatory motion, (i) Motion of Halley’s comet around the sun., (ii) Motion of the pendulum of a wall clock., (ii) ce of liquid in a U-tube when liquid is once compressed in one limb and then left to, , , , Hint : In oscillatory motion, there is to and fro motion about some mean position., 2. Is circular motion an example of oscillatory motion?, , Hint : Think — is there to and fro motion about some mean position which is the basic concept, for oscillatory motion?, , , , , , PERIOD AND FREQUENCY, , Period is the smallest interval of time after which the motion is repeated. It is denoted by the symbol, T. Its SI unit is second. Other convenient units (for the motion which are either too fast or too slow) are, microsecond (10-® s) abbreviated as 1 s (for period of vibrations of a quartz crystal) and on the other hand,, the orbital period of the planet mercury is 88 earth days and that of the Halley's comet is 76 years., Frequency is defined as the number of oscillations per unit time. It is the reciprocal of time period T. It is, represented by the symbol v., , The relation between v and Tis, , , , v=, , -(1), , i, , Aakash Educational Services Pvt. Ltd. Regd. Office : Aakash Tower, 8, Pusa Road, New Deihi-110005 Ph.011-47623456

Page 4 :
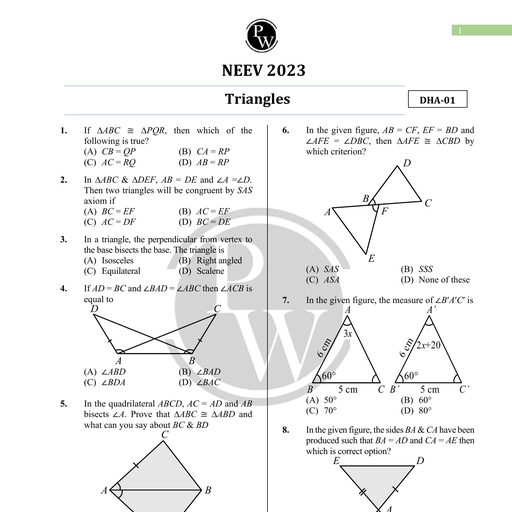
54 Oscillations Board & Competitive Exams., Its SI unit is hertz (abbreviated as Hz), named after Heinrich Rudolf Hertz (1857-1894), the discoverer of radio, waves., , 1 hertz = 1 Hz = 1 oscillation per second = 1s“? ...(2), , Note : Frequency, v is not necessarily an integer., , “ae Knowledge Cloud, , Harmonic oscillation is that oscillation which can be expressed in terms of single harmonic function, (i.e., sine function or cosine function). A harmonic oscillation of constant amplitude and of single frequency, is called simple harmonic motion. Simple harmonic oscillation is the simplest form of oscillatory motion., This motion arises when the force on the oscillating body is directly proportional to its displacement from, the mean position (or the equilibrium position). Further at any point, in its oscillation, this force is directed, towards the mean position., , Mathematically, a SHM can be expressed as, , , , , , x = Asinwt = Asin 2a «.(3), or x= Acosut = Acos =m +4), , Here, y = displacement of body from mean position at any instant t., A= maximum displacement or amplitude of oscillation., , @ = angular frequency (= 2nv) = (#), , v = frequency, T = time period, , Put t-2 in equation (3), n, y=Atin5 =a, , Put t=5 in equation (3), y = Asinn = 0, , Put = in equation (3), , 3a, = Asin =-,, y n> a, , Put t = T in equation (3), y = Asin2xn = 0, , , , , , , , Aakash Educational Services Pvt. Ltd. Regd. Office : Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph.011-47623456

Page 5 :
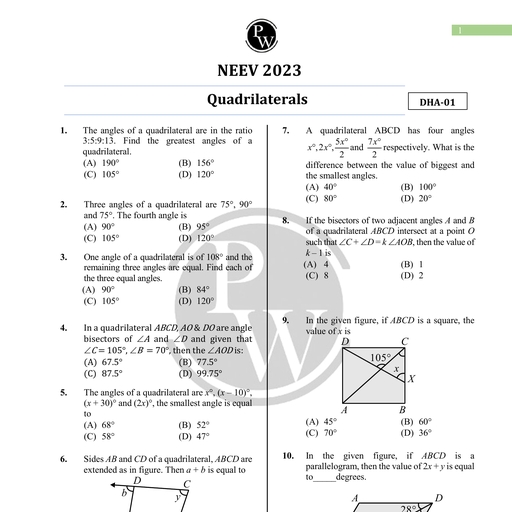
Board & Competitive Exams. Oscillations 55, , , , , , Taking points between 0 and a and, , , , or x=, , r é Z, 5 ana 37, 7 and 7 and joining all these points, you get the, , graph of figure-a. Do the same for equation (4) and you will get graph of figure-b., , , , , , , , Figure-b Figure-a, , Non-harmonic oscillation is that oscillation which cannot be expressed in terms of single harmonic function., It is a combination of two or more than two harmonic oscillations. Mathematically, it may be expressed as, , x = Asinwt + Bsin2wt, , -..(5), Asin 22 ¢ +Bsin aa, T ee, , Graphically, it can be represented by a curve of the type shown in given figure., , x, , NIM, , , , , , , , Example 1: Categorize the motion as periodic or oscillatory motion, @ Motion of planets around the sun, (ii) | A weighted test tube floating in a liquid pressed down and released, (iii) | Motion of hands of a clock, Solution : Periodic motion — (i), (ii) and (iii), Oscillatory motion — (ii), —, Example 2: A nurse in a hospital, noted for a patient that heart was beating 75 times a minute. Find its, frequency and time period., Solution : Number of oscillations (beats) in 60 s = 75, , Number of oscillations (beats) in 1s = 55 = 1.25, , Beat frequency of heart = 1.25 s~’ = 1.25 Hz, , 1__1 _ogs, , Time period, T =— 725, , , , Aakash Educational Services Pvt. Ltd. Regd. Office : Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph.011-47623456