Page 1 :
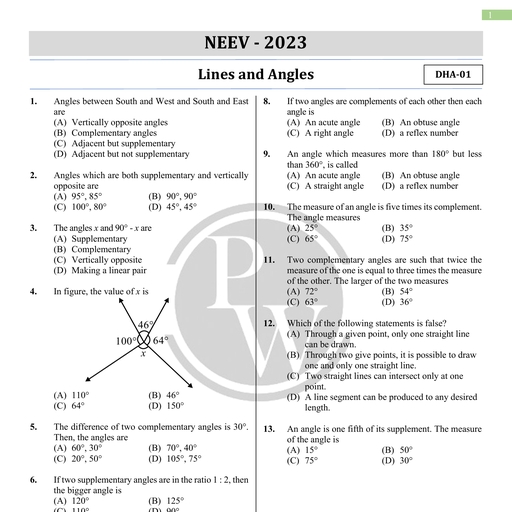
Chapter 12, , Thermodynamics, , Chapter Contents, , Introduction Introduction, The study of heat and its transformation to mechanical energy is called, Thermal Equilibri, ° um thermodynamics. It comes from a Greek word meaning “Movement of, © Zeroth Law of Heat”. The science of thermodynamics was developed in the early, Thermodynamics nineteenth century, before the atomic theory of matter was understood., , As earlier no one knew about electrons and other microscopic particles,, , © Heat, Internal Energy and so the workers in thermodynamics used the microscopic notions in, , Work: their models such as work, pressure and temperature., , e First Law of The foundation of thermodynamics is the conservation of energy and, Thermodynamics the fact that heat flows spontaneously from hot to cold body and not, , the other way around., , © Specific Heat Capacity ‘The difference between the mechanics and thermodynamics should, , © Thermodynamic State always be kept in our mind. In mechanics, our interest is in the motion, Variables and Equation of of particles or bodies under the action of forces and torques. While, State thermodynamics deal with the internal microscopic state of the body, , without considering with the motion of the system as a whole., © Thermodynamic Processes _n this chapter we shall study the laws of thermodynamics, various, , Engi thermodynamic processes, basic theory of heat engines, refrigerators, ©) Heat and Carnot engine., , e Refrigerators and Heat, , , , Pumps THERMAL EQUILIBRIUM, © Second Law of In mechanics the term ‘Equilibrium’ means that the net external force and, Tr i o torque on a system are zero. But in thermodynamics the term ‘equilibrium’, is quite different. To understand the term thermodynamic equilibrium let us, e Reversible and Irreversible first take few examples, when hot water is mixed with cold water, the final, Processes temperature of the mixture is somewhere between the initial hot and cold, temperatures. Similarly when a cube of ice is dropped in hot tea, the ice melts., © Carnot Engine and the temperature of tea decreases. In both of these examples when the, , temperature of the mixture becomes almost stable with the surrounding there, , © Some Important Definitions is no further exchange of energy. In general, whether or not a system is in, , e Formulae Chart a state of equilibrium depends on the surroundings and the nature of the wall, that separates the system from the surroundings., , © Quick Recap Let's consider two systems A and B, which are separated by an insulating, , wall i.e. a wall that does not allow the flow of energy also known as, adiabatic wall., , Aakash Educational Services Pvt. Ltd.-Regd. Office: Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph.011-47623456

Page 2 :
272 Thermodynamics Board & Competitive Exams., , , , These system are also insulated from rest of the surroundings by adiabatic walls as shown in the Fig.(a)., A thermometer is used to measure the temperature of A and B and are different initially. Now the adiabatic, wall between A and B is replaced by a conducting wall i.e., a wall that allows energy flow from one to another, also known as diathermic wall as shown in Fig. (b)., , Adiabatic, wall, , , , Fig. (a) Fig. (b), Fig.: (a) Both the systems separated by an adiabatic wall with each other and with, surroundings. (b) Both the systems soperae’ by diathermic wall with each, other and by adiabatic wall with surroundings., Now the thermometer records same temperature for both the systems. This concludes that there is no further, exchange of heat between them., , This state in thermodynamics is called thermal equilibrium. So we may say in thermal equilibrium, the, temperatures of the two systems are equal., , , , Example 1: When are the two bodies in thermal equilibrium?, , Solution : The two bodies are in thermal equilibrium if there is no exchange of heat between them., , , , What is the essential condition for thermodynamic equilibrium?, , 2. If a body A is in thermal equilibrium with three different bodies B, C and D then, (1) Bis in thermal equilibrium with C (2) Cis in thermal equilibrium with D, (3) Dis in thermal equilibrium with B (4) All of these, , , , , , ZEROTH LAW OF THERMODYNAMICS, , We have seen that through adiabatic wall heat cannot be transferred while through conducting wall heat can, be transferred easily. To understand what zeroth law of thermodynamics actually states, let us imagine a, situation where two systems A and B are separated by an adiabatic wall, while both of these systems are, further in contact with a third systems C by a conducting wall. Now the states of the systems (i.e., their, macroscopic variables) will change until both the systems A and B come in thermal equilibrium with the third, system C. After the thermal equilibrium is achieved if the adiabatic wall between A and B is replaced by a, conducting wall while the conducting wall between the system C and both the systems A and 8 are replaced, by adiabatic wall. It is found that there is no further change in the states of the system. In simple language, ‘we may say that when any two systems are in thermal equilibrium with each other, there must be a physical, quantity that has the same value for both. This physical quantity (/.e., a thermodynamic variable) whose value, is equal for two systems in thermal equilibrium is called temperature (7)., , , , , , Aakash Educational Services Pvt. Ltd.-Regd. Office: Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph.011-47623456

Page 3 :
Board & Competitive Exams. Thermodynamics 273, , , , Thus “when two bodies are in thermal equilibrium, their temperatures are equal and vice versa.”, , Note : System in thermal equilibrium must have same temperature. This is a necessary condition for, systems to be in thermal equilibrium, , The above discussed experimental facts is concluded in zeroth law of thermodynamics which states that “If, two systems are in thermal equilibrium with a third system separately are in thermal equilibrium, with each other.”, , Note : Two or more systems are said to be in thermal equilibrium, if there is no exchange of heat energy, between them when they are brought in thermal contact., , This law came into light in 1931 by R. H. Fowler long after the first and second law of thermodynamics had, been discovered and numbered. As the concept of temperature is fundamental to those two laws, the law, that establishes temperature as a valid concept should have the lowest number. Hence the above stated law, is numbered as zeroth law of thermodynamics., , HEAT, INTERNAL ENERGY AND WORK, , The concept of temperature arised from the zeroth law of thermodynamics. Temperature determines the, direction of flow of heat when two bodies are placed in thermal contact. Heat is that form of energy which, gets transferred between a system and its surrounding because of temperature difference between them. Heat, flows from the body at a higher temperature to the body at lower temperature. The flow stops when the, temperature equalises. i.e., the two bodies are then in thermal equilibrium., , , , We shall now study the concept of internal energy and work, , Internal Energy : It is sum of the kinetic energies and potential energies of all the constituent molecules, of the system., , It is denoted by ‘U’. U depends only on the state of the system. It is a state variable which is independent, of the path taken to arrive at that state. If we neglect the small intermolecular forces in a gas, the internal, energy of the gas is just the sum of the kinetic energies associated with various random motions of its, molecules., , Note : intemal energy of a system does not depend on the motion of a system as a whole i.e., the sum, of the kinetic energy of only the constituent molecules due to their randomness inside the system is, considered., , , , , , , , , , , , , , , , , , (a) (b), , Fig.: (a) Internal energy U of a gas is the sum of the kinetic and potential energy of its molecules, when the box is at rest. Kinetic energy due to various types of motion (translational, rotational,, vibrational)is to be included in U. (b) If the same box is moving as a whole with some velocity,, the kinetic energy of the box is not to be included in U., , Aakash Educational Services Pvt. Ltd.-Regd. Office: Aakash Tower, 8, Pusa Road, New Dethi-110005 Ph.011-47623456

Page 4 :
274 Thermodynamics Board & Competitive Exams., , Work done by gas : When a piston of a cylinder is pushed down, the work done by the gas is taken to, be negative. Similarly when the gas expands the work done by the gas is taken to be positive., , Formula for work done is : w = P-av, , , , Fig.: Heat and work are two distinct modes of energy transfer to a system that results in change, in its internal energy (i) Heat is energy transfer due to temperature difference. (ii) Work is, energy transfer brought without the difference in temperature., , Note : Both heat and work are two different modes for the alteration of the state of a thermodynamic, system and changing its internal energy., , Remember that heat differs by internal energy as a thermodynamic system is characterised by its internal, energy, not heat. A statement like ‘a gas in a given state has a certain amount of heat.’ is as, meaningless as the statement that ‘a gas In a given state has a certain amount of work’. While a, statement that ‘a gas In a given state has a certain amount of inernal energy’ is a meaningful statement., Similarly we may say that ‘a certain amount of heat is supplied to the system’ or ‘a certain amount, of work was done by the system’ are meaningful., , =, , Example 2: The figure shows a P-V graph of the thermodynamic behaviour of an ideal gas. Find out from this, graph (i) work done by the gas in the process A — B, B +» C, C-» DandD > A, (ii) work done, by the gas in complete cycle A B+ C+D A., , , , , , , , , , 14, “E12 > 5, 210, ° y, mS, Cc, t 2 — &, , , , pp, 1.0 2.0 3.0 40 50 60 7.0, —~ V (Litre), , Aakash Educational Services Pvt. Ltd.-Regd. Office: Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph.011-47623456

Page 5 :
Board & Competitive Exams. Thermodynamics 275, , Solution : (i), , (ii), , , , The work done in a thermodynamic process is equal to the area enclosed between the, P-V curve and the volume-axis., , Work done by the gas in the process A > Bis, W, = area ABB’ A'= AB x AA, = (6.0 -1.0 ) litre x (12 * 105) N/m?, = 5.0 « 10° m3 x (12 = 105) N/m?, = 6000 N-m = 6000 joule., Work done in the process B — C is zero since volume remains constant., Work done on the gas in the process C > Dis, , w,, , /, = area DCB'A’, , = DC x A’ D = (-5.0 = 10°) = (2 « 105) = —1000 joule. (Negative sign is taken because, volume decreases), , Work done in the process D — A is also zero, because volume remains constant., , Work W, is positive, while W, is negative. Hence the net work done in the whole cycle is, W = W, — W, = 6000 — 1000 = 5000 joule, , This net work is done by the gas., , , , =, Example 3: Consider the process on a system shown in figure below. During the process, the work done by, , the system., Ae ge 2, Volume, , (1) Continuously increases, , (2) Continuously decreases, , (3) First increases then decreases, (4) ‘First decreases then increases, , Solution : Don't get tempted to mark the answer (3). We said that the area under the p-V diagram is equal, , to the work done. So, if we move from 1 to 2 the area under the graph is continuously increasing, so the correct response will be (1)., , Note : In this question the rate with which the work is being done by the system is first, decreasing then increasing., , , , , , , , Aakash Educational Services Pvt. Ltd.-Regd. Office: Aakash Tower, 8, Pusa Road, New Dethi-110005 Ph.011-47623456