Page 1 :
Chapter 6, , Work, Energy and Power, , @ Introduction, @ Work, , ¢ Kinetic Energy, , Work Done by a Variable, Force, , ¢ Notion of Work and Kinetic, Energy : The Work-Energy, Theorem, , The Work-Energy Theorem, Jor a Variable Force, , ¢ The Concept of Potential, Energy, , ¢ The Conservation of, Mechanical Energy, , e The Potential Energy of a, Spring, , e Various forms of Energy:, , The Law of Conservation of, , Energy, ¢ Power, ¢ Collisions, ¢ Some Important Definitions, e Formulae Chart, Quick Recap, , Chapter Contents, , Introduction, , Do you often ask, “why should I study Physics”? What has this got to, do with my life? Well, you might be the first person to invent a car that, runs on waste or nuclear fuel or a space ship that is faster than speed, of light ... or for that matter a person who comes out with a solution to, technical problems. You can reason from basic concepts all the way to, valid conclusions about the reality around you, and this understanding, gives the fun. Physics is also the foundation of all engineering and, technology. Could any engineer design a space ship, an LED TV or a, mobile phone which all of you use without first understanding the, basic laws of physics? It is one of the fundamental goals of physics to, understand the terms ‘work’, ‘energy’ and ‘power’. The topics are indeed, important. Success of a civilization depends on acquiring and efficiently, ‘using’ energy. We talk about work done by a machine and its power., In physics, these terms have different meaning. A little challenge for, the inquisitive brains. Assuming that a racing car does not lose, traction, the time it takes to race from rest through a distance s depends, mainly on engine's power P. Derive the time ¢ in terms of s and P, assuming that the power is constant. You will be able to answer this, after studying this chapter. Answer to this is given in one of the solved, examples. You may compare your answer with our solution. Our aim, is to understand these three physical quantities ‘work’, ‘energy’ and, ‘power’, But we need to understand a mathematical concept, namely, the scalar product of two vectors before proceeding to understand this, chapter. In this chapter, we shall study notion of work and kinetic, energy. the work-energy theorem, work, kinetic energy, work done by, a variable force, the work-energy theorem for a variable force, the, concept of potential energy, the conservation of mechanical energy,, motion in a vertical circle, the potential energy of a spring, various, forms of energy, the law of conservation of energy, power, collisions, (elastic and inelastic in one and two dimensions)., , Aakash Educational Services Pvt. Ltd.-Regd. Office: Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph.011-47623456
Page 2 :
102 Work. Energy and Power Board & Competitive Exams., , The Scalar Product, There are two ways of multiplying vectors (i) scalar product (ii) vector product. We shall study the latter in, next chapter. The scalar product or dot product of any two vectors A and B, denoted as A.B (read as, A dot B) is defined as, , A-B = ABcoso =), , where A and B are magnitudes of vectors A and B respectively and 0 is the smaller angle between them., , Dot product is called scalar product as A, B and cos@ are scalars. Both vectors have a direction but their, scalar product does not have a direction., , Geometrical interpretation:, R, , , , i, i, c, ; °o, 7 A Q %-Bcosd [ Q, (a) (b) (c), Fig.:1, Let AandB be represented by OG and OR respectively. Let ~QOR be 0. In figure (b) draw RS 1 OQ., OS = Bcos0 is the projection of B onto A. In figure (c), draw QS 1 OR, OS = Acos0 is the projection of A onto B., , A.B = ABcos® = A(Bcos@) = B(Acos 0), A-6 is the product of magnitude of A and component of & along A (or projection of B onto A)., Altematively, it is the product of magnitude of 8 and the component of A along 6 (or projection of A onto B )., , Properties :, (i) Dot product is commutative, ie, [A-B=B-Al ---(2), , A-B = ABcose, B-A=BAcoso, , A-B=B-A as ABcos0 = BAcos0, (i.e., dot product is commutative), (ii) Dot product is distributive, , ie, |A-(B+C)=A-B+A-C ---(3), , (iii) Dot product of a vector with itself gives square of its magnitude, ie. (4), , (vy) [A-(AB)=2(A-B) -.-(5), where A is a real number., , ™ [AA-(AB) = 27(A-B) -..(6), , where A is a real number., , Aakash Educational Services Pvt. Ltd.-Regd. Office: Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph.011-47623456
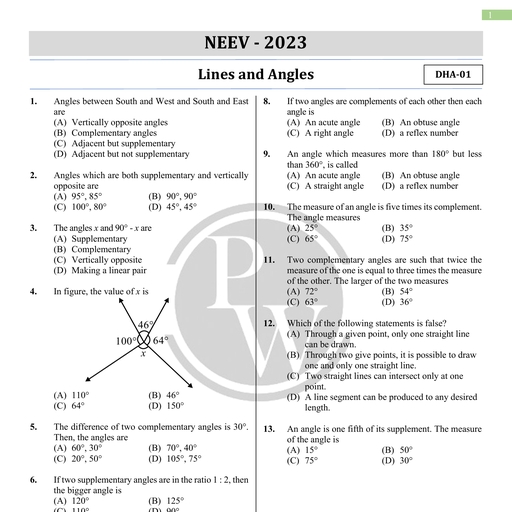
Page 3 :
Board & Competitive Exams. Work, Energy and Power 103., , Special cases :, Case-4: Two vectors are perpendicular (/.e., 0 = 90° also called orthogonal vectors), , A.B = ABcos90° =, For unit vectors 7 .j =7-k =k-i =0, Case-il: Two vectors are parallel (i.e., 0 = 0°), A.B = ABcos0 = AB(1) = AB, ‘or unit vectors, , n, , ieiej-jokeke1, Case-ill : Two vectors are antiparallel (i.e., 0 = 180°), , A.B = ABcos180 = —AB, As cos180 =—1, Understanding dot product in cartesian coordinates :, , Let A=A,i+A,j+A,K& B=B,7+B,j+B,K, A.B = (Aji +A,j+A,k)-(B,i + Byj + Bk), = A,i-(B,i + Bj +B,k)+ A,j -(B,i +B,]j + Bk) + A,k -(B,i + Byj + B,k), = A,B,(i-1)+ A,B, (7. j) + A,B, (i -k) + A,B) -1)+ A,B, (J -j)+ A,B, Gk) + A,B, (k-7) + A,B, (k -]), , +A,B,(k-k), = A,B,(1) + A,B,(0) + A,B,(0) + A,B,(0) + A,B(1) + A,B,(0) + A,B,(0) + A,B,{0) + A,B,(1)], , (G-i=]-j=k-k=1 and 7-j=j-k=k-i=0), = A,B, + A,B, + AB,, , a, = A-A=AA,+AA, +AA, , or AP = AZ + AS + AP ---(8), , As A-A=(A)(A)cos0 = A?, , , , Example 1: /f the magnitude of two vectors are 4 and 6 and the magnitude of their scalar product is 12/2,, what is the angle between the vectors?, , , , Solution : A=4,B=6,A.B=12/2, A.B = ABcos0, cose = AB _12v2 _ 1, , , , , , Aakash Educational Services Pvt. Ltd.-Regd. Office: Aakash Tower, 8, Pusa Road, New Dethi-110005 Ph.011-47623456
Page 4 :
104 Work, Energy and Power Board & Competitive Exams., , Example 2 :, , Solution :, , , , Find the angle between force F =(5i +4] +5k) unit and displacement d =(3i +4] -—3k) unit., , Also find the projection of F ond., F.d=F,d,+F,dy +F,d, = 5(3) + 4(4) + 5(-3) = 16 unit, FOP =F? =F2 +2 +F? =5? +4? +5? = 66 unit, , d.d =0? =d? +07 +0? = 3? + 4? +(-3)* = 34 unit, , F .d = Fdcoso, , Projection of F, , , , , , d =F cos0 = J66 x0.34 = 2.76, , Try Yourself, , a, , , , If the magnitudes of two vectors are 3 and 4 and magnitude of their scalar product is 6, what, is the angle between the vectors?, Hint: A=3,B=4 A.B=6, , A:B, cosé = —_—, AB, , If the magnitudes of two vectors are 2 and 3, and magnitude of their scalar product is 23,, what is the angle between the vectors?, Hint: A=2,B=3 A-B = 2/3, , A-B, , 80 = 5B, , Find the angle between the force fF =(5/+4j-3k) units and displacement, d =(3/] +4] +5k) unit. Also, find the projection of F on d., Hint: F-d =F,d, +F,d, +F,d,, , F-P=F? =F2 +3 +F? d-d=d? =d?+d}+d?, F-d, , cose = —_*, Fd, , Projection of F on d = F cos, Find the angle between the F =(4/ +3]) units and displacement d = (4j -3k)unit. Also find, the projection of F on d., , Hint: F-d =F,d, +F,d, +F,d,, , , , PP =F? =F2 +72 +F2 d-d=d? =d?+d2+d?, Fed —, cos0 = Projection of F ond = Fcos@, , , , Aakash Educational Services Pvt. Ltd.-Regd. Office: Aakash Tower, 8, Pusa Road, New Deihi-110005 Ph.011-47623456
Page 5 :
Board & Competitive Exams. Work, Energy and Power 105., WORK, , If a force F is applied on a body in a direction different from the direction of displacement of the body and, the body undergoes a displacement d in the positive X-direction as shown in figure 2., , ve, , ~~ gd ——_», Direction of motion —», Fig. :2, You may imagine a boy pulling a toy car with string, , 4, a, , , , , , , , , , , , , , , , <+— Direction of motion, Fig. :3, , The work done by the force is defined as the product of component of the force in the direction of the, displacement and the magnitude of this displacement., So, W =(Fcos0)d =F-d ---(9), , Try to understand the logic behind this — body is moving horizontally so, we need to take product of effective, component of force along the direction of motion (i.e., Fcos®) with the displacement to get work done, , (i.e., F(cos0)d or F-d). Force F and displacement d are vectors but work (W) is scalar as dot product, is a scalar ((Fcos@)d). No direction is associated with it. If force is applied along the direction of motion or, body moves along the direction of force i.e., 0 = 0., , W = (Fcos0)d = Fd As cos0° = 1 (This is what you studied in IX class)., In terms of rectangular components, F andd, are, FP =F,i+F,j] =F,k; d = xi + yj +zk, , , , , , , , , Eek, , , , , , fe} =, ann 5, , , , , , , , = Fig.:4, Imagine a point in the corner of a room and call it origin O. Now, imagine a point in space. Its distance from, x-axis, y-axis and z-axis (length, breadth and height of room) are F,, F, and F, respectively. Visualize a force, as a vector in three dimensions. F,, F, and F, are components of the force along x-axis, y-axis and z-axis, respectively. Now imagine that the body moves under the action of this force and now imagine a point in space, as the head of position vector d (final position of the body)., , d=d,i+d,j+a,k, , Aakash Educational Services Pvt. Ltd.-Regd. Office: Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph.011-47623456