Page 1 :
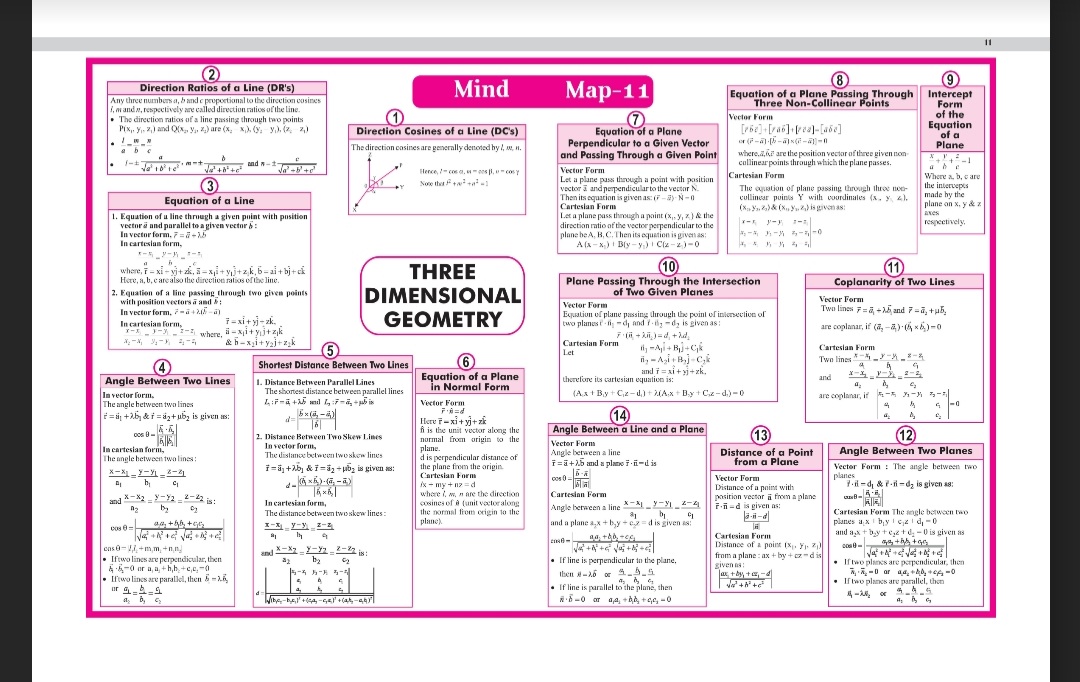
11, (2, Direction Ratios of a Line (DR's), Any three numbers a, b and e proportional to the dircction cosines, , mand, respectively are called direction ratios ofthe line., • The direction ratios of a line passing through two points, Pix,, Y, 2,) and Q(x, y ) are (x, ) (y, y) ( ), Mind, Мар-11, Equation of a Plane Passing Through Intercept, Form, of the, Equation, of a, Plane, Three Non-Collinear Points, Vecetor Form, (7, Direction Cosines of a Line (DC's), Equation of a Plane, or (-a) -s-a-n, where.aki are the position vectar of three given non, callinear points through which the plane passes., Perpendicular to a Given Vector, and Passing Through a Given Point, Vector Form, Let a plane pass through a point with pasition, vector i and perpendicularto the vectar N., Then its equarion is given as: (7-a)- N-0, Cartesian Form, Let a plane pass throuugh a paint (x,, y, z)& the, direction ratio of the vector perpendicular to the, plane be A, B, C. Then its equation is given as:, A (A - x,) Bly - y) C(z -z)-0, The direction casines are generally denoted hy, M., nd n-t, Herce, co, s ,cos Y, (3), Equation of a Line, Cartesian Form, The ouation of plane passing through throe neo-, cullinear points Y with vourdinales (x. y, a)., (Ku Yn a& (X, Y,isgivenas:, Where a b, e are, the intervepes, made hy the, plane on x. y &, axes, 1. Equation of u line thruugh a given puint with pusition, vector ä and parallel to a given vector :, In vector form. 7-5, In cartesian form,, -5 y- -, - y- 1-, - - --0, respectively, ,, 10, Plane Passing Through the Intersection, of Two Given Planes, where, F- xi - vj+ ai, -x,i + yj+z k, 6 - ai + bị + ck, Here, a, b, c arealso the direction ralios ofthe line., 2. Equation of a line passing through two given points, with position veetors a and, In vectur form, 7 -a +2 -a), In cartesian form,, Y-3- _ - where, i - xi+ yıj+zk, THREE, DIMENSIONAL, GEOMETRY, Coplanarity of Two Lines, Vector Form, Vector Form, Twa lines 7=a +5 and 7-, +ub,, Equation of plane passing through tbe point of iniersection of, two planes i- fi -d, and 7-g -dz is given as, are coplanar, if (a, -4) x6)-0, 7 i + ki, )d, + hd,, ti, -Ai + Bj+ Cé, i, -Azi + Bj-C,k, and i = xi + yj+ zk., & h-xi+ yaj+zk, Cartesian Form, Let, Cartesian Form, Twe lines *-y- -, Shortest Distance Between Two Lines, Angle Between Two Lines, Equation of a Plane, in Normal Form, therefore its cartesian equation is:, and, 1. Distance Between Parallel Lines, The shortest distance between parallel lines, (Ax + By + Cz-d)+2A,x + By + Cz-d.)-0, are coplanar, if -A - -, Invector form,, The angle hetween twa lines, Vector Form, 14, Angle Between a Line and a Plane, E=i +2b & i = iz+uby is given s:, Here F-xi+ yj+ zk, ĥ is the unit vector along the, nurmal from origin to the, plane., d is perpendicular distance of, the plane from the origin, Cartesian Form, ix - my + n2=d, where i, w, n are the direction, cosines of a (unit voctor along, the normal fiom crigin to the, plane)., d=, 13, 12, 2. Distance Retween Two Skew Lines, In vector form,, The distance between twoskew lines, Vector Form, In cartesian form., The angle hetween twa lines:, Angle between a line, i=ä+5 md a plane 7-i-d is, Distance of a Point, from a Plane, Angle Between Two Planes, i=ä, +b, & i=3z- ubz is given s:, Vector Form: The angle between two, Vector Form, Distance of a paint with, posirion vectar ä from a plane, Fi-d is given as:, 5-i-d, cos 0-, planes, 7-ii -d, & r-i -dz is given as:, Cartesian Form, and X-X2 --Y2-2 i, by, In cartesian form,, The distance between two skew lines:, Angle herween a line -Y- z-4, me a by, Cartesian Form The ngle botwoen two, planes ax b,yiczid, 0, and ax +by tezetd -0 is given as, and a plane a,x +bye d is given as:, vos, Cartesian Form, Distance of a point (x. Y 7), from a plane : ax + by + cz-dis, cos-, cas 0-+m, m+nn, • Iftwo lines are perpendicular, then, 45-0 or a, a+ h,h +c,e,-0, • Il'wo lines are parallel, then 5-2k, ur, and -X2--22-22, by, V4+4++, • Iftwo plancs are perpendicular, then, , A -0 or a4, +A0, + -0, • If two planes are parallel, then, 4-, o 4A4, is:, • If line is perpendiculur tn the plane,, -16 --, given s, ax +by, +,-4, -4 -A -, Iben -26 or 4..5, • If line is parallel to the plane, then, a b,