Page 1 :
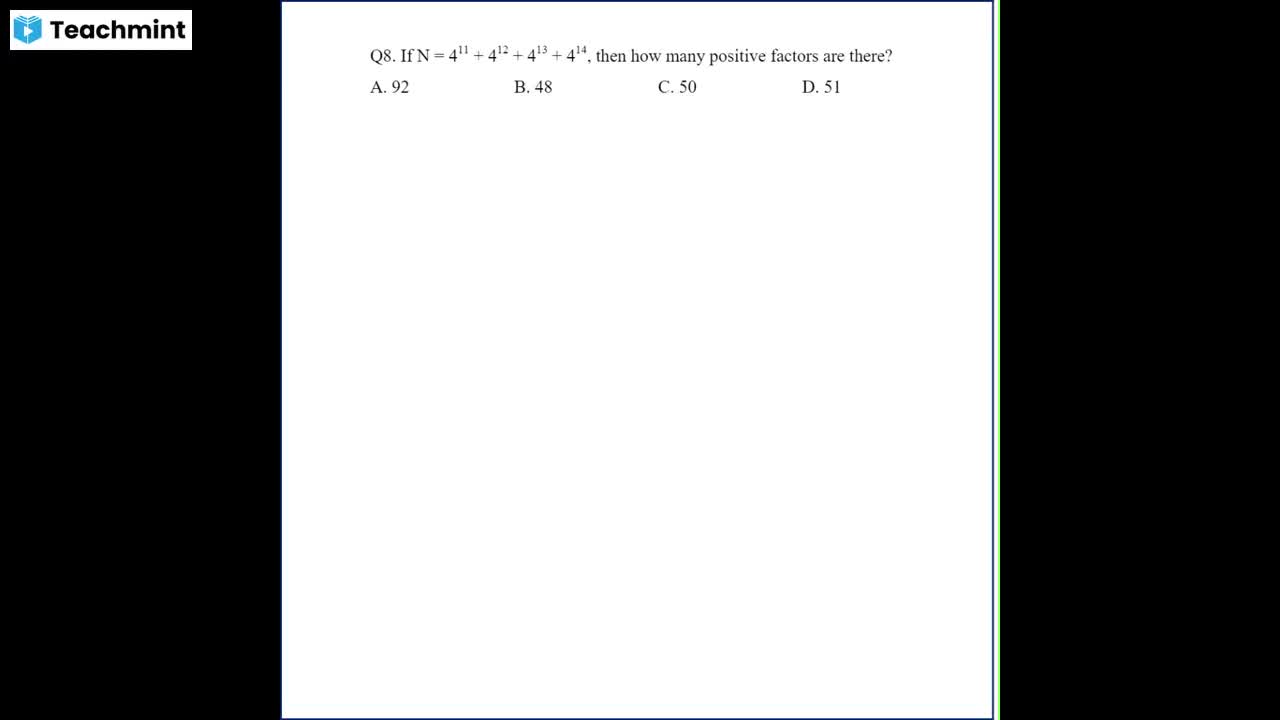
i repeating a three digit number; for, ; example, 235, 235 or 718, 718 etc. Any, i number of this form is always exactly, ; divisible by:, i (1) 8 only, (3) 12 only, , (2) 11 only, (4) 1001, , i i Q2. A number when divided by 6 leaves, i jremainder 4. When the square of the, ' same number is divided by 6, the, i remainder is:, , i qd)1 (2)2, , i Q3. If two numbers are each divided by, i the same divisor, the remainders are, i respectively 2 and 3. If the sum of the, two numbers be divided by the same, \ divisor, the remainder is 0. The divisor is, i()9 (2)7 (3) 5 (43, , i, 1 Q4. In a division sum, the divisor is 50, i times the quotient and remainder is 6, i times the quotient. If the dividend is, i 3248, then the remainder is, , 1(1)36 (2)56 (3) 136 (4) 156, , i Q5. A student was asked to divide a, ; number by 5 and add 21 to the quotient., iHe, however, first added 40 to the, ‘ number and then divided it by 5, getting, \ 120 as the answer. The correct answer, i should have been, , i) 131 (2) 135 (3) 133. (4) 137, , i 1 Q6. Which one of the following will, ; completely divide 5 BI 4 58 4 5839, , (1) 150 (2) 160 (3) 155 (4) 30, , i, , 3)4 (4)6, , \ Q7. How many numbers between 400, i and 950 are divisible by 4, 5 and 6?, iM7 @s8& G9 )10, , i, i Q8. Two numbers 12345 and 5819,, + when divided by a certain number of, , three digits, leaves the same remainder., The sum of digits of such a three-digit, number is, 8 9, , 3)10 4) 11, , Q9. If ‘n’ be any natural number, then by, which largest number (Ww =n’) is always, divisible?, (1) 13 (2) 26, , (3) 12 (4) 28, , Q10. A 4-digit number is formed by, repeating a 2-digit number such as 7979,, 4141, etc. Any number of this form is, always exactly divisible by:, , (d)7 (@)11, , (3) 13 (4) Smallest 3-digit prime number, , QI1. If the sum of the digits of any, integer lying between 100 and 400 is, subtracted from the number, the result, , always is, (1) divisible by 6 (2) divisible by 2, (3) divisible by9 (4) divisible by 5, , Q12. The maximum value of C in the, following equation 4A5 + 3B1 + 9C8 =, 1704 is (where A, B, C each stands for, any digit)., , ()8 (2)9, , Q13. The sum of four numbers is 72., When 4 and 3 are added to the first two;, and 2 and 5 are subtracted from the 3rd, and 4th, the numbers will be equal. The, numbers are, , (1) 14, 15, 20, 23, (3) 15, 20, 23, 14, , GB)7 )5, , (2) 15, 14, 23, 20, (4) 14, 20, 15, 23, , Q14. Ifa *b=a+b+ab, then the value, of 2 * 3 * 4 is:, ()50 =(2)51_—s (3) 58 (4) 59, , Q15. A number is doubled and 7 is, added. If the resultant is trebled, it, becomes 93. What is that number?, , (1) 12 (2) 21, , (3) 15 (4) None of these