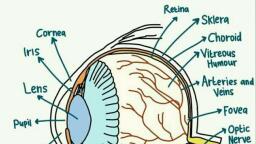
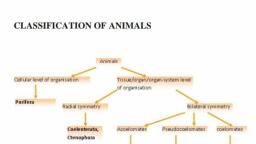
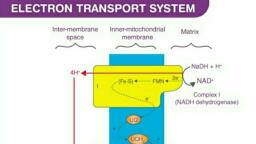
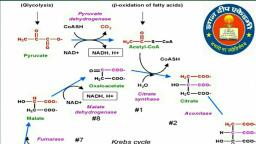
Page 1 :
ELECTROSTATICS, , ELECTRIC CHARGE, , Charge is the property associated with matter due to which it produces and experiences electrical and magnetic, effects. The excess or deficiency of electrons in a body gives the concept of charge., , Types of charge :, , (i) Positive charge : It is the deficiency of electrons as compared to proton., (i) Negative charge : It is the excess of electrons as compared to proton., , SI unit of charge : ampere second i.e. Coulomb Dimension : [A T], Practical units of charge are ampere _ hour (=3600 C) and faraday (= 96500 C), , Millikan calculated quanta of charge by ‘Highest common factor' (H.C.F.) method and it is equal to charge of, electron., , 1 C = 3 x 10? stat coulomb, 1 absolute - coulomb = 10 C, 1 Faraday = 96500 C., , SPECIFIC PROPERTIES OF CHARGE, , Charge is a scalar quantity : It represents excess or deficiency of electrons., , Charge is transferable : If a charged body is put in contact with an another body, then charge can be, transferred to another body., , Charge is always associated with mass, , Charge cannot exist without mass though mass can exist without charge., , . So the presence of charge itself is a convincing proof of existence.of mass., . In charging, the mass of a body changes., , . When body is given positive charge, its mass, decreases., , . When body is given negative charge, its mass increases., , Charge is quantised, , The quantization’ of electric charge is the property by virtue of which all free charges are integral multiple of a, basic unit of charge*fepresented by e. Thus charge q of a body is always given by, , q=ne n = positive integer or negative integer, The quantum of charge is the charge that an electron or proton carries., Note : Charge on a proton = (-) charge on an electron = 1.6 10-°'C, Charge is conserved, , In an isolated system, total charge does not change with time, though individual charge may change i.e. charge, can neither be created nor destroyed. Conservation of charge is also found to hold good in all types of reactions, either chemical (atomic) or nuclear. No exceptions to the rule have ever been found., , Charge is invariant, Charge is independent of frame of reference. i.e. charge on a body does not change whatever be its speed., , Accelerated charge radiates energy, , , , = = constant v# constant (i.e. time varying), v= 0 (i.e at rest) v z @, motte sie Cateih | pote, only E produces both E and, (dlectric field) (mregnetic field) and radiates energy, but no radiation, , , , , , , , , , , , Attraction - Repulsion, Similar charges repel each other while dissimilar attract
Page 2 :
METHODS OF CHARGING, . Friction: If we rub one body with other body, electrons are transferred from one body to the other., , Transfer of electrons takes places from lower work function body to higher work function body., , , , , , , , Positive charge Negative charge, , Gess rod Silkdoth, , Weollen doth Rubber shoes, Amber, Plastic objects, Dryhair Carb, , Rannel or cat skin Eboniterod, , Note : Gouds becomecharged by friction, , , , , , , , . Electrostatic induction, , If a charged body is brought near a metallic neutral body, the charged body will attract opposite charge and, repel similar charge present in the neutral body. As a result of this one side of the neutral body becomes, negative while the other positive, this process is called ‘electrostatic induction’., , + Charging a body by induction (in four successive steps), , , , , , daring =O charging d=ve charging q=ve q=ve, body ZO. body a A body, z ~, —a — Qn Ss @:, charged body is uncharged body is, uncharged body is cherging, brought near connected to disconnected body, uncharged body earth from the earth is removed, step-1 step-2 step-3, step4, , , , , , , , , , , , Some important facts associated with induction, (i) Inducing body neither gains nor loses charge, , (i) | The nature of induced charge is always opposite to that of inducing charge, , (iii) Induction takes place only in bodies (either conducting or non conducting) and not in particles., Conduction, , The process of transfer of charge by contact of two bodies is known as conduction. If a charged body is put in, , contact with uncharged body, the uncharged body becomes charged due to transfer of electrons from one body, to the other., , + The charged body loses some of its charge (which is equal to the charge gained by the uncharged body), + The charge gained by the uncharged body is always lesser than initial charge present on the charged body., + Flow of charge depends upon the potential difference of both bodies. [No potential difference => No conduction]., , + Positive charge flows from higher potential to lower potential, while negative charge flows from lower to, higher potential.
Page 3 :
open nev Poms, + Charge differs from mass in the following sense., , (i) In SI units, charge is a derived physical quantity while mass is fundamental quantity., , (ii) Charge is always conserved but mass is not (Note : Mass can be converted into energy E=mc?, , (ii) The quanta of charge is electronic charge while that of mass it is yet not clear., , (iv) For a moving charged body mass increases while charge remains constant., , + True test of electrification is repulsion and not attraction as attraction may also take place between a charged, and an uncharged body and also between two similarly charged bodies., , . For a non relativistic (i.e. v << c) charged particle, specific charge = =constant, , For a relativistic charged particle 4 decreases as v increases, where v is speed of charged body., m, , Example, , When a piece of polythene is rubbed with wool, a charge of - 2. 10-7 C is developed on polythene. What, is the amount of mass, which is transferred to polythene., , Solution, Q_ _2x1077, From Q = ne, So, the number of electrons transferred 1 = —~=. ~~ =. 25107, e 1.6107, , Now mass of transferred electrons = n _—mass»0f,oneselectton = 1.25 10! 9.1 10°74 = 1138 10°9 kg, , Example, , 10” a - particles«(Nuclei/of helium) per*second falls on a neutral sphere, calculate time in which sphere gets, charged by 2yuC., , Solution, Number of a - particles falling in t second = 10, Charge on @ - particle = +2e , So charge incident in time t = (10"t).(2e), , -18, , Given charge is 2 pC a 2 10¢ = (10)(2e) = t = 6.25s, , “16x10, , COULOMB'S LAW, , The electrostatic force of interaction between two static point electric charges is directly proportional to the, , product of the charges, inversely proportional to the square of the distance between them and acts along the, straight line joining the two charges., , If two points charges q, and q, separated by a distance r. Let F be the electrostatic force between these two, charges. According to Coulomb's law., , Pieg, quand Pat o> Oz O>-+0, , 2 Fram Qh q Tim? Fee Fiz, , , , , , , , ke 1 Nm?, F- where =9x10°—S, , = - 5, x 3 ane, c }. coulomb's constant or electrostatic force constant
Page 4 :
Coulomb's law in vector form, , , , , , > k E, F,, F,, = force on q, due to q, = aa thy ——__+_, r nh Ty &, > Kade . =, Fy 7 2 2 fz (here ij, is unit vector from q, to q, ), Coulomb's law in terms of position vector y qn Ce, a, =f kqigg = i, ., F, “> <p -®) / *, li -2|, , Principle of superposition, , The force is a two body interaction, i.e., electrical force between two point charges is independent of presence, or absence of other charges and so the principle of superposition is valid, i.e., force on a charged particle due, , to number of point charges is the resultant of forces due to individual point charges, i.e., F, = F,,+F,,+--, Note : Nuclear force is many body interaction, so principle of superposition is not valid in case of nuclear force., , When a number of charges are interacting, the total force on a_ given charge is vector sum of the forces exerted, on it by all other charges individually, , , , kqoa: , kq09 koa kaodn 2 “gq, 2, Fe te tt, a in vector form F =kqq yay,, q t) tr T, =n Ny, , SOME IMPORTANT POINTS REGARDING .COULOMB'S LAW AND ELECTRIC FORCE, , The law is based on physical observations and is not:logically derivable from any other concept. Experiments till, today reveal its universal nature., , The law is analogous’ to Newton’s law of gravitation : F = G a with the difference that :, , (a) Electric force between charged particles is much stronger than gravitational force, i.e., F, >>F,. This is, why when both F, and F, are present, we neglect F,., , (b) Electric force can be attractive or repukive while gravitational force is always attractive., (c) Electric force depends on the nature of medium between the charges while gravitational force does not., , (d) The force is an action-reaction pair, i.e., the force which one charge exerts on the other is equal and, opposite to the force which the other charge exerts on the first., , The force is conservative, i.e., work done in moving a point charge once round a closed path under the action, , of Coulomb's force is zero., , The net Coulomb's force on two charged particles in free space and in a medium filled upto infinity are, CA 1 a9 FOE, , py aM P = ge op SR, , , , F=, , , , , , 4ne,, , Dielectric constant (K) of a medium is numerically equal to the ratio of the force on two point charges in free, space to that in the medium filled upto infinity., , The law expresses the force between two point charges at rest. In applying it to the case of extended bodies of, finite size care should be taken in assuming the whole charge of a body to be concentrated at its ‘centre’ as this, is true only for spherical charged body, that too for external point., , Although net electric force on both particles change in the presence of dielectric but force due to, one charge particle on another charge particle does not depend on the medium between them., , Electric force between two charges does not depend on neighbouring charges.
Page 5 :
Example, , If the distance between two equal point charges is doubled and their individual charges are also doubled,, what would happen to the force between them?, , , , , , , , Solution, 1 1 (2q)(2q) 1 4q? 1 @, _ axq - et or i= a1 >Fo 4ne, 2 veel) Again, F' 4ne, (2rF or F ane, ar? ane, 1? F, , So, the force will remain the same., , Example, , A particle of mass m carrying charge tq), , is revolving around a fixed charge ‘-q,' in a circular path of, radius r. Calculate the period of revolution., , , , Solution, age | 4n*mr, 4n€9 2 = mra* = 72, , (4ney)t?(4n2mr) negmr, 2g AA AEOR AER TM) =, T i or T = 4nr a:92, , where Ff is the vector drawn from source charge is test charge., , , , Example, , The force of repulsion between two point charges is F,, , charges are replaced by spheres of radii 25 cm havi, between their centres is 1 m,, , Solution, , In 2™ case due to mutual repulsion,, , when these arevat a distance of 1 m. Now the point, , ing the'charge same as that of point charges. The distance, then compare the force’of'¥epulsion in two cases., , the effective distance between their centre of charges will be increased, , 1, (d' > d) so force of répulsion decreases as F oe, , , , EQUILIBRIUM OF CHARGED PARTICLES, , In equilibrium net electric force on eve:, , ry charged particle is zero. The equilibrium of a charged particle, under, the action of Colombian forces alone, , can never be stable., © Equilibrium of three point charges, , tae Ke, ———— ___., () Two charges must be of like nature asF, = See 0 Q q Q, x r-x ttn pe, , K Ki, (i) Third charge should be of unlike nature as Fo = KQiQ , KQia _ 9, x, , Q iy, Therefore x = 1 Q1Qe, , VQ, + Ja" a ae