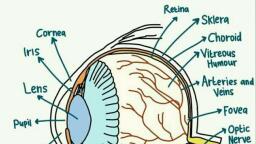
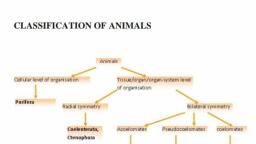
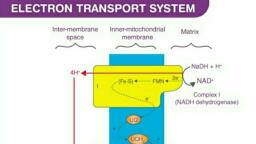
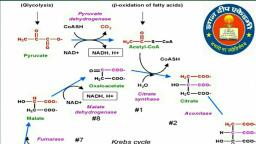
Page 1 :
44 Units, Dimensions and Measurement, 11., , SI unit of pressure is, [EAMCET 1980; DPMT 1984; CBSE PMT 1988;, NCERT 1976; AFMC 1991; USSR MEE 1991], , Units, 12., 1., , (b), , (c), , (d) Atmosphere, , The unit of angular acceleration in the SI system is, [SCRA 1980; EAMCET 1981], , 3., , (a) Time, (b) Mass, (c) Distance, (d) Energy, The magnitude of any physical quantity, (a) Depends on the method of measurement, (b) Does not depend on the method of measurement, (c) Is more in SI system than in CGS system, (d) Directly proportional to the fundamental units of mass, length, and time, Which of the following is not equal to watt, , 4., , (a) Joule/second, (c) (Ampere)  ohm, Newton–second is the unit of, , [SCRA 1991; CPMT 1990], , 2, , Stress, (a),  N /m 2, Strain, , (b) Surface tension = N/m, , (c) Energy  kg-m/sec, , (d) Pressure  N /m, , [MNR 1986], , 14., , 15., , (d) 9192631770 time periods of Cs clock, One nanometre is equal to, 9, , (a) 10 mm, , (b) 10, , 7, , 8., , The unit of Stefan's constant  is, , (a), , W m 2 K 1, , (b) W m 2 K 4, , (c), , W m 2 K 4, , (d) W m 2 K 4, , Which of the following is not a unit of energy, (a), , W- s, , (b) kg - m /sec, , (c), , N- m, , (d) Joule, , In S  a  bt  ct 2 . S, The unit of c is, , (c) 1 micron  10 cm, , ms 1, , 17., , (a) Work, (c) Pressure, Unit of energy in SI system is, , [MP PMT 1993], , (b) m, (d) ms 2, [CPMT 1990; CBSE PMT 1993; BVP 2003], , [SCRA 1986; MNR 1986], , 19., , (b) Momentum, (d) Angular momentum, [CPMT 1971; NCERT 1976], , (a) Erg, (b) Calorie, (c) Joule, (d) Electron volt, A cube has numerically equal volume and surface area. The volume, of such a cube is, [CPMT 1971, 74], (a) 216 units, (b) 1000 units, (c) 2000 units, , (d) 3000 units, , Wavelength of ray of light is 0.00006 m . It is equal to, [CPMT 1977], , (b) 1 micron  10 6 cm, (d) 1 micron  10, , The unit of power is, (a) Joule, (b) Joule per second only, (c) Joule per second and watt both, (d) Only watt, A suitable unit for gravitational constant is, 1, , (a), , kg - m sec, , (c), , N m 2 kg 2, , (b), , Nm, , [AIIMS 1985], , is measured in metres and t in seconds., , Joule-second is the unit of, , (c) 10 cm, (d) 10 cm, A micron is related to centimetre as, , 5, , 10., , (d) m kg 1 K, , 16., , 18., , cm, , rad s 2, , 9, , (a) 1 micron  10 8 cm, , 9., , 6, , (c), , (c), , (b) 652189.63 time periods of Kr clock, , 7., , (b) m s 2, , (a) None, , (a) 1650763.73 time periods of Kr clock, (c) 1650763.73 time periods of Cs clock, , N kg 1, , CBSE PMT 2002], , 2, , One second is equal to, , (a), , [AFMC 1986; MP PET 1992; MP PMT 1992;, , (a) Velocity, (b) Angular momentum, (c) Momentum, (d) Energy, Which of the following is not represented in correct unit, [NCERT 1984; MNR 1995], , 6., , 13., , (b) Ampere  volt, (d) Ampere/volt, [CPMT 1984, 85; MP PMT 1984], , 5., , cm of Hg, , Light year is a unit of, [MP PMT 1989; CPMT 1991; AFMC 1991,2005], , 2., , Dynes / cm 2, , (a) Pascal, , 4, , cm, , 20., , [CPMT 1985], , 21., , (a), , 6 microns, , (b) 60 microns, , (c), , 600 microns, , (d) 0.6 microns, , Electron volt is a unit of, , [MP PMT 1993], , (a) Charge, (b) Potential difference, (c) Momentum, (d) Energy, Temperature can be expressed as a derived quantity in terms of any, of the following, [MP PET 1993; UPSEAT 2001], , [MNR 1988], 1, , sec, , (d) kg m sec 1, , 22., , (a) Length and mass, (b) Mass and time, (c) Length, mass and time, (d) None of these, Unit of power is, , [NCERT 1972; CPMT 1971; DCE 1999]

Page 2 :
Units, Dimensions and Measurement 45, (a) Kilowatt, (c) Dyne, 23., , (b) Kilowatt-hour, (d) Joule, , Density of wood is 0.5 gm / cc in the CGS system of units. The, corresponding value in MKS units is, , 36., , (a), , m / sec, , (b) m / sec 2, , (c), , m 2 / sec, , (b) m / sec 3, , One million electron volt (1 MeV ) is equal to, , [CPMT 1983; NCERT 1973; JIPMER 1993], , 24., , (a) 500, (c) 0.5, Unit of energy is, (a) J / sec, (c), , 25., , 26., , (b) W att day, (d), , gm-cm / sec, , 38., , (a) Force, (c) Power, The unit of potential energy is, , 39., , Newton/met re 2 is the unit of, , 30., , (a) Energy, (b) Momentum, (c) Force, (d) Pressure, The unit of surface tension in SI system is, , 40., , 41., , [CPMT 1985; ISM Dhanbad 1994; AFMC 1995], , 42., , Dyne / cm 2, , (b), , Newton / m, , 31., , (c) Dyne / cm, (d) Newton / m 2, The unit of reduction factor of tangent galvanometer is, , 32., , (a) Ampere, (b) Gauss, (c) Radian, (d) None of these, The unit of self inductance of a coil is, , 43., , [CPMT 1987; AFMC 2004], , [MP PMT 1983, 92; SCRA 1986; CBSE PMT 1993;, CPMT 1984, 85, 87], , 35., , (a), , g(cm / sec 2 ), , (b), , g(cm / sec)2, , (c), , g(cm 2 / sec), , (d), , g(cm / sec), , Which of the following represents a volt, , (a) Farad, (c) Weber, Henry/ohm can be expressed in, (a) Second, (c) Mho, The SI unit of momentum is, , 44., , 45., , (b) Henry, (d) Tesla, [CPMT 1987], , (b), , (c), , kg .m 2, sec, , (d) kg  Newton, , In which of the following systems of unit, W eber is the unit of, magnetic flux, (a) CGS, (b) MKS, (c) SI, (d) None of these, Tesla is a unit for measuring, [CBSE PMT 1993], (a) Magnetic moment, (b) Magnetic induction, (c) Magnetic intensity, (d) Magnetic pole strength, If the unit of length and force be increased four times, then the unit, of energy is, [Kerala PMT 2005], (a) Increased 4 times, (b) Increased 8 times, (c) Increased 16 times, (d) Decreased 16 times, Oersted is a unit of, [SCRA 1989], (a) Dip, (b) Magnetic intensity, (c) Magnetic moment, (d) Pole strength, Ampere  hour is a unit of, , 47., , (a) Quantity of electricity, (b) Strength of electric current, (c) Power, (d) Energy, The unit of specific resistance is, , [SCRA 1980, 89; ISM Dhanbad 1994], , [SCRA 1986, 89; CPMT 1987], , kg, m, , Kilowatt hour is a unit of, [NCERT 1975; AFMC 1991], (a) Electrical charge, (b) Energy, (c) Power, (d) Force, What is the SI unit of permeability, [CBSE PMT 1993], (a) Henry per metre, (b) Tesla metre per ampere, (c) Weber per ampere metre, (d) All the above units are correct, , 46., , (b) Coulomb, (d) Metre, , (a), , (b) Watt/Ampere, (d) Coulomb/Joule, , [SCRA 1991; CBSE PMT 1993; DPMT 2005], , [MP PMT 1984; AFMC 1986; CPMT 1985, 87; CBSE PMT 1993; KCET 1999;, DCE 2000, 01], , 34., , [AFMC 1991], , (a) Joule/second, (c) Watt/Coulomb, , 29., , 33., , [DCE 1993], , (b) Momentum, (d) Acceleration, , [CPMT 1990; AFMC 1991], , 1 km / sec, , The unit for nuclear dose given to a patient is, (a) Fermi, (b) Rutherford, (c) Curie, (d) Roentgen, Volt/metre is the unit of, [AFMC 1991; CPMT 1984], (a) Potential, (b) Work, (c) Force, (d) Electric intensity, , (a), , (d) 10 7 eV, , Erg  m 1 can be the unit of measure for, , (d) 1 m / sec, , 28., , (b) 10 eV, , 4, , 37., 2, , (b) Velocity of sound (332 m / sec), , 27., , 6, , (c) 10 eV, , [NCERT 1974; CPMT 1975], , Kilowatt, , 5, , (a) 10 eV, , Which is the correct unit for measuring nuclear radii, (a) Micron, (b) Millimetre, (c) Angstrom, (d) Fermi, One Mach number is equal to, (a) Velocity of light, (c), , [JIPMER 1993, 97], , (b) 5, (d) 5000, , kg .m, sec, , [SCRA 1989; MP PET 1984; CPMT 1975], , The velocity of a particle depends upon as v  a  bt  ct ; if the, velocity is in m / sec , the unit of a will be, , 2, , (a), , Ohm/cm, , (c), , Ohmcm, , 2, , [CPMT 1990], , 48., , (b) Ohm/cm, (d) (Ohmcm)1, , The binding energy of a nucleon in a nucleus is of the order of a, few, [SCRA 1979]

Page 3 :
46 Units, Dimensions and Measurement, , 49., , 50., , (a), , eV, , (b), , (c), , MeV, , (d) Volts, , Parsec is a unit of, (a) Distance, (c) Time, , 62., , Ergs, , In SI, Henry is the unit of, [MP PET 1984; CBSE PMT 1993; DPMT 1984], , (a) Self inductance, (c) (a) and (b) both, , [SCRA 1986; BVP 2003; AIIMS 2005], , (b) Velocity, (d) Angle, , 63., , The unit of e.m. f . is, , 64., , (a) Joule, (b) Joule-Coulomb, (c) Volt–Coulomb, (d) Joule/Coulomb, Which of the following is not the unit of time, , 65., , (a) Micro second, (c) Lunar months, (e) Solar day, Unit of self inductance is, , If u 1 and u 2 are the units selected in two systems of measurement, and n 1 and n 2 their numerical values, then, [SCRA 1986], , 51., , (a), , n1 u 1  n 2 u 2, , (b) n1 u1  n 2 u 2  0, , (c), , n1 n 2  u 1 u 2, , (d) (n1  u1 )  (n 2  u 2 ), , 1 eV is, , [SCRA 1986], , (a) Same as one joule, (c) 1V, 52., , 53., , 54., , (a) 1000W, , (b) 36  10 J, , (c) 1000 J, , (d) 3600 J, , 57., , 58., , 59., , 60., , J, , C, , (c), , kg  m 2, , 66., , 2, , (b) kg /cm, (d), , 69., [MP PMT 1984], , Nm, , (c), , N /m 2, , (d), , Nm 2, , 70., , Unit of Stefan's constant is, 1, , [MP PMT 1989], 2 1, , (b), , Jm s K, , (d), , Js, , Amperemetre, , (c), , W ebermetre 2, , 2, , Curie is a unit of, , [MP PET 1989], , 71., , (b), , Amperemetre, , (c), , W eber/metre, [CBSE PMT 1992; CPMT 1992], , (a) Energy of -rays, , (b) Half life, , (c) Radioactivity, Hertz is the unit for, , (d) Intensity of -rays, , 72., , 61., , (b) Force, (d) Magnetic flux, , (a) 10 24 F, , (b) 10 18 F, , (c) 10 12 F, , (d) 10 6 F, , (b) Magnetic flux – W eber, (c) Power – Farad, (d) Capacitance – Henry, The units of modulus of rigidity are, , [MP PMT 1997], , (a), , Nm, , (b), , N/m, , (c), , Nm 2, , (d), , N /m 2, , The unit of absolute permittivity is, (a), , Fm (Farad-meter), 2, , [MNR 1983; SCRA 1983; RPMT 1999], , (a) Frequency, (c) Electric charge, One pico Farad is equal to, , (a) Pressure, (b) Strain, (c) Compressibility, (d) Force, One yard in SI units is equal, [MP PMT 1995], (a) 1.9144 metre, (b) 0.9144 metre, (c) 0.09144 kilometre, (d) 1.0936 kilometre, Which of the following is smallest unit, [AFMC 1996], (a) Millimetre, (b) Angstrom, (c) Fermi, (d) Metre, Which one of the following pairs of quantities and their units is a, proper match, (a) Electric field – Coulomb / m, , 4, , Unit of magnetic moment is, (a), , 68., , 2, , Joule m, , (b), , J m 2, , Newton  metre, Volt metre, (d), Ampere, Coulomb, To determine the Young's modulus of a wire, the formula is, F L, Y  , ; where L = length, A  area of cross-section of the, A L, wire, L  change in length of the wire when stretched with a force, F . The conversion factor to change it from CGS to MKS system is, (a) 1, (b) 10, (c) 0.1, (d) 0.01, Young's modulus of a material has the same units as, [MP PMT 1994], , [MP PMT 1984], , N/m, , (c), , 67., , (d) 10 14 m 2, , (a), , Js, , (b), , (b) 10 30 m 2, , Unit of stress is, , (a), , [MP PET 1982], , Joule/Coulomb  Second, Ampere, , (c), , 5, , Unit of moment of inertia in MKS system, , kg  cm, , (b) Leap year, (d) Parallactic second, , Newton - second, (a), Coulomb  Ampere, , Universal time is based on, [SCRA 1989], (a) Rotation of the earth on its axis, (b) Earth's orbital motion around the earth, (c) Vibrations of cesium atom, (d) Oscillations of quartz crystal, The nuclear cross-section is measured in barn, it is equal to, , (a), , [CPMT 1986; AFMC 1986], , [CPMT 1991; NCERT 1990; DPMT 1987; AFMC 1996], , [AFMC 1986; SCRA 1986, 91], , (c) 10 28 m 2, , 56., , (d) 1.6  10, , 19, , 1kW h , , (a) 10 20 m 2, , 55., , (b) 1.6  10, , 19, , (b) Mutual inductance, (d) None of the above, , 73., , (b), 2, , [CMEET Bihar 1995], , Fm, , 1, , (Farad/meter), , (c) Fm (Farad/ metre ), (d) F (Farad), (e) None of these, Match List-I with List-II and select the correct answer using the, codes given below the lists, [SCRA 1994], List-I, List-II, I. Joule, A. Henry  Amp/sec, II. Watt, B. Farad  Volt, III. Volt, C. Coulomb  Volt

Page 4 :
Units, Dimensions and Measurement 47, D. Oersted  cm, E. Amp  Gauss, , IV. Coulomb, , 82., , F. Amp 2  Ohm, 83., , Codes:, , 74., , (a), , I  A, II  F, III  E, IV  D, , (b), , I  C, II  F, III  A, IV  B, , (c), , I  C, II  F, III  A, IV  E, , (d), , I  B, II  F, III  A, IV  C, , 84., , Which relation is wrong, (a) 1 Calorie = 4.18 Joules, (b) 1 Å  10, , 10, , [RPMT 1997], , 85., , m, , (c) 1 MeV  1.6  10, , 13, , Joules, , 86., , (d) 1 Newton  10 5 Dynes, 75., , 76., , 77., , 78., , 79., , (a), , km/s, , (b) kms, , (c), , km /s 2, , (d) kms 2, , a , , The equation  P  2  (V  b) constant. The units of a are, V , , , (a), , Dyne  cm 5, , (b), , Dyne  cm 4, , (c), , Dyne/cm 3, , (d), , Dyne/cm 2, , 80., , 81., , kgm/s, , 87., , 88., , 89., , (a), , kgm 2 /s 2, , (b), , (c), , Joule/s, , (d) kgms 2, , Joules, , Which of the following is not the unit of energy, , (b) Vm 1, , (c), , JC 1, , (d), , (b), , J-kg 1, , (c), , J-kg, , (d), , J-kg 2, , Which one of the following is not a unit of young's modulus, , 272.85 K, , (c), , 273 K, , (d), , 273.2 K, , Nm, , (d) Mega Pascal, , The unit of L / R is (where L = inductance and R = resistance), , 92., , 93., , 95., , (a) sec, (b) sec 1, (c) Volt, (d) Ampere, Which is different from others by units, [Orissa JEE 2002], (a) Phase difference, (b) Mechanical equivalent, (c) Loudness of sound, (d) Poisson's ratio, Length cannot be measured by, [AIIMS 2002], (a) Fermi, (b) Debye, (c) Micron, (d) Light year, The value of Planck's constant is, [CBSE PMT 2002], (a), , 6.63  10 34 J-sec, , (b) 6.63  10 34 J /sec, , (c), , 6.63  10 34 kg-m 2, , (d) 6.63  10 34 kg /sec, , A physical quantity is measured and its value is found to be nu, where n  numerical value and u  unit. Then which of the, following relations is true, [RPET 2003], (a), , n  u2, , (b) n  u, , (c), , n u, , (d) n , , [UPSEAT 2000], , (b), , (b), , 91., , The correct value of 0 o C on the Kelvin scale is, 273.15 K, , Dyne cm, , 2, , 2, , In C.G.S. system the magnitutde of the force is 100 dynes. In another, system where the fundamental physical quantities are kilogram,, metre and minute, the magnitude of the force is, (a) 0.036, (b) 0.36, (c) 3.6, (d) 36, , JC 1 m 1, , (a), , 1 1995], [AFMC, Nm, , 90., , [UPSEAT 1999], , NC 1, , J [MNR 1995; AFMC 1995], , (c), , (d) Joule, , (a), , (a), , (a), , 94., , (b) kgm, , Which is not a unit of electric field, , (a) Calorie, (b) Joule, (c) Electron volt, (d) Watt, [CBSE PMT 1993], Which of the following is not a unit of time [UPSEAT 2001], (a) Leap year, (b) Micro second, (c) Lunar month, (d) Light year, The S.I. unit of gravitational potential is, [AFMC 2001], , [KCET 2005], , Which of the following quantity is expressed as force per unit area, (a) Work, (b) Pressure, (c) Volume, (d) Area, Match List-I with List-II and select the correct answer by using the, codes given below the lists, [NDA 1995], List-I, List-II, (a) Distance between earth and stars 1. Microns, (b) Inter-atomic distance in a solid 2. Angstroms, (c) Size of the nucleus, 3. Light years, (d) Wavelength of infrared laser, 4. Fermi, 5. Kilometres, Codes, a b c d, a b c d, (a) 5 4 2 1, (b) 3 2 4 1, (c) 5 2 4 3, (d) 3 4 1 2, Unit of impulse is, [CPMT 1997], , (c), , 2, , [MP PET 2000], , If x  at  bt 2 , where x is the distance travelled by the body in, kilometres while t is the time in seconds, then the units of b are, , (a) Newton, , 'Torr' is the unit of, [RPMT 1999, 2000], (a) Pressure, (b) Volume, (c) Density, (d) Flux, Which of the following is a derived unit, [BHU 2000], (a) Unit of mass, (b) Unit of length, (c) Unit of time, (d) Unit of volume, Dyne/cm is not a unit of, [RPET 2000], (a) Pressure, (b) Stress, (c) Strain, (d) Young's modulus, The units of angular momentum are [MP PMT 2000], , 96., , Faraday is the unit of, , 1, u, [AFMC 2003]

Page 5 :
48 Units, Dimensions and Measurement, , 97., , 98., , 99., , (a) Charge, (c) Mass, Candela is the unit of, (a) Electric intensity, (c) Sound intensity, The unit of reactance is, (a) Ohm, (c) Mho, The unit of Planck's constant is, , (b) emf, (d) Energy, , (a), (b), (c), (d), , [UPSEAT 1999; CPMT 2003], , (b) Luminous intensity, (d) None of these, , 2., , Dimensional formula ML1T 2 does not represent the physical, quantity, [Manipal MEE 1995], (a) Young's modulus of elasticity, (b) Stress, (c) Strain, (d) Pressure, , 3., , Dimensional formula ML2T 3 represents, , 4., , (a) Force, (c) Energy, The dimensions of calorie are, , [MP PET 2003], , (b) Volt, (d) Newton, , [RPMT 1999; MP PET 2003; Pb. PMT 2004], , 100., , 101., , (a) Joule, (c) Joule/m, Number of base SI units is, (a) 4, (c) 3, SI unit of permittivity is, (a), , 102., , 2, , 2, , 1, , 2, , 2, , 2, , C m N, , (b) Joule/s, (d) Joule-s, , [KCET 2004], 1, , 2, , 2, , 2, , 2, , 1, , (c) C m N, (d) C m N, Which does not has the same unit as others, , [Orissa PMT 2004], , 103., , (a), 104., , 105., , Nm, , 1, , [EAMCET 1981; MP PMT 1996, 2001], , [MP PET 2003], , (b) 7, (d) 5, (b) C m N, , (a) Watt-sec, (c) eV, Unit of surface tension is, , 5., , (b) Kilowatt-hour, (d) J-sec, [Orissa PMT 2004], , (b), , Nm, , 6., , 2, , (c) N 2m 1, (d) Nm 3, Which of the following system of units is not based on units of, mass, length and time alone, [Kerala PMT 2004], (a) SI, (b) MKS, (c) FPS, (d) CGS, The unit of the coefficient of viscosity in S.I. system is, (a), , m / kg-s, , (b) m-s/kg 2, , (c), , kg /m-s 2, , (d) kg /m-s, , The unit of Young’s modulus is, (a), , 107., , Nm 2, , (c), 108., , 10 12 m, , 7., , (a), , ML2T 2, , (b), , MLT 2, , (c), , ML2T 1, , (d), , ML2T 3, , Whose dimensions is ML2 T 1, (a) Torque, (c) Power, , If L and R are respectively the inductance and resistance, then, L, the dimensions of, will be, R, , (a), , M 0 L0 T 1, , (b), , M 0 LT 0, , (c), , M 0 L0 T, , Which pair has the same dimensions, [EAMCET 1982; CPMT 1984, 85;, , (b), , Pb. PET 2002; MP PET 1985], , Nm 2, , (a), (b), (c), (d), , (b) 10 15 m, (d) 1012 m, , 8., , Work and power, Density and relative density, Momentum and impulse, Stress and strain, , If C and R represent capacitance and resistance respectively, then, the dimensions of RC are, , How many wavelength of Kr 86 are there in one metre, , [CPMT 1981, 85; CBSE PMT 1992, 95; Pb. PMT 1999], , (a) 1553164.13, (b) 1650763.73, (c) 652189.63, (d) 2348123.73, Which of the following pairs is wrong [AFMC 2003], (a) Pressure-Baromter, (b) Relative density-Pyrometer, (c) Temperature-Thermometer, (d) Earthquake-Seismograph, , (a), 9., , 10., , 0, , 0, , M L T, , 2, , Select the pair whose dimensions are same, , (b), , M 0 L0 T, , (c) ML1, (d) None of the above, Dimensions of one or more pairs are same. Identify the pairs, (a) Torque and work, (b) Angular momentum and work, (c) Energy and Young's modulus, (d) Light year and wavelength, Dimensional formula for latent heat is, , Dimensions, 1., , [CPMT 1989], , (b) Angular momentum, (d) Work, , [Pb. PET 2001], , [MNR 1985; UPSEAT 2000; Pb. PET 2004], , 109., , [CPMT 1985], , (d) Cannot be represented in terms of M, L and T, , (c) Nm, (d) Nm 1, One femtometer is equivalent to, [DCE 2004], (a) 1015 m, , (b) Power, (d) Work, , [CPMT 1986; CBSE PMT 1988; Roorkee 1995; MP PET/PMT, 1998; DCE 2002], , [J & K CET 2004], , 106., , Pressure and stress, Stress and strain, Pressure and force, Power and force, , [MNR 1987; CPMT 1978, 86; IIT 1983, 89; RPET 2002], , (a), , 0 2, , M LT, , 2, , (b), , MLT 2

Page 6 :
Units, Dimensions and Measurement 49, , 11., , (c) ML2T 2, (d) ML2T 1, Dimensional formula for volume elasticity is, , (a), , [MP PMT 1991, 2002; CPMT 1991; MNR 1986], , (a), , 1 2, , M L T, , (b), , 1 3, , M L T, , 21., , 2, , 2, , (c) M L T, (d) M 1 L1T 2, The dimensions of universal gravitational constant are, 1 2, , 12., , 2, , [MP PMT 1984, 87, 97, 2000; CBSE PMT 1988, 92; 2004, MP PET 1984, 96, 99; MNR 1992; DPMT 1984;, , 22., , CPMT 1978, 84, 89, 90, 92, 96; AFMC 1999;, RPET 2001; Pb. PMT 2002, 03; UPSEAT 1999;, BCECE 2003, 05;], , (a), , M LT, 1, , 13., , 2, , (b), , 1 3, , M LT, , 2, , 23., , 2, , (c) ML T, (d) ML2T 2, The dimensional formula of angular velocity is, [JIPMER 1993; AFMC 1996; AIIMS 1998], , (a), 14., , 0 0, , M LT, , 1, , (c) M 0 L0 T 1, The dimensions of power are, , (b), , MLT 1, , (d), , ML0 T 2, , (a), 15., , (c) M 1 L2T 1, The dimensions of couple are, (a), , 16., , M LT, , 3, , ML2T 2, , (b), , M 2 L1T 2, , (d), , M 1 L1T 2, , 24., , (a) Momentum, (b) Force, (c) Rate of change of momentum, (d) Torque, Which of the following is dimensionally correct, (a) Pressure = Energy per unit area, (b) Pressure = Energy per unit volume, (c) Pressure = Force per unit volume, (d) Pressure = Momentum per unit volume per unit time, Planck's constant has the dimensions (unit) of, [CPMT 1983, 84, 85, 90, 91; AIIMS 1985; MP PMT 1987;, EAMCET 1990; RPMT 1999; CBSE PMT 2001;, MP PET 2002; KCET 2004], , 25., , [CPMT 1972; JIPMER 1993], , (b), , MLT 2, , (a) Energy, (b), (c) Work, (d), The equation of state of some, a , ,  P  2  (V  b)  RT . Here P, V, , , , [CBSE PMT 1991, 96; NCERT 1984; MP PET 1992;, CPMT 1974, 79, 87, 97; MP PMT 1992, 94;, MNR 1995; AFMC 1995], , CMC Vellore 1982; CPMT 1973, 82, 86;, MP PMT 1987; BHU 1995; IIT 1983;, , (a), , Pb. PET 2000], , 17., , (b), , ML2T 1, , (c) MLT 1, (d) M 0 L2T 2, The dimensional formula for impulse is, , 26., , 18., , (b), , MLT 1, , 27., , (a), 19., , 20., , ML T, , (b), , 1, , ML T, , (a), , M 0 LT 1, , (b), , M 0 L0 T 2, , (c), , M 0 L0 T 1, , (d), , MLT 3, , AFMC 2003; RPMT 1999; Kerala PMT 2002], , (c) M LT, (d) ML2 T 2, If L denotes the inductance of an inductor through which a, [CPMT 1982, 85, 87], , (a), , 2, , ML T, , 2, , (b) Not expressible in MLT, , 2, , 28., , (c) MLT, (d) M 2 L2 T 2, Of the following quantities, which one has dimensions different from, the remaining three, [AIIMS 1987; CBSE PMT 1993], , The dimensional formula for Planck's constant (h) is, [DPMT 1987; MP PMT 1983, 96; IIT 1985; MP PET 1995;, , MLT, , current i is flowing, the dimensions of Li 2 are, , 3, , (c) ML2T 2, (d) ML1T 2, The dimensional formula for r.m.s. (root mean square) velocity is, , (b), , 2, , 1, , 2, , [MNR 1984; IIT 1982; MP PET 2000], 2, , ML1 T 2, , (c) M 0 L3 T 0, (d) M 0 L6 T 0, If V denotes the potential difference across the plates of a capacitor, , (a) Not expressible in MLT, , (c) ML2T 1, (d) M 2 LT 1, The dimensional formula for the modulus of rigidity is, 2, , (b), , [CPMT 1982], , AFMC 1998; BCECE 2003], , MLT 2, , ML5 T 2, , of capacitance C , the dimensions of CV 2 are, , [EAMCET 1981; CBSE PMT 1991; CPMT 1978;, , (a), , is the pressure, V is the, , The dimensions of ' a' are, , [CBSE PMT 1988, 92; EAMCET 1995; DPMT 1987;, , ML2T 2, , Linear momentum, Angular momentum, gases can be expressed as, , volume, T is the absolute temperature and a, b, R are constants., , (c) ML1T 3, (d) ML2T 2, Dimensional formula for angular momentum is, , (a), , ML2T 2, , (c) ML2T 1, (d) ML2T 2, Out of the following, the only pair that does not have identical, dimensions is, [MP PET/PMT 1998; BHU 1997], (a) Angular momentum and Planck's constant, (b) Moment of inertia and moment of a force, (c) Work and torque, (d) Impulse and momentum, The dimensional formula for impulse is same as the dimensional, formula for, , [CPMT 1974, 75; SCRA 1989], 1 2, , (b), , [CPMT 1982, 83; CBSE PMT 1993; UPSEAT 2001], , NCERT 1975; DPET 1993; AIIMS 2000;, , 2 2, , ML2T 3, , 29., , (a) Energy per unit volume, (b) Force per unit area, (c) Product of voltage and charge per unit volume, (d) Angular momentum per unit mass, A spherical body of mass m and radius r is allowed to fall in a, medium of viscosity  . The time in which the velocity of the body

Page 7 :
50 Units, Dimensions and Measurement, increases from zero to 0.63 times the terminal velocity (v) is called, , 37., , time constant ( ) . Dimensionally  can be represented by, mr 2, (a), 6, , (c), 30., , (b), , m, 6rv, ,  6mr , , ,  g2 , , , , 32., , (a), 38., , (d) None of the above, 39., , 1, 1, ,y , 2, 2, , (b) x  , , 1, 1, ,y  , 2, 2, , (c) x , , 1, 1, ,y  , 2, 2, , (d) x  , , 1, 1, ,y , 2, 2, , 33., , (a), , v  g , , (c), , v 2  g, , (c), , 1, , (d) v 2  g 1 3, , 2, , 2, , M L T Q, , 2, , M 1 L2 T 2 Q, , (b), (d), , (a), (c), 35., , ML T Q, 1, , 2, , ML T Q, , 1, , (b), (d), , 42., , 43., , M 1 L2 TQ 2, , 3, , 2, , (a), (c), , ML T, , MLT, , 2, , K, , K, , 1, , 45., , ML T Q, , (d), , (d), , M 1 L2 T 4 A 2, , 2, , ML T, , 2, , 0, , 0, , 2, , 0, , 0, , 0, , (b), , 1, , MLT Q, , MLT, , 1, , M L T, , (b), , 0, , M L0 T 2, , (c) M 2 L0 T 2, (d) MLT 2, Which of the following quantities has the same dimensions as that, of energy, [AFMC 1991; CPMT 1976; DPMT 2001], (a) Power, (b) Force, (c) Momentum, (d) Work, L, during growth and decay of, R, current in all inductive circuit is same as that of, , The dimensions of "time constant", , (b) Resistance, (d) Time, , The period of a body under SHM i.e. presented by T  P a D b S c ;, where P is pressure, D is density and S is surface tension. The, value of a, b and c are [CPMT 1981], (a), , , , 1, , 3 1, , ,1, 2 2, , (b) 1,  2, 3, , 1, 3, 1, 1, (d) 1, 2,, , ,, 2, 2, 2, 3, Which of the following pairs of physical quantities has the same, dimensions, [CPMT 1978; NCERT 1987], (a) Work and power, (b) Momentum and energy, (c) Force and power, (d) Work and energy, , (c), 46., , (b), , ML2 T 4 A 2, , (c) M L T, (d) None of these, If C and L denote capacitance and inductance respectively, then, the dimensions of LC are, , 1, , 47., , [MP PMT 1993], 1, , (b), , [MP PET 1993; EAMCET 1994], , (c) T 1, (d) T 2, The dimensions of coefficient of thermal conductivity is, 2, , MLT 4 A 2, , (a) Constant, (c) Current, , (b) T, , 2, , (c), , 2, , M L TQ, , where  is the angular velocity and v is the linear velocity. The, dimension of k is, [MP PMT 1993], , 36., , M L T A, , (a), , 2, , x, , Y  A sin   k , v, , , LT, , 4, , [CPMT 1981; MP PET 1997], , The equation of a wave is given by, , (a), , 2, , (a), , (a), , [MP PET 1993], 2, , 1, , 41., , Q stands for the dimensions of charge, is, 1, , (c) M 0 LT 1, (d) M 0 L1 T 1, Dimensional formula of capacitance is, , [CPMT 1975], , 44., , The dimensions of resistivity in terms of M , L, T and Q where, , 3, , LT 0, , (a) Power, (b) Momentum, (c) Force, (d) Couple, Dimensional formula of heat energy is, , [MP PET 1993], 1, , (b), , MLT 1 represents the dimensional formula of, , (b) v 2  g, , The dimensions of Farad are, (a), , 34., , 1, , M 0 LT 2, , 40., , [NCERT 1979; CET 1992; MP PET 2001; UPSEAT 2000], 1, , M 0 L1 T 2, , [CPMT 1976, 81, 86, 91], , The quantities A and B are related by the relation, m  A / B ,, where m is the linear density and A is the force. The dimensions, of B are of, (a) Pressure, (b) Work, (c) Latent heat, (d) None of the above, The velocity of water waves v may depend upon their wavelength,  , the density of water  and the acceleration due to gravity g ., The method of dimensions gives the relation between these, quantities as, 2, , (b), , [CPMT 1978; MP PMT 1979; IIT 1983], , The frequency of vibration f of a mass m suspended from a, spring of spring constant K is given by a relation of this type, , (a) x , , 0, 2, M[AIIMS, LT 1987], , (c) ML1 T 2, (d) ML2 T 2, Dimensional formula of velocity of sound is, (a), , f  C m x K y ; where C is a dimensionless quantity. The value of, x and y are, [CBSE PMT 1990], , 31., , Dimensional formula of stress is, , MLT, , MLT, , 3, , 3, , K, , K, , 1, , The velocity of a freely falling body changes as g p hq where g is, acceleration due to gravity and h is the height. The values of p, and q are, [NCERT 1983; EAMCET 1994], (a) 1,, (c), , 1, 2, , 1, ,1, 2, , (b), , 1 1, ,, 2 2, , (d) 1, 1

Page 8 :
Units, Dimensions and Measurement 51, 48., , 49., , Which one of the following does not have the same dimensions, (a) Work and energy, (b) Angle and strain, (c) Relative density and refractive index, (d) Planck constant and energy, Dimensions of frequency are, [CPMT 1988], (a), , 50., , 51., , M 0 L1T 0, , (b), , 57., , 58., , M 0 L0 T 1, , (c) M 0 L0 T, (d) MT 2, Which one has the dimensions different from the remaining three, (a) Power, (b) Work, (c) Torque, (d) Energy, A small steel ball of radius r is allowed to fall under gravity, through a column of a viscous liquid of coefficient of viscosity  ., After some time the velocity of the ball attains a constant value, known as terminal velocity v T . The terminal velocity depends on (i), the mass of the ball m , (ii)  , (iii) r and (iv) acceleration due to, gravity g . Which of the following relations is dimensionally correct, [CPMT 1992; CBSE PMT 1992;, , 59., , (c), , 52., , mg, r, , (b) vT , , 60., , 61., , vT  rmg, , (d) vT , , mgr, , , , (d) Current, , 63., , (a), (c), 54., , LT, , 1, , (b), 3, , 2, , M L Q T, , 2, , (d), , 64., , 2, , (c), , 2, , 2, , M L T, , (b), , 2, , M L I T, , The dimensions of CV, , (d), 2, , 2, , M L T, , 1, , The dimension of, ,  0 0, , is that of [SCRA 1986], , (a) Velocity, (b) Time, [CBSE PMT 1988], (c) Capacitance, (d) Distance, An athletic coach told his team that muscle times speed equals, power. What dimensions does he view for muscle, MLT 2, , (b), , ML2T 2, , (c) MLT 2, (d) L, The foundations of dimensional analysis were laid down by, (a) Gallileo, (b) Newton, (c) Fourier, (d) Joule, The dimensional formula of wave number is, M 0 L0 T 1, , (b), , M 0 L1T 0, , (c) M 1 L1T 0, (d) M 0 L0 T 0, The dimensions of stress are equal to [MP PET 1991, 2003], (a) Force, (b) Pressure, (d), , 1, P ressure, , The dimensions of pressure are, MLT, , 2, , (c) ML1T 2, Dimensions of permeability are, , (b), , ML2T 2, , (d), , MLT 2, , 2, , 1 1, , A M LT, , 2, , (b), , MLT 2, , (c) ML0 T 1, (d) A 1 MLT 2, Dimensional formula of magnetic flux is, [DCE 1993; IIT 1982; CBSE PMT 1989, 99;, DPMT 2001; Kerala PMT 2005], , 66., , (a), , ML2T 2 A 1, , (b), , ML0 T 2 A 2, , (c), , M 0 L2 T 2 A 3, , (d), , ML2 T 2 A 3, , If P represents radiation pressure, c represents speed of light and, Q represents radiation energy striking a unit area per second, then, non-zero integers, dimensionless, are, , M 2 L2 T 1, 1, , AT 2, , 2, , (b) Kinetic energy, (d) Power, , M 1 L4 T 2, , (d), , (a), 65., , 3 2, , The dimensions of physical quantity X in the equation Force, X, is given by, [DCE 1993], , Density, (a), , 56., , 1, , F 1 A 2 T 1, , [CBSE PMT 1991; AIIMS 2003], , The expression [ML2 T 2 ] represents [JIPMER 1993, 97], (a) Pressure, (c) Momentum, , 55., , L T, , (c), , (a), , the dimensions of  0  0 are, 2, , F 1 T 2, , [CPMT 1977; MP PMT 1994], ,  0 and  0 denote the permeability and permittivity of free space,, 1, , (b), , (c) Work, ,  0 LV, , (c) Voltage, 53., , 62., , :  0 is the permittivity of free space,, t, L is length, V is potential difference and t is time. The, dimensions of X are same as that of [IIT 2001], (a) Resistance, (b) Charge, The quantity X , , FT 2, , (a), , r, mg, , (a), , (a), , NCERT 1983; MP PMT 2001], , (a) vT , , [CPMT 1985], The Martians, use force (F) , acceleration (A) and time (T ) as, their fundamental physical quantities. The dimensions of length on, Martians system are, [DCE 1993], , x, y, , and, , z, , such that, , PxQycz, , is, , [AFMC 1991; CBSE PMT 1992;, CPMT 1981, 92; MP PMT 1992], , 1, , matches with the dimensions of, [DCE 1993], , (a), , x  1, y  1, z  1, , (b), , x  1, y  1, z  1, , (a), , 2, , L I, , (b), , L I, , (c), , x  1, y  1, z  1, , (c), , LI 2, , (d), , 1, LI, , (d), , x  1, y  1, z  1, , 2 2, , 67., , Inductance L can be dimensionally represented as, [CBSE PMT 1989, 92; IIT 1983; CPMT 1992;, DPMT 1999; KCET 2004; J&K CET 2005]

Page 9 :
52 Units, Dimensions and Measurement, (a), 68., , ML2 T 2 A 2, , (c) ML2 T 2 A 2, Dimensions of strain are, (a) MLT, , 69., , (a), , ML2 T 4 A 3, , (d), , [MP PET 1984; SCRA 1986], , (b) ML T 1, 2, , T 1, , 79., , (b) T 2, , (c) T 3, (d) T 0, Dimensions of kinetic energy are, , 71., , ML2T 2, , (b), , M 2 LT 1, , (c) ML2T 1, (d), Dimensional formula for torque is, , ML3 T 1, , 72., , L2 MT 2, , [AIIMS 1993; CPMT 1992; Bihar PET 1984;, MP PMT 1987, 89, 91; AFMC 1986;, CBSE PMT 1992; KCET 1994;, DCE 1999; AIEEE 2004;, DPMT 2004], , 73., , ML2T 2, , (b), , ML2T 1, , (c), , ML1T 1, , (d), , MLT, , The dimension of quantity (L / RCV ) is, (a) [A], (c), , 74., , [ A 1 ], 0 1, , M LT, , 0, , 1, , 78., , 1, , 1 3, , 2, , (b), , A T M L, , A 2T 4 M 1 L3, , (c) A 2T 4 ML3, (d) A 2T 4 M 1 L3, Dimensions of the following three quantities are the same, (a) Work, energy, force, (b) Velocity, momentum, impulse, (c) Potential energy, kinetic energy, momentum, (d) Pressure, stress, coefficient of elasticity, The dimensions of Planck's constant and angular momentum are, respectively, [CPMT 1999; BCECE 2004], (a), , ML2T 1 and MLT 1, 1, , 83., , (b), , ML2T 1 and ML2T 1, , (c) MLT and ML T, (d) MLT 1 and ML2T 2, Let [ 0 ] denotes the dimensional formula of the permittivity of the, 2, , 1, , M  mass , L  length , T  Time and I  electric current ,, then, [IIT 1998], , (b) [ 0 ]  M 1 L3 T 4 I 2, , (d) None of these, , (c) [ 0 ]  MLT 2 I 2, , (d) [ 0 ]  ML2 T 1 I, , 1 1, , (b), , M LT, , 1, , 84., , (b), , [MNR 1994], , Dimensions of CR are those of, [EAMCET (Engg.) 1995; AIIMS 1999], , 1, , ML1T 2, , 2, , (a) [ 0 ]  M 1 L3 T 2 I, , (a) Angular momentum, work, (b) Work, torque, (c) Potential energy, linear momentum, (d) Kinetic energy, velocity, The dimensions of surface tension are, (a), , Fv3 A 2, , (b) [ A 2 ], , 85., , (a) Frequency, , (b) Energy, , (c) Time period, , (d) Current, , The physical quantity that has no dimensions, [EAMCET (Engg.) 1995], , [MP PMT 1994, 99; UPSEAT 1999], , 77., , 82., , [Roorkee 1994], , [MP PET 1994; CPMT 1996], , 76., , (b), , [MP PET 1997], , (c) M L T, (d) M 1 L1T 1, The pair having the same dimensions is, 1 2, , FA1v, , vacuum and [0 ] that of the permeability of the vacuum. If, , The dimension of the ratio of angular to linear momentum is, (a), , 75., , 81., , (c) L2 MT 3, (d) LMT 2, Dimensions of coefficient of viscosity are, , (a), , L, R, , (d), , (c) Fv2 A 1, (d) F 2v 2 A 1, The dimensions of permittivity  0 are, (a), , L1 MT 2, , (b), , R, L, , L, R, , [MP PET 1997; AIIMS-2004; DCE-2003], , [DPMT 1984; IIT 1983; CBSE PMT 1990; MNR 1988; AIIMS 2002; BHU 1995,, 2001; RPMT 1999;, RPET 2003; DCE 1999, 2000; DCE 2004], , (a), , 80., , (b), , If velocity v , acceleration A and force F are chosen as, fundamental quantities, then the dimensional formula of angular, momentum in terms of v, A and F would be, (a), , [Bihar PET 1983; DPET 1993; AFMC 1991], , (a), , R, L, , (c), , (c) MLT 2, (d) M 0 L0 T 0, Dimensions of time in power are, [EAMCET 1982], (a), , 70., , 1, , [MP PMT 1996, 2000, 02; MP PET 1999], , ML2 T 4 A 3, , (b), , 86., , (a) Angular Velocity, , (b) Linear momentum, , (c) Angular momentum, , (d) Strain, , 1, , ML T, , 2, , represents, , MLT 2, , [EAMCET (Med.) 1995; Pb. PMT 2001], , (a) Stress, , 2, , (c) ML T, (d) MT, In the following list, the only pair which have different dimensions,, is, [Manipal MEE 1995], (a) Linear momentum and moment of a force, (b) Planck's constant and angular momentum, (c) Pressure and modulus of elasticity, (d) Torque and potential energy, If R and L represent respectively resistance and self inductance,, which of the following combinations has the dimensions of, frequency, , (b) Young's Modulus, (c) Pressure, (d) All the above three quantities, 87., , Dimensions of magnetic field intensity is, [RPMT 1997; EAMCET (Med.) 2000; MP PET 2003], , (a) [M L T A ], , (b) [MLT 1 A 1 ], , (c) [ML0 T 2 A 1 ], , (d) [MLT 2 A], , 0, , 1, , 0, , 1

Page 10 :
Units, Dimensions and Measurement 53, 88., , 89., , 98., Dimension of electric current is, The force F on a sphere of radius ' a' moving in a medium with, velocity 'v ' is given by F  6av . The dimensions of  are, [CBSE PMT 1997;, (a) DPMT, [M 02000], L0 T 1 Q], (a), , ML1 T 1, , (b), , MT 1, , (c), , MLT 2, , (d), , ML3, , 99., , Which physical quantities have the same dimension, [CPMT 1997], , (a) Couple of force and work, , 90., , 91., , (c) Latent heat and specific heat, , (a), , (d) Work and power, , (c) PVT 2, (d) P 1 VT 2, The physical quantity which has dimensional formula as that of, Energy, [CPMT 1997], is, [EAMCET (Eng.) 2000], Mass  Length, (a) Force, (b) Power, (c) Pressure, (d) Acceleration, , Two quantities A and B have different dimensions. Which, mathematical operation given below is physically meaningful, AB, , (a), , A/B, , (b), , (c), , AB, , (d) None, , Given that v is speed, r is the radius and g is the acceleration, due to gravity. Which of the following is dimensionless, v 2 / rg, , 101., , 102., , v2g /r, , (a) Surface tension, , (b) Solar constant, , (c) Density, , (d) Compressibility, , 3, , A force F is given by F  at  bt 2 , where t is time. What are, the dimensions of a and b, 3, , 2, , and ML T, , 4, , (b), , 103., , (a), , MT 2, , (b), , MLT 1, , (c), , MLT 2, , (d), , ML1 T 1, , If the speed of light (c) , acceleration due to gravity (g) and, pressure (p) are taken as the fundamental quantities, then the, dimension of gravitational constant is, , 104., , 96., , 2, , (a), , c g p, , (c), , cg 3 p 2, , 0, , 2, , (b) c g p, , 105., , 106., , 1, , (d) c 1 g 0 p 1, , If the time period (T ) of vibration of a liquid drop depends on, , 107., , T  k r 3 / S, , (b) T  k  1 / 2 r 3 / S, , (c), , T  k r 3 / S 1 / 2, , (d) None of these, , ML3 T 1 Q 2 is dimension of, (a) Resistivity, (c) Resistance, , (b) Conductivity, (d) None of these, , [RPET 2000], , (b), , ML2 T 3, , (c) ML2 T 1, (d) MLT 2, A physcial quantity x depends on quantities y and z as follows:, , (a), , x and B, , (b) C and z 1, , (c), , y and B / A, , (d), , x and A, , Which of the following pair does not have similar dimensions, (a) Stress and pressure, (b) Angle and strain, (c) Tension and surface tension, (d) Planck's constant and angular momentum, Out of the following which pair of quantities do not have same, dimensions, [RPET 2001], (a) Planck's constant and angular momentum, (b) Work and energy, (c) Pressure and Young's modulus, (d) Torque & moment of inertia, Identify the pair which has different dimensions, (a), (b), (c), (d), , [AMU (Med.) 2000], , (a), , ML2 T 2, , [KCET 2001], , surface tension (S ) , radius (r) of the drop and density ( ) of the, liquid, then the expression of T is, , 97., , Ev, , x  Ay  B tan Cz , where A, B and C are constants. Which of, the following do not have the same dimensions, , [AMU (Med.) 1999], 0, , (b), , 2, , (c) Fv, (d) Fv 2, Dimensions of luminous flux are, [UPSEAT 2001], (a), , MLT 3 and MLT 4, , (c) MLT 1 and MLT 0, (d) MLT 4 and MLT 1, The dimensions of inter atomic force constant are, , 2, , Ev, , 2, 1, , [UPSEAT 1999], , 95., , P 1 V 2 T 2, , If energy (E) , velocity (v) and force (F) be taken as fundamental, [CET, quantity,, then1998], what are the dimensions of mass, (a), , (d) v 2 rg, , The physical quantity which has the dimensional formula M T, is, [CET 1998], , MLT, , (b), , [AMU 2000], , 1, , (a), , PV 2 T 2, , (b) v 2 r / g, , [AFMC 2001; BHU 1998, 2005], , 94., , (c) [M 2 LT 1 Q], (d) [M 2 L2 T 1 Q], The fundamental physical quantities that have same dimensions in, the dimensional formulae of torque and angular momentum are, (a) Mass, time, (b) Time, length, (c) Mass, length, (d) Time, mole, If pressure P , velocity V and time T are taken as fundamental, physical quantities, the dimensional formula of force is, , (c), , 93., , (b) [ML2 T 1 Q], , (b) Force and power, , (a), , 92., , 100., , [CBSE PMT 2000], , 108., , Planck's constant and angular momentum, Impulse and linear momentum, Angular momentum and frequency, Pressure and Young's modulus, , The dimensional formula M 0 L2 T 2 stands for, (a) Torque, (b) Angular momentum, (c) Latent heat, (d) Coefficient of thermal conductivity, , [KCET 2001]

Page 11 :
54 Units, Dimensions and Measurement, 109., , Which of the following represents the dimensions of Farad, [AMU (Med.) 2002], , (a), (c), 110., , 1 2, , 4, , M L T A, 2, , 2, , ML T A, , 2, , 1, , 2, , 2, , 2, , (b), , ML T A, , (d), , MT 2 A 1, , If L, C and R denote the inductance, capacitance and resistance, respectively, the dimensional formula for C 2 LR is, 2, , 111., , 1 0, , 0, , 0, , 3 0, , (a) [ML T I ], , (b) [M L T I ], , (c) [M 1 L2 T 6 I 2 ], , (d) [M 0 L0 T 2 I 0 ], , If the velocity of light (c) , gravitational constant (G) and Planck's, constant (h) are chosen as fundamental units, then the dimensions, of mass in new system is, [UPSEAT 2002], (a), (c), , c 1 / 2 G 1 / 2 h1 / 2, c, , 1/2, , G, , 1 / 2 1 / 2, , h, , (b) c 1 / 2 G 1 / 2 h 1 / 2, (d) c 1 / 2 G 1 / 2 h1 / 2, , [UPSEAT 2002]

Page 12 :
Units, Dimensions and Measurement 55, 112., , Dimensions of charge are, (a), , 0, , 0, , M L T, , 1, , A, , 1, , [DPMT 2002], , (b), , MLTA, , 124., , 1, , (a), , 1, , 113., , (c) T A, (d) TA, According to Newton, the viscous force acting between liquid layers, v, of area A and velocity gradient v / z is given by F  A, z, where  is constant called coefficient of viscosity. The dimension of, [JIPMER 2001, 02],  are, (a) [ML2 T 2 ], , 114., , 115., , 116., , 117., , 125., , 118., , 126., , ML1 T 2 Q 2, , (b), , 127., , 128., , 129., , (a) [ML T , , ], , (c) [ML0 T 2 1 ], 121., , 130., , ML2 T 2 Q 2, , 132., , (a), 122., , M L T, , 134., , (d) [ML2 T 1 1 ], , (b), , M LT, , 135., , 136., , 137., [AFMC 2003; AIIMS 2005], , ML2 T 1, , (b), , ML2T 3 A 2, , (c), , ML1T 2, , (d) None of these, , ML2 T 2, , (c), , ML1 T 2, , (d), , ML1 T 1, , The dimension of, , R, are, L, , T2, , [MP PET 2003], , (b) T, , (c) T, (d) T 2, The dimensions of shear modulus are [MP PMT 2004], MLT 1, , (b), , ML2T 2, , (c) ML1T 2, (d) MLT 2, Pressure gradient has the same dimension as that of, (a) Velocity gradient, (b) Potential gradient, (c) Energy gradient, (d) None of these, If force (F), length (L) and time (T) are assumed to be fundamental, units, then the dimensional formula of the mass will be, FL1T 2, , (b), , FL1T 2, , (c) FL1T 1, (d) FL2T 2, The dimensions of universal gas constant is, (a) [ML2T 2 1 ], , (b) [M 2 LT 2 ], , (c) [ML3 T 1 1 ], , (d) None of these, , [Pb. PET 2003], , In the relation y  a cos(t  kx ) , the dimensional formula for k is, (c) [M 0 L1T 0 ], Position of a body, , (b) [M 0 LT 1 ], (d) [M 0 LT ], with acceleration 'a', , is given, , by, , [Orissa JEE 2005], [AIEEE 2003], , (a), , (b), , x  Ka m t n , here t is time. Find dimension of m and n., , 1, , (a) Speed and (0 0 )1 / 2, , 123., , (b) [MLT 2 Q 1 ], , MLT 1, , (a) [M 0 L1T 1 ], , (c) M 1 L1 T 2, (d) M 1 L0 T 1, The physical quantities not having same dimensions are, , (b) Torque and work, (c) Momentum and Planck's constant, (d) Stress and Young's modules, Dimension of R is, , (b) k  3 / 2a3 / 2 / T, , (a), , (a), 133., , (b) [ML2 T 2 ], , 0 1, , / T, , [AFMC 2004], , [Orissa JEE 2003], 2, , a, , [BHU 2003], , (c) [ML2 T 1 Q], (d) [ML2 T 2 Q], Dimensions of potential energy are, [MP PET 2003], , (a), , 1, The dimensions of K in the equation W  Kx 2 is, 2, 1 0, , k, , 1/2 3/2, , 1, , [MP PET 2002; Pb. PET 2001], 1, , M 0 LT 2, , (c) k  1 / 2 a 3 / 2 / T 3 / 4, (d) k  1 / 2 a1 / 2 / T 3 / 2, The dimensions of electric potential are, [UPSEAT 2003], , (a), , 131., , (a) Gravitational constant, (b) Planck's constant, (c) Power of a convex lens, (d) None, The dimensional formula for Boltzmann's constant is, 2, , (b), , (a) [ML2T 2Q 1 ], , MLT 1, , (c) MLT 2 Q 1, (d) ML2 T 2 Q 1, Which of the following quantities is dimensionless, , 2, , LT, , (c) MLT 2, (d) ML2T 2, Frequency is the function of density ( ) , length (a) and surface, (a), , [MP PET 2002], , 120., , ML1T 2, , tension (T ) . Then its value is, , (c) M 0 LT 1, (d) ML0 T 1, The dimensions of emf in MKS is, [CPMT 2002], (a), , 119., , (b), , (b), , ML, , [CPMT 2003], , 1, , [BHU 2003; CPMT 2004], , [Orissa JEE 2002], , ML1 T 1, , 3, , (c) MLT 2, (d) Dimensionless, The dimensional formula for young's modulus is, (a), , (b) [ML1 T 1 ], , (c) [ML2 T 2 ], (d) [M 0 L0 T 0 ], Identify the pair whose dimensions are equal, [AIEEE 2002], (a) Torque and work, (b) Stress and energy, (c) Force and stress, (d) Force and work, The dimensions of pressure is equal to, [AIEEE 2002], (a) Force per unit volume, (b) Energy per unit volume, (c) Force, (d) Energy, Which of the two have same dimensions, [AIEEE 2002], (a) Force and strain, (b) Force and stress, (c) Angular velocity and frequency, (d) Energy and strain, An object is moving through the liquid. The viscous damping force, acting on it is proportional to the velocity. Then dimension of, constant of proportionality is, (a), , The dimensional formula of relative density is, , (a), , m 1 , n 1, , (b) m  1, n  2, , (c), , m  2, n  1, , (d) m  2, n  2, , "Pascal-Second" has dimension of, [AFMC 2005], (a) Force, (b) Energy, (c) Pressure, (d) Coefficient of viscosity, In a system of units if force (F), acceleration (A) and time (T) are, taken as fundamental units then the dimensional formula of energy, is, [BHU 2005], (a), , FA2 T, , (b), , FAT 2

Page 13 :
56 Units, Dimensions and Measurement, , 138., , 139., , 140., , (c) F 2 AT, (d) FAT, Out of the following pair, which one does not have identical, dimensions, [AIEEE 2005], (a) Moment of inertia and moment of force, (b) Work and torque, (c) Angular momentum and Planck's constant, (d) Impulse and momentum, The ratio of the dimension of Planck's constant and that of moment, of inertia is the dimension of, [CBSE PMT 2005], (a) Frequency, (b) Velocity, (c) Angular momentum, (d) Time, Which of the following group have different dimension, , 6., , 7., , 8., , [IIT JEE 2005], , 141., , 142., , (a) Potential difference, EMF, voltage, (b) Pressure, stress, young's modulus, (c) Heat, energy, work-done, (d) Dipole moment, electric flux, electric field, Out of following four dimensional quantities, which one quantity is, to be called a dimensional constant, [KCET 2005], (a) Acceleration due to gravity, (b) Surface tension of water, (c) Weight of a standard kilogram mass, (d) The velocity of light in vacuum, Density of a liquid in CGS system is 0.625 g / cm 3 . What is its, magnitude in SI system, [J&K CET 2005], (a) 0.625, (b) 0.0625, (c) 0.00625, (d) 625, , 9., , 10., , The period of oscillation of a simple pendulum is given by, , 12., , 2., , l, where l is about 100 cm and is known to have 1mm, g, accuracy. The period is about 2s. The time of 100 oscillations is, measured by a stop watch of least count 0.1 s. The percentage error, in g is, (a) 0.1%, (b) 1%, (c) 0.2%, (d) 0.8%, The percentage errors in the measurement of mass and speed are, 2% and 3% respectively. How much will be the maximum error in, the estimation of the kinetic energy obtained by measuring mass, and speed, (a) 11%, (b) 8%, (c) 5%, (d) 1%, The random error in the arithmetic mean of 100 observations is x;, then random error in the arithmetic mean of 400 observations, would be, , 13., , T  2, , 5., , 14., , -1, , 0 .1  100, 0 .1, (d) 3 ,  100, 3 .53, 5 .3, A thin copper wire of length l metre increases in length by 2%, when heated through 10ºC. What is the percentage increase in area, when a square copper sheet of length l metre is heated through, 10ºC, (a) 4%, (b) 8%, (c) 16%, (d) None of the above, , (d), , 3, , In the context of accuracy of measurement and significant figures in, expressing results of experiment, which of the following is/are, correct, (1) Out of the two measurements 50.14 cm and 0.00025 ampere,, the first one has greater accuracy, (2) If one travels 478 km by rail and 397 m. by road, the total, distance travelled is 478 km., , 15., , 1, x, 4, , 1, x, 2, What is the number of significant figures in 0.310×10, (a) 2, (b) 3, (c) 4, (d) 6, Error in the measurement of radius of a sphere is 1%. The error in, the calculated value of its volume is [AFMC 2005], (a) 1%, (b) 3%, , (c) 2x, , 4., , (b), , -1, , -1, , (c), , 1., , (a) 4x, , -1, , -2, , 11., , Errors of Measurement, , 3., , (c) 5%, (d) 7%, The mean time period of second's pendulum is 2.00s and mean, absolute error in the time period is 0.05 s. To express maximum, estimate of error, the time period should be written as, (a) (2.00  0.01) s, (b) (2.00 +0.025) s, (c) (2.00  0.05) s, (d) (2.00  0.10) s, A body travels uniformly a distance of (13.8 0.2) m in a time (4.0,  0.3) s. The velocity of the body within error limits is, (a) (3.45  0.2) ms, (b) (3.45  0.3) ms, (c) (3.45  0.4) ms, (d) (3.45  0.5) ms, The percentage error in the above problem is, (a) 7%, (b) 5.95%, (c) 8.95%, (d) 9.85%, The unit of percentage error is, (a) Same as that of physical quantity, (b) Different from that of physical quantity, (c) Percentage error is unit less, (d) Errors have got their own units which are different from that, of physical quantity measured, The decimal equivalent of 1/20 upto three significant figures is, (a) 0.0500, (b) 0.05000, (c) 0.0050, (d) 5.0 × 10, Accuracy of measurement is determined by, (a) Absolute error, (b) Percentage error, (c) Both, (d) None of these, The radius of a sphere is (5.3  0.1) cm. The percentage error in its, volume is, 0.1, 0 .1, (a), (b) 3 ,  100,  100, 5 .3, 5 .3, , (a) Only (1) is correct, , (b) Only (2) is correct, , (c) Both are correct, , (d) None of them is correct., , A physical parameter a can be determined by measuring the, parameters b, c, d and e using the relation a = b  c  / d  e  ., If the maximum errors in the measurement of b, c, d and e, are b 1 %, c 1 %, d 1 % and e 1 %, then the maximum error in, the value of a determined by the experiment is, (a) ( b1  c1  d 1  e 1 )%, (b) ( b1  c1  d 1  e 1 )%, (c) ( b1  c1  d 1  e 1 )%

Page 14 :
Units, Dimensions and Measurement 57, (d) ( b1  c1  d 1  e 1 )%, 16., , 17., , The relative density of material of a body is found by weighing it, first in air and then in water. If the weight in air is (5.00 0.05 ), Newton and weight in water is (4.00  0.05) Newton. Then the, relative density along with the maximum permissible percentage, error is, (a) 5.0  11%, , (b) 5.0  1%, , (c) 5.0  6%, , (d) 1.25  5%, , (c) 5.2%, , 19., , 21., , 22., , 27., , (d), , (c), , 5, %, 2, , 28., , (a) 0.1 s, , (b) 0.11 s, , (c) 0.01 s, , (d) 1.0 s, , , , , , b, , , , , , c, , , , (d) None of these, , A physical quantity A is related to four observable a, b, c and d as, a 2b 3, , , the percentage errors of measurement in, , c d, a, b, c and d are 1%,3%,2% and 2% respectively. What is the, percentage error in the quantity A, [Kerala PET 2005], , (a) 12%, (c) 5%, , (b) 7%, (d) 14%, , (b) 2%, , (c) 3%, (d) 4%, In an experiment, the following observation's were recorded : L =, 2.820 m, M = 3.00 kg, l = 0.087 cm, Diameter, D = 0.041 cm, 4, MgL, Taking g = 9.81 m /s 2 using the formula , Y=, , the maximum, D 2 l, permissible error in Y is, (a) 7.96%, (b) 4.56%, (c) 6.50%, (d) 8.42%, According to Joule's law of heating, heat produced H  I 2 Rt,, where I is current, R is resistance and t is time. If the errors in the, measurement of I, R and t are 3%, 4% and 6% respectively then, error in the measurement of H is, (a)  17%, (b)  16%, (c)  19%, (d)  25%, If there is a positive error of 50% in the measurement of velocity of, a body, then the error in the measurement of kinetic energy is, (a) 25%, (b) 50%, (c) 100%, (d) 125%, A physical quantity P is given by P=, , A3 B 2, 3, D2, , 1., , (b) 4.43 cm, (d) 4 cm, , If the acceleration due to gravity is 10 ms 2 and the units of, length and time are changed in kilometer and hour respectively, the, numerical value of the acceleration is, [Kerala PET 2002], , 2., , 3., , (a) 360000, (b) 72,000, (c) 36,000, (d) 129600, If L, C and R represent inductance, capacitance and resistance, respectively, then which of the following does not represent, dimensions of frequency, [IIT 1984], 1, R, (a), (b), RC, L, 1, C, (c), (d), L, LC, Number of particles is given by n   D, , (a), , M 0 LT 2, , 4., , (b), , M 0 L2 T 4, , (c) M LT, (d) M 0 L2 T 1, With, the, usual, notations,, the, following, 1, S t  u  a(2 t  1) is, 2, (a) Only numerically correct, (b) Only dimensionally correct, (c) Both numerically and dimensionally correct, (d) Neither numerically nor dimensionally correct, 0, , [DCE 2003], , n 2  n1, crossing a unit, x 2  x1, , area perpendicular to X-axis in unit time, where n1 and n 2 are, number of particles per unit volume for the value of x meant to, x 2 and x 1 . Find dimensions of D called as diffusion constant, , . The quantity which, , C, brings in the maximum percentage error in P is, (a) A, (b) B, (c) C, (d) D, If L  2.331 cm, B  2.1 cm , then L  B , , (a) 4.431 cm, (c) 4.4 cm, , a, , follows, A , , The length of a cylinder is measured with a meter rod having least, count 0.1 cm. Its diameter is measured with vernier calipers having, least count 0.01 cm. Given that length is 5.0 cm. and radius is 2.0, cm. The percentage error in the calculated value of the volume will, be, , 4, , 24., , (b) 0.94  0.01 cm, (d) 0.94  0.005 cm, , A physical quantity is given by X  M a Lb T c . The percentage error, in measurement of M, L and T are  ,  and  respectively. Then, maximum percentage error in the quantity X is, (a) a  b  c, (b) a  b  c, , (b) 7%, , 1, , 23., , 2009, 4.156 and 1.217  10 4 is[Pb. PET 2003], (a) 1, (b) 2, (c) 3, (d) 4, If the length of rod A is 3.25  0.01 cm and that of B is 4.19  0.01, cm then the rod B is longer than rod A by, [J&K CET 2005], , The period of oscillation of a simple pendulum in the experiment is, recorded as 2.63 s, 2.56 s, 2.42 s, 2.71 s and 2.80 s respectively. The, average absolute error is, , (a) 1%, 20., , 26., , The number of significant figures in all the given numbers 25.12,, , (a) 0.94  0.00 cm, (c) 0.94  0.02 cm, , V, The resistance R =, where V= 100  5 volts and i = 10  0.2, i, amperes. What is the total error in R, (a) 5%, , 18., , 25., , 3, , equation

Page 15 :
58 Units, Dimensions and Measurement, 5., , If the dimensions of length are expressed as G x c y h z ; where G, c, , 6., , and h are the universal gravitational constant, speed of light and, Planck's constant respectively, then, [IIT 1992], 1, 1, 1, 1, (a) x  , y , (b) x  , z , 2, 2, 2, 2, 1, 3, 3, 1, (c) y  , z , (d) y   , z , 2, 2, 2, 2, A highly rigid cubical block A of small mass M and side L is, fixed rigidly onto another cubical block B of the same dimensions, and of low modulus of rigidity  such that the lower face of A, completely covers the upper face of B . The lower face of B is, rigidly held on a horizontal surface. A small force F is applied, perpendicular to one of the side faces of A . After the force is, withdrawn block A executes small oscillations. The time period of, which is given by, , 7., , 8., , 9., , M, L, , (c) 2, , ML, , 11., , (d) h 1 / 2 c 3 / 2 G 1 / 2, , X  3YZ 2 find dimension of Y in (MKSA) system, if X and Z, are the dimension of capacity and magnetic field respectively, 3, , 2, , 4, , (a), , M L T, , (c), , M 3 L2 T 4 A 4, , A, , 1, , 2, , (b), , ML, , (d), , M 3 L2 T 8 A 4, , Z, , e k, , , P is pressure, Z is the distance, k is, , Boltzmann constant and  is the temperature. The dimensional, formula of  will be, [IIT (Screening) 2004], In the relation P , , 0 2, , 0, , (a) [M L T ], (c), , [M 1 L0 T 1 ], , 15., , (a), (b), (c), (d), (e), 1., , 2., , 3., 4., , 1/2, , 12., , 13., , (b) [ML0 T 1 ], , (c) [ML1T 0 ], Column I, , (d) [M 0 L0 T 0 ], Column II, , (J), , LT 1, [IIT 1992], , A wire has a mass 0.3  0.003 g , radius 0.5  0.005 mm and, length 6  0.06 cm . The maximum percentage error in the, measurement of its density is, [IIT (Screening) 2004], (a) 1, (b) 2, (c) 3, (d) 4, If 97.52 is divided by 2.54, the correct result in terms of significant, figures is, (a) 38.4, (b) 38.3937, (c) 38.394, (d) 38.39, , 5., 6., , If both assertion and reason are true and the reason is the correct, explanation of the assertion., If both assertion and reason are true but reason is not the correct, explanation of the assertion., If assertion is true but reason is false., If the assertion and reason both are false., If assertion is false but reason is true., [MP PMT, Assertion, : 2003], ‘Light year’ and ‘Wavelength’ both measure, distance., , Reason, , :, , Both have dimensions of time., , Assertion, , :, , Light year and year, both measure time., , Reason, , :, , Assertion, , :, , Because light year is the time that light takes to, reach the earth from the sun., Force cannot be added to pressure., , Reason, , :, , Because their dimensions are different., , Assertion, , :, , Linear mass density has the dimensions of [M L T ]., , Reason, , :, , Because density is always mass per unit volume., , Assertion, , :, , Rate of flow of a liquid represents velocity of flow., , Reason, , :, , The dimensions of rate of flow are [M L T ]., , Assertion, , :, , Units of Rydberg constant R are m, , Reason, , :, ,  1, 1, It follows from Bohr’s formula v  R  2  2, n,  1 n2, , [BHU 2004], , (a) [M 0 LT 1 ], , MLT 3 I 1, , Choose any one of the following four responses :, , (b) [M L T ], , p F, The frequency of vibration of string is given by  , ., 2l  m , Here p is number of segments in the string and l is the length. The, dimensional formula for m will be, , (I), , [AMU 1995], , 1 2 1, , (d) [M 0 L2T 1 ], , (E) ML2T 2, , Choose the correct match, (a) (i) G, (ii) H, (iii) C, (iv) B, (v) C, (b) (i) D, (ii) H, (iii) I, (iv) B, (v) G, (c) (i) G, (ii) H, (iii) I, (iv) B, (v) G, (d) None of the above, , M, 2, L, , h1 / 2 c 3 / 2 G 1 / 2, , (v) Decibel, , (G) T 1, (H) L, , 14., , (d), , The pair(s) of physical quantities that have the same dimensions, is, (are), [IIT 1995], (a) Reynolds number and coefficient of friction, (b) Latent heat and gravitational potential, (c) Curie and frequency of a light wave, (d) Planck's constant and torque, The speed of light (c) , gravitational constant (G) and Planck's, constant (h) are taken as the fundamental units in a system. The, dimension of time in this new system should be, (a) G 1 / 2 h1 / 2 c 5 / 2, (b) G 1 / 2 h1 / 2 c 1 / 2, 1 / 2 1 / 2 3 / 2, (c) G h c, (d) G 1 / 2 h1 / 2 c 1 / 2, If the constant of gravitation (G) , Planck's constant (h) and the, velocity of light (c) be chosen as fundamental units. The dimension, of the radius of gyration is, [AMU (Eng.) 1999], 1 / 2 3 / 2 1 / 2, G, (a) h c, (b) h1 / 2 c 3 / 2 G 1 / 2, (c), , 10., , L, M, , (b) 2, , (A) MLT 2, (B) M, (C) Dimensionless, (D) T, (F) MT 3, , [IIT 1992], , (a) 2, , (i) Curie, (ii) Light year, (iii) Dielectric strength, (iv) Atomic weight, , 1, , 0, , 1, , –1, , 0, , –1, , –1, , where the symbols have their usual meaning., , , ,, , 

Page 16 :
Units, Dimensions and Measurement 59, 7., , Assertion, , : Parallex method cannot be used for measuring, distances of stars more than 100 light years away., , Reason, , : Because parallex angle reduces so much that it, cannot be measured accurately., , 8., , Assertion, , :, , 9., , Reason, Assertion, , Reason, , : This is because zeros are not significant., : Out of three measurements l = 0.7 m;, l, = 0.70 m and l = 0.700 m, the last one is most, accurate., : In every measurement, only the last significant digit, is not accurately known., : Mass, length and time are fundamental physical, quantities., : They are independent of each other., : Density is a derived physical quantity., : Density cannot be derived from the fundamental, physical quantities., : Now a days a standard metre is defined as in terms, of the wavelength of light., : Light has no relation with length., : Radar is used to detect an aeroplane in the sky, : Radar works on the principle of reflection of waves., : Surface tension and surface energy have the same, dimensions., : Because both have the same S.I. unit, , 15., , Assertion, , : In y  A sin( t  kx ), (t  kx ) is dimensionless., , 16., , Reason, Assertion, Reason, , : Because dimension of   [M 0 L0 T ]., : Radian is the unit of distance., : One radian is the angle subtended at the centre of, a circle by an arc equal in length to the radius of, the circle., : A.U. is much bigger than Å., : A.U. stands for astronomical unit and Å stands, from Angstrom., : When we change the unit of measurement of a, quantity, its numerical value changes., : Smaller the unit of measurement smaller is its, numerical value., : Dimensional constants are the quantities whose, value are constant., : Dimensional constants are dimensionless., : The time period of a pendulum is given by the, , Reason, 10., , Assertion, , 11., , Reason, Assertion, Reason, , 12., , 13., 14., , 17., , 18., , Assertion, Reason, Assertion, Reason, Assertion, , Assertion, Reason, Assertion, Reason, , 19., , Assertion, , 20., , Reason, Assertion, , Number of significant figures in 0.005 is one and, that in 0.500 is three., , formula, T  2 g/l ., Reason, , 21., , 22., , 23., , : According to the principle of homogeneity of, dimensions, only that formula is correct in which, the dimensions of L.H.S. is equal to dimensions of, R.H.S., 1 T, , where symbols have, 2l m, standard meaning, m represent linear mass density., The frequency has the dimensions of inverse of, time., The graph between P and Q is straight line, when, P/Q is constant., The straight line graph means that P proportional, to Q or P is equal to constant multiplied by Q., Avogadro number is the number of atoms in one, gram mole., , Assertion, , : In the relation f , , Reason, , :, , Assertion, , :, , Reason, , :, , Assertion, , :, , 24., , Reason, Assertion, Reason, , :, :, :, , Avogadro number is a dimensionless constant., L/R and CR both have same dimensions., L/R and CR both have dimension of time., , 25., , Assertion, , :, , The quantity (1/  0  0 ) is dimensionally equal to, velocity and numerically equal to velocity of light., , Reason, , :, ,  0 is permeability of free space and  0 is the, permittivity of free space., , Units, 1, , c, , 2, , b, , 3, , d, , 4, , c, , 5, , c, , 6, , d, , 7, , c, , 8, , d, , 9, , c, , 10, , c, , 11, , a, , 12, , c, , 13, , c, , 14, , b, , 15, , d, , 16, , d, , 17, , c, , 18, , a, , 19, , b, , 20, , d, , 21, , d, , 22, , a, , 23, , a, , 24, , b, , 25, , d, , 26, , b, , 27, , d, , 28, , d, , 29, , d, , 30, , b, , 31, , a, , 32, , b, , 33, , a, , 34, , b, , 35, , a, , 36, , b, , 37, , a, , 38, , b, , 39, , b, , 40, , b, , 41, , d, , 42, , c, , 43, , c, b, , 44, , c, , 45, , b, , 46, , a, , 47, , c, , 48, , c, , 49, , a, , 50, , a, , 51, , b, , 52, , b, , 53, , c, , 54, , c, , 55, , c, , 56, , c, , 57, , b, , 58, , a, , 59, , c, , 60, , a, , 61, , c, , 62, , c, , 63, , d, , 64, , d, , 65, , b, , 66, , c, , 67, , a, , 68, , b, , 69, , c, , 70, , b, , 71, , d, , 72, , b, , 73, , b, , 74, , d, , 75, , c, , 76, , b, , 77, , b, , 78, , b, , 79, , c, , 80, , c, , 81, , a, , 82, , a, , 83, , d, , 84, , c, , 85, , b, , 86, , d, , 87, , d, , 88, , b, , 89, , a, , 90, , c, , 91, , a, , 92, , d, , 93, , b, , 94, , a, , 95, , d, , 96, , a, , 97, , b, , 98, , a, , 99, , d, , 100, , b, , 101, , d, , 102, , d, , 103, , a, , 104, , a, , 105, , d, , 106, , b, , 107, , b, , 108, , b, , 109, , b, , Dimensions, 1, , a, , 2, , c, , 3, , b, , 4, , a, , 5, , b, , 6, , c, , 7, , c, , 8, , b, , 9, , ad, , 10, , a, , 11, , d, , 12, , b, , 13, , a, , 14, , a, , 15, , a, , 16, , b, , 17, , b, , 18, , d, , 19, , a, , 20, , c, , 21, , b, , 22, , a, , 23, , b, , 24, , d, , 25, , a, , 26, , d, , 27, , a, , 28, , d, , 29, , d, , 30, , d, , 31, , c, , 32, , c, , 33, , a, , 34, , a, , 35, , b, , 36, , b, , 37, , c, , 38, , c, , 39, , a, , 40, , b, , 41, , a, , 42, , b, , 43, , d, , 44, , d, , 45, , a

Page 17 :
60 Units, Dimensions and Measurement, 46, , d, , 47, , b, , 48, , d, , 49, , b, , 50, , a, , 51, , a, , 52, , d, , 53, , b, , 54, , b, , 55, , c, , 56, , c, , 57, , d, , 58, , a, , 59, , a, , 60, , c, , 61, , b, , 62, , b, , 63, , c, , 64, , a, , 65, , a, , 66, , b, , 67, , a, , 68, , d, , 69, , c, , 70, , a, , 71, , a, , 72, , c, , 73, , c, , 74, , a, , 75, , b, , 76, , d, , 77, , a, , 78, , a, , 79, , b, , 80, , b, , 81, , d, , 82, , b, , 83, , bc, , 84, , c, , 85, , d, , 86, , d, , 87, , c, , 88, , a, , 89, , a, , 90, , a, , 91, , a, , 92, , b, , 93, , b, , 94, , a, , 95, , b, , 96, , a, , 97, , a, , 98, , a, , 99, , c, , 100, , a, , 101, , d, , 102, , b, , 103, , b, , 104, , d, , 105, , c, , 106, , d, , 107, , c, , 108, , c, , 109, , a, , 110, , b, , 111, , c, , 112, , d, , 113, , b, , 114, , a, , 115, , b, , 116, , c, , 117, , d, , 118, , d, , 119, , d, , 120, , a, , 121, , a, , 122, , c, , 123, , b, , 124, , d, , 125, , a, , 126, , a, , 127, , a, , 128, , b, , 129, , c, , 130, , c, , 131, , d, , 132, , a, , 133, , a, , 134, , c, , 135, , b, , 136, , d, , 137, , b, , 138, , a, , 139, , a, , 140, , d, , 141, , d, , 142, , d, , Errors of Measurement, 1, , c, , 2, , b, , 3, , b, , 4, , b, , 5, , b, , 6, , c, , 7, , b, , 8, , c, , 9, , c, , 10, , a, , 11, , b, , 12, , b, , 13, , a, , 14, , c, , 15, , d, , 16, , a, , 17, , b, , 18, , b, , 19, , c, , 20, , c, , 21, , b, , 22, , d, , 23, , c, , 24, , c, , 25, , d, , 26, , c, , 27, , a, , 28, , d, , Critical Thinking Questions, 1, , d, , 2, , d, , 3, , d, , 4, , c, , 5, , bd, , 6, , d, , 7, , abc, , 8, , a, , 9, , a, , 10, , d, , 11, , a, , 12, , c, , 13, , a, , 14, , d, , 15, , a, , Assertion and Reason, 1, , c, , 2, , d, , 3, , a, , 4, , c, , 5, , d, , 6, , a, , 7, , a, , 8, , c, , 9, , b, , 10, , a, , 11, , c, , 12, , c, , 13, , a, , 14, , c, , 15, , c, , 16, , e, , 17, , b, , 18, , c, , 19, , c, , 20, , e, , 21, , b, , 22, , a, , 23, , c, , 24, , a, , 25, , b

Page 18 :
Units, Dimensions and Measurement, 27., , (d), , 28., , (d), , 29., , (d), , 30., , (b) Surface tension =, , Units, , 31., , (a), , 1., 2., 3., 4., , (c) Light year is a distance which light travels in one year., (b) Because magnitude is absolute., (d) Watt=Joule/second = Ampere×volt = Ampere ×Ohm, (c) Impulse = change in momentum = F × t, So the unit of momentum will be equal to Newton-sec., , 32., , (b), , 33., , (a), , 5., , (c) Unit of energy will be kg - m 2 /sec 2, , 34., , 6., , (d) It is by standard definition., , 7., , (c) 1 nm  10 9 m  10 7 cm, , 35., , 8., , (d) 1 micron  10 6 m  10 4 cm, , 36., , (b) 1 MeV  10 6 eV, , 9., , (c) Watt = Joule/sec., , 37., , (a) Energy (E) = F × d  F , , 2, , F, , Gm 1 m 2, , Fd 2,  Nm 2 / kg 2, ;  G, m1m 2, , 10., , (c), , 11., , (a), , 12., , (c) Angular acceleration , , 13., , E, (c) Stefan's law is E   (T 4 )    4, T, , d2, , Angular velocity rad, , Time, sec 2, , Energy, where, E , Area  Time, , Watt, , m2, , Watt - m 2,  W att m 2 K 4, K4, (b) Kg-m/sec is the unit of linear momentum, , , , 14., 15., , (d) ct, , 2, , must have dimensions of L, ,  c must have dimensions of L / T 2 i.e. LT 2 ., , 17., , dL, (d)  ,  dL    dt  r  F  dt, dt, i.e. the unit of angular momentum is joule-second., (c), , 18., , (a) Volume of cube = a 3, , 16., , E, , L, , , I, , 61, , dV, dx, , , , Force, = Newtons / metre, Length, , Wb,  Henry ., A, , L, is a time constant of L-R circuit so Henry/ohm can be, R, expressed as second., ,  m , (b) mv  kg , ,  sec , (a) Quantities of similar dimensions can be added or subtracted so, unit of a will be same as that of velocity., , E, so Erg/metre can be the unit of, d, , force.,  cm, (b) Potential energy  mgh  g,  sec 2, , 39., , (b), , 40., 41., 42., 43., 44., , (b), (d), (c), (b,c), (c) Energy = force  distance, so if both are increased by 4 times, then energy will increase by 16 times., , 45., 46., , (b) 1 Oerstead = 1 Gauss = 10 4 Tesla, (a) Charge = current  time, L, RA, (c) R     ,  ohm  cm, A, L, (c), (a) Astronomical unit of distance., (a) Physical quantity (p) = Numerical value (n)  Unit (u), If physical quantity remains constant then n  1/u  n u = n u, ., , 47., 48., 49., 50., , ,  cm , cm  g, , ,  sec , , 2, , 38., , watt,  volt, ampere, , 1, , Surface area of cube = 6a 2, according to problem a = 6a  a = 6, 3, , 2, ,  V  a  216 units., 3, , 19., , (b) 6  10 5  60  10 6  60 microns, , 20., 21., 22., 23., , (d), (d) Because temperature is a fundamental quantity., (a), (a) 1 C.G.S unit of density = 1000 M.K.S. unit of density,  0.5 gm/cc  500 kg /m 3, , 24., 25., , (b), (d), , 26., , (b) Mach number , , 51., , (b) 1 eV  1.6  10 19 coulomb  1 volt  1.6  10 19 J ., , 52., 53., 54., , (b) 1 kW h  1  10 3  3600 W  sec  36  10 5 J, (c) According to the definition., (c), , 55., , (c) As I  MR 2  kg  m 2, , 56., , (c), , 57., , (b), , 58., , Stress , , Force, N, = 2, Area, m, , Q,  AT 4    Jm  2 s 1 K  4, t, (a) M = Pole strength  length, , = amp  metre  metre  amp  metre 2, Velocityof object, ., Velocityof sound, , 59., 60., , (c) Curie = disintegration/second, (a), , 61., , (c) Pico prefix used for 10 12, , 1, , 2, , 2

Page 19 :
62 Units, Dimensions and Measurement, 62., 63., 64., 65., , (c), (d) Unit of e.m. f . = volt = joule/coulomb, (d), (b), , 66., , (c), , Y , , dyne, 10 5 N, F L, =, ,  0.1 N / m 2, ., A L, cm 2 10  4 m 2, , 67., , (a), , Y , , Stress, Force/Area, ,  Y  Pressure ., Strain Dimensionless, , 101., 102., , 1 q1 q 2, 1 q1 q 2, ,  C 2 m  2 N 1, 4  r 2, 4 Fr2, (d) Joule-sec is the unit of angular momentum where as other, units are of energy., , (d), , F, , 104., , F,  Nm 1, l, (a) Because in S.I. system there are seven fundamental quantities., , 105., , (d) []  ML1 T 1 so its unit will be kg/m-sec., , 106., 107., 108., 109., , (b), (b), (b) According to the definition., (b) Pyrometer is used for measurement of temperature., , 103., , (a), , T, , 68., , (b) 1 yard  36 inches  36  2.54 cm  0.9144m ., , 69., , (c) 1 fermi  10 -15 metre, , 70., 71., 72., 73., 74., , (b), (d), (b), (b), (d) 1 Newton = 10 Dyne, , 75., 76., 77., 78., , (c) [x ]  [bt 2 ]  [b]  [x / t 2 ]  km / s 2, (b) Units of a and PV are same and equal to dyne × cm ., (b), (b), , 79., , (c) Impulse = Force  time  (kg-m/s 2 )  s  kg-m/s, , 80., 81., 82., 83., 84., 85., 86., , (c), (a) K  C  273.15, (a), (d), (c), (b), (d) Watt is a unit of power, , 2., , (c) Strain , , L,  dimensionless quantity, L, , 3., , (b) Power , , Work ML2 T 2, ,  ML2 T 3, Time, T, , 4., , (a) Calorie is the unit of heat i.e., energy., , 87., , (d) 1 lightyear  9.46  1015 meter, , 5., , (b) Angular momentum = mvr  MLT 1  L  ML2 T 1, , 88., , (b), , 6., , (c), , 89., , (a), , 7., , (c) Impulse = change in momentum so dimensions of both, quantities will be same and equal to MLT, , Dimensions, , 5, , 2, , V, , 1., , (a) Pressure , , 4, , Stress , , Force,  ML1 T  2, Area, , Restoring force,  ML1 T 2, Area, , So dimensions of energy  ML2 T 2, , Joule, W, so, SI unit =, m, kg, , L, = Time constant, R, –1, , 90., , (c), , M, n 2  n1  1,  M2, , , , , , , 1, ,  L1, , L,  2, ,  T, , T,  2, , , , , , ,  gm   cm   sec ,  , = 100,  , ,  kg   m   min , , 2, , 1, , , , , , , 1, , 1, , 1, , 2, ,  gm   cm   sec ,   2, = 100 3,  , , ,  10 gm   10 cm   60 sec , 1, , 8., , (b), , ∵ [R]  [ML2 T 3 I 2 ] and [C]  [M 1 L2 T 4 I 2 ], 9., , (a,d) [Torque] = [work] = [ML T ], 2, , [Light year] = [Wavelength] = [L], 10., , (a), , 3600,  3 .6, 10 3, [L/R] is a time constant so its unit is Second., , 92., 93., 94., , (d) Poission ratio is a unitless quantity., (b), (a), , 95., 96., 97., 98., 99., 100., , 1, (d) P  nu  n , u, (a) 1 Faraday = 96500 coulomb., (b), (a), (d), (b), , Q  mL  L , , =, , 2, , (a), , –2, , 2, , n=, 91., , RC  T, , 11., , ML2 T 2,  [M 0 L2 T 2 ], M, , Force/Area, Volume strain, Strain is dimensionless, so, , (d) Volume elasticity =, , =, 12., , Q, (Heat is a form of energy), m, , (b), , Force MLT 2, ,  [ML1 T 2 ], Area, L2, , F, , Gm1 m 2, d, ,  [G] , , 2, , G, , Fd 2, m1m 2, , [MLT 2 ][L2 ],  [M 1 L3 T  2 ], [M 2 ]

Page 20 :
Units, Dimensions and Measurement, , Now comparing the dimensions of quantities in both sides we, 1, 1, get x  y  0 and 2y  1  x   , y , 2, 2, , [M 0 L0 T 0 ], ,  [T 1 ], , [] , t, [T ], , 13., , (a) Angular velocity =, , 14., , (a) Power =, , 15., , (a) Couple = Force  Arm length = [MLT 2 ][L] [ML2 T 2 ], , 16., , (b) Angular momentum = mvr, , Work done  ML2 T 2 , 2 3, ,   [ML T ], Time,  T, , , 31., , (c), , (b) Impulse = Force  Time = [MLT 2 ][T ]  [MLT 1 ], , 18., , (d) Modulus of rigidity =, , 19., , (a), , 20., , (c), , 21., , (b) Moment of inertia  mr 2  [M ] [L2 ], , Shear stress,  [ML1 T 2 ], Shear strain, , [ A] [MLT 2 ], ,  [L2 T 2 ], [m ], [ML1 ], This is same dimension as that of latent heat., , = [MLT 2 ][L] [ML2 T 2 ], 22., , (a) Momentum = mv  [MLT 1 ], Impulse = Force  Time = [MLT 2 ]  [T ]  [MLT 1 ], (b) Pressure =, , 24., , (d) [h]  [Angularmomentum]  [ML2 T 1 ], , 25., ,  a , (a) By principle of dimensional homogenity  2   P , V , ,  [a]  [P] [V 2 ]  [ML1 T 2 ]  [L6 ] = [ML5 T 2 ], (d), , 1, CV 2  Stored energy in a capacitor = [ML2 T 2 ], 2, , 27., , (a), , 1 2, Li = Stored energy in an inductor = [ML2 T 2 ], 2, , 28., , (d) Energy per unit volume =, , (a) Farad, , 34., , (a), , 35., , x, (b) From the principle of homogenity   has dimensions of T., v, , 36., , (b), , is, the, unit, of, capacitance, [Q], Q, = M 1 L2 T 2 Q 2, C =, V [ML2 T  2 Q 1 ], , [ML2 T 2 ],  [ML1 T  2 ], [L3 ], , , , dQ,  d ,   KA , , dt,  dx , ,  [K] =, , [ML2 T 2 ], [L],  2, = MLT 3 K 1, [T ], [L ] [K ], , Force [MLT 2 ], ,  [ML1 T  2 ], Area, [L2 ], , 37., , (c) Stress =, , 38., , (c), , 39., ,  Q   Q2, (a) [C] =    , V  W, , 40., , (b) Momentum = mv = [MLT 1 ], , 41., , (a), , Q  [ML2 T 2 ] (All energies have same dimension), , 42., , (b), , f, , 1, ,   A 2T 2 , 1  2 4 2, ,   ML2 T  2   [M L T A ],  , , ,  LC , , 1, ,  [M 0 L0 T 2 ], , 2 LC, (d) Energy = Work done [Dimensionally], , 44., , (d), , V Q, VIt, Power  Time, , , Volume Volume, Volume, , 45., , [ML2 T 3 ][T ],  [ML1 T 2 ], [L3 ], , [ML2 T 1 ],  [L2 T 1 ], Angular momentum per unit mass =, [M ], So angular momentum per unit mass has different dimension., , (d) Time constant   [T ] and Viscosity   [ML1 T 1 ], For options (a), (b) and (c) dimensions are not matching with, time constant., (d) By putting the dimensions of each quantity both the sides we, , and, , RA, i.e. dimension of resistivity is [M L3 T 1Q 2 ], l, , 43., , , , 30., , 33., , [MLT 2 ],  [ML1 T  2 ], Force per unit area =, [L2 ], Product of voltage and charge per unit volume, , , 29., , (c) Let v x  kg y z   . Now by substituting the dimensions of, each quantities and equating the powers of M, L and T we get,   0 and x  2, y  1, z  1 ., , Force, Energy, = ML1 T 2, , Area, Volume, , 23., , 26., , 32., , E  hv  [ML2 T 2 ]  [h][T 1 ]  [h]  [ML2 T 1 ], , Moment of Force = Force  Perpendicular distance, , M , m = linear density = mass per unit length =  , L, , A= force = [MLT 2 ]  [B]=, ,  [MLT 1 ][L]  [ML2 T 1 ], , 17., , 63, , f2, , L,  Time constant., R, (a) By substituting the dimension of each quantity we get, , T  [ML1 T 2 ]a [L3 M ]b [MT 2 ]c, , By solving we get a = – 3/2, b = 1/2 and c = 1, 46., , (d), , 47., , (b) v  g p h q (given), By substituting the dimension of each quantity and comparing, the powers in both sides we get [LT 1 ]  [LT 2 ] p [L]q,  p  q  1,  2 p  1, p , , 48., , (d) [Planck constant] = [ML2 T 1 ] and, [Energy] = [ML2 T 2 ], , get [T 1 ]  [M ]x [MT 2 ]y, 49., , (b) Frequency , , 1,  [M 0 L0 T 1 ], T, , 1, 1, ,q , 2, 2

Page 21 :
64 Units, Dimensions and Measurement, 50., 51., , Power =, , 73., , (c), , L, T, L 1, , ,  [ A 1 ], RCV  R  CV Q, ,  mg   M  LT 2 , 1, (a) we get ,  = [LT ] ., , 1 1,  r   ML T  L , This option gives the dimension of velocity., , 74., , (a), , Angularmomentum, mvr, ,  r  [M 0 L1 T 0 ], Linear momentum, mv, , 75., , (b) Dimension of work and torque = [ML2 T 2 ], , 76., , (d) Surface tension =, , 77., , (a) Linear momentum = Mass  Velocity = [MLT 1 ], , Energy, Time, (a) By substituting dimension of each quantity in R.H.S. of option, , (a), , 52., , (d) [  0 L ] = [C]  X , , 53., , (b) C , , 1, , 0 0, ,  0 LV, t, , CV Q, ,  = current, t, t, ,  1 ,   0  0   2  (where C = velocity of light), C , ,  [ 0  0 ]  L2 T 2, 54., , (b), , 55., , M , (c) [X] = [F] × [] = [MLT  2 ]   3   [M 2 L 2 T  2 ], L , , 56., , 1, 1, , , (c) Both are the formula of energy .  E  CV 2  LI 2 , 2, 2, , , , 57., , (d) Acceleration =, 1, , 78., , (a), , 79., , (b), ,  [ML2 T 1 ]  k[M z Lx  y  z T  x  2 y  2 z ], z 1, , 59., , (a) According to problem muscle × speed = power, , 60., , power, ML2 T 3,  muscle =, = MLT 2, , speed, LT 1, (c), , 61., , (b) Wave number =, , 62., 63., , (b) [Pressure] =[stress] = [ML1 T 2 ], (c),  2I I l, (a) F  0 1 2   0  [F][ A]2  [MLT 2 A 2 ], 4 r, , (b) By substituting the dimension of, [ML1T 2 ]x [MT 3 ]y [LT 1 ]z  [MLT ]0, x  z  0, ,  x  2y  2 z  1, , …(iii), , [L]  [Fv3 A 2 ], , 80., , given, , quantities, , By comparing the power of M, L, T in both sides x  y  0, 2 x  3 y  z  0, The only values of, corresponds to (b)., , …(i), …(ii), , So dimension of L in terms of v, A and f, , F, [MLT 2 ] [L2 ], A,  [ML2 T  2 A 1 ], IL, [ A] [L], , 66., , x yz  2, , On solving (i), (ii) and (iii) x  3, y  2, z  1, , 1,  dimension is [M 0 L1 T 0 ], , , (a)   BA , , L  v x A y F z  L  kv x A y F z, Putting the dimensions in the above relation, , [ML2T 1 ]  k[LT 1 ]x [LT 2 ]y [MLT 2 ]z, ,  C  velocity of light, , 65., , R, V/I, 1, ,   Frequency, L V T / I T, , Comparing the powers of M, L and T, , (a), , 64., , Moment of a force = Force  Distance = [ML2T 2 ], , distance,  A  LT 2  L  AT 2, time 2, , 58., ,  0 0, , Force, [MLT 2 ], ,  [MT  2 ], Length, L, , .....(ii), …(iii), x , y, z satisfying (i), (ii) and (iii), , (b), , F, , 1 q1q 2, 4 0 r 2, ,  0 , , | q1 | | q 2 |, [ A 2T 2 ], ,  [ A 2 T 4 M 1 L 3 ], 2, [F] [r ], [MLT  2 ] [L2 ], , 81., 82., 83., , (d) [Pressure] = [Stress] = [coefficient of elasticity] = [ML1 T 2 ], (b), (b, c), , 84., , (c) Capacity  Resistance =, , Charge, Volt, , Potential amp, , amp  second  Volt, =,  Second, Volt  amp, .....(i), 85., (d) Strain has no dimensions., 86., (d), , 87., , (c), , B, , F [MLT 2 ], ,  [MT  2 A 1 ], IL, [ A] [L], , 88., , (a)  , , 68., , 1 2, Li hence L  [ML2T 2 A 2 ], 2, (d) Strain is dimensionless., , F, [MLT 2 ], ,  [ML1 T 1 ], av [L][LT 1 ], , 69., , (c) Dimensions of power is [ML2 T 3 ], , 89., , 70., , (a) Kinetic energy =, , 71., , (a) Torque = force  distance = [ML T ], , (a) Couple of force = | r  F |  [ML2 T 2 ],  , Work = [F.d ]  [ML2 T 2 ], (a) Quantities having different dimensions can only be divided or, multiplied but they cannot be added or subtracted., , 67., , 72., , (a), , E, , 1, mv 2  M [LT 1 ]2  [ML2 T  2 ], 2, 2, , (c), , dv, F   . A,  []  [ML1 T 1 ], dx, , 90., , 2, , 91., , (a) Angle of banking : tan  , , v2, v2, . i.e., is dimensionless., rg, rg

Page 22 :
Units, Dimensions and Measurement, 92., , (b) Solar constant is energy received per unit area per unit time i.e., 2, , 93., , 94., , 95., , (a), , [Cz ]  [M 0 L0 T 0 ]  Dimension less, , 2, , [ML T ],  [M 1 T  3 ], [L2 ] [T ], (b) From, the, principle, of, dimensional, F, F, , , [a]     [MLT  3 ] and [b]   2   [MLT  4 ], t, t , , B, have the same dimension, A, but x and A have the different dimensions., , x and B ; C and Z 1 ; y and, , homogenity, 105., , (c) Tension = [MLT 2 ] , Surface Tension = [MT 2 ], , K  Y  r0 = [ML1T 2 ]  [L] = [MT 2 ], , 106., , (d) Torque = [ML2T 2 ] , Moment of inertia = [ML2 ], , Y = Young's modulus and r0 = Interatomic distance, , 107., , (c) Angular momentum = [ML2T 1 ] , Frequency = [T 1 ], , 108., , (c) Latent Heat L , , 109., , (a), , 110., , R, 1 , (b) C 2 LR  [C 2 L2 ]     [T 4 ]     [T 3 ], L, T , , (b) Let [G]  c x g y p z, by substituting the following dimensions :, [G]  [M 1 L3 T 2 ], [c]  [LT 1 ], [g]  [LT 2 ], , [ p]  [ML1T 2 ], and by comparing the powers of both sides, we can get x  0, y  2, z  1, ,  [G]  c 0 g 2 p 1, 96., , (a) Let T  S x r y  z, 111., , [S ]  [MT 2 ], [r]  [L], [ ]  [ML3 ], , so T  r / S  T  k, (a) Resistivity [  ] , , Now comparing both sides we will get, x  1 / 2; y  1 / 2, z  1 / 2, , r 3, 112., , So m  c1 / 2G1 / 2h1 / 2, (d) Charge = Current  Time = [AT ], , 113., , (b), , S, , [R].[ A], where [R]  [ML2 T 1 Q 2 ], [l], , (a), , 99., , (c) Torque = [ML2T 2 ] , Angular momentum = [ML2T 1 ] So, mass and length have the same dimensions, (a) Let F  P x V y T z, by substituting the following dimensions :, and comparing the dimension of both sides, Energy, [ML2 T 2 ], ,  [LT  2 ], mass  length, [M ] [L], , 101., , (d), , 102., , (b) Let m  E x v y F z, By substituting the following dimensions :, [E]  [ML2T 2 ], [v]  [LT 1 ], [F]  [MLT 2 ], , 115., , (b), , 116., , (c)  , , 117., ,  F   MLT 2 ,  [MT 1 ], (d) F  v  F  kv  [k ]     , 1 ,  v   LT , , 118., , (d) e  L, , 119., , 1,  [L1 ], focal length, All quantities have dimensions, , R, k     [ML2 T  2 1 ], N, , 120., , (a), , 121., , (a) W , , From the dimensional homogenity, x, B, [ x ]  [ Ay]  [B]     [y]   , A,  A, , di,  A,  [e ]  [ML2 T  2 A  2 ]  , dt, T , , Power =, , x  1, y  2, z  0 . So [m]  [Ev ], x  Ay  B tan Cz, , d,  [T 1 ] and frequency [n]  [T 1 ], dt, , (d) [G]  [M 1 L3 T 2 ];[h]  [ML2 T 1 ], , 2, , (d), , Energy, ML2 T 2, ,  [ML1 T  2 ] = Pressure, Volume, L3, ,  ML2 T 2 , 2  2 1, [e ]  ,   [ML T Q ],  AT , , and by equating the both sides, (b), , v,  [T 1 ], z, , (a), , x  1, y  2, z  2 , so F  PV 2T 2, , 104., , v,  []  [ML1T 1 ], z, , 114., , [P]  [ML1T 2 ] [V ]  [LT 1 ], [T ]  [T ], , 103., , F  A, , As F  [MLT  2 ], A  [L2 ],, , Q [Q], ,  [M 0 L0 T 1Q], t, [T ], , 98., , 100., , LC  T, , [C]  LT 1 ; [G]  [M 1 L3 T 2 ] and [h]  [ML2T 1 ], ,  [ ]  [ML3 T 1Q 2 ], I, , Q, [ AT ], ,  [M 1 L 2T 4 A 2 ], V [ML2T  3 A 1 ], , (c) Let m  C x Gy hz, By substituting the following dimensions :, , and by comparing the power of both the sides, x  1 / 2, y  3 / 2, z  1 / 2, 3, , C, , Q Energy [ML2 T 2 ], , ,  [L2 T  2 ], m, mass, [M ], , L, As    T and, R, , by substituting the dimension of [T ]  [T ], , 97., , 65, , 1 2, [W ]  ML2 T 2 , 2, kx  [k ]  2  ,   [MT ], 2, [ x ]  L2 

Page 23 :
66 Units, Dimensions and Measurement, 122., , (c) Momentum [MLT 1 ] , Planck's constant [ML2 T 1 ], V  ML2 T 3 A 1 , 2 3 2, , R,  = [ML T A ], A, I, , , , 123., , (b), , 124., , (d) Relative density =, , 125., , (a), , 126., , (a) Let, , Densityof substance,  [M 0 L0 T 0 ], density of water, , n  k  a ab T c, , [ ]  [ML3 ], [a]  [L], , where, , (a), , 140., 141., 142., , (d), (d), (d) CGS, SI, N 1U1  N 2 U2, , , , k  1 / 2a3 / 2, 1, 3, 1, and c , , ,b , 2, 2, 2, T, , V, , W,  [ML2 T  2 Q 1 ], Q, , 127., , (a), , 128., , (b), , 129., , (c), , 130., , (c) Shear modulus =, , 131., , (d) Velocity gradient , , 132., , V [ML2 T 3 A 1 ], ,  [MLT 3 A 1 ], x, [L], 2, , 3., 4., , 135., , Dimension, , t  (dimensionless), , of, ,  , , 1, 1,   L1, X, L, , (b) As x  Ka m  t n, , M, , 0, , LT, , 0, ,   LT  T   L T, n, , m, , 2 m n, , (b), (b) Number of significant figures are 3, because 10 is decimal, multiplier., 4, (b)  V  r 3, 3, 3, ,  3  1 = 3%, (c) Mean time period T = 2.00 sec, , & Mean absolute error  T = 0.05 sec., To express maximum estimate of error, the time period should, be written as (2.00  0.05) sec, , hence, , , , (b) Here, S  (13.8  0.2) m, and t  (4.0  0.3) sec, ,  m  1 and 2m  n  0  n  2 ., , Expressing it in percentage error, we have,, S  13.8 , , (d), , NSm 2  Nm 2  S  Pascal-second., , 137., , (b), , E  KF a A b T c, , ML T   MLT  LT  T , ML T   M L T, , 2, , 2, , 2, , 2, , 2 a, , a a b, , 2 b, ,  E  KFAT ., 2, , 0.2,  100%  13.8  1 .4 %, 13.8, , and t  4.0 , , c, , 2 a  2b c, ,  a 1 , ab  2  b 1, and 2a  2b  c  2  c  2, (a), , 6., , 7., , 2 m, , 1, mv 2, 2, ,  % error is volume  3  % error in radius, ,  [K]  [L1 ], , 136., , 138., , 5., 2, , PV  ML1 T 2  L3 , 2  2 1, , (a)  R ,   [ML T  ], , T, , , , K, , (b)  E , , = % error in mass + 2 × % error in velocity, =2+2×3=8%, , P [ML1 T 2 ], ,  [ML 2 T  2 ], x, [L], , and comparing both sides, we get m  FL T, , [Kx ] =, , 2., , % Error in K.E., , (a) Let m  KF a Lb T c, Substituting the dimension of, , (c), , 3, , 4 2 l, T2, 1mm, 0.1, Here % error in l =,  100 ,  100  0.1%, 100cm, 100, 0.1, and % error in T =,  100  0.05%, 2  100,  % error in g = % error in l + 2(% error in T),  0.1  2  0.05 = 0.2 %, , (c), , E [ML T ], ,  [MLT  2 ], x, [L], , 1, , 134., ,  1 g   1cm ,  0 .625 , , ,  1kg   1m , , T  2 l/g  T 2  4 2 l/g  g , , 1., , 2, , [F]  [MLT 2 ], [C]  [L] and [T ]  [T ], , 133., , 3, , Errors of Measurement, , v [LT 1 ], ,  [T 1 ], x, [L], , and pressure gradient , , , ,  0.625  10 3  10 6  625, , Shearing stress, F, ,  [ML1 T 2 ], Shearing strain, A, , Energy gradient , , , , M  L ,  N 2  N1  1    1 ,  M 2   L2 , , L / R is a time constant so (R / L)  T 1, , Potential gradient , , , , N 1 M1 L13  N 2 M 2 L23, , and, , [T ]  [MT 2 ], Comparing both sides, we get, a, ,  , , h  ML2 T 1 , 1, ,  T, I  ML2 , , 139., , V , , 8., , 0.3,  100%  4  7.5 %, 4, , s 13.8  1.4, ,  (3.45  0.3) m / s., t, 4  7.5, , (c) % error in velocity = %error in L + %error in t, 0.2, 0.3,  100 ,  100, 13.8, 4, = 1.44 + 7.5 = 8.94 %, 

Page 24 :
Units, Dimensions and Measurement, 9., 10., , (c), (a), , T , , 1,  0 .05, 20, , 11., , (b), , 12., , 4, (b)  V  r 3, 3, , 19., ,  % error in volume,  3  % error in radius., , 13., , 14., 15., , 3  0 .1, ,  100, 5.3, (a) Since percentage increase in length = 2 %, Hence, percentage increase in area of square sheet, , , , , , (d) a  b c / d e, , 20., , 16., , 21., , 22., , Weight in water  (4.00  0.05) N, , , , 18., , 5, 0.2,  100 ,  100  (5  2)% = 7%, 100, 10, , (b) Average value , , 2.63  2.56  2.42  2.71  2.80, 5,  2.62 sec, , error, , 23., 24., , (d) Kinetic energy E , , 1, mv 2, 2, , E, v2  v 2,  100 ,  100, E, v2, , E,  100  125%, E, (c) Quantity C has maximum power. So it brings maximum error, in P., (c) Given, L  2.331 cm, ,  2.33 (correct upto two decimal places), and B  2.1 cm  2.10 cm,  L  B  2.33  2.10  4.43 cm .  4.4 cm, , 25., 26., 27., 28., , | T2 |  2.62  2.56  0.06, , Since minimum significant figure is 2., (d) The number of significant figures in all of the given number is, 4., (c), (a) Percentage error in X  a  b  c, (d) Percentage error in A, 1, , ,   2  1  3  3  1  2   2 %  14%, 2, , , , Critical Thinking Questions, , | T3 |  2.62  2.42  0.20, | T5 |  2.80  2.62  0.18, , Mean absolute error, , Y, , (b) H  I 2 R t, , Now | T1 |  2.63  2.62  0.01, , | T4 |  2.71  2.62  0.09, , in, , , , 5 .00  0 .05, 0 .1 , , , ,   100  5.0  (1  10)%, 1 .00  5 .00 1 .00 ,  5.0  11%, , 17., , permissible, ,  [(1.5)2  1]  100, , weight in air, Now relative density , weight loss in water, , V, I,  R, ,  100 , ,  100 ,  100, (b)  , V, I,  R,  max, , maximum, ,  M g L 2 D l , Y,  100  , , , ,    100, Y, g, L, D, l ,  M, , , , Loss of weight in water  (1.00  0.1) N, , 5 .00  0 .05, 1 .00  0 .1, Now relative density with max permissible error, , so, , D 2 l, , H,  2 I R t ,  100  , ,    100, H, R, t ,  I,  (2  3  4  6)%  16%, , (a) Weight in air  (5.00  0.05) N, , i.e. R . D , , 4 MgL, , , , d, e,  100   .,  100, d, e, ,  b1  c1  d1  e1 %, , Y , , 1, 1, 1, 1 ,  1, , , ,  2, ,   100, 300, 981, 2820, 41, 87, , ,  0.065  100  6.5%, , So maximum error in a is given by, ,  ., , (c), , =, , , , b, c,  a, ,  100 ,  .,  100   .,  100, , b, c,  a,  max, , (c) Volume of cylinder V  r 2 l, Percentage error in volume, V, 2 r, l,  100 ,  100   100, V, r, l, 0.01, 0.1, , ,  2,  100 ,  100   (1  2)% = 3 %, 2.0, 5 .0, , , ,  2  2% = 4%, (c) Since for 50.14 cm, significant number = 4 and for 0.00025,, significant numbers = 2, , , | T1 |  | T2 |  | T3 |  | T4 |  | T5 |, 5, , 0.54,  0.108  0.11sec, 5, , , , Decimal equivalent upto 3 significant figures is 0.0500, , 67, , 1, , 1., , L  T , (d) n 2  n1  1   1 ,  L 2   T2 , 1, , 2, , 1, ,  meter   sec ,  10 ,  , ,  km   hr , ,  m   sec , n 2  10  3  , ,  10 m   3600 sec , , 2, ,  129600, , 2

Page 25 :
68 Units, Dimensions and Measurement, 2., 3., , By substituting the dimension of [k ]  [L], , 1, C,    does not represent the dimension of, L, 2 LC, frequency, (d) [n] = Number of particles crossing a unit area in unit time =, [L2 T 1 ], , (d) f , , [h]  [ML2T 1 ], [c]  [LT 1 ], [G]  [M 1 L3 T 2 ], , and by comparing the power of both sides, we can get x  1 / 2, y  3 / 2, z  1 / 2, , n2   n1   number of particles per unit volume = [L ], , So dimension of radius of gyration are [h]1 / 2 [c]3 / 2 [G]1 / 2, , –3, , [x 2 ]  [x 1 ] = positions, , , , , , [n] x 2  x 1  L T  [L],  D, = L2 T 1, , n2  n1 , [L 3 ], , 4., , 2, , 1, , , , , , (c) We can derive this equation from equations of motion so it is, numerically correct., S t = distance travelled in t second =, th, , 10., 11., , (d) Y , , Distance,  [LT 1 ], time, ,  , , 1, a(2 t  1)  [LT 1 ], 2, As dimensions of each term in the given equation are same,, hence equation is dimensionally correct also., , 5., , (b, d) Length  G c h, y, , 1, 3, 1, ,y   ,z , 2, 2, 2, (d) By substituting the dimensions of mass [M], length [L] and, x, , 6., , , , 7., , 2, , , , torque is ML2 T 2, 8., , , , P2  F , F,   m  2 2, 4l 2  m , l , , 14., , (d)  Density,  , , , , , , , , , , M, M,  2, V, r L, , M, r L, 2, , M, r, L, , 0.003, 0.005 0.06,  2, , 0.3, 0.5, 6, ,  0.01  0.02  0.01  0.04, ,  Percentage error , 15., , , , , ,  100  0 .04  100  4 %, , (a), , Assertion and Reason, , Putting the dimensions in the above relation,  [M 0 L0 T 1 ]  [LT 1 ] x [M 1 L3 T 2 ]y [ML2 T 1 ]z,  [M 0 L0 T 1 ]  [M y  z Lx  3 y  2 z T  x  2 y  z ], , 1., 2., 3., , Comparing the powers of M, L and T, y  z  0, , …(i), , x  3y  2z  0, , …(ii), ,  x  2y  z  1, , …(iii), , On solving equations (i) and (ii) and (iii), 5, 1, ,y  z , 2, 2, , Hence dimension of time are [G1 / 2 h1 / 2 c 5 / 2 ], 9., ,  2 , , (a), , (a) Time  c x G y h z  T  kc x G y h z, , x, ,  [M  3 L 2 T 8 A 4 ], , z, should be dimensionless, k, , 1/2, , 13., , , , Curie and frequency of a light wave both have dimension, [T 1 ] . But dimensions of Planck's constant is [ML2 T 1 ] and, , [MT  2 A 1 ] 2, ,  MLT 2 ,  [m ]   2  2   [ML1 T 0 ],  L T , , M, coefficient of rigidity ML T, we get T  2, is the, L, right formula for time period of oscillations, (a, b, c) Reynolds number and coefficient of friction are, dimensionless., Latent heat and gravitational potential both have dimension, [L2T 2 ] ., 1, , M 1 L2 T 4 A 2, ,  , , [MLT 2 ],  [ ]    ,  [M 0 L2 T 0 ] ., 1  2, ,  p  [ML T ], , P F, 2l  m , , (c)  , , L= [M 1 L3 T 2 ]x [LT 1 ]y [ML2 T 1 ]z, By comparing the power of M, L and T in both sides we get,  x  z  0 , 3 x  y  2 z  1 and 2 x  y  z  0, By solving above three equations we get, , , , k, [ML2 T 2 K 1  K ],  [ ] ,  [MLT  2 ], z, [L], , and P , , 12., , z, , 3Z, , 2, , (a) In given equation,, , u = velocity = [LT 1 ] and, , x, , X, , (a) Let radius of gyration [k ]  [h]x [c]y [G]z, , 4., 5., , (c) Light year and wavelength both represents the distance, so, both has dimension of length not of time., (d) Light year measures distance and year measures time. One light, year is the distance traveled by light in one year., (a) Addition and subtraction can be done between quantities, having same dimension., (c) Density is not always mass per unit volume., (d) Rate of flow of liquid is expressed as the volume of liquid, flowing per second and it has dimension [L3 T 1 ]., , 6., 7., , (a), (a) As the distance of star increases, the parallax angle decreases,, and great degree of accuracy is required for its measurement., Keeping in view the practical limitation in measuring the, parallax angle, the maximum distance of a star we can measure, is limited to 100 light year.

Page 26 :
Units, Dimensions and Measurement, 8., , 9., 10., , 11., 12., 13., 14., , (c) Since zeros placed to the left of the number are never, significant, but zeros placed to right of the number are, significant., (b) The last number is most accurate because it has greatest, significant figure (3)., (a) As length, mass and time represent our basic scientific, notations, therefore they are called fundamental quantities and, they cannot be obtained from each other., (c) Because density can be derived from fundamental quantities., (c) Because representation of standard metre in terms of, wavelength of light is most accurate., (a) As radar is most accurate instrument used to detect aeroplane, in sky based on principle of reflection of radio waves., (c) As surface tension and surface energy both have different S.I., unit and same dimensional formula., , 15., , (c) As  (angular velocity) has the dimension of [T 1 ] not [T ] ., , 16., 17., , (e) Radian is the unit of plane angle., (b) A.U. is used (Astronomical units) to measure the average, distance of the centre of the sun from the centre of the earth,, while angstrom is used for very short distances. 1 A.U. =, 1.5  10 11 m; 1 Å  10 10 m., , 18., , 1, , 19., , 20., , 2, , constant  n  1 / u i.e., smaller the unit of measurement,, greater is its numerical value., (c) Dimensional constants are the quantities whose value are, constant and they posses dimensions. For example, velocity of, light in vacuum, universal gravitational constant, Boltzman, constant, Planck’s constant etc., (e) Let us write the dimension of various quantities on two sides of, the given relation., L.H.S.  T  [T ], R.H.S.  2 g / l , , LT 2,  [T 1 ], L, , (  2 has no dimension). As dimensions of L.H.S. is not equal, to dimension of R.H.S. therefore according to principle of, homogeneity the relation, T  2 g / l is not valid., , 21., , (b) From, f , or, m , , 1, 2l, , T, T, , f2  2, m, 4l m, , T, [MLT 2 ] M, Mass,  2 2 , , = linear mass, 2 2, L, length, 4l f, LT, , density., 22., , (a) According to statement of reason, as the graph is a straight, line, P  Q, or P = constant  Q, , i.e., 23., , P, = constant, Q, , (c) Avogadro number (N) represents the number of atoms in 1, gram mole of an element, i.e. it has the dimensions of mole ., -1, , 24., , (a) Unit of quantity (L/R) is Henry/ohm., , As Henry = ohm  sec, hence unit of L/R is sec i.e., [L/R] = [T]., Similarly, unit of product CR is farad  ohm or,, Coulomb Volt, Sec  Amp, or,, or, sec i.e. [CR] =, , Amp, Volt, Amp, , [T] therefore [L/R] and [CR] both have the same dimension., 25., , (b) Both assertion and reason are true but reason is not the, correct explanation of assertion., [ 0 ]  [M 1 L3 T 4 I 2 ] , [ 0 ]  [MLT 2 I 2 ], , , 1, ( 0 / 4 )  4E0, , , , 9  10 9,  9  10 16, 10  7, ,  3  10 8 m / s., , Therefore, , 1, , 0 0, , has dimension of velocity and numerically, , equal to velocity of light., , (c) We know that Q  n1 u1  n 2 u 2 are the two units of, measurement of the quantity Q and n , n are their respective, numerical values. From relation Q1  n1 u1  n 2 u 2 , nu =, , 69

Page 27 :
70 Units, Dimensions and Measurement, , 1., , The surface tension of a liquid is 70 dyne / cm . In MKS system its, value is, , From the equation tan  , , 9., , A dimensionally consistent relation for the volume V of a liquid of, coefficient of viscosity  flowing per second through a tube of, , [CPMT 1973, 74; AFMC 1996; BHU 2002], , 2., , (a), , 70 N/m, , (b) 7  10 2 N/m, , (c), , 7  10 3 N/m, , (d) 7  10 2 N/m, , The SI unit of universal gas constant (R) is, [MP Board 1988; JIPMER 1993; AFMC 1996;, MP PMT 1987, 94; CPMT 1984, 87; UPSEAT 1999], , (a), , W attK 1mol 1, , (b), , Newton K 1mol 1, , (c), , Joule K 1 mol 1, , (d), 3., , Erg K, , 1, , mol, , rg, , one can obtain the angle of, v2, banking  for a cyclist taking a curve (the symbols have their usual, meanings). Then say, it is, (a) Both dimensionally and numerically correct, (b) Neither numerically nor dimensionally correct, (c) Dimensionally correct only, (d) Numerically correct only, , 8., , radius r and length l and having a pressure difference p across, its end, is, , 1, , The unit of permittivity of free space  0 is, , (a) V , , pr 4, 8l, , (b) V , , (c) V , , 8 p l, r 4, , (d) V , , [MP PET 1993; MP PMT 2003; CBSE PMT 2004], , (a) Coulomb/Newton-metre, 2, , (b) Newton- metre /Coulomb, (c), , 4., , 10., 2, , Coulomb 2 /(Newton-metre)2, , (a), , The temperature of a body on Kelvin scale is found to be X K ., When it is measured by a Fahrenheit thermometer, it is found to be, , (b) a  LT 2 , b  LT , c  L, , X 0 F . Then X is, , (c), [UPSEAT 2000], , 5., , 301.25, 574.25, 313, 40, , 11., , (a), 6., , 7., , C N m, , 2, , (b), , 2, , Nm C, , 2, , (c) Nm 2C 2, (d) Unitless, The SI unit of surface tension is, (a) Dyne/cm, (b) Newton/cm, (c) Newton/metre, (d) Newton-metre, , a  LT 2 , b  L, c  T, , From the dimensional consideration, which of the following equation, is correct, [CPMT 1983], (a) T  2, , R3, GM, , (b) T  2, , GM, R3, , T  2, , GM, R2, , (d) T  2, , R2, GM, , (c), 12., [DCE 2003], , E, m, l and G denote energy, mass, angular momentum, , gravitational constant respectively, then the dimension of, are, (a) Angle, (c) Mass, , [AIIMS 1985], , (b) Length, (d) Time, , and, , El2, m 5G2, , 8lr4, , a  L2 , b  T , c  LT 2, , [AFMC 2004], 1, , p , , (d) a  L, b  LT , c  T 2, , What are the units of K  1/4 0, 2, , 8 pr 4, , The velocity v (in cm / sec ) of a particle is given in terms of time, b, t (in sec) by the relation v  at , ; the dimensions of a, b, tc, and c are, [CPMT 1990], , (d) Coulomb 2 / Newton-metre 2, , (a), (b), (c), (d), , l, , The position of a particle at time t is given by the relation, v , x (t)   0  (1  c t ) , where v 0 is a constant and   0 . The,  , dimensions of v 0 and  are respectively, [CBSE PMT 1995], 0 1, , 1, , and T, , 1, , (a), , M LT, , (b), , M 0 L1T 0 and T 1, , (c), , M 0 L1T 1 and LT 2, , (d), , M 0 L1T 1 and T

Page 28 :
Units, Dimensions and Measurement, 13., , The equation of state of some gases can be expressed as, a  R, , Where P is the pressure, V the volume, , P  2  , V, V , , the absolute temperature and a and b are constants. The, dimensional formula of a is, , 17., , [UPSEAT 2002; Orissa PMT 2004], , (a) [ML5 T 2 ], (c), 14., , 1, , [ML T, , 2, , (b) [M 1 L5 T 2 ], 5, , (d) [ML T, , ], , 2, , 18., , ], , a  t2, a, in the equation P , , where P is, bx, b, pressure, x is distance and t is time, are, 2, , (a), , MT, , (c), , ML3 T 1, , Dimensions of, , 1, , 100, 5.3, , (a), , 3  6 .01 , , (c), ,  3  0 .1 , ,   100,  5 .3 , , (b), , 1, 100,  0 .01 , 3, 5 .3, , (d), , 0.1,  100, 5 .3, , The pressure on a square plate is measured by measuring the force, on the plate and the length of the sides of the plate. If the maximum, error in the measurement of force and length are respectively 4%, and 2%, The maximum error in the measurement of pressure is, , 0 0, , 2, , (b), , M LT, , (d), , LT 3, , 3, , 19., , (b) [L1T ], , (c) [L2T 2 ], , (d) [L2T 2 ], , The dimensions of e / 4 0 hc , where e,  0 , h and c are, electronic charge, electric permittivity, Planck’s constant and velocity, of light in vacuum respectively [UPSEAT 2004], 2, , (a) [M 0 L0 T 0 ], , (b) [M 1 L0 T 0 ], , [M 0 L1T 0 ], , (d) [M 0 L0 T 1 ], , (a) 1%, (b) 2%, (c) 6%, (d) 8%, While measuring the acceleration due to gravity by a simple, pendulum, a student makes a positive error of 1% in the length of, the pendulum and a negative error of 3% in the value of time, period. His percentage error in the measurement of g by the, , , , , , relation g  4 2 l / T 2 will be, , [AIEEE 2003], , (a) [LT 1 ], , (c), , [CPMT 1993], , , where symbols have their usual meaning,, , are, , 16., , If radius of the sphere is (5.3  0.1) cm. Then percentage error in, its volume will be, [Pb. PET 2000], , The dimensions of, , [KCET 2003], , 15., , 71, , 20., , (a) 2%, (b) 4%, (c) 7%, (d) 10%, The length, breadth and thickness of a block are given by, l  12 cm, b  6 cm and t  2.45 cm, The volume of the block according to the idea of significant figures, should be, [CPMT 2004], (a) 1  10 2 cm 3, , (b) 2  10 2 cm 3, , (c) 1.763  10 2 cm 3, , (d) None of these, , (SET -1)

Page 29 :
72 Units, Dimensions and Measurement, , 1., , = [ML5 T 2 ], , (b) 1 dyne  10 5 Newton, 1 cm  10 2 m, 70, , dyne 70  10 5 N, , cm, m, 10  2, , 14., , (a) [a]  [T 2 ] and [b] , , = 7  10 2 N / m ., 2., , (c), , PV  nRT  R , , 3., , (d), , F, ,  [b]  [M 1 T 4 ], PV, Joule, ,  JK 1 mol 1, nT, mole  Kelvin, , Q Q, 1, . 12 2, 4 0, r, Q2, ,  0 , , F  r2, , [T 2 ], a, So   ,  [MT  2 ],  b  [M 1 T 4 ], , (b), , 5., , (b) Unit of  0  C 2 / N-m 2 Unit of K = Nm 2 C 2, , 6., , (c), , 7., , (a) [E]  [ML T ], [m]  [M ], [l]  [ML T ] and, , 2, , 2, , (a) [e ]  [ AT ], 0  [M 1 L3 T 4 A 2 ], [h]  [ML2 T 1 ], , ,  , , e2, A 2T 2, ,    1 3 4 2, 2 1, 1 , 4, , , hc,   M L T A  ML T  LT , 0, , ,  [M 0 L0 T 0 ], , 17., , 1, , M 3 L6 T 4, El 2 [ML2 T 2 ][ML2 T 1 ] 2, ,  [M 0 L0 T 0 ], 5 2, m G, [M 5 ][M 1 L3 T  2 ] 2, M 3 L6 T  4, , (c) Volume of sphere (V ) , , 18., , v2, ., rg, , 9., 10., , (c), , the, , principle, , of, , dimensional, , homogenity, , [v]  [at]  [a]  [LT 2 ] . Similarly [b]  [L] and [c]  [T ], R3, (a) By substituting the dimensions in T  2, GM, , we get, 12., , L3, T, M L T 2  M, , 13., , 1 3, , (a) Dimension of t = [M 0 L0 T 0 ]  [] = [T 1 ], v, Again  0, , , P, , 0 .1 , r, ,  100   3 ,   100, 5.3 , r, , , F F,  , so maximum error in pressure (P), A l2, , F, l,  P, ,  100 , ,  100  2  100, , P, F, l, , max, , 19., , (c) Percentage error in g = (%error in l) + 2(% error in T), 1% + 2(3%) = 7%, , 20., , (b) Volume V  l  b  t, , pr 4, , From, , (d), , 4 3, r, 3, , =4%+2×2%=8%, , pr 4, (a) Formula for viscosity  , V , 8 Vl, 8l, , 11., , % error in volume  3 , , (c) Given equation is dimensionally correct because both sides are, dimensionless but numerically wrong because the correct, equation is tan  , , 0 0, , and [c]  [LT 1 ], , [G]  [M 1 L3 T 2 ] Substituting the dimension of above, quantities in the given formula :, , 8., ,  c 2  [L2 T  2 ], , 16., , 0 0, , , , 1, , (d) C , , F  32 K  273, x  32 x  273, , , ,  x  574.25, 9, 5, 9, 5, , 4., , 1, , 15., , So  0 has units of Coulomb 2 / Newton-m 2, , 2, , [a  t 2 ], T2, , 1  2, [P] [ x ] [ML T ][L], , , 1,   [L] so [v 0 ]  [LT ], , , (a) By the principle of dimensional homogenity,  a , [P]   2   [a]  [P]  [V 2 ]  [ML1T  2 ] [L6 ], V , , =, ,  12  6  2.45  176.4 cm 3, , V  1.764  10 2 cm 3, since, the minimum number of significant figure is one in, breadth, hence volume will also contain only one significant, figure. Hence, V  2  10 2 cm 3 .